Topic square root of 180 simplified: Unlock the secrets of simplifying the square root of 180 with our comprehensive guide. Learn the step-by-step process, understand the mathematical properties, and explore practical applications. Whether you're a student or a math enthusiast, this guide will make the concept clear and easy to grasp.
Table of Content
- Simplifying the Square Root of 180
- Introduction to Square Roots
- Understanding the Square Root of 180
- Prime Factorization Method
- Step-by-Step Simplification Process
- Mathematical Properties of the Square Root of 180
- Examples of Simplifying Similar Square Roots
- Common Mistakes to Avoid
- Practical Applications of Square Roots
- FAQs About Square Roots
- Additional Resources and References
- YOUTUBE: Video hướng dẫn cách đơn giản hóa căn bậc hai của 180 một cách dễ hiểu và chi tiết. Thích hợp cho học sinh và những người yêu thích toán học.
Simplifying the Square Root of 180
The process of simplifying the square root of 180 involves breaking down the number into its prime factors and then simplifying accordingly.
Step-by-Step Simplification
- Find the prime factorization of 180:
- Group the prime factors into pairs:
- Take one factor out of each pair:
- Simplify the expression:
180 = 2 × 2 × 3 × 3 × 5
\( \sqrt{180} = \sqrt{2 \times 2 \times 3 \times 3 \times 5} \)
\( \sqrt{2 \times 2} \times \sqrt{3 \times 3} \times \sqrt{5} \)
\( 2 \times 3 \times \sqrt{5} \)
Conclusion
Therefore, the simplified form of the square root of 180 is \( 6\sqrt{5} \).
Properties of the Square Root of 180
- Perfect Square: 180 is not a perfect square.
- Irrational Number: The simplified form \( 6\sqrt{5} \) indicates that the square root of 180 is an irrational number.
- Decimal Form: The approximate decimal form of \( 6\sqrt{5} \) is 13.416.
Verification
You can verify the simplification by squaring \( 6\sqrt{5} \):
\( (6\sqrt{5})^2 = 36 \times 5 = 180 \)
This confirms that the simplified form \( 6\sqrt{5} \) is correct.

READ MORE:
Introduction to Square Roots
The concept of square roots is fundamental in mathematics. The square root of a number is a value that, when multiplied by itself, gives the original number. For instance, the square root of 180 is a number which, when squared, results in 180. Mathematically, this is represented as:
\[
\sqrt{180} \times \sqrt{180} = 180
\]
Square roots are denoted using the radical symbol (√), followed by the number for which we want to find the square root. In this context, √180 signifies the square root of 180.
Square roots can be perfect or imperfect. A perfect square root results in an integer, while an imperfect or irrational square root results in a non-repeating, non-terminating decimal. For example, the square root of 16 is 4, a perfect square, while the square root of 180 is approximately 13.416, an irrational number.
Understanding square roots involves familiarity with various methods to compute them:
- Prime Factorization Method: This method involves breaking down the number into its prime factors and simplifying the square root by grouping the prime factors.
- Long Division Method: A systematic approach to find the square root by dividing the number in a manner similar to long division.
- Repeated Subtraction Method: Involves subtracting consecutive odd numbers from the given number until zero is reached.
For example, the prime factorization of 180 is \(2 \times 2 \times 3 \times 3 \times 5\). Grouping the factors in pairs, we get:
\[
\sqrt{180} = \sqrt{(2 \times 2) \times (3 \times 3) \times 5} = \sqrt{4 \times 9 \times 5} = 2 \times 3 \times \sqrt{5} = 6\sqrt{5}
\]
This shows that the simplified form of the square root of 180 is \(6\sqrt{5}\).
Square roots have various applications in real life, including in areas such as geometry, physics, and finance. They are essential for calculating areas, understanding quadratic equations, and determining standard deviations in statistics.
Understanding the Square Root of 180
The square root of 180 is an interesting number to explore due to its properties and the methods used to simplify it. The square root of 180, denoted as √180, can be simplified to its most basic form using the prime factorization method.
Prime Factorization Method:
- First, find the prime factors of 180. This can be done by continuously dividing 180 by its smallest prime factor:
- 180 ÷ 2 = 90
- 90 ÷ 2 = 45
- 45 ÷ 3 = 15
- 15 ÷ 3 = 5
- 5 ÷ 5 = 1
- Write the prime factorization of 180:
180 = 2 × 2 × 3 × 3 × 5
- Group the prime factors into pairs:
(2 × 2) × (3 × 3) × 5
- Take one factor from each pair and bring it out of the square root:
√(2 × 2) × √(3 × 3) × √5 = 2 × 3 × √5
- Multiply the factors outside the square root:
2 × 3 = 6
Therefore, the simplified form of √180 is 6√5.
Decimal Approximation:
The square root of 180 in decimal form is approximately 13.416. This can be useful for practical applications where an approximate value is sufficient.
Mathematical Properties:
- √180 is an irrational number, meaning it cannot be expressed as a simple fraction.
- In exponential form, √180 is written as 1801/2.
- The exact form of √180 in its simplest radical form is 6√5.
Understanding the square root of 180 and its simplification process helps in various mathematical calculations and provides a clear example of how prime factorization can be used to simplify square roots.
Prime Factorization Method
The prime factorization method is a systematic way to simplify the square root of a number by breaking it down into its prime factors. Let's go through the process of simplifying the square root of 180 using this method step-by-step.
- Find the Prime Factors:
First, we need to find the prime factors of 180. We start with the smallest prime number, which is 2, and divide 180 by 2.
- 180 ÷ 2 = 90
- 90 ÷ 2 = 45
- 45 ÷ 3 = 15
- 15 ÷ 3 = 5
- 5 ÷ 5 = 1
So, the prime factors of 180 are 2, 2, 3, 3, and 5.
- Group the Prime Factors:
Next, we group the prime factors in pairs of the same number:
\(180 = 2 \times 2 \times 3 \times 3 \times 5\)
- Extract the Square Roots:
We can now extract the square roots of the paired numbers:
\(\sqrt{180} = \sqrt{(2 \times 2) \times (3 \times 3) \times 5} = \sqrt{2^2 \times 3^2 \times 5}\)
This simplifies to:
\(\sqrt{180} = 2 \times 3 \times \sqrt{5} = 6\sqrt{5}\)
Thus, the simplified form of the square root of 180 is \(6\sqrt{5}\).
| Prime Factors | Grouped Factors | Simplified Form |
| 2, 2, 3, 3, 5 | (2 × 2), (3 × 3), 5 | 6√5 |
Step-by-Step Simplification Process
The square root of 180 can be simplified by following these detailed steps:
- Prime Factorization:
First, find the prime factors of 180. Prime factorization involves breaking down 180 into its prime components.
180 = 2 × 90
180 = 2 × 2 × 45
180 = 2 × 2 × 3 × 15
180 = 2 × 2 × 3 × 3 × 5
Therefore, 180 can be expressed as 22 × 32 × 5.
- Group the Factors:
Group the factors into pairs of equal factors.
√180 = √(22 × 32 × 5)
- Simplify the Radical:
Take one factor from each pair out of the square root.
√(22) = 2
√(32) = 3
So, √180 = 2 × 3 × √5 = 6√5
- Result:
The simplified form of √180 is 6√5.
In decimal form, the square root of 180 is approximately 13.416.
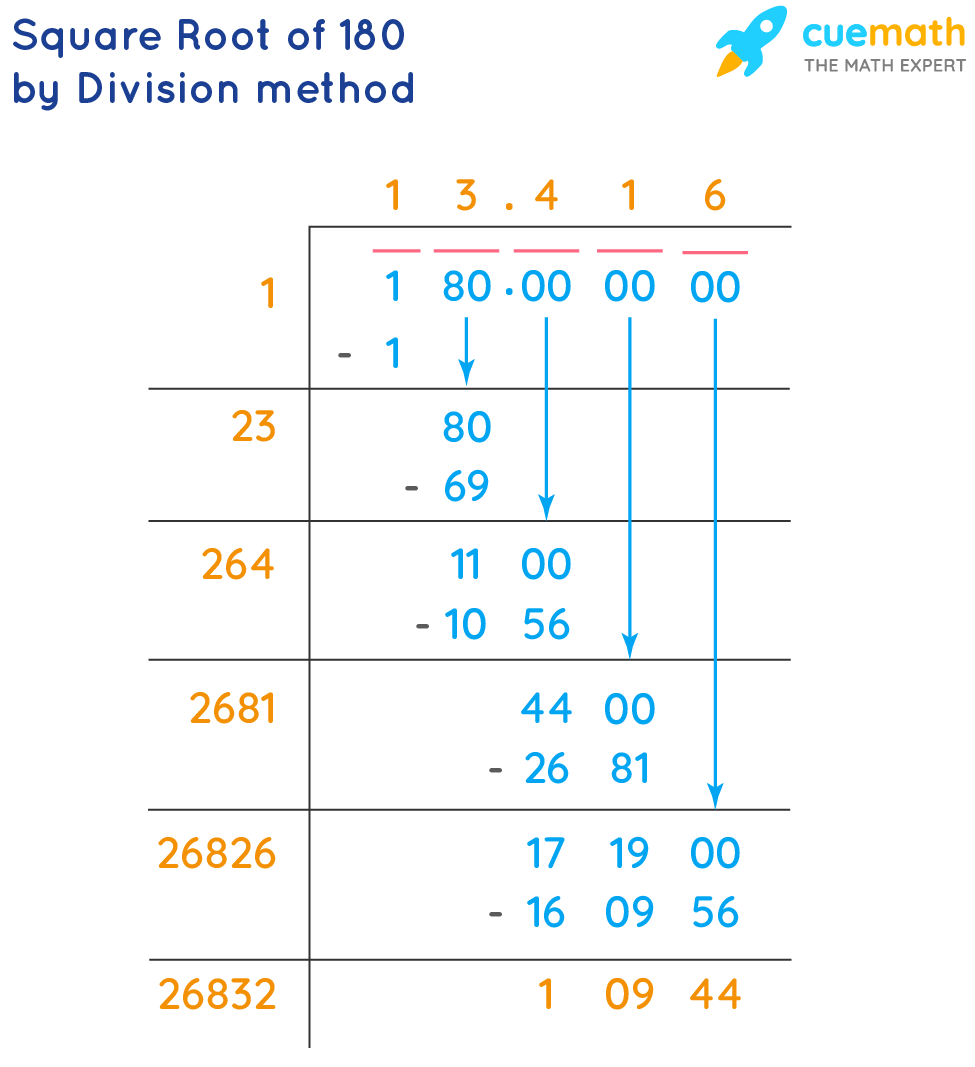
Mathematical Properties of the Square Root of 180
The square root of 180, denoted as \( \sqrt{180} \), possesses several interesting mathematical properties. Here, we will explore these properties in detail.
- Radical Form: The square root of 180 can be simplified into its radical form as \( 6\sqrt{5} \). This is derived from the prime factorization of 180, where 180 is factored into prime numbers.
- Irrational Number: \( \sqrt{180} \) is an irrational number. This means it cannot be expressed as a simple fraction and its decimal representation is non-repeating and non-terminating. The approximate value of \( \sqrt{180} \) is 13.416.
- Exponential Form: In exponential notation, the square root of 180 is written as \( 180^{1/2} \).
- Decimal Form: When expressed as a decimal, \( \sqrt{180} \approx 13.416 \). For practical purposes, it can be rounded to different decimal places:
- To the nearest tenth: 13.4
- To the nearest hundredth: 13.42
- To the nearest thousandth: 13.416
Step-by-Step Simplification Process
To understand why \( \sqrt{180} \) simplifies to \( 6\sqrt{5} \), we can follow the prime factorization method:
- First, find the prime factors of 180: \( 180 = 2^2 \times 3^2 \times 5 \).
- Express 180 under the square root sign: \( \sqrt{180} = \sqrt{2^2 \times 3^2 \times 5} \).
- Apply the product rule for square roots: \( \sqrt{a \times b} = \sqrt{a} \times \sqrt{b} \).
- Simplify the expression: \( \sqrt{2^2 \times 3^2 \times 5} = \sqrt{2^2} \times \sqrt{3^2} \times \sqrt{5} \).
- Calculate the square roots of the perfect squares: \( \sqrt{2^2} = 2 \) and \( \sqrt{3^2} = 3 \).
- Multiply these results together: \( 2 \times 3 = 6 \).
- Combine the simplified parts: \( \sqrt{180} = 6\sqrt{5} \).
These properties highlight the intriguing nature of square roots, especially when dealing with non-perfect squares like 180.
Examples of Simplifying Similar Square Roots
Simplifying square roots involves finding the simplest radical form of a number. Below are step-by-step examples for various square roots similar to √180:
Example 1: Simplifying √50
To simplify √50:
- Find the prime factorization of 50:
- 50 = 2 × 25
- 25 = 5 × 5
- Therefore, 50 = 2 × 5 × 5
- Group the factors into pairs:
- (5 × 5)
- Rewrite the square root:
- √50 = √(2 × 5²) = 5√2
Example 2: Simplifying √72
To simplify √72:
- Find the prime factorization of 72:
- 72 = 2 × 36
- 36 = 6 × 6
- 6 = 2 × 3
- Therefore, 72 = 2 × 2 × 2 × 3 × 3
- Group the factors into pairs:
- (2 × 2) and (3 × 3)
- Rewrite the square root:
- √72 = √(2² × 2 × 3²) = 6√2
Example 3: Simplifying √98
To simplify √98:
- Find the prime factorization of 98:
- 98 = 2 × 49
- 49 = 7 × 7
- Therefore, 98 = 2 × 7 × 7
- Group the factors into pairs:
- (7 × 7)
- Rewrite the square root:
- √98 = √(2 × 7²) = 7√2
Example 4: Simplifying √200
To simplify √200:
- Find the prime factorization of 200:
- 200 = 2 × 100
- 100 = 10 × 10
- 10 = 2 × 5
- Therefore, 200 = 2 × 2 × 2 × 5 × 5
- Group the factors into pairs:
- (2 × 2) and (5 × 5)
- Rewrite the square root:
- √200 = √(2² × 2 × 5²) = 10√2
Example 5: Simplifying √245
To simplify √245:
- Find the prime factorization of 245:
- 245 = 5 × 49
- 49 = 7 × 7
- Therefore, 245 = 5 × 7 × 7
- Group the factors into pairs:
- (7 × 7)
- Rewrite the square root:
- √245 = √(5 × 7²) = 7√5
These examples illustrate how to simplify square roots by prime factorization and grouping the factors into pairs to find the simplest radical form.
Common Mistakes to Avoid
Simplifying square roots can be tricky, and it's easy to make mistakes. Here are some common pitfalls and how to avoid them:
-
Incorrect Prime Factorization:
Make sure you correctly break down the number into its prime factors. For example, the prime factors of 180 are \(2 \times 2 \times 3 \times 3 \times 5\). Incorrect factorization can lead to wrong simplification results.
-
Forgetting to Pair Factors:
When simplifying, group the prime factors in pairs. Each pair of identical factors can be taken out of the square root. For \( \sqrt{180} \), this is \( \sqrt{(2 \times 2) \times (3 \times 3) \times 5} = 6\sqrt{5} \).
-
Incorrect Simplification of Mixed Radicals:
Ensure that only the correct pairs are simplified and the remaining factors stay under the square root. For example, don't simplify \( \sqrt{5} \) further as it does not have a pair.
-
Ignoring Irrational Numbers:
Recognize that the square root of a non-perfect square will be irrational. For instance, \( \sqrt{180} = 6\sqrt{5} \), and \( \sqrt{5} \) remains an irrational number.
-
Misapplying Properties of Radicals:
Remember that \( \sqrt{a \times b} = \sqrt{a} \times \sqrt{b} \) holds true, but \(\sqrt{a + b} \ne \sqrt{a} + \sqrt{b} \). Ensure you apply the correct properties when simplifying.
-
Errors in Arithmetic:
Double-check your calculations when performing arithmetic operations on the factors. Small mistakes can lead to incorrect simplification.
-
Misunderstanding the Final Form:
When you arrive at the simplified form, make sure it is in the simplest form possible. For \( \sqrt{180} \), the simplified form is \( 6\sqrt{5} \), not \( 2 \times 3\sqrt{5} \).
By being aware of these common mistakes, you can avoid them and simplify square roots correctly.
Practical Applications of Square Roots
The concept of square roots extends beyond theoretical mathematics and finds various practical applications in different fields. Here are some key areas where square roots, such as the square root of 180, play an essential role:
- Architecture and Engineering: Square roots are crucial for calculating distances and designing elements. For example, to find the diagonal length of a square plot with an area of 180 square meters, you would use the square root of 180, which is approximately 13.416 meters.
- Statistics: In statistics, the square root is used to calculate the standard deviation, a measure of the spread of a set of values. If a dataset has a variance of 180, the standard deviation would be the square root of 180, or approximately 13.416.
- Physics: Square roots are often involved in calculations related to force, energy, and motion. For instance, in kinematics, the square root of 180 might be used to determine velocity or acceleration in certain scenarios.
- Education: Square roots are commonly used in educational settings to help students understand mathematical properties and improve problem-solving skills. Exercises involving the square root of 180 can be found in algebra, geometry, and calculus curricula.
- Accident Investigations: Investigators use square roots to determine the speed of a vehicle from the length of skid marks. If the skid marks measure a certain length, the speed can be calculated using the square root of a related formula.
Understanding the practical applications of square roots helps bridge the gap between theoretical math and real-world problem-solving, highlighting their significance in various domains.

FAQs About Square Roots
-
What is a square root?
The square root of a number is a value that, when multiplied by itself, gives the original number. For example, the square root of 180 is a number \( x \) such that \( x \times x = 180 \).
-
Is the square root of 180 a rational number?
No, the square root of 180 is not a rational number. It is an irrational number because it cannot be expressed as a simple fraction. Its decimal representation is non-terminating and non-repeating, approximately equal to 13.416.
-
How do you simplify the square root of 180?
To simplify the square root of 180, you can use the prime factorization method:
- Find the prime factors of 180: \( 180 = 2 \times 2 \times 3 \times 3 \times 5 \).
- Group the prime factors into pairs: \( (2 \times 2) \) and \( (3 \times 3) \).
- Take one factor from each pair and multiply: \( 2 \times 3 = 6 \).
- Write the simplified form: \( \sqrt{180} = 6\sqrt{5} \).
-
How do you find the square root of 180 using the long division method?
Here are the steps for finding the square root of 180 using the long division method:
- Group the digits of 180 into pairs, starting from the decimal point: \( 1|80.00 \).
- Find the largest digit \( x \) such that \( x \times x \leq 1 \). The first digit is 1.
- Subtract the square of the digit from the number and bring down the next pair of digits.
- Double the first digit to get the new divisor and find the next digit of the square root by trial and error.
- Continue the process until you reach the desired precision.
-
What are some practical applications of square roots?
Square roots are used in various real-life applications, such as calculating areas, distances, and in fields like engineering, physics, and finance.
-
How can I verify my calculation of a square root?
You can verify your calculation by squaring the obtained square root value. If the result is the original number, then your calculation is correct.
Additional Resources and References
For those looking to delve deeper into the topic of square roots, particularly the square root of 180, here are some valuable resources and references:
- - This resource provides a step-by-step guide to simplifying the square root of 180 using various methods such as prime factorization and long division.
- - Explore detailed explanations and examples of how to find the square root of 180, including interactive questions and solved examples.
- - Byju's offers an in-depth look at different methods to calculate the square root of 180, including prime factorization, long division, and repeated subtraction methods.
- - This site provides a calculator for finding the square root of 180 and explains the steps for simplifying it through prime factorization.
- - Learn how to calculate the square root of 180 manually and using tools like Excel and Google Sheets.
These resources will help you understand the concept of square roots better and provide practical examples and applications of square root calculations.
Video hướng dẫn cách đơn giản hóa căn bậc hai của 180 một cách dễ hiểu và chi tiết. Thích hợp cho học sinh và những người yêu thích toán học.
Đơn giản hóa căn bậc hai của 180 - Hướng dẫn chi tiết
READ MORE:
Video hướng dẫn cách đơn giản hóa căn bậc hai của 180 một cách dễ hiểu và chi tiết. Thích hợp cho học sinh và những người yêu thích toán học.
Đơn giản hóa căn bậc hai của 180