Topic simplify the square root of 20: Unlock the secrets to simplifying the square root of 20 with our easy-to-follow guide. Whether you're a student or just looking to refresh your math skills, this article will walk you through the process step by step, ensuring you understand each part of the simplification method. Master the art of simplifying square roots today!
Table of Content
- How to Simplify the Square Root of 20
- Introduction to Square Roots
- Understanding the Radical Symbol
- Basics of Simplifying Square Roots
- Methods to Simplify the Square Root of 20
- Prime Factorization Method
- Long Division Method
- Examples and Practice Problems
- Common Mistakes to Avoid
- Advanced Techniques and Concepts
- Applications in Real Life
- Additional Resources and References
- YOUTUBE: Xem video hướng dẫn chi tiết về cách rút gọn căn bậc hai của 20 (sqrt(20)). Nhận biết xem liệu video này phù hợp với bài viết về đề tài rút gọn căn bậc hai của 20 hay không.
How to Simplify the Square Root of 20
Simplifying the square root of 20 involves expressing it in its simplest radical form. Follow these steps to simplify:
Step-by-Step Simplification Process
-
Identify the factors of 20. The number 20 can be factored into:
\[ 20 = 2 \times 10 \]
\[ 10 = 2 \times 5 \]
So, \( 20 \) can be written as:
\[ 20 = 2^2 \times 5 \]
-
Apply the square root to each factor:
\[ \sqrt{20} = \sqrt{2^2 \times 5} \]
-
Separate the factors under the square root:
\[ \sqrt{20} = \sqrt{2^2} \times \sqrt{5} \]
-
Simplify the square root of the perfect square:
\[ \sqrt{2^2} = 2 \]
-
Combine the simplified terms:
\[ \sqrt{20} = 2 \sqrt{5} \]
Conclusion
The simplified form of the square root of 20 is:
This form is the simplest radical form and cannot be simplified further.

READ MORE:
Introduction to Square Roots
Square roots are mathematical operations that find the original number which, when multiplied by itself, gives the desired value. The symbol for the square root is √, and it is used to denote the principal square root of a number. Understanding square roots is fundamental in algebra and helps simplify expressions involving radical terms.
Here is an essential property of square roots:
- If a and b are non-negative, then √(ab) = √a * √b.
For example, consider the square root of 20:
We start by breaking down 20 into its prime factors:
- List the factors of 20: 1, 2, 4, 5, 10, 20.
- Identify the perfect squares from the factors: 1, 4.
- Divide 20 by the largest perfect square: 20 ÷ 4 = 5.
- Take the square root of the perfect square: √4 = 2.
- Combine these results to get the simplified form: √20 = 2√5.
This simplification process makes it easier to work with square roots in equations and other mathematical contexts.
Understanding the Radical Symbol
The radical symbol (√) represents the square root operation. It is used to denote the principal square root of a number, which is the positive number that, when multiplied by itself, equals the original number. For example, the square root of 9 is 3, because 3 * 3 = 9.
The radical symbol can be used for various mathematical operations, such as simplifying square roots. Simplifying a square root involves expressing it in the simplest form. This is often done by factoring the number inside the radical to identify perfect squares.
- Identify the perfect square factor: For example, to simplify √20, first recognize that 20 can be factored into 4 and 5, where 4 is a perfect square.
- Separate the factors: Rewrite √20 as √(4 × 5).
- Apply the property of square roots: √(4 × 5) = √4 × √5.
- Simplify the square root of the perfect square: √4 = 2.
- Combine the results: Therefore, √20 simplifies to 2√5.
This process of simplification makes it easier to work with square roots in various mathematical contexts, such as algebraic equations and calculus.
Here is a summary table for visual understanding:
| Step | Expression |
|---|---|
| Original | √20 |
| Factorization | √(4 × 5) |
| Separate | √4 × √5 |
| Simplify | 2√5 |
Understanding the radical symbol and the process of simplification is crucial in mathematics, as it aids in solving equations and understanding the properties of numbers more deeply.
Basics of Simplifying Square Roots
Understanding how to simplify square roots is a fundamental concept in mathematics. Simplifying square roots involves expressing the square root of a number in its simplest form. Here, we will explore the step-by-step process of simplifying the square root of a non-perfect square number, such as 20.
-
Identify the Prime Factors:
Start by finding the prime factors of the number inside the square root. For 20, the prime factors are:
\(20 = 2 \times 2 \times 5\)
-
Group the Factors:
Next, group the prime factors into pairs. Each pair of identical factors can be taken out of the square root:
\(\sqrt{20} = \sqrt{2 \times 2 \times 5} = \sqrt{2^2 \times 5}\)
-
Simplify the Square Root:
Take the square root of each pair of identical factors and move them outside the radical symbol:
\(\sqrt{2^2} \times \sqrt{5} = 2\sqrt{5}\)
Therefore, the simplified form of \(\sqrt{20}\) is \(2\sqrt{5}\).
This method can be applied to simplify the square root of any number by breaking it down into its prime factors and simplifying the expression step by step.
Methods to Simplify the Square Root of 20
Simplifying the square root of 20 can be done using two primary methods: the Prime Factorization Method and the Long Division Method.
Prime Factorization Method
Prime factorization involves breaking down the number inside the square root into its prime factors. Here are the steps:
- First, factorize 20 into its prime factors:
\[ 20 = 2 \times 2 \times 5 \]
- Next, express the square root of 20 using these prime factors:
\[ \sqrt{20} = \sqrt{2 \times 2 \times 5} \]
- Since 2 is a perfect square, take the square root of 2 squared out of the radical:
\[ \sqrt{2 \times 2 \times 5} = 2 \sqrt{5} \]
- Thus, the simplest form of \(\sqrt{20}\) is:
\[ \sqrt{20} = 2\sqrt{5} \]
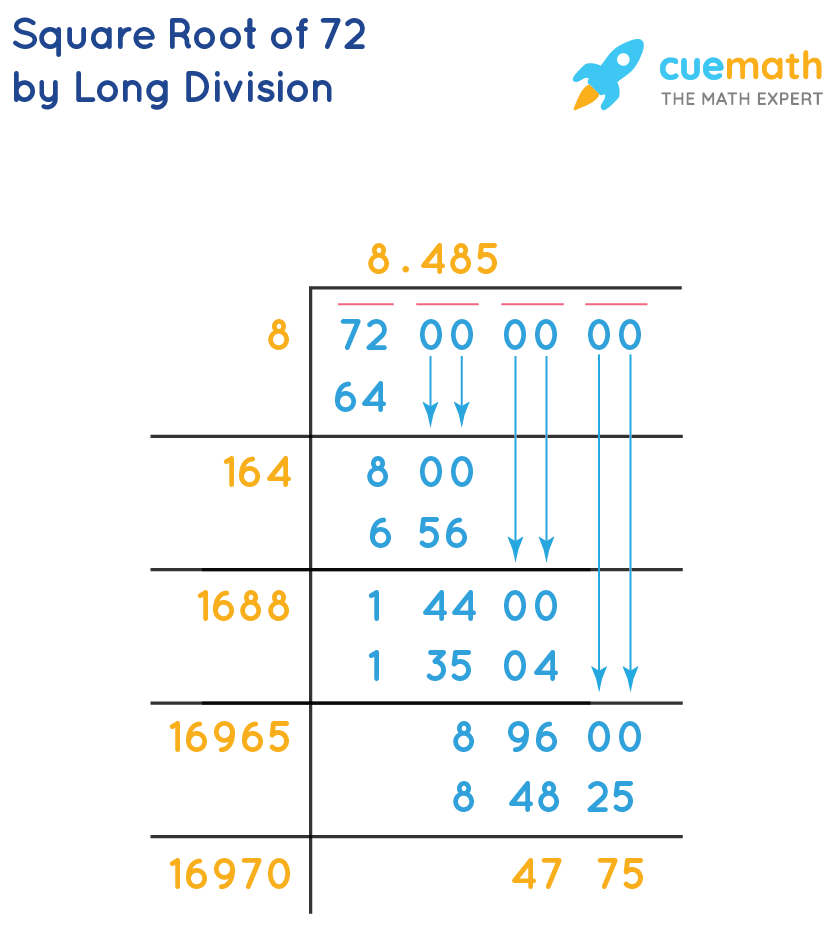
Long Division Method
The long division method is another technique to find the square root of a number. This method involves more steps but can be used to find an accurate decimal value of the square root. Here are the steps:
- Set up the number 20 in pairs of digits from right to left.
- Find the largest number whose square is less than or equal to the first pair (in this case, 20). This number is 4, because \(4^2 = 16\).
- Subtract 16 from 20 to get a remainder of 4, and bring down the next pair of digits (if any).
- Double the number obtained in step 2 (which is 4) to get 8. This becomes the starting digit for the next divisor.
- Determine the largest digit (X) such that \(8X \times X\) is less than or equal to the new dividend (which is 400 in this example).
- Continue this process to find more decimal places of the square root of 20.
Using this method, the approximate value of \(\sqrt{20}\) is 4.472.
By using either of these methods, we can simplify the square root of 20 accurately and understand the underlying process.

Prime Factorization Method
The Prime Factorization Method is a systematic way to simplify the square root of a number by breaking it down into its prime factors. Here is a detailed step-by-step process to simplify the square root of 20 using prime factorization:
-
First, we need to find the prime factors of 20. The prime factors of 20 are:
\[ 20 = 2 \times 2 \times 5 \]
-
Next, express the square root of 20 using these prime factors:
\[ \sqrt{20} = \sqrt{2 \times 2 \times 5} \]
-
Identify and group the pairs of prime factors. In this case, we have one pair of 2s:
\[ \sqrt{2 \times 2 \times 5} = \sqrt{(2 \times 2) \times 5} \]
-
Take the square root of the paired prime factors. The square root of \(2 \times 2\) is 2:
\[ \sqrt{(2 \times 2) \times 5} = 2 \times \sqrt{5} \]
-
Therefore, the simplest form of \(\sqrt{20}\) is:
\[ \sqrt{20} = 2\sqrt{5} \]
This method shows how breaking down the number into its prime factors can help simplify the square root. The result, \(2\sqrt{5}\), is the simplified form of \(\sqrt{20}\).
Long Division Method
The long division method is a systematic way to find the square root of a number by iteratively guessing and refining the quotient. Here is the step-by-step process to simplify the square root of 20 using this method:
- Preparation:
Write 20 as 20.000000, grouping digits in pairs from the decimal point. For 20, it looks like "20.00 00 00".
- Find the Largest Square:
Identify the largest square number smaller than or equal to 20, which is 16 (42). Write 4 above the line as the first digit of the root.
4 16 | 20.00 00 00 --- 4 - Subtract and Bring Down:
Subtract 16 from 20 to get 4, then bring down the next pair of zeros to make it 400.
- Double and Find the Next Digit:
Double the current result (4) to get 8. Now find a digit (X) such that 80X multiplied by X is less than or equal to 400. Here, X is 4, because 84 × 4 = 336.
4.4 16 | 20.00 00 00 --- 400 336 ---- 64 - Repeat for Precision:
Subtract 336 from 400 to get 64, then bring down the next pair of zeros to get 6400. Double the quotient (44) to get 88. Choose a digit (Y) so that 880Y multiplied by Y is just under 6400. Here, Y is 7, because 887 × 7 = 6209.
4.47 16 | 20.00 00 00 --- 400 336 ---- 6400 6209 ---- 191 - Continue for Desired Accuracy:
Repeat the process until you reach the desired level of accuracy. For the square root of 20, continuing this process gives us about 4.472 as we extend the division.
Examples and Practice Problems
Here are some examples and practice problems to help you understand how to simplify the square root of 20. Follow the step-by-step process for each example.
Example 1: Simplifying the Square Root of 20
- Start with the expression: \( \sqrt{20} \)
- Factor 20 into its prime factors: \( 20 = 2^2 \times 5 \)
- Rewrite the square root using these factors: \( \sqrt{20} = \sqrt{2^2 \times 5} \)
- Separate the perfect square from the rest: \( \sqrt{2^2 \times 5} = \sqrt{2^2} \times \sqrt{5} \)
- Simplify the square root of the perfect square: \( \sqrt{2^2} = 2 \)
- Combine the results: \( 2 \sqrt{5} \)
Therefore, \( \sqrt{20} = 2 \sqrt{5} \).
Practice Problems
Try simplifying the following square roots. Use the steps provided above to help you:
- \( \sqrt{50} \)
- \( \sqrt{72} \)
- \( \sqrt{98} \)
Solutions
- \( \sqrt{50} = 5 \sqrt{2} \)
- \( \sqrt{72} = 6 \sqrt{2} \)
- \( \sqrt{98} = 7 \sqrt{2} \)
By practicing these problems, you'll become more comfortable with the process of simplifying square roots.
Common Mistakes to Avoid
When simplifying square roots, there are several common mistakes that can lead to incorrect results. Being aware of these pitfalls can help ensure accuracy and confidence in your calculations.
-
Forgetting to Check for Perfect Squares:
Always check if the number inside the radical (the radicand) can be factored into a perfect square. Simplifying square roots often involves identifying these perfect squares to break down the expression.
For example, in simplifying , recognizing that 4 is a perfect square factor of 20 helps simplify the expression correctly.
-
Rushing Through the Simplification Process:
Simplification should be a step-by-step process. Rushing can cause errors, especially when dealing with more complex radicands. Take your time to ensure each step is performed correctly.
Carefully write out each factor and perform the simplification methodically to avoid mistakes.
-
Ignoring the Product and Quotient Rules:
Using the product rule and the quotient rule correctly is crucial for simplifying square roots. Misapplying these rules can lead to incorrect results.
-
Incorrectly Simplifying Non-Perfect Squares:
When dealing with non-perfect squares, it’s important to correctly identify and simplify them. Misidentifying factors or simplifying incorrectly can yield the wrong result.
-
Not Rationalizing the Denominator:
In expressions with radicals in the denominator, always rationalize the denominator by multiplying the numerator and denominator by a term that eliminates the radical from the denominator.
For example, to rationalize
By being mindful of these common mistakes, you can simplify square roots more accurately and confidently.

Advanced Techniques and Concepts
In this section, we will explore advanced techniques and concepts for simplifying square roots, focusing on the square root of 20. These methods go beyond basic simplification and provide deeper insights into the properties and applications of square roots.
Using the Product Rule
The product rule for square roots states that:
\[ \sqrt{ab} = \sqrt{a} \cdot \sqrt{b} \]
We can apply this rule to simplify more complex expressions. For instance:
\[ \sqrt{20} = \sqrt{4 \cdot 5} = \sqrt{4} \cdot \sqrt{5} = 2\sqrt{5} \]
Using the Quotient Rule
The quotient rule for square roots allows us to simplify the square root of a fraction by separating the numerator and the denominator:
\[ \sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}} \]
For example:
\[ \sqrt{\frac{20}{4}} = \frac{\sqrt{20}}{\sqrt{4}} = \frac{2\sqrt{5}}{2} = \sqrt{5} \]
Combining Radical Expressions
When dealing with multiple radical expressions, we can use the product and quotient rules to combine and simplify them:
\[ \sqrt{20} \cdot \sqrt{5} = \sqrt{20 \cdot 5} = \sqrt{100} = 10 \]
Similarly:
\[ \frac{\sqrt{20}}{\sqrt{5}} = \sqrt{\frac{20}{5}} = \sqrt{4} = 2 \]
Advanced Factorization
Advanced factorization involves recognizing and extracting higher-order perfect squares from under the radical sign. This is particularly useful for simplifying more complex expressions:
Consider the expression:
\[ \sqrt{80} \]
We can factor 80 as \(16 \times 5\), where 16 is a perfect square:
\[ \sqrt{80} = \sqrt{16 \times 5} = \sqrt{16} \cdot \sqrt{5} = 4\sqrt{5} \]
Simplifying Radicals with Variables
When radicals include variables, the same rules apply. For example:
\[ \sqrt{45x^2} = \sqrt{9 \cdot 5 \cdot x^2} = \sqrt{9} \cdot \sqrt{5} \cdot \sqrt{x^2} = 3x\sqrt{5} \]
Applications and Real-Life Uses
Understanding advanced techniques for simplifying square roots is essential in higher mathematics and various practical applications, such as engineering, physics, and computer science. Mastery of these concepts allows for efficient problem-solving and a deeper understanding of mathematical principles.
Applications in Real Life
Understanding how to simplify the square root of 20 can be valuable in various practical scenarios:
- Engineering: In engineering fields such as construction and manufacturing, knowing the simplified form of square roots helps in precise calculations of measurements and materials needed.
- Finance: Financial analysts and economists often use simplified square roots in calculating interest rates, investment returns, and other financial metrics.
- Physics: Physicists frequently encounter square roots in equations related to velocity, acceleration, and energy calculations.
- Computer Science: Algorithms and data structures sometimes require square root calculations, where simplifying square roots can optimize performance.
Additional Resources and References
- : Provides clear explanations and examples on simplifying square roots.
- : Offers video tutorials and practice exercises on simplifying square roots and related concepts.
- : Detailed explanations, examples, and practice problems focusing on radicals and simplification techniques.
- : An online tool for step-by-step solutions to various math problems, including simplifying square roots.
READ MORE:
Xem video hướng dẫn chi tiết về cách rút gọn căn bậc hai của 20 (sqrt(20)). Nhận biết xem liệu video này phù hợp với bài viết về đề tài rút gọn căn bậc hai của 20 hay không.
Video Hướng dẫn Cách Rút gọn Căn bậc hai của 20: Sqrt(20)