Simplifying Square Root of 72: Easy Step-by-Step Guide

The process of simplifying the square root of 72 involves breaking it down into its prime factors and then simplifying those factors. Here’s a step-by-step guide:

Prime Factorization

First, we find the prime factors of 72. The prime factorization of 72 is:

\[
72 = 2^3 \times 3^2
\]

This means that 72 can be expressed as the product of \(2^3\) and \(3^2\).

Simplifying the Square Root

Next, we apply the square root to each of these factors:

\[
\sqrt{72} = \sqrt{2^3 \times 3^2}
\]

Using the property of square roots that \(\sqrt{a \times b} = \sqrt{a} \times \sqrt{b}\), we get:

\[
\sqrt{72} = \sqrt{2^3} \times \sqrt{3^2}
\]

Breaking Down Further

We know that \(\sqrt{3^2} = 3\), and we can break down \(\sqrt{2^3}\) as follows:

\[
\sqrt{2^3} = \sqrt{2^2 \times 2} = \sqrt{2^2} \times \sqrt{2} = 2\sqrt{2}
\]

Combining the Results

Now we combine the simplified parts:

\[
\sqrt{72} = 2\sqrt{2} \times 3
\]

Which simplifies to:

\[
\sqrt{72} = 6\sqrt{2}
\]

Final Result

Therefore, the simplified form of \(\sqrt{72}\) is:

\[
\boxed{6\sqrt{2}}
\]

Square roots are a fundamental concept in mathematics, representing a value that, when multiplied by itself, gives the original number. The square root of a number \( x \) is denoted as \( \sqrt{x} \). For example, \( \sqrt{9} = 3 \) because \( 3 \times 3 = 9 \).

Square roots have several important properties:

• Non-Negative Result: The square root of a non-negative number is always non-negative. For example, \( \sqrt{16} = 4 \) and \( \sqrt{0} = 0 \).
• Perfect Squares: Numbers like 1, 4, 9, 16, 25, etc., are called perfect squares because their square roots are integers.
• Prime Factorization: To simplify square roots, we often use the method of prime factorization, which involves breaking down the number into its prime factors.

Let's look at an example to understand the simplification process:

1. Start with the number under the square root, such as \( 72 \).
2. Find the prime factors of \( 72 \): \( 72 = 2^3 \times 3^2 \).
3. Apply the square root to each factor: \( \sqrt{72} = \sqrt{2^3 \times 3^2} = \sqrt{2^3} \times \sqrt{3^2} \).
4. Simplify further using the properties of square roots: \( \sqrt{2^3} = 2\sqrt{2} \) and \( \sqrt{3^2} = 3 \).
5. Combine the results: \( \sqrt{72} = 2\sqrt{2} \times 3 = 6\sqrt{2} \).

Thus, the simplified form of \( \sqrt{72} \) is \( 6\sqrt{2} \). This method can be applied to any non-negative number to simplify its square root effectively.

The square root of 72, represented as \( \sqrt{72} \), is a value that, when multiplied by itself, equals 72. To simplify \( \sqrt{72} \), we need to break it down into its prime factors and identify the perfect squares. Here’s a detailed step-by-step process to simplify the square root of 72:

1. First, list the factors of 72: 1, 2, 3, 4, 6, 8, 9, 12, 18, 24, 36, 72.
2. Identify the perfect squares from this list: 1, 4, 9, 36.
3. Choose the largest perfect square factor, which is 36.
4. Divide 72 by 36: \( 72 \div 36 = 2 \).
5. Take the square root of 36, which is 6.
6. Combine the results: \( \sqrt{72} = \sqrt{36 \times 2} = \sqrt{36} \times \sqrt{2} = 6\sqrt{2} \).

Thus, the simplified form of \( \sqrt{72} \) is \( 6\sqrt{2} \). This method can be applied to any non-negative number to simplify its square root effectively. Understanding the prime factorization and perfect squares helps in simplifying square roots easily.

Prime factorization is the process of breaking down a number into its prime factors, which are prime numbers that multiply together to give the original number. This method is essential for simplifying square roots, as it helps identify pairs of factors that can be extracted from under the radical sign.

To understand how to use prime factorization to simplify the square root of 72, follow these steps:

1. List the prime factors of 72.
   • Start by dividing 72 by the smallest prime number, which is 2: 72 ÷ 2 = 36.
   • Continue dividing by 2: 36 ÷ 2 = 18.
   • Divide by 2 again: 18 ÷ 2 = 9.
   • Now divide by the next smallest prime number, which is 3: 9 ÷ 3 = 3.
   • Finally, divide by 3: 3 ÷ 3 = 1.

   So, the prime factors of 72 are: 2 × 2 × 2 × 3 × 3.

2. Group the prime factors into pairs.

   The prime factors of 72 can be grouped as: (2 × 2) × (3 × 3) × 2.

3. Apply the square root to each pair.

   Take the square root of each pair of prime factors: √(2 × 2) = 2 and √(3 × 3) = 3.

4. Multiply the results outside the radical.

   Multiply the results of the square roots of the pairs: 2 × 3 = 6.

5. Combine the simplified factors.

   Since one factor (2) did not have a pair, it remains under the radical: √72 = 6√2.

Therefore, the simplified form of the square root of 72 is 6√2. This method can be applied to any number to simplify its square root using prime factorization.

Simplifying the square root of 72 involves breaking it down into its prime factors and then simplifying those factors. Follow these detailed steps:

1. List the Factors:

   First, list the factors of 72 to find its prime factorization. The factors are 1, 2, 3, 4, 6, 8, 9, 12, 18, 24, 36, 72.

2. Identify Perfect Squares:

   From the list, identify the perfect squares. These are 1, 4, 9, and 36.

3. Divide by the Largest Perfect Square:

   Divide 72 by the largest perfect square found, which is 36:

   \[ 72 \div 36 = 2 \]

4. Square Root of the Perfect Square:

   Calculate the square root of 36:

   \[ \sqrt{36} = 6 \]

5. Simplify the Square Root:

   Combine the results from the previous steps to simplify the square root:

   \[ \sqrt{72} = 6 \sqrt{2} \]

By following these steps, you can simplify the square root of 72 to its simplest radical form, which is \( 6 \sqrt{2} \).

To simplify the square root of 72, we need to break it down into its prime factors. This process involves identifying all the prime numbers that multiply together to give 72.

1. First, we start with the number 72 and divide it by the smallest prime number, which is 2:

   \(72 \div 2 = 36\)

2. Next, we continue dividing by 2 as long as the result is an even number:

   \(36 \div 2 = 18\)

   \(18 \div 2 = 9\)

3. When we reach 9, which is no longer even, we switch to the next smallest prime number, which is 3:

   \(9 \div 3 = 3\)

   \(3 \div 3 = 1\)

By following these steps, we can express 72 as a product of prime factors:

\(72 = 2 \times 2 \times 2 \times 3 \times 3\)

We can also write this using exponents to make it easier to manage:

\(72 = 2^3 \times 3^2\)

Now that we have broken down 72 into its prime factors, we can move on to the next step of simplifying the square root.

To simplify the square root of 72, we first need to break it down into its prime factors. This helps us identify and separate the perfect squares from the rest of the factors, making it easier to simplify. Here's a step-by-step guide:

1. Factorize 72: Start by finding the prime factors of 72. We have:

   72 = 2 × 2 × 2 × 3 × 3

2. Group the Factors: Next, group the prime factors into pairs of identical numbers:

   (2 × 2) × (3 × 3) × 2

3. Identify Perfect Squares: Notice that (2 × 2) and (3 × 3) are perfect squares:

   2² and 3²

4. Apply the Square Root: Take the square root of each perfect square separately:

   √(2²) = 2

   √(3²) = 3

5. Combine Simplified Factors: Multiply the simplified square roots together along with the remaining factor inside the radical:

   2 × 3 × √2 = 6√2

Therefore, the square root of 72 simplified is:

√72 = 6√2

This step-by-step process ensures that we break down the square root into manageable parts, apply the square root to each perfect square, and then combine the results for the simplest form.

Once we have simplified the prime factors of 72 under the square root, we need to combine them to find the final simplified form. Here are the detailed steps to achieve this:

1. Recall that the prime factorization of 72 is \( 2^3 \times 3^2 \). We separated these factors earlier:

   \[
   \sqrt{72} = \sqrt{2^3 \times 3^2}
   \]

2. Apply the property of square roots that allows us to take the square root of each factor individually:

   \[
   \sqrt{2^3 \times 3^2} = \sqrt{2^3} \times \sqrt{3^2}
   \]

3. Simplify the square roots of the factors:

   \[
   \sqrt{2^3} = \sqrt{2^2 \times 2} = \sqrt{2^2} \times \sqrt{2} = 2\sqrt{2}
   \]

   \[
   \sqrt{3^2} = 3
   \]

4. Combine the simplified factors by multiplying them together:

   \[
   \sqrt{72} = 2\sqrt{2} \times 3
   \]

   Which simplifies further to:

   \[
   2 \times 3 \times \sqrt{2} = 6\sqrt{2}
   \]

5. Thus, the square root of 72 in its simplest form is:

   \[
   \boxed{6\sqrt{2}}
   \]

By following these steps, we ensure that we have correctly combined the simplified factors of 72. This method can be applied to any similar problems involving the simplification of square roots with multiple factors.

After simplifying the square root of 72 to \(6\sqrt{2}\), it is crucial to verify the correctness of this result. Here are the steps to ensure that our simplification is accurate:

1. Square the Simplified Expression: We start by squaring the simplified form \(6\sqrt{2}\).

   Calculate \( (6\sqrt{2})^2 \):

   \[
   (6\sqrt{2})^2 = 6^2 \cdot (\sqrt{2})^2 = 36 \cdot 2 = 72
   \]

   This confirms that squaring \(6\sqrt{2}\) indeed gives us the original number 72.

2. Check with Prime Factorization: Verify the intermediate steps by reviewing the prime factorization used during simplification.

   72 was factorized into \(2^3 \cdot 3^2\). We grouped these factors into \((2^2 \cdot 3^2) \cdot 2 = (4 \cdot 9) \cdot 2 = 36 \cdot 2\), which led to \(\sqrt{36 \cdot 2} = \sqrt{36} \cdot \sqrt{2} = 6\sqrt{2}\).

   Thus, each step in the factorization and simplification process is validated.

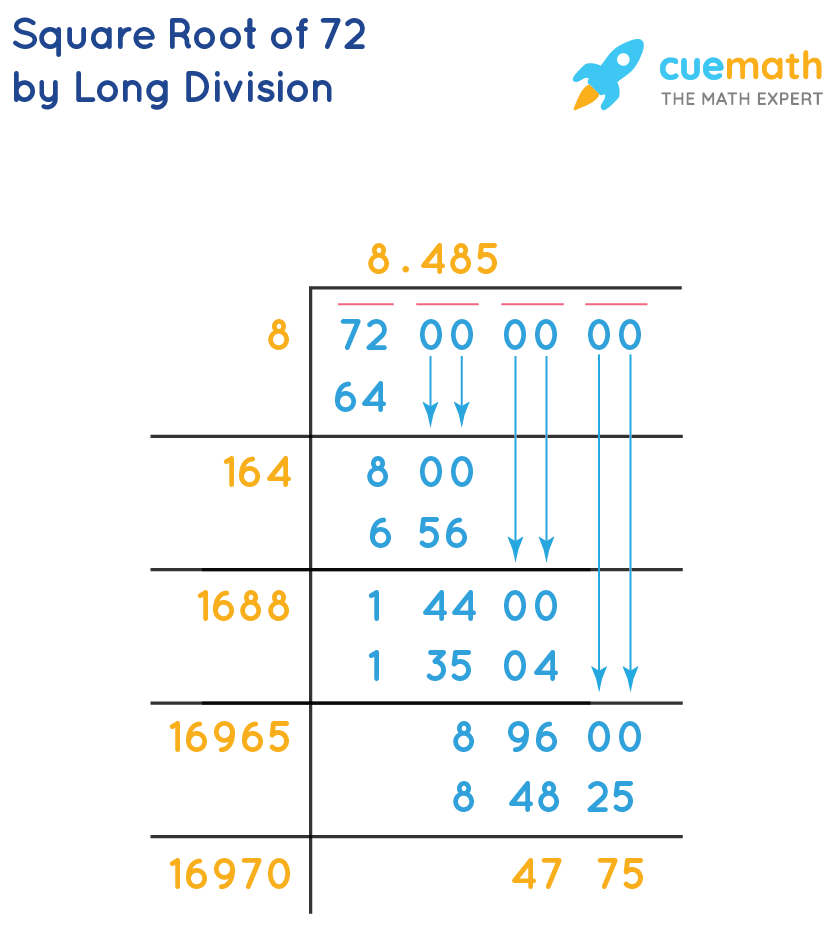
3. Verify by Calculation: Use a calculator to find the square root of 72 and compare it with the simplified form.

   Using a calculator, \( \sqrt{72} \approx 8.485 \). Also, \( 6 \cdot \sqrt{2} \approx 6 \cdot 1.414 = 8.485 \).

   Both results are identical, confirming the accuracy of the simplified expression.

By following these steps, we ensure that our simplified result \(6\sqrt{2}\) is indeed correct and accurately represents \(\sqrt{72}\).

When simplifying the square root of 72, certain pitfalls can hinder the accuracy of your solution. Being aware of these common mistakes can enhance your understanding and ensure a correct simplification process.

• Overlooking Prime Factorization: A common mistake is not fully breaking down 72 into its prime factors. Ensure you reach the most basic prime numbers for accurate simplification. The correct prime factorization is \(2 \times 2 \times 2 \times 3 \times 3\).
• Incorrect Grouping of Factors: Misgrouping the prime factors can lead to erroneous results. Remember that you need pairs of the same factor to simplify under the square root. For example, group \(2 \times 2\) and \(3 \times 3\) correctly.
• Forgetting to Multiply Outside the Root: After extracting square roots from the prime factors, some forget to multiply these values outside the square root, which is crucial for reaching the final simplified form. For \( \sqrt{72} \), you should multiply the extracted factors \(2\) and \(3\) to get \(6\).
• Misplacing the Leftover Factor: It is easy to forget or incorrectly place the leftover prime factor that doesn’t form a pair. This factor must remain under the square root for correct simplification. Here, the remaining \(2\) stays under the root as \( \sqrt{2} \), resulting in \(6\sqrt{2}\).
• Confusing Addition with Multiplication: Ensure that when you're simplifying, you multiply the numbers outside the square root rather than adding them. This is a common error that changes the outcome significantly.

By avoiding these mistakes, you can simplify the square root of 72 accurately and efficiently, enhancing your mathematical skills and understanding.

Square roots play a crucial role in various fields, including geometry, physics, engineering, and finance. Understanding how to simplify and use square roots, such as the square root of 72, can be incredibly beneficial. Here are some practical applications:

• Geometry

  Square roots are often used in geometry to determine distances and lengths. For example, the Pythagorean theorem involves calculating the length of the hypotenuse of a right triangle. If the lengths of the other two sides are known, you can find the hypotenuse using the square root.

• Physics

  In physics, square roots are used to calculate various properties such as the magnitude of vectors, the distance traveled under acceleration, and energy computations. Simplifying square roots can make these calculations more manageable.

• Engineering

  Engineers use square roots in various calculations, such as determining the natural frequency of structures, calculating load distributions, and analyzing signals. For instance, the stress and strain in materials often involve square roots.

• Finance

  Square roots are used in finance to calculate volatility and risk. For example, the standard deviation, which measures the amount of variation or dispersion in a set of values, is the square root of the variance.

• Statistics

  In statistics, square roots are used to determine standard deviation and variance. These measures help in understanding data distributions and making informed decisions based on statistical analysis.

• Computer Science

  Square roots are used in algorithms for encryption, image processing, and game development. They help in optimizing calculations and improving the efficiency of various computational processes.

These applications demonstrate the importance of understanding square roots and their simplification in various real-world scenarios. Mastering this mathematical concept can enhance problem-solving skills and enable more precise and efficient calculations across different domains.

Simplifying the square root of 72 is a fundamental skill that demonstrates the practical applications of mathematical principles in everyday life. By breaking down the problem into smaller, manageable steps, we can see the elegance and utility of mathematics.

1. Firstly, we use prime factorization to express 72 as a product of its prime factors: \( 72 = 2^3 \times 3^2 \).

2. Next, we apply the square root to each factor separately, leveraging the property of square roots: \( \sqrt{72} = \sqrt{2^3 \times 3^2} \).

3. We then simplify the expression by taking the square root of the perfect squares: \( \sqrt{2^3 \times 3^2} = 3 \sqrt{2 \times 2} \) which simplifies to \( 6\sqrt{2} \).

The simplified form of \( \sqrt{72} \) is \( 6\sqrt{2} \). This method of simplification not only helps in solving mathematical problems more efficiently but also enhances our understanding of how numbers interact.

• Enhanced Understanding: Simplifying square roots deepens our grasp of number properties and relationships, a crucial aspect in advanced mathematics.
• Practical Utility: From geometry to physics, the ability to simplify square roots is vital in various fields, allowing for more straightforward problem-solving and analysis.
• Building Foundations: Mastering these concepts lays a solid foundation for tackling more complex mathematical challenges, fostering analytical thinking and precision.

In conclusion, the process of simplifying the square root of 72 not only illustrates a key mathematical principle but also underscores the importance of these skills in both academic and real-world applications. By continuously practicing and applying these techniques, we can develop a robust mathematical foundation that will benefit us in numerous ways.