How to Simplify Square Root of 343: A Step-by-Step Guide

To simplify the square root of 343, we need to express it in its simplest radical form. Here are the steps:

Step-by-Step Solution

1. Find the prime factorization of 343.
2. Group the prime factors in pairs.
3. Simplify the expression by taking out the pairs.

Prime Factorization of 343

First, we find the prime factors of 343:

• 343 ÷ 7 = 49
• 49 ÷ 7 = 7
• 7 ÷ 7 = 1

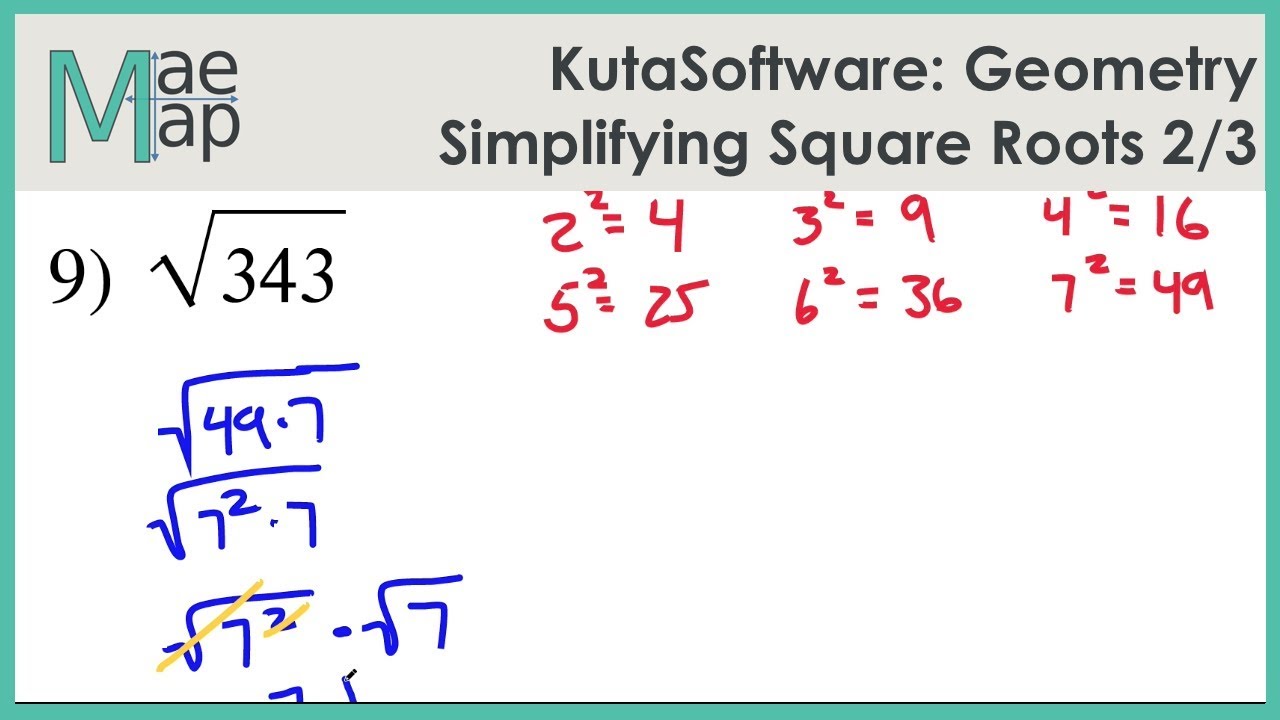
So, the prime factorization of 343 is:

\(343 = 7 \times 7 \times 7\)

Grouping the Prime Factors

Next, we group the prime factors in pairs:

\(343 = 7^2 \times 7\)

Simplifying the Expression

We take out the pair of 7s from under the square root:

\(\sqrt{343} = \sqrt{7^2 \times 7} = 7\sqrt{7}\)

Final Answer

Therefore, the simplified form of the square root of 343 is:

\(\sqrt{343} = 7\sqrt{7}\)

The square root of a number is a value that, when multiplied by itself, gives the original number. In mathematical terms, if \( x \) is the square root of \( y \), then \( x^2 = y \).

Square roots are fundamental in various fields, including algebra, geometry, and even real-life applications. Understanding how to simplify square roots is an essential skill in mathematics.

Here are some key points to understand about square roots:

• The square root symbol is \( \sqrt{} \).
• Every positive number has two square roots: one positive and one negative. For example, the square roots of 9 are 3 and -3 because \( 3^2 = 9 \) and \( (-3)^2 = 9 \).
• The square root of zero is zero.
• Negative numbers do not have real square roots because no real number squared gives a negative result.

Simplifying square roots involves breaking down the number inside the square root into its prime factors and then simplifying. This process helps to express the square root in its simplest form.

Let's take a look at the square root of 343 as an example:

1. Find the prime factors of 343.
2. Group the prime factors in pairs.
3. Simplify the square root expression by taking out the pairs.

By mastering the technique of simplifying square roots, you can solve various mathematical problems more efficiently.

Simplifying square roots involves expressing the square root in its simplest radical form. This process is essential for making calculations easier and for understanding the underlying factors of a number. Here’s a detailed, step-by-step guide to understanding square root simplification:

1. Identify the Square Root:

   The square root of a number \( n \) is a value that, when multiplied by itself, gives \( n \). For example, the square root of 343 is denoted as \( \sqrt{343} \).

2. Prime Factorization:

   Break down the number into its prime factors. Prime factorization of 343 is:

   \[ 343 = 7 \times 7 \times 7 = 7^3 \]

3. Group the Factors:

   Group the prime factors in pairs. Since 343 is \( 7^3 \), it can be grouped as:

   \[ \sqrt{343} = \sqrt{7^2 \times 7} \]

4. Simplify the Square Root:

   Take one factor from each pair out of the square root:

   \[ \sqrt{7^2 \times 7} = 7 \sqrt{7} \]

   So, the simplified form of \( \sqrt{343} \) is \( 7 \sqrt{7} \).

5. Result in Decimal Form:

   The simplified form can also be converted into a decimal for practical use:

   \[ 7 \sqrt{7} \approx 18.520 \]

This method can be applied to any number to simplify its square root. Understanding this process is crucial for various mathematical applications, from solving equations to performing higher-level calculus.

The prime factorization method is a powerful technique for simplifying square roots. This method involves expressing the number inside the square root as a product of its prime factors. Here, we will apply this method to simplify the square root of 343.

Step-by-step process to simplify \( \sqrt{343} \) using the prime factorization method:

1. Find the prime factors of 343.
2. Group the prime factors in pairs.
3. Simplify the square root by taking out pairs of prime factors.

Let’s start with the prime factorization of 343:

\( 343 = 7 \times 7 \times 7 = 7^3 \)

Now, express 343 under the square root:

\( \sqrt{343} = \sqrt{7^3} \)

Next, separate the exponent in a way that one part is an even number:

\( \sqrt{343} = \sqrt{7^2 \times 7} \)

Since \( 7^2 \) is a perfect square, we can take it out of the square root:

\( \sqrt{343} = \sqrt{7^2} \times \sqrt{7} \)

\( \sqrt{343} = 7 \times \sqrt{7} \)

Therefore, the simplified form of \( \sqrt{343} \) is:

\( \sqrt{343} = 7\sqrt{7} \)

This method allows us to break down the square root into a simpler form, making it easier to handle in further calculations. Using this technique, the square root of 343 is simplified to \( 7\sqrt{7} \).

Simplifying the square root of 343 involves breaking it down into its prime factors and then simplifying the radical expression. Follow these steps:

1. Find the prime factorization of 343:

   • 343 is not a prime number. Divide it by the smallest prime number:
   • \[ 343 \div 7 = 49 \]
   • 49 is also not a prime number. Divide it by 7 again:
   • \[ 49 \div 7 = 7 \]
   • Now, 7 is a prime number. So, the prime factorization of 343 is:
   • \[ 343 = 7 \times 7 \times 7 = 7^3 \]

2. Express the square root of 343 using its prime factors:

   \[
   \sqrt{343} = \sqrt{7 \times 7 \times 7}
   \]

3. Simplify the square root by grouping the factors into pairs:

   \[
   \sqrt{7 \times 7 \times 7} = \sqrt{(7 \times 7) \times 7} = \sqrt{49 \times 7}
   \]

   Since \(\sqrt{49} = 7\), we can simplify further:

   \[
   \sqrt{49 \times 7} = 7 \sqrt{7}
   \]

4. Therefore, the simplified form of \(\sqrt{343}\) is:

   \[
   \sqrt{343} = 7\sqrt{7}
   \]

By following these steps, you can simplify the square root of 343 to \(7\sqrt{7}\).

Simplifying square roots is a fundamental skill in mathematics that offers several significant benefits. Understanding and applying this process can enhance problem-solving efficiency and deepen comprehension of mathematical concepts.

• Eases Complex Calculations: Simplified square roots reduce the complexity of expressions, making them easier to work with in various mathematical operations. For example, simplifying \\(\sqrt{343} = 7\sqrt{7}\\) makes it more manageable in further calculations.
• Solves Quadratic Equations: Simplified square roots are crucial in solving quadratic equations, allowing for cleaner and more straightforward solutions. This is especially helpful in algebra and calculus where precise solutions are necessary.
• Facilitates Higher Mathematical Learning: Mastering the simplification of square roots lays a strong foundation for understanding more advanced topics in mathematics, such as calculus, trigonometry, and complex numbers.
• Applications in Real Life: Simplified square roots are used in various real-world applications, including engineering, physics, and computer science. They play a critical role in measurements, calculations, and algorithms, making tasks more efficient and accurate.
• Promotes Mathematical Elegance: Simplifying square roots often leads to more elegant and aesthetically pleasing solutions, which can be satisfying and motivating for students and professionals alike.

In conclusion, the importance of simplifying square roots extends beyond mere academic exercises. It is a practical skill that enhances problem-solving abilities, supports advanced learning, and finds numerous applications in everyday life and professional fields.

Below are several examples that demonstrate how to simplify various square roots using the prime factorization method:

• Simplifying √50:
  1. Prime factorize 50: 50 = 2 × 5²
  2. Rewrite the square root: √50 = √(2 × 5²)
  3. Separate the factors: √50 = √2 × √(5²)
  4. Simplify the square root of the perfect square: √(5²) = 5
  5. Combine the results: √50 = 5√2
• Simplifying √72:
  1. Prime factorize 72: 72 = 2³ × 3²
  2. Rewrite the square root: √72 = √(2³ × 3²)
  3. Separate the factors: √72 = √(2² × 2) × √(3²)
  4. Simplify the square roots of the perfect squares: √(2²) = 2 and √(3²) = 3
  5. Combine the results: √72 = 2 × 3 × √2 = 6√2
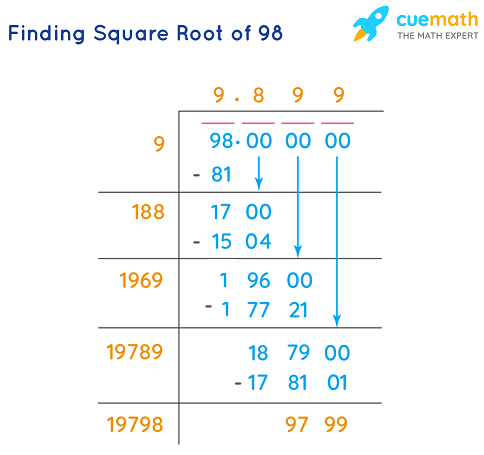
• Simplifying √98:
  1. Prime factorize 98: 98 = 2 × 7²
  2. Rewrite the square root: √98 = √(2 × 7²)
  3. Separate the factors: √98 = √2 × √(7²)
  4. Simplify the square root of the perfect square: √(7²) = 7
  5. Combine the results: √98 = 7√2
• Simplifying √200:
  1. Prime factorize 200: 200 = 2³ × 5²
  2. Rewrite the square root: √200 = √(2³ × 5²)
  3. Separate the factors: √200 = √(2² × 2) × √(5²)
  4. Simplify the square roots of the perfect squares: √(2²) = 2 and √(5²) = 5
  5. Combine the results: √200 = 2 × 5 × √2 = 10√2

These examples show how to use the prime factorization method to simplify square roots step-by-step, ensuring a clear understanding of the process.

When simplifying square roots, there are several common mistakes that students often make. Being aware of these pitfalls can help you avoid them and ensure that your simplification process is accurate. Here are some of the most frequent mistakes and how to avoid them:

• Forgetting to Check for Perfect Squares:

  Always check if the number under the square root is a perfect square before attempting to simplify. For example, √49 simplifies to 7 because 49 is a perfect square.

• Incorrect Factorization:

  Ensure you correctly factorize the number into its prime factors. For example, to simplify √72, correctly factor it as 2 × 2 × 2 × 3 × 3, which simplifies to 6√2.

• Misapplying the Product Property of Square Roots:

  Remember that √(a × b) = √a × √b. For instance, √50 can be simplified as √(25 × 2) = √25 × √2 = 5√2. Be careful not to apply this property incorrectly.

• Not Simplifying Completely:

  Ensure that you simplify the square root completely. For example, √18 can be broken down to √(9 × 2) = 3√2, instead of leaving it as √18.

• Ignoring Negative Roots:

  When solving equations, remember that both positive and negative roots should be considered. For example, the equation x² = 9 has solutions x = 3 and x = -3.

• Incorrectly Combining Terms:

  Avoid adding or subtracting square roots directly unless they are like terms. For example, √3 + √3 = 2√3, but √3 + √2 cannot be simplified further.

• Assuming Linear Properties Apply:

  Remember that square roots do not follow the same rules as linear functions. For example, √(a + b) ≠ √a + √b.

By being mindful of these common mistakes, you can improve your ability to simplify square roots correctly and efficiently.

Here are some practice problems to help you master the art of simplifying square roots. Each problem is followed by a detailed solution.

1. Simplify \( \sqrt{75} \)

   Solution:

   1. Factor 75 into its prime factors: \( 75 = 3 \times 5 \times 5 \).
   2. Group the pairs of prime factors: \( \sqrt{75} = \sqrt{3 \times 5^2} \).
   3. Take the square root of the pairs: \( \sqrt{75} = 5\sqrt{3} \).

2. Simplify \( \sqrt{50} \)

   Solution:

   1. Factor 50 into its prime factors: \( 50 = 2 \times 5 \times 5 \).
   2. Group the pairs of prime factors: \( \sqrt{50} = \sqrt{2 \times 5^2} \).
   3. Take the square root of the pairs: \( \sqrt{50} = 5\sqrt{2} \).

3. Simplify \( \sqrt{98} \)

   Solution:

   1. Factor 98 into its prime factors: \( 98 = 2 \times 7 \times 7 \).
   2. Group the pairs of prime factors: \( \sqrt{98} = \sqrt{2 \times 7^2} \).
   3. Take the square root of the pairs: \( \sqrt{98} = 7\sqrt{2} \).

4. Simplify \( \sqrt{200} \)

   Solution:

   1. Factor 200 into its prime factors: \( 200 = 2 \times 2 \times 2 \times 5 \times 5 \).
   2. Group the pairs of prime factors: \( \sqrt{200} = \sqrt{2^3 \times 5^2} \).
   3. Take the square root of the pairs: \( \sqrt{200} = 10\sqrt{2} \).

Simplified square roots have numerous applications in various fields. Understanding these applications can provide a deeper appreciation of the importance of square roots in everyday life and professional practices.

• Finance:

  Square roots are used in finance to calculate stock market volatility. This involves taking the square root of the variance of stock returns, helping investors assess the risk of an investment.

• Architecture:

  Engineers use square roots to determine the natural frequencies of structures such as bridges and buildings. This helps predict how structures will respond to different forces, ensuring safety and stability.

• Science:

  Square roots are essential in scientific calculations, including determining the velocity of moving objects, radiation absorption by materials, and sound wave intensity.

• Statistics:

  In statistics, square roots are used to calculate standard deviation, a measure of data dispersion. The standard deviation is the square root of the variance, indicating how much data points deviate from the mean.

• Geometry:

  Square roots are crucial in geometry for calculating the area and perimeter of shapes and solving problems involving right triangles, as illustrated by the Pythagorean theorem.

• Computer Science:

  Square roots play a role in computer programming for encryption algorithms, image processing, and game physics, among other applications.

• Cryptography:

  Cryptographic systems utilize square roots for digital signatures, key exchange systems, and secure communication channels to ensure data security.

• Navigation:

  Square roots are used in navigation to calculate distances between points on a map and estimate the course direction, aiding pilots and sailors.

• Electrical Engineering:

  Electrical engineers use square roots to compute power, voltage, and current in circuits, which is crucial for designing and maintaining electrical systems.

• Cooking:

  In cooking, adjusting recipe quantities often involves mathematical calculations using square roots to maintain flavor balance when scaling up or down.

• Photography:

  The aperture of a camera lens, expressed as an f-number, relates to the square root of the lens area. Changing the f-number affects the amount of light entering the camera, impacting photo exposure.

• Computer Graphics:

  In 2D and 3D graphics, square roots are used to calculate distances between points and vector lengths, essential for rendering accurate images.

• Telecommunication:

  Square roots help determine signal strength in wireless communication, following the inverse square law where signal strength decreases with the square of the distance from the transmitter.

Simplifying the square root of 343 helps us understand the process of breaking down a number into its prime factors and using those factors to find the simplest radical form. By applying the prime factorization method, we can express \( \sqrt{343} \) as \( 7\sqrt{7} \). This simplification is not only useful for mathematical calculations but also enhances our comprehension of square roots and their properties.

To recap, the steps to simplify \( \sqrt{343} \) are as follows:

1. Find the prime factors of 343, which are \( 7 \times 7 \times 7 \) or \( 7^3 \).
2. Express the square root as \( \sqrt{7^2 \times 7} \).
3. Separate the factors under the square root to \( \sqrt{7^2} \times \sqrt{7} \).
4. Simplify \( \sqrt{7^2} \) to 7, resulting in \( 7\sqrt{7} \).

Understanding this process aids in simplifying other complex square roots and enhances problem-solving skills in algebra. Practice these steps with other numbers to gain confidence in your ability to simplify square roots effectively.

In conclusion, mastering the simplification of square roots is a valuable mathematical skill with practical applications in various fields. Whether in academics, engineering, or everyday calculations, knowing how to simplify square roots can make complex problems more manageable and improve your overall mathematical proficiency.