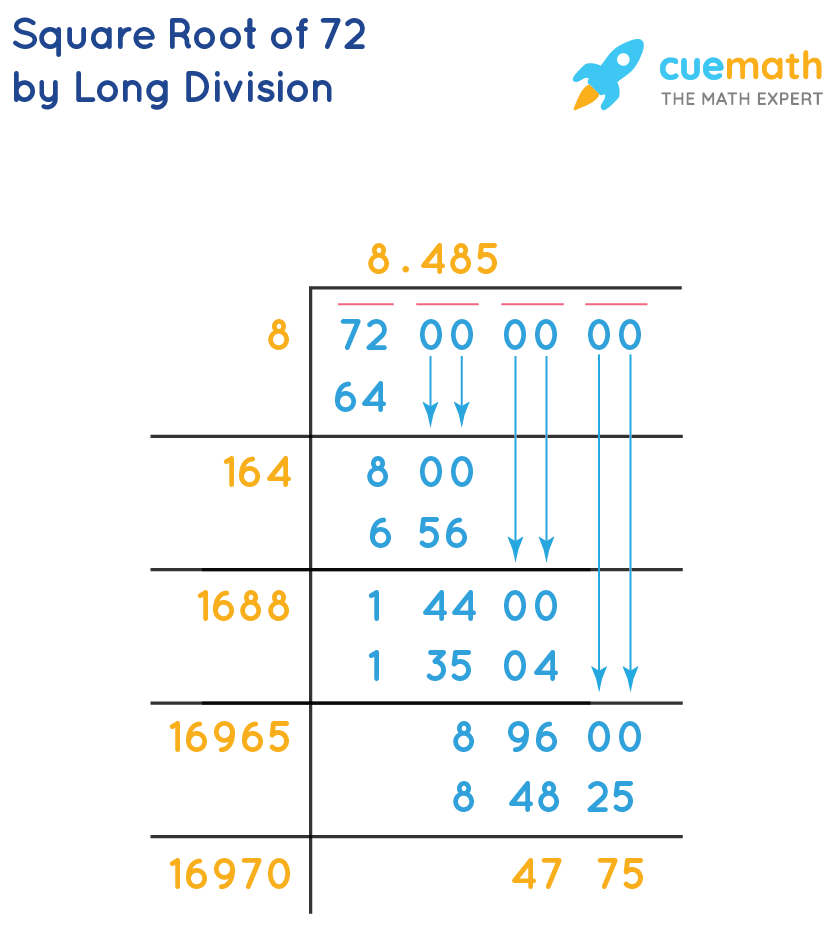
Topic how do you simplify the square root of 72: Simplifying the square root of 72 may seem challenging, but with the right steps, it's quite straightforward. By breaking it down into prime factors, identifying perfect squares, and using radical simplification, you can simplify √72 to its simplest form, which is 6√2. Read on to discover detailed methods and tips to master this fundamental math skill!
Table of Content
Simplifying the Square Root of 72
To simplify the square root of 72, we can follow these steps:
Step 1: Prime Factorization
First, find the prime factors of 72. The prime factorization of 72 is:
\[ 72 = 2 \times 36 \]
\[ 36 = 2 \times 18 \]
\[ 18 = 2 \times 9 \]
\[ 9 = 3 \times 3 \]
So, the prime factors of 72 are:
\[ 72 = 2 \times 2 \times 2 \times 3 \times 3 \]
Step 2: Group the Prime Factors
Group the prime factors into pairs of the same number:
\[ 72 = (2 \times 2) \times (3 \times 3) \times 2 \]
Step 3: Simplify the Square Root
Take the square root of each pair of prime factors and move them outside the square root symbol:
\[ \sqrt{72} = \sqrt{(2 \times 2) \times (3 \times 3) \times 2} \]
\[ = \sqrt{2^2 \times 3^2 \times 2} \]
\[ = 2 \times 3 \times \sqrt{2} \]
\[ = 6\sqrt{2} \]
Conclusion
Therefore, the simplified form of \(\sqrt{72}\) is:
\[ \sqrt{72} = 6\sqrt{2} \]

READ MORE:
Introduction
Understanding how to simplify the square root of 72 involves a series of clear, logical steps. Simplification of square roots is a fundamental skill in mathematics, helping to make complex expressions more manageable. In this guide, we will explore the methods to simplify √72, including prime factorization and identifying perfect squares. By following these steps, you'll find that simplifying √72 to 6√2 is straightforward and intuitive.
To simplify the square root of 72, we use the prime factorization method, which breaks down a number into its prime factors. Here's a detailed step-by-step process:
- List the factors of 72: 1, 2, 3, 4, 6, 8, 9, 12, 18, 24, 36, 72.
- Identify the perfect squares from the list: 1, 4, 9, 36.
- Divide 72 by the largest perfect square identified: \( 72 \div 36 = 2 \).
- Calculate the square root of the largest perfect square: \( \sqrt{36} = 6 \).
- Combine the results to simplify the square root of 72: \( \sqrt{72} = 6\sqrt{2} \).
This approach not only simplifies the expression but also makes it easier to work with in various mathematical applications. By mastering the technique of simplifying square roots, you enhance your ability to handle more complex algebraic expressions with confidence.
Understanding Square Roots
The square root of a number is a value that, when multiplied by itself, gives the original number. For example, the square root of 72 is a number which, when squared, equals 72. This concept is fundamental in mathematics and has various applications in different fields.
To understand square roots better, consider the following points:
- Notation: The square root of 72 is written as \( \sqrt{72} \). The symbol \( \sqrt{} \) is called the radical, and the number inside it is the radicand.
- Inverse of Squaring: Finding the square root is the opposite of squaring a number. If \( x^2 = 72 \), then \( x = \sqrt{72} \).
- Properties: Square roots can be simplified by factoring the radicand into its prime factors. For instance, \( 72 = 2^3 \times 3^2 \), which can be broken down to simplify \( \sqrt{72} \).
- Radical Form: The simplified form of \( \sqrt{72} \) is \( 6\sqrt{2} \), where 6 is the coefficient and \( \sqrt{2} \) is the simplest form of the square root that cannot be further simplified.
Understanding how to simplify square roots is crucial as it makes further mathematical operations easier and more manageable. By breaking down numbers into their prime factors and applying the properties of square roots, we can simplify complex expressions and solve problems efficiently.
Basics of Simplifying Square Roots
Simplifying square roots involves expressing a square root in its simplest form. The process requires understanding prime factorization and recognizing perfect squares within the number. Here, we'll break down the steps to simplify the square root of 72.
Step-by-Step Process
- Identify the factors of 72. The prime factors of 72 are 2 and 3.
- 72 = 2 × 2 × 2 × 3 × 3
- Group the factors into pairs of equal factors.
- (2 × 2) and (3 × 3) with one 2 left over
- Take one factor out of each pair and multiply them together.
- √(2 × 2) = 2
- √(3 × 3) = 3
- Multiply the numbers outside the square root.
- 2 × 3 = 6
- Combine the result with the remaining factor inside the square root.
- 6√2
Result
The simplified form of √72 is 6√2. This method ensures that the square root is expressed in its simplest radical form, making it easier to understand and use in further calculations.
Prime Factorization Method
Simplifying the square root of a number involves breaking it down into its prime factors. The prime factorization method is a reliable way to simplify the square root of 72. Here’s a step-by-step guide:
-
Factorize 72 into prime factors: Start by breaking down 72 into its prime factors.
\(72 = 2 \times 36\)
\(36 = 2 \times 18\)
\(18 = 2 \times 9\)
\(9 = 3 \times 3\)
So, \(72 = 2 \times 2 \times 2 \times 3 \times 3\). -
Group the prime factors: Group the factors into pairs of identical numbers.
\(72 = (2 \times 2 \times 2) \times (3 \times 3)\)
\(72 = (2^3) \times (3^2)\) -
Simplify using square root properties: Use the property of square roots that \(\sqrt{a \times b} = \sqrt{a} \times \sqrt{b}\).
\(\sqrt{72} = \sqrt{2^3 \times 3^2} = \sqrt{2^3} \times \sqrt{3^2}\)
\(\sqrt{2^3} = \sqrt{2^2 \times 2} = \sqrt{2^2} \times \sqrt{2} = 2 \sqrt{2}\)
\(\sqrt{3^2} = 3\)
Therefore, \(\sqrt{72} = 2 \sqrt{2} \times 3 = 6 \sqrt{2}\).
Using the prime factorization method, we have simplified the square root of 72 to \(6\sqrt{2}\).

Step-by-Step Simplification Process
Simplifying the square root of 72 involves breaking down the number into its prime factors and then applying the properties of square roots to simplify the expression.
-
Start by finding the prime factorization of 72:
- 72 can be divided by 2 (its smallest prime factor): \( 72 \div 2 = 36 \)
- Continue dividing by 2: \( 36 \div 2 = 18 \)
- Continue dividing by 2: \( 18 \div 2 = 9 \)
- 9 is not divisible by 2, so move to the next smallest prime factor, 3: \( 9 \div 3 = 3 \)
- Divide by 3 again: \( 3 \div 3 = 1 \)
So, the prime factors of 72 are: \( 2 \times 2 \times 2 \times 3 \times 3 \) or \( 2^3 \times 3^2 \).
-
Next, group the prime factors into pairs of the same number:
- From \( 2^3 \), we can take out one pair of 2's: \( 2^2 \times 2 = 4 \times 2 \)
- From \( 3^2 \), we can take out one pair of 3's: \( 3^2 = 9 \)
-
Simplify the square root by taking the square root of each pair of numbers:
- \( \sqrt{2^2} = 2 \)
- \( \sqrt{3^2} = 3 \)
So, \( \sqrt{72} = \sqrt{2^3 \times 3^2} = \sqrt{(2^2 \times 2) \times 3^2} = 2 \times 3 \times \sqrt{2} = 6\sqrt{2} \)
Therefore, the simplified form of \( \sqrt{72} \) is \( 6\sqrt{2} \).
Example of Simplifying \(\sqrt{72}\)
In this section, we will walk through an example of simplifying the square root of 72 using the prime factorization method. This step-by-step guide will help you understand the process and apply it to other numbers.
-
List the Factors: Start by listing all the factors of 72. These are 1, 2, 3, 4, 6, 8, 9, 12, 18, 24, 36, and 72.
-
Identify Perfect Squares: From the list of factors, identify the perfect squares. The perfect squares are 1, 4, 9, and 36.
-
Divide by the Largest Perfect Square: Divide 72 by the largest perfect square identified, which is 36.
\[ \frac{72}{36} = 2 \]
-
Calculate the Square Root of the Perfect Square: The square root of 36 is 6.
\[ \sqrt{36} = 6 \]
-
Express the Original Square Root in Simplest Form: Combine the results of the previous steps to express the square root of 72 in its simplest form.
\[ \sqrt{72} = \sqrt{36 \times 2} = \sqrt{36} \times \sqrt{2} = 6\sqrt{2} \]
Thus, the simplified form of \(\sqrt{72}\) is \(6\sqrt{2}\).
Common Mistakes to Avoid
Simplifying square roots can be challenging, and it's easy to make mistakes. Here are some common errors and tips to help you avoid them:
-
Forgetting to Simplify Completely:
Ensure you simplify the square root as much as possible. For example, with \(\sqrt{72}\), continue simplifying until you get \(6\sqrt{2}\) and not just stop at any intermediate steps.
-
Misidentifying Prime Factors:
Incorrect factorization can lead to wrong answers. Double-check your prime factorization. For \(72\), the correct factors are \(2^3 \times 3^2\).
-
Overlooking Perfect Square Factors:
Missing perfect square factors within the radicand can prevent full simplification. Always look for and extract these factors. For example, in \(\sqrt{72}\), the factor \(36\) (which is \(6^2\)) should be identified and simplified.
-
Incorrect Pairing of Factors:
Ensure you pair identical factors correctly. Each pair should simplify to a whole number outside the square root. In \(\sqrt{72}\), the pairs \(2^2\) and \(3^2\) are correctly simplified to \(6\sqrt{2}\).
-
Mixing Up Operations:
Remember that simplifying a square root is different from other operations like polynomial factoring. Keep the operations distinct and apply the appropriate method for simplifying square roots.
-
Rushing Through the Process:
Take your time to work through each step carefully. Rushing can lead to mistakes, so it's essential to follow the process methodically.
By being mindful of these common pitfalls, you can improve your proficiency in simplifying square roots, ensuring accurate and efficient results.
Additional Tips and Tricks
Simplifying square roots can be made easier with some additional tips and tricks. Here are a few strategies to help you simplify the square root of 72 and other similar problems:
- Prime Factorization: Always start by finding the prime factorization of the number under the square root. For 72, this means breaking it down into its prime factors: \( 72 = 2^3 \times 3^2 \).
- Grouping Pairs: Group the prime factors into pairs. Each pair of the same number can be taken out of the square root. For example, \( \sqrt{72} = \sqrt{2^3 \times 3^2} = \sqrt{(2^2 \times 3^2) \times 2} = 2 \times 3 \times \sqrt{2} = 6\sqrt{2} \).
- Using Multiplication: Use the property \( \sqrt{a \times b} = \sqrt{a} \times \sqrt{b} \) to break down the square root into simpler parts. This can sometimes make the simplification process more straightforward.
- Simplifying Before Multiplying: If you have a product of square roots, simplify each one individually before multiplying. This can often lead to simpler calculations and a clearer final answer.
- Remember the Rules: Keep in mind the basic rules of square roots and exponents, such as \( \sqrt{a^2} = a \) and \( \sqrt{ab} = \sqrt{a} \times \sqrt{b} \).
- Practice Different Problems: Practicing a variety of problems will help you become more comfortable with the process of simplifying square roots. Try problems with different levels of complexity to build your skills.
By using these tips and tricks, you'll be able to simplify square roots more effectively and with greater confidence. Keep practicing, and soon these methods will become second nature!
Practical Applications
The square root of 72 (\(\sqrt{72}\)) has various practical applications across different fields. Understanding these applications can help in grasping the importance of simplifying square roots.
1. Geometry and Construction
In geometry, \(\sqrt{72}\) is used to find the diagonal lengths of squares and rectangles when working with dimensions. For example, if a square has an area of 72 square units, the length of its diagonal is \(\sqrt{72}\).
2. Physics
In physics, the square root of 72 might be encountered when calculating magnitudes of vectors or when dealing with equations involving distance and time. For instance, in kinematic equations where square roots are used to determine resultant velocities or distances.
3. Engineering
Engineers often use square roots in designing systems and structures. For instance, \(\sqrt{72}\) can appear in calculations for stress and strain, where precise measurements are crucial for safety and efficiency.
4. Financial Calculations
In finance, square roots are used in various formulas such as calculating standard deviations, which measure volatility in stock prices. Understanding and simplifying square roots like \(\sqrt{72}\) helps in making accurate financial predictions and assessments.
5. Computer Graphics
In computer graphics, the square root function is used to calculate distances between points in 3D space. This is essential in rendering realistic images and animations. For example, determining the distance between two points in a 3D model may involve computing \(\sqrt{72}\) as part of the distance formula.
6. Simplifying Complex Calculations
Many complex mathematical problems can be simplified using square roots. For example, simplifying \(\sqrt{72}\) to \(6\sqrt{2}\) can make subsequent calculations more manageable.
By recognizing these applications, one can appreciate the utility of understanding and simplifying square roots in both academic and real-world contexts.
Cách Tốt Nhất Để Đơn Giản Hóa Căn Bậc Hai Của Một Số, Căn Bậc Hai (72)
READ MORE:
Cách Tính Căn Bậc Hai Của 72: Căn Bậc Hai (72)