Topic simplify 72 square root: Unlock the secrets to simplifying the square root of 72 with our comprehensive guide. Whether you're a student tackling math homework or a curious learner, this article will provide you with clear, easy-to-follow steps and practical applications to master simplifying square roots effortlessly. Let's simplify √72 together!
Table of Content
- Simplifying the Square Root of 72
- Introduction to Simplifying Square Roots
- Understanding Square Roots
- Prime Factorization Method
- Step-by-Step Simplification of √72
- Common Mistakes to Avoid
- Practical Applications of Simplified Square Roots
- Frequently Asked Questions
- Additional Resources and Practice Problems
- YOUTUBE: Hướng dẫn cách tốt nhất để đơn giản hóa căn bậc hai của số 72.
Simplifying the Square Root of 72
To simplify the square root of 72, we need to find the prime factors of 72 and then apply the properties of square roots.
Step-by-Step Simplification
- Find the prime factorization of 72:
- 72 = 2 × 36
- 36 = 2 × 18
- 18 = 2 × 9
- 9 = 3 × 3
- Group the prime factors into pairs:
- (2 × 2) and (3 × 3), with one 2 remaining.
- Take the square root of each pair of factors:
- The square root of 2 × 2 is 2.
- The square root of 3 × 3 is 3.
- Multiply the results of the square roots:
- 2 × 3 = 6
- Combine the results with the remaining factor:
- 6√2
So, 72 = 2 × 2 × 2 × 3 × 3.
Therefore, the simplified form of the square root of 72 is:
\[ \sqrt{72} = 6\sqrt{2} \]
Conclusion
The simplification process involves breaking down the number into its prime factors, grouping them into pairs, and taking the square root of those pairs. The simplified form of \( \sqrt{72} \) is \( 6\sqrt{2} \).

READ MORE:
Introduction to Simplifying Square Roots
Simplifying square roots is a fundamental skill in algebra that involves expressing a square root in its simplest form. This process often makes complex expressions easier to work with and understand. To simplify a square root, we typically follow a methodical approach that involves factoring and identifying perfect squares.
The square root of a number is a value that, when multiplied by itself, gives the original number. For instance, the square root of 72 is a number which, when squared, equals 72. Here's a step-by-step guide to simplifying the square root of 72:
- List Factors: Start by listing the factors of 72. These factors are numbers that multiply together to equal 72. The factors of 72 are: 1, 2, 3, 4, 6, 8, 9, 12, 18, 24, 36, and 72.
- Identify Perfect Squares: From the list of factors, identify the perfect squares. A perfect square is a number that has an integer as its square root. For 72, the perfect squares among the factors are 1, 4, 9, and 36.
-
Divide by the Largest Perfect Square: Divide 72 by the largest perfect square from the previous step. Here, 36 is the largest perfect square factor of 72. Performing the division, we get:
\( 72 \div 36 = 2 \) - Calculate the Square Root of the Perfect Square: Find the square root of the largest perfect square identified. The square root of 36 is 6.
-
Combine Results: Combine the results of the division and the square root calculation to express the original square root in its simplest form. Thus:
\( \sqrt{72} = \sqrt{36 \times 2} = \sqrt{36} \times \sqrt{2} = 6\sqrt{2} \)
By following these steps, we have simplified \( \sqrt{72} \) to \( 6\sqrt{2} \). This simplified form is often more useful in mathematical expressions and problem-solving.
Understanding Square Roots
The concept of square roots is fundamental in mathematics, providing a way to reverse the process of squaring a number. A square root of a number x is a value that, when multiplied by itself, yields x. This can be expressed as:
\[
\sqrt{x} = r \quad \text{if and only if} \quad r^2 = x
\]
Here, \(\sqrt{x}\) denotes the square root, and \(r\) is the value such that \(r^2 = x\).
Properties of Square Roots
- Every positive number has two square roots: one positive and one negative. For example, the square roots of 25 are 5 and -5 because \(5^2 = 25\) and \((-5)^2 = 25\).
- The principal square root is the non-negative square root, denoted by \(\sqrt{x}\). When we write \(\sqrt{25}\), we mean the principal square root, which is 5.
- Square roots of perfect squares (e.g., 1, 4, 9, 16) are integers, while those of non-perfect squares are irrational numbers (e.g., \(\sqrt{2}\), \(\sqrt{3}\)).
- Negative numbers do not have real square roots because a negative number squared will always be positive.
Examples
Consider the following examples to illustrate the concept:
- \(\sqrt{36} = 6\) because \(6 \times 6 = 36\).
- \(\sqrt{49} = 7\) because \(7 \times 7 = 49\).
- \(\sqrt{81} = 9\) because \(9 \times 9 = 81\).
Square Roots in Algebra
Square roots are also widely used in algebra, particularly in solving quadratic equations. For example, to solve \(x^2 - 9 = 0\), we can follow these steps:
- Add 9 to both sides: \(x^2 = 9\).
- Take the square root of both sides: \(x = \pm \sqrt{9}\).
- Simplify: \(x = \pm 3\).
Visual Representation
To visualize the concept of square roots, consider a square with an area of \(A\). The length of each side of the square is the square root of \(A\). For example, a square with an area of 49 square units has sides of length \(\sqrt{49} = 7\) units.
Understanding square roots helps in various mathematical and real-world applications, from geometry to physics, making it a crucial concept to master.
Prime Factorization Method
The prime factorization method is a straightforward approach to simplify the square root of a number by breaking it down into its prime factors. Here’s a step-by-step guide to simplify the square root of 72 using the prime factorization method:
- Prime Factorization: Begin by expressing the number 72 as a product of its prime factors.
- 72 can be factored into prime numbers as follows:
- 72 = 2 × 36
- 36 = 2 × 18
- 18 = 2 × 9
- 9 = 3 × 3
- So, 72 = 2 × 2 × 2 × 3 × 3
- Group the Factors: Next, pair the identical prime factors together.
- In the case of 72, we have three 2's and two 3's:
- (2 × 2) and (3 × 3)
- Simplify Inside the Radical: Take one number from each pair outside the square root.
- From the pairs of 2's and 3's:
- √72 = √(2 × 2 × 2 × 3 × 3)
- Since we have pairs, we take one 2 and one 3 outside:
- √72 = 2 × 3 × √2
- Multiply the Numbers Outside the Radical: Multiply the numbers taken outside the square root.
- 2 × 3 = 6
- So, √72 = 6√2
Thus, the simplified form of the square root of 72 is 6√2.
Step-by-Step Simplification of √72
Simplifying the square root of 72 involves breaking it down into simpler components. Here is a detailed, step-by-step method to achieve this:
-
Prime Factorization: Start by expressing 72 as a product of its prime factors.
\[
72 = 2 \times 2 \times 2 \times 3 \times 3
\] -
Group the Prime Factors: To simplify the square root, group the prime factors into pairs.
\[
72 = (2 \times 2) \times (3 \times 3) \times 2
\] -
Take the Square Root of Each Pair: Take the square root of each pair of prime factors.
\[
\sqrt{72} = \sqrt{(2 \times 2) \times (3 \times 3) \times 2}
\]\[
= \sqrt{(2^2) \times (3^2) \times 2}
\] -
Simplify: Simplify by taking the square root of the pairs and multiplying the results.
\[
\sqrt{2^2} = 2 \quad \text{and} \quad \sqrt{3^2} = 3
\]\[
\sqrt{72} = 2 \times 3 \times \sqrt{2}
\]\[
= 6\sqrt{2}
\]
Therefore, the simplified form of \(\sqrt{72}\) is \(6\sqrt{2}\). This method ensures that all possible simplifications are performed, resulting in the simplest form of the square root.

Common Mistakes to Avoid
When simplifying square roots, including the square root of 72, there are several common pitfalls that can lead to incorrect results. Being aware of these mistakes will help you simplify square roots more accurately and efficiently.
- Not Fully Factorizing: Failing to break down the number into all of its prime factors can result in an incomplete simplification. Always ensure you have identified all prime factors.
- Overlooking Pairs of Factors: Each pair of identical factors under the square root can be simplified as a single factor outside the radical. Missing these pairs means the square root is not fully simplified.
- Misplacing Factors: When extracting factors from under the square root, make sure you multiply them correctly outside the radical. Incorrect placement can alter the final value.
- Ignoring Remaining Factors: If any factors do not form a pair, they must remain under the square root. Forgetting to include these remaining factors in your final answer is a common error.
- Confusing Addition and Multiplication: Simplifying square roots involves multiplication of factors, not addition. Mixing up these operations can lead to incorrect results.
- Stopping Simplification Too Early: Ensure you have simplified the square root completely. Sometimes students stop too soon, missing further simplification opportunities.
By avoiding these common mistakes, you can simplify square roots more accurately, ensuring a better understanding and application of mathematical principles.
Practical Applications of Simplified Square Roots
The skill of simplifying square roots extends beyond theoretical mathematics and finds practical applications in various real-world scenarios. Here are some key areas where simplified square roots, such as √72 simplified to 6√2, are useful:
- Geometry and Architecture
In geometry, simplified square roots are often used to calculate distances, areas, and volumes. For instance, if an architect needs to determine the diagonal length of a square room with an area of 72 square units, they can use the simplified form 6√2 to easily find the length.
- Physics and Engineering
Engineers and physicists frequently use simplified square roots in their calculations. For example, when determining forces, stresses, or velocities, the ability to simplify roots can make complex calculations more manageable and less error-prone. In physics, the simplified square root is crucial in solving problems involving the Pythagorean theorem.
- Education and Problem Solving
For students, mastering the simplification of square roots is essential in algebra and geometry. This skill aids in solving equations and understanding the properties of numbers. Simplified roots like 6√2 help make sense of larger problems by breaking them down into simpler parts.
- Real-life Applications
Simplified square roots appear in various everyday contexts. For example, when calculating the area of a plot of land, designing electronic components, or even during financial computations, having a simplified form like 6√2 can streamline processes and enhance accuracy.
Overall, the ability to simplify square roots such as √72 to 6√2 is not only a mathematical convenience but also a practical tool in numerous fields, making calculations more straightforward and solutions more elegant.
Frequently Asked Questions
Here are some common questions and answers related to simplifying the square root of 72:
-
Q: What is the square root of 72?
A: The square root of 72 is approximately 8.48528137424, but it can be simplified to \( 6\sqrt{2} \).
-
Q: Why is the square root of 72 simplified to \( 6\sqrt{2} \)?
A: Simplifying \( \sqrt{72} \) involves prime factorization. We express 72 as \( 72 = 2^3 \times 3^2 \). By taking the square root, we get \( \sqrt{72} = \sqrt{2^3 \times 3^2} = \sqrt{2^2 \times 3^2 \times 2} = 6\sqrt{2} \).
-
Q: Is \( 6\sqrt{2} \) the exact value of \( \sqrt{72} \)?
A: Yes, \( 6\sqrt{2} \) is the exact simplified form of \( \sqrt{72} \). The decimal form 8.48528137424 is an approximation.
-
Q: Can \( \sqrt{72} \) be simplified further?
A: No, \( 6\sqrt{2} \) is the simplest form of \( \sqrt{72} \).
-
Q: What is the principal square root?
A: The principal square root is the non-negative root of a number. For 72, the principal square root is \( \sqrt{72} = 8.48528137424 \), or in simplified form, \( 6\sqrt{2} \).
-
Q: Are there other methods to find the square root of 72?
A: Yes, other methods include the repeated subtraction method and the long division method, though the prime factorization method is typically the simplest for this type of problem.
Additional Resources and Practice Problems
To help you master simplifying the square root of 72, here are some valuable resources and practice problems:
Online Tutorials and Lessons
- - Comprehensive lessons and practice problems on simplifying square roots, including interactive exercises and video tutorials.
- - Step-by-step video tutorials and practice problems to enhance your understanding of simplifying square roots.
Worksheets and Printable Resources
- - A collection of printable worksheets focusing on finding and simplifying square roots, suitable for various grade levels.
- - Practice problems and explanations to reinforce your skills in simplifying square roots.
Practice Problems
- Simplify \( \sqrt{72} \)
- Simplify \( \sqrt{50} \)
- Simplify \( \sqrt{98} \)
- Simplify \( \sqrt{200} \)
- Simplify \( \sqrt{32} \)
Use the resources above to solve these problems and check your answers:
| Problem | Answer |
|---|---|
| \( \sqrt{72} \) | \( 6\sqrt{2} \) |
| \( \sqrt{50} \) | \( 5\sqrt{2} \) |
| \( \sqrt{98} \) | \( 7\sqrt{2} \) |
| \( \sqrt{200} \) | \( 10\sqrt{2} \) |
| \( \sqrt{32} \) | \( 4\sqrt{2} \) |
By practicing these problems and utilizing the additional resources, you will gain a solid understanding of simplifying square roots. Happy learning!
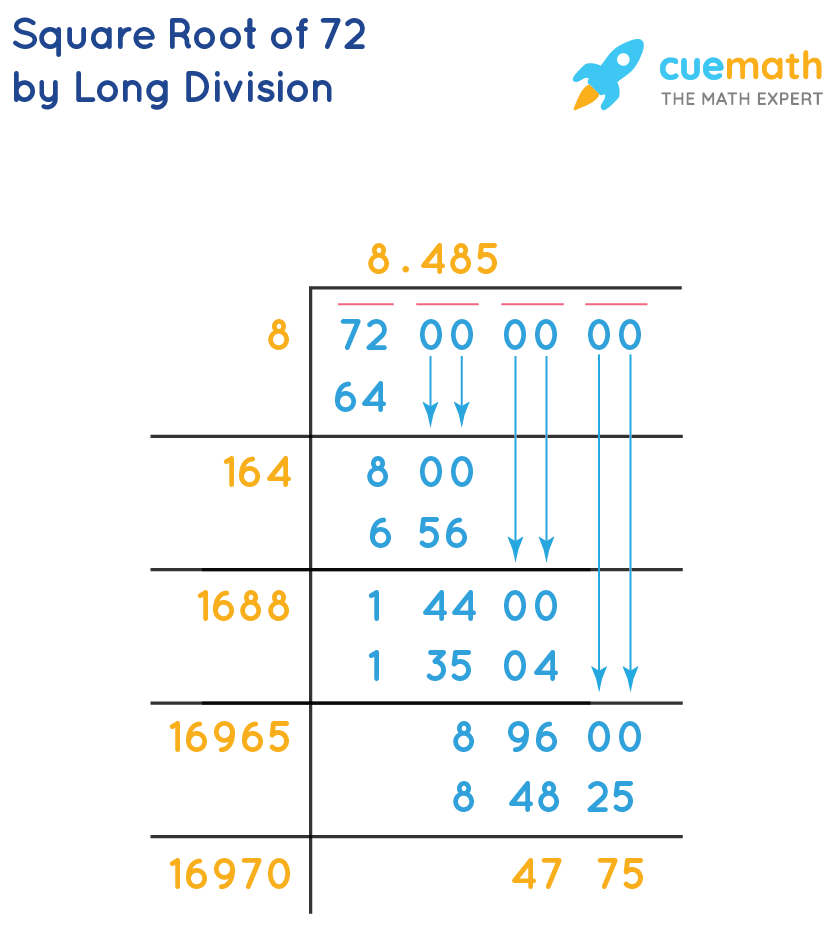
Hướng dẫn cách tốt nhất để đơn giản hóa căn bậc hai của số 72.
Cách Tốt Nhất Để Đơn Giản Hóa Căn Bậc Hai Của Một Số, √(72)
READ MORE:
Hướng dẫn cách đơn giản hóa căn bậc hai của số 72.
Căn Bậc Hai: Đơn Giản Hóa √(72)