Square Root of 72 Simplified Radical Form: A Complete Guide

The square root of 72 can be simplified by expressing 72 as a product of its prime factors.

Prime Factorization

First, let's find the prime factorization of 72:

Grouping the Factors

To simplify the square root, we group the factors into pairs:

Taking the Square Root of Each Pair

We can take the square root of each pair of numbers:

Multiplying these together:

Result

So, the simplified form of the square root of 72 is:

Thus, we can write:

The square root of a number is a value that, when multiplied by itself, gives the original number. It is one of the fundamental operations in mathematics and is symbolized by the radical sign (√). Understanding square roots is essential for solving various mathematical problems, especially those involving quadratic equations and geometry.

For example, the square root of 25 is 5 because 5 × 5 = 25. Similarly, the square root of 36 is 6 because 6 × 6 = 36. These examples illustrate perfect squares, where the result of the square root is an integer. However, not all numbers are perfect squares. When a number is not a perfect square, its square root is often expressed in its simplified radical form.

Square roots can be both positive and negative because both (5 × 5) and (-5 × -5) equal 25. However, by convention, the square root symbol (√) refers to the positive root, also known as the principal square root.

In addition to being used in solving equations, square roots have practical applications in various fields, including engineering, physics, and computer science. For instance, they are used in calculating areas and volumes, analyzing signals, and optimizing algorithms.

Understanding the concept of square roots and their simplification is crucial for progressing in higher-level math and applied sciences. The following sections will provide a comprehensive guide on simplifying square roots, using the square root of 72 as a detailed example.

The concept of simplified radical form is fundamental in algebra. It involves expressing a square root in its simplest form. This means reducing the expression to a format where no perfect square factors other than 1 remain under the radical sign. Let's explore this concept in detail.

To simplify a square root, follow these steps:

1. Identify the factors of the number: List all the factors of the number under the square root. For example, the factors of 72 are 1, 2, 3, 4, 6, 8, 9, 12, 18, 24, 36, and 72.
2. Find the largest perfect square factor: From the list of factors, identify the largest perfect square. For 72, the largest perfect square is 36.
3. Rewrite the square root: Express the square root as a product of the square root of the largest perfect square and another square root. For 72, this would be:

   \[\sqrt{72} = \sqrt{36 \times 2}\]

4. Simplify the expression: Take the square root of the perfect square and multiply it by the remaining square root. For 72, the square root of 36 is 6, so:

   \[\sqrt{72} = 6\sqrt{2}\]

This process ensures that the square root is expressed in its simplest radical form. The final result, \(6\sqrt{2}\), is the simplest form of \(\sqrt{72}\).

Understanding simplified radical forms is essential for solving algebraic equations and simplifying expressions involving square roots. It also helps in performing more complex mathematical operations where radicals are involved.

The prime factorization method is a powerful technique to simplify the square root of a number by breaking it down into its prime factors. Let's apply this method to simplify the square root of 72.

1. Find the Prime Factors of 72: Begin by decomposing 72 into its prime factors. The prime factorization of 72 is:

   \[ 72 = 2 \times 2 \times 2 \times 3 \times 3 \]

2. Group the Prime Factors in Pairs: Next, group the prime factors in pairs to prepare for taking the square root:

   \[ 72 = (2 \times 2) \times (3 \times 3) \times 2 \]

3. Take the Square Root of Each Pair: Take the square root of each pair of prime factors. For the remaining unpaired factor, leave it inside the square root symbol:

   \[ \sqrt{72} = \sqrt{(2 \times 2) \times (3 \times 3) \times 2} \]

   \[ = 2 \times 3 \times \sqrt{2} \]

4. Combine the Results: Multiply the results from the previous step to get the simplified form of the square root:

   \[ = 6 \sqrt{2} \]

Therefore, the simplified radical form of the square root of 72 is:

\[ \sqrt{72} = 6 \sqrt{2} \]

To simplify the square root of 72, we can follow a systematic method. Here is a step-by-step guide:

1. Prime Factorization: Begin by finding the prime factorization of 72. This involves breaking down 72 into its prime factors:

   \[ 72 = 2 \times 2 \times 2 \times 3 \times 3 \]

2. Group the Prime Factors: Next, group the prime factors into pairs of the same number:

   \[ 72 = (2 \times 2) \times 2 \times (3 \times 3) \]

3. Simplify the Radical: Take the square root of each pair of prime factors and move them outside the radical:

   \[ \sqrt{72} = \sqrt{(2 \times 2) \times 2 \times (3 \times 3)} \]

   \[ \sqrt{72} = 2 \times 3 \times \sqrt{2} \]

   \[ \sqrt{72} = 6 \sqrt{2} \]

4. Final Simplified Form: The simplified radical form of the square root of 72 is:

   \[ \sqrt{72} = 6 \sqrt{2} \]

By following these steps, we have simplified the square root of 72 to its simplest radical form, which is \(6 \sqrt{2}\). This method ensures accuracy and helps in understanding the concept of simplifying square roots.

To simplify the square root of 72, we follow these detailed steps:

1. Prime Factorization:

   First, we find the prime factors of 72.

   • 72 = 2 × 2 × 2 × 3 × 3

2. Group the Factors:

   Next, we group the prime factors in pairs:

   • 72 = (2 × 2) × (2) × (3 × 3)

3. Apply the Square Root:

   We then apply the square root to the grouped factors:

   • \(\sqrt{72} = \sqrt{(2 \times 2) \times (2) \times (3 \times 3)}\)
   • \(\sqrt{72} = \sqrt{4 \times 18}\)

4. Simplify the Radicals:

   We can simplify the square root of each perfect square:

   • \(\sqrt{4} = 2\)
   • \(\sqrt{18} = \sqrt{9 \times 2} = 3\sqrt{2}\)

5. Combine the Results:

   Finally, we combine the simplified parts:

   • \(\sqrt{72} = 2 \times 3\sqrt{2}\)
   • \(\sqrt{72} = 6\sqrt{2}\)

Thus, the simplified radical form of the square root of 72 is \(6\sqrt{2}\).

When simplifying the square root of 72, there are several common mistakes that can lead to incorrect results. Being aware of these pitfalls can help ensure accuracy and enhance your understanding of radical simplification. Here are some common mistakes to avoid:

• Not Fully Factorizing:

  Failing to break down the number into all of its prime factors can lead to an incorrect simplification. Ensure that you factorize 72 completely into \(2 \times 2 \times 2 \times 3 \times 3\).

• Overlooking Pairs of Factors:

  Each pair of identical factors under the square root can be taken out as a single factor. Missing a pair means not simplifying the square root fully. For 72, we have pairs of 2s and 3s, which should be extracted correctly.

• Misplacing Factors:

  When extracting factors from under the square root, ensure you multiply them correctly outside the radical. Misplacement can alter the value of the expression. For example, extracting pairs of 2s and 3s correctly results in \(6\sqrt{2}\).

• Ignoring Remaining Factors:

  If any factors under the square root do not form a pair, they must remain under the square root. Forgetting to include these factors in your final answer is a common mistake. For 72, after extracting pairs, the remaining factor under the root is 2.

• Confusing Addition and Multiplication:

  Remember that the rules for simplifying square roots involve multiplication of factors, not addition. Confusing these operations can lead to incorrect results. Always multiply the extracted factors outside the radical.

• Mathematical Errors in Simplification:

  Simple arithmetic errors during the final calculation steps can lead to incorrect results. Double-check your work for accuracy to avoid these mistakes.

By paying close attention to these details and steps, you can avoid common mistakes and ensure the correct simplification of the square root of 72, yielding the accurate result of \(6\sqrt{2}\).

Simplifying the square root of 72 can be approached in various ways. Here are some alternative methods:

1. Prime Factorization Method

This method involves breaking down the number into its prime factors.

1. Find the prime factors of 72: \(72 = 2^3 \times 3^2\).
2. Group the prime factors into pairs: \(72 = (2 \times 2) \times (3 \times 3) \times 2\).
3. Take one factor from each pair outside the square root: \(\sqrt{72} = \sqrt{(2^2) \times (3^2) \times 2} = 2 \times 3 \times \sqrt{2}\).
4. Simplify: \(\sqrt{72} = 6\sqrt{2}\).

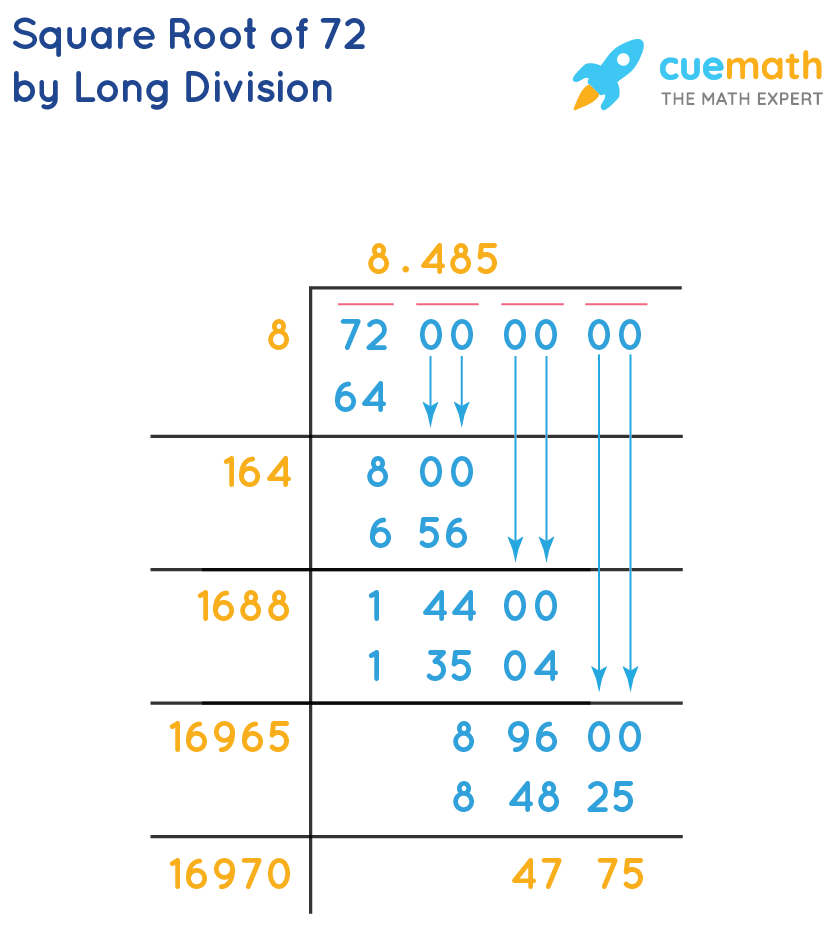
2. Long Division Method

This method is useful for finding more precise decimal values of square roots.

1. Set up the long division for 72. Pair the digits from right to left.
2. Find the largest number whose square is less than or equal to 72 (8 in this case, as \(8^2 = 64\)).
3. Subtract and bring down pairs of zeros, continuing the process to get more decimal places.
4. The approximate value of \(\sqrt{72}\) using this method is 8.485.

3. Using Perfect Squares

This method involves recognizing and using perfect squares within the number.

1. Identify the largest perfect square factor of 72, which is 36.
2. Rewrite 72 as a product of 36 and 2: \(72 = 36 \times 2\).
3. Express the square root: \(\sqrt{72} = \sqrt{36 \times 2} = \sqrt{36} \times \sqrt{2} = 6\sqrt{2}\).

4. Estimation Method

For a quick estimate, use numbers close to 72 that are perfect squares.

• Find the square roots of perfect squares around 72, such as 64 and 81. Their square roots are 8 and 9, respectively.
• Since 72 is closer to 64, \(\sqrt{72}\) will be slightly more than 8. Using approximation, \(\sqrt{72} \approx 8.5\).

Simplified radicals, such as the square root of 72 in its simplest form (\(6\sqrt{2}\)), are not just theoretical constructs but have practical applications in various fields. Here are some ways simplified radicals are used:

• Geometry and Trigonometry: Simplified radicals often appear in calculations involving geometric shapes. For example, the diagonal of a square with side length 6 can be expressed as \(6\sqrt{2}\). This is useful in architectural design and computer graphics.
• Physics: In physics, radicals are used in formulas for calculating distances, velocities, and other measurements that involve square roots. For instance, the formula for the period of a pendulum involves the square root of its length.
• Engineering: Engineers use simplified radicals when dealing with wave frequencies, resonance frequencies, and other calculations that require precise measurements.
• Finance: In finance, radicals can appear in models that involve growth rates, such as compound interest calculations where the time period is expressed under a square root.
• Computer Science: Algorithms often involve square roots and their simplified forms, especially in areas like cryptography, where large primes and their properties are essential.
• Astronomy: Calculations of distances between celestial objects, which involve large numbers, often make use of simplified radicals to express these distances more succinctly.
• Daily Life: Simplified radicals can also help in everyday calculations, such as determining the size of a TV screen given the diagonal or the dimensions of a space.

Understanding and using simplified radicals allow for more efficient and accurate calculations in these and many other applications.

Practicing simplification of square roots helps reinforce the concepts and techniques used. Here are some practice problems along with their solutions to aid in understanding.

Problem 1: Simplify \(\sqrt{72}\)

Solution:

1. Prime factorize 72: \(72 = 2^3 \times 3^2\).
2. Rewrite the square root using the prime factors: \(\sqrt{72} = \sqrt{2^3 \times 3^2}\).
3. Group the prime factors into pairs: \(\sqrt{72} = \sqrt{(2^2 \times 3^2) \times 2}\).
4. Take the square root of the paired factors: \(\sqrt{72} = \sqrt{(2^2) \times (3^2) \times 2} = 2 \times 3 \times \sqrt{2}\).
5. Simplify: \(\sqrt{72} = 6\sqrt{2}\).

Problem 2: Simplify \(\sqrt{50}\)

Solution:

1. Prime factorize 50: \(50 = 2 \times 5^2\).
2. Rewrite the square root using the prime factors: \(\sqrt{50} = \sqrt{2 \times 5^2}\).
3. Take the square root of the paired factor: \(\sqrt{50} = 5 \times \sqrt{2}\).
4. Simplify: \(\sqrt{50} = 5\sqrt{2}\).

Problem 3: Simplify \(\sqrt{98}\)

Solution:

1. Prime factorize 98: \(98 = 2 \times 7^2\).
2. Rewrite the square root using the prime factors: \(\sqrt{98} = \sqrt{2 \times 7^2}\).
3. Take the square root of the paired factor: \(\sqrt{98} = 7 \times \sqrt{2}\).
4. Simplify: \(\sqrt{98} = 7\sqrt{2}\).

Problem 4: Simplify \(\sqrt{200}\)

Solution:

1. Prime factorize 200: \(200 = 2^3 \times 5^2\).
2. Rewrite the square root using the prime factors: \(\sqrt{200} = \sqrt{2^3 \times 5^2}\).
3. Group the prime factors into pairs: \(\sqrt{200} = \sqrt{(2^2 \times 5^2) \times 2}\).
4. Take the square root of the paired factors: \(\sqrt{200} = 2 \times 5 \times \sqrt{2}\).
5. Simplify: \(\sqrt{200} = 10\sqrt{2}\).

Additional Practice Problems

• Simplify \(\sqrt{32}\).
• Simplify \(\sqrt{45}\).
• Simplify \(\sqrt{63}\).
• Simplify \(\sqrt{75}\).

These practice problems will help solidify your understanding of simplifying square roots and identifying common mistakes to avoid.

In conclusion, simplifying the square root of 72 involves breaking down the number into its prime factors and identifying perfect squares. Through this process, we determined that the simplified form of \(\sqrt{72}\) is \(6\sqrt{2}\). This method not only simplifies the expression but also makes it easier to work with in various mathematical contexts.

Understanding and applying the concept of simplified radical forms can significantly enhance one's ability to solve problems involving square roots efficiently. By following the prime factorization method, you can simplify square roots of other non-perfect squares as well.

We have explored various methods to find the simplified radical form, identified common mistakes to avoid, and examined practical applications. Simplifying radicals is a fundamental skill in mathematics, useful in algebra, geometry, and beyond.

We hope this comprehensive guide has provided clarity and a thorough understanding of simplifying the square root of 72. Continue practicing with different numbers to strengthen your skills and confidence in dealing with radicals.

• What is the simplified radical form of the square root of 72?

The simplified radical form of the square root of 72 is \(6\sqrt{2}\). This is derived by breaking down 72 into its prime factors (2 x 2 x 2 x 3 x 3) and simplifying accordingly.

• How do you simplify the square root of 72 step by step?


To simplify \(\sqrt{72}\):




1. Perform prime factorization: \(72 = 2^3 \times 3^2\).


2. Group the prime factors into pairs: \(72 = (2^2 \times 3^2) \times 2\).


3. Take one factor from each pair out of the square root: \(\sqrt{72} = \sqrt{(2^2 \times 3^2) \times 2} = 6\sqrt{2}\).




• Why is the square root of 72 not a whole number?

The square root of 72 is not a whole number because 72 is not a perfect square. A perfect square is an integer that is the square of another integer. Since no integer squared equals 72, its square root is an irrational number.

• Can the square root of 72 be expressed as a decimal?

Yes, the square root of 72 can be expressed as a decimal. It is approximately equal to 8.485. However, this is an approximation, and the exact value is better represented in its simplified radical form, \(6\sqrt{2}\).

• What are some common mistakes when simplifying the square root of 72?


Common mistakes include:




• Not performing complete prime factorization.


• Incorrectly grouping the factors.


• Mathematical errors during calculation.


• Forgetting to simplify completely by not taking out all pairs of factors.




• What are the practical applications of knowing the simplified form of \(\sqrt{72}\)?

The simplified form of \(\sqrt{72}\) can be used in various fields such as geometry (for calculating diagonal lengths), physics (for solving equations involving speed and energy), engineering (for design and structural calculations), and statistics (for calculating standard deviations).