Square Root of 20 in Simplest Radical Form: A Step-by-Step Guide

Finding the simplest radical form of the square root of 20 involves breaking down the number into its prime factors and simplifying.

Step-by-Step Process

1. First, find the prime factorization of 20: \(20 = 2^2 \times 5\).
2. Next, write the square root of 20 using its prime factors: \(\sqrt{20} = \sqrt{2^2 \times 5}\).
3. Separate the factors inside the square root: \(\sqrt{2^2} \times \sqrt{5}\).
4. Simplify the square root of the perfect square: \(2 \times \sqrt{5}\).

Simplified Form

Therefore, the square root of 20 in its simplest radical form is:

\(\sqrt{20} = 2\sqrt{5}\)

Summary Table

This simplification helps in various mathematical calculations and makes the expression easier to work with.

The square root of 20, denoted as √20, can be simplified to its simplest radical form using mathematical techniques. Understanding how to simplify square roots helps in various mathematical problems and is an essential skill for students. This section will guide you step-by-step on how to simplify √20 effectively.

1. First, we factorize 20 into its prime factors:
   • 20 = 2 × 10
   • 10 = 2 × 5
2. Combining these, we get:

   20 = 2 × 2 × 5

3. Taking the square root of both sides:

   √20 = √(2 × 2 × 5)

4. Simplifying the expression by pairing the 2s:

   √20 = 2√5

Thus, the simplest radical form of √20 is 2√5. This process involves breaking down the number into its prime factors and then simplifying the square root by identifying pairs of factors.

Square roots are fundamental in mathematics and are used to determine a number which, when multiplied by itself, gives the original number. For instance, the square root of 20, denoted as √20, can be simplified to its simplest radical form for easier manipulation in mathematical equations.

To find the square root of a number, we often use methods such as prime factorization and the long division method. Let's explore these methods step by step for the number 20.

1. Prime Factorization:
   • First, factorize 20 into its prime factors: 20 = 2 × 2 × 5.
   • Next, group the prime factors into pairs: (2 × 2) and 5.
   • The pair (2 × 2) can be taken out of the square root, resulting in 2√5.
   • Thus, the simplest radical form of √20 is 2√5.
2. Long Division Method:
   • Estimate a number (n) whose square is less than 20. Here, 4 is a good guess since 4 × 4 = 16.
   • Use 4 as the divisor and quotient: divide 20 by 4 to get a quotient of 4 and a remainder of 4.
   • Double the quotient and use it to form the new divisor (8). Bring down the next pair of zeros.
   • Continue the division process to refine the value: √20 ≈ 4.4721359549...

By understanding these methods, we can easily determine that the square root of 20 in its simplest radical form is 2√5, and its decimal approximation is approximately 4.4721. This knowledge helps in solving various mathematical problems involving square roots.

Prime factorization is a method used to express a number as the product of its prime factors. For the square root of 20, we can use prime factorization to simplify the radical form.

1. First, factorize the number 20. Since 20 is even, it is divisible by 2:
   • 20 = 2 × 10
2. Next, factorize 10:
   • 10 = 2 × 5
3. Combine these factorizations:
   • 20 = 2 × 2 × 5
4. Now, express 20 as the product of its prime factors:
   • 20 = 2² × 5
5. Take the square root of both sides:
   • √20 = √(2² × 5)
6. Separate the square root into individual roots:
   • √20 = √(2²) × √5
7. Simplify the square root of 2²:
   • √(2²) = 2
8. Combine the simplified terms:
   • √20 = 2√5

Therefore, the square root of 20 in its simplest radical form is 2√5. This method ensures that the radical is simplified to its lowest terms, making it easier to understand and use in further calculations.

The square root of 20 can be simplified to its simplest radical form. This process involves breaking down the number 20 into its prime factors and then simplifying it step by step. Here is a detailed guide on how to achieve this:

1. First, find the prime factorization of 20. The prime factors of 20 are 2 and 5.
2. Express 20 as a product of its prime factors: \(20 = 2 \times 2 \times 5\).
3. Group the prime factors into pairs: \(2 \times 2\) and \(5\).
4. Simplify the pairs: the square root of \(2 \times 2\) is 2, so we have \(2 \sqrt{5}\).

Therefore, the square root of 20 in its simplest radical form is \(2 \sqrt{5}\).

For those interested in the decimal form, the square root of 20 is approximately 4.472.

To simplify the square root of 20, follow these detailed steps:

1. Prime Factorization: Start by factorizing 20 into its prime factors.

   • 20 = 2 × 10
   • 10 = 2 × 5
   • So, 20 = 2 × 2 × 5

2. Grouping Factors: Group the factors into pairs of the same number under the square root.

   √20 = √(2 × 2 × 5)

3. Simplifying the Radical: Extract the pairs from under the radical.

   √(2 × 2 × 5) = 2√5

4. Result: The simplest radical form of √20 is 2√5.

By following these steps, you can simplify the square root of 20 to its simplest radical form, 2√5, easily and accurately.

To simplify the square root of 20, it is essential to identify the perfect squares within the number. A perfect square is a number that can be expressed as the product of an integer with itself. For example, \(1, 4, 9, 16,\) and \(25\) are perfect squares because they are \(1^2, 2^2, 3^2, 4^2,\) and \(5^2\) respectively.

When breaking down 20, we look for the largest perfect square that is a factor of 20:

• 20 can be factored into \(4 \times 5\)
• 4 is a perfect square (\(2^2\))

Thus, the square root of 20 can be simplified by separating the perfect square from the non-perfect square:

• \(\sqrt{20} = \sqrt{4 \times 5}\)
• Using the product property of square roots: \(\sqrt{4 \times 5} = \sqrt{4} \times \sqrt{5}\)
• Simplifying \(\sqrt{4}\): \(\sqrt{4} = 2\)

Therefore, the simplified form of \(\sqrt{20}\) is \(2\sqrt{5}\).

Simplifying non-perfect squares involves breaking down the radicand into its prime factors and identifying any perfect square factors. Here is a detailed, step-by-step process to simplify the square root of 20:

1. Identify the Factors:

   First, identify the factors of 20: 1, 2, 4, 5, 10, and 20.

2. Find the Perfect Squares:

   From the factors, identify the perfect squares. In this case, 4 is the perfect square factor of 20.

3. Divide by the Perfect Square:

   Divide 20 by the largest perfect square you identified:

   \( \frac{20}{4} = 5 \)

4. Simplify the Square Root:

   Rewrite the square root of 20 as the product of the square root of 4 and the square root of 5:

   \( \sqrt{20} = \sqrt{4 \times 5} = \sqrt{4} \times \sqrt{5} \)

5. Calculate the Square Root of the Perfect Square:

   Since the square root of 4 is 2, you can simplify further:

   \( \sqrt{20} = 2 \sqrt{5} \)

Thus, the square root of 20 in its simplest radical form is \( 2 \sqrt{5} \). This step-by-step method can be applied to any non-perfect square to simplify it effectively.

Once we have simplified the square root of 20 to its simplest radical form, which is \(2\sqrt{5}\), we need to understand how to combine it with other terms. Here’s a step-by-step guide:

1. Identify Like Terms: When combining radical expressions, ensure that the terms under the radicals are the same. For example, \(2\sqrt{5}\) and \(3\sqrt{5}\) can be combined because they both have \(\sqrt{5}\) as a factor.
2. Add or Subtract the Coefficients: If the radicals are the same, you can add or subtract the coefficients. For example:
   • \(2\sqrt{5} + 3\sqrt{5} = (2 + 3)\sqrt{5} = 5\sqrt{5}\)
   • \(5\sqrt{5} - \sqrt{5} = (5 - 1)\sqrt{5} = 4\sqrt{5}\)
3. Multiply and Simplify: When multiplying radical terms, you multiply the coefficients and the radicands (the numbers under the radical) separately. For example:
   • \(2\sqrt{5} \times 3\sqrt{2} = (2 \times 3)(\sqrt{5} \times \sqrt{2}) = 6\sqrt{10}\)
4. Divide and Simplify: Similarly, when dividing radical terms, you divide the coefficients and the radicands separately. For example:
   • \(\frac{6\sqrt{10}}{2\sqrt{5}} = \frac{6}{2} \times \frac{\sqrt{10}}{\sqrt{5}} = 3 \times \sqrt{2} = 3\sqrt{2}\)

Combining simplified terms correctly is crucial for solving equations and simplifying expressions in mathematics. Practice with different examples to master this technique.

To express the square root of 20 in its simplest radical form, follow these steps:

1. Factorize the Number: Break down 20 into its prime factors:

   \(20 = 2 \times 10\)

   Further factorizing 10, we get:

   \(10 = 2 \times 5\)

   Therefore, the prime factorization of 20 is:

   \(20 = 2 \times 2 \times 5\)

2. Group the Factors: Identify pairs of factors to simplify the radical:

   \(\sqrt{20} = \sqrt{2 \times 2 \times 5}\)

3. Simplify the Radical: Extract the pairs out of the square root:

   \(\sqrt{2 \times 2 \times 5} = \sqrt{2^2 \times 5} = 2\sqrt{5}\)

Therefore, the final simplified form of the square root of 20 is:

\(\sqrt{20} = 2\sqrt{5}\)

This simplified radical form is useful in various mathematical contexts, making calculations more manageable and providing a clear understanding of the underlying factors.

Simplified radical forms have numerous practical applications in various fields such as geometry, algebra, engineering, and physics. Understanding how to simplify square roots can make complex calculations easier and more intuitive. Here are some key applications:

• Geometry:

  In geometry, simplified radicals are often used to express lengths of sides in right triangles, areas, and other measurements. For example, if the area of a square is 20 square units, the side length of the square is the square root of 20, which simplifies to \(2\sqrt{5}\) units.

• Trigonometry:

  Simplified radicals are used in trigonometric functions to express the lengths of sides in special triangles, such as 30-60-90 triangles and 45-45-90 triangles. These values are critical in solving trigonometric equations and problems.

• Algebra:

  In algebra, simplified radicals are used to solve quadratic equations and other polynomial equations. For instance, when solving for the roots of an equation, the results may include square roots that need to be simplified for clearer expression and further calculation.

• Physics and Engineering:

  In physics and engineering, simplified radicals help in solving problems related to wave functions, quantum mechanics, and material science. For example, calculating the diagonal distance across a rectangular surface may involve simplifying the square root of a sum of squares.

• Construction and Architecture:

  Architects and builders use simplified radicals to determine the lengths of materials needed for various projects. For example, when calculating the length of a ramp or the diagonal support in a truss, the square roots of certain measurements need to be simplified for practical use.

• Computer Graphics:

  In computer graphics, simplified radicals are used in algorithms that calculate distances and transformations in 3D space. For instance, the distance between two points in 3D space often involves square roots that are simplified to optimize the computations.

Understanding and applying simplified radical forms is essential in these fields to ensure accuracy and efficiency in calculations.

When simplifying the square root of 20 to its simplest radical form, there are several common mistakes that students often make. Here are some key points to keep in mind to avoid these errors:

• Incorrect Prime Factorization: Ensure you correctly factorize 20 into its prime factors. The correct factorization is:

  20 = 2 × 2 × 5

  Avoid mistakes such as missing a factor or misidentifying the prime factors.

• Forgetting to Pair Factors: When simplifying the square root, remember to look for pairs of prime factors. In this case, we have one pair of 2s:

  \(\sqrt{20} = \sqrt{2 \times 2 \times 5} = \sqrt{2^2 \times 5}\)

  This allows you to take 2 out of the square root.

• Improper Simplification: After identifying pairs, simplify the expression correctly:

  \(\sqrt{20} = \sqrt{2^2 \times 5} = 2\sqrt{5}\)

  Some students might incorrectly leave the expression inside the square root or make arithmetic errors.

• Not Identifying Perfect Squares: Recognize and utilize perfect squares to simplify the radical. For 20, the perfect square is 4 (since \(4 = 2^2\)), which simplifies the process.

• Mixing Up Decimal and Radical Forms: Understand that \(\sqrt{20}\) and its simplified radical form \(2\sqrt{5}\) are exact, whereas using decimal approximations like 4.47 can lead to inaccuracies in further calculations.

By carefully following these steps and being mindful of common pitfalls, you can correctly simplify the square root of 20 and apply these principles to other similar problems.

Here are some additional examples and practice problems to help you understand how to simplify square roots:

Example 1: Simplify √50

1. Prime factorize 50: 50 = 2 × 5 × 5.
2. Group the prime factors into pairs: √(2 × 5 × 5).
3. Take the square root of each pair: 5√2.
4. Final answer: √50 = 5√2.

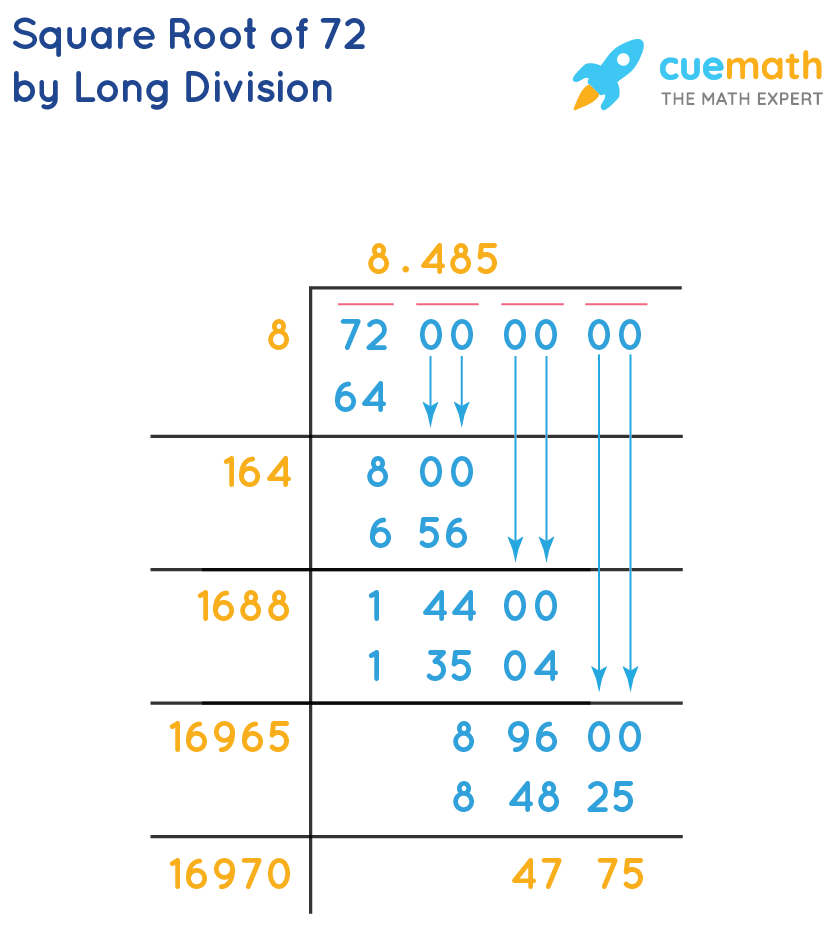
Example 2: Simplify √72

1. Prime factorize 72: 72 = 2 × 2 × 2 × 3 × 3.
2. Group the prime factors into pairs: √(2 × 2 × 2 × 3 × 3).
3. Take the square root of each pair: 6√2.
4. Final answer: √72 = 6√2.

Practice Problems

• Simplify √18
• Simplify √45
• Simplify √98
• Simplify √200

For each practice problem, follow these steps:

1. Prime factorize the number under the radical.
2. Group the prime factors into pairs.
3. Take the square root of each pair.
4. Write the final simplified form.

Example Solutions

• √18 = 3√2
• √45 = 3√5
• √98 = 7√2
• √200 = 10√2

Practice these problems to strengthen your understanding of simplifying square roots. Remember, the key steps are to prime factorize, group into pairs, and then simplify.

Understanding the square root of 20 in its simplest radical form provides a solid foundation for tackling more complex mathematical problems. By breaking down the square root into its prime factors, we identified that 20 can be expressed as 2 × 2 × 5. This allowed us to simplify √20 to 2√5, as the pair of 2s could be taken out from under the radical sign, leaving √5 inside.

This simplification not only makes calculations more manageable but also demonstrates the elegance of mathematics in reducing expressions to their simplest forms. The process highlights the importance of prime factorization and recognizing perfect squares, which are crucial skills in various areas of math.

Applications of simplified radical forms are numerous, from solving equations to simplifying complex expressions in algebra and calculus. Knowing how to simplify square roots is essential for students, as it enhances their problem-solving capabilities and prepares them for more advanced mathematical concepts.

By avoiding common mistakes, such as misidentifying factors or incorrect simplification steps, students can confidently approach problems involving square roots. Practicing with additional examples and problems will reinforce these skills and ensure a strong grasp of the topic.

Ultimately, the ability to simplify the square root of 20 to 2√5 serves as a valuable tool in a mathematician’s toolkit, exemplifying the clarity and precision that mathematics strives to achieve.