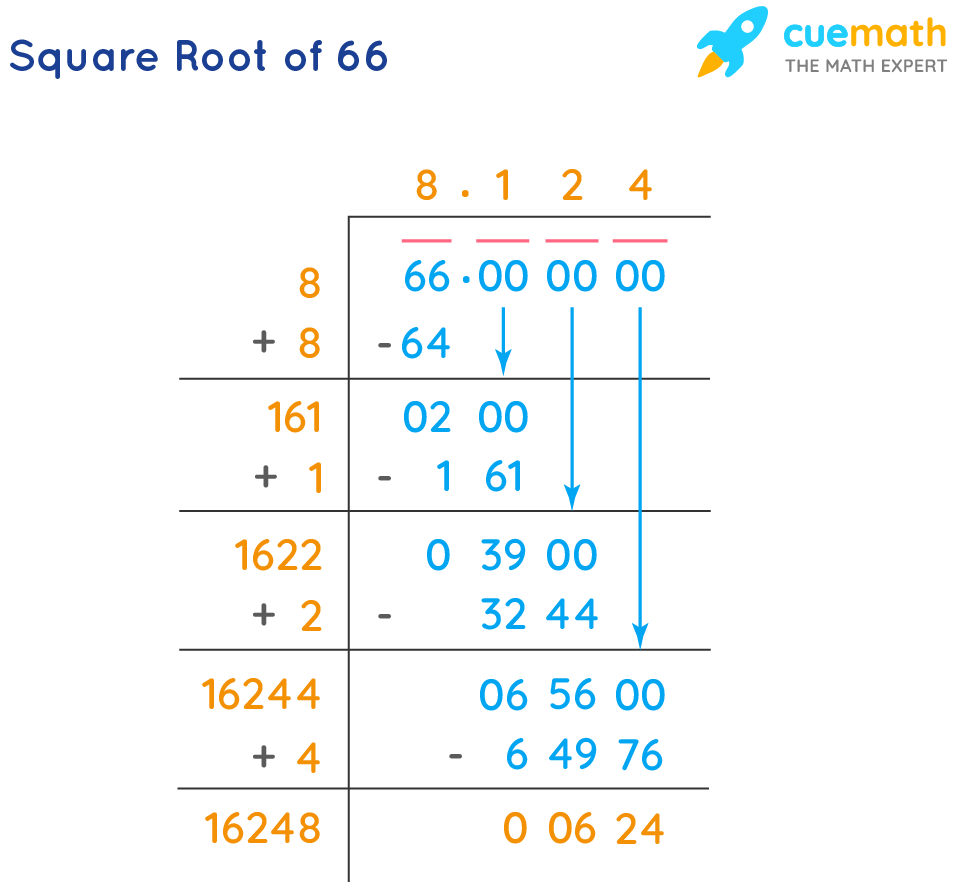
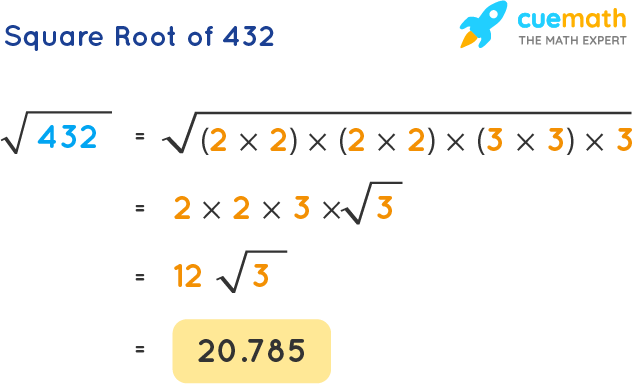
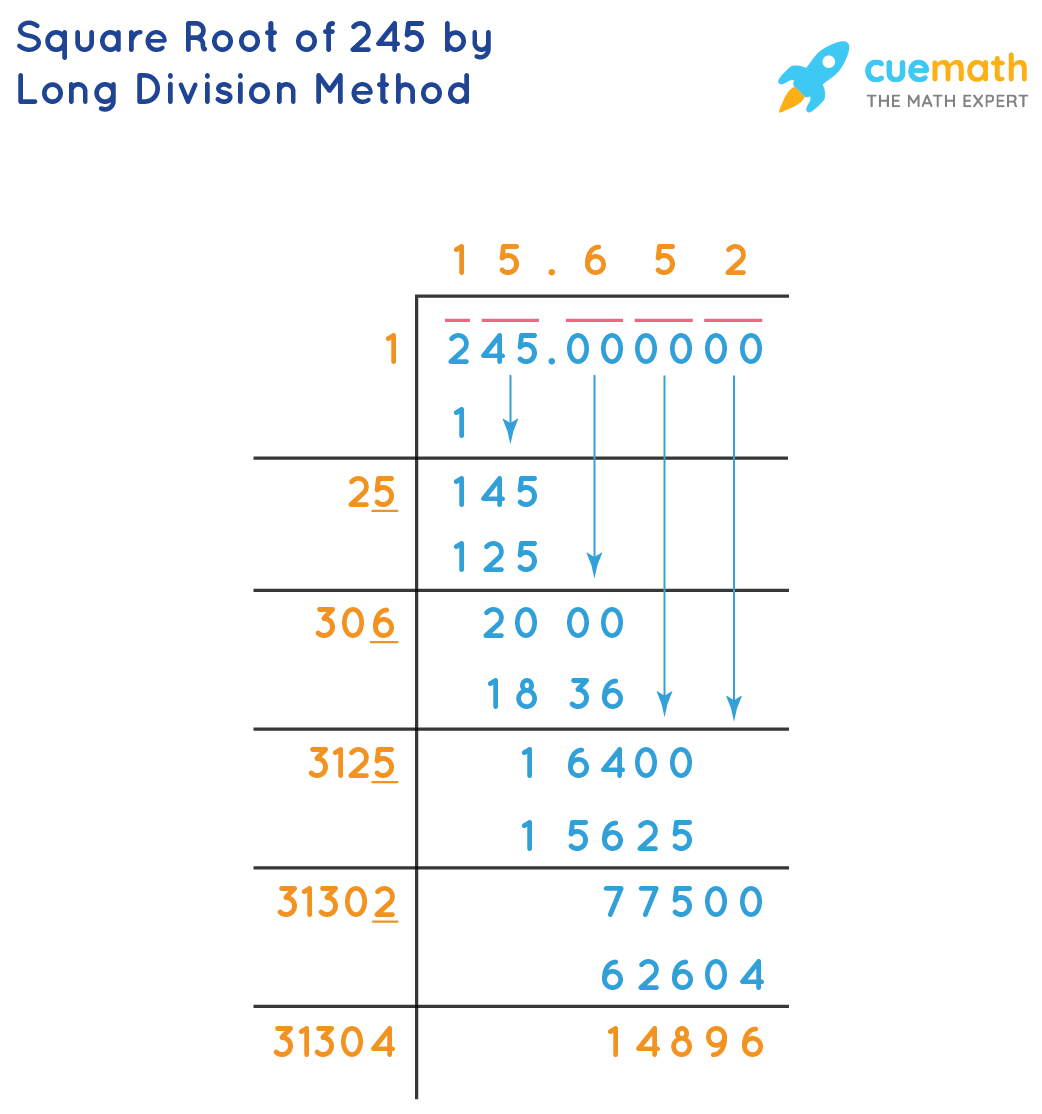
Topic how to solve using square roots: Unlock the power of solving equations with our comprehensive guide on how to solve using square roots. This article simplifies the process, offering step-by-step instructions, practical examples, and tips to master this essential mathematical technique. Perfect for students, educators, and math enthusiasts looking to enhance their problem-solving skills.
Table of Content
- Steps to Solve Quadratic Equations Using Square Roots
- Additional Examples
- Additional Examples
- Introduction to Solving Equations Using Square Roots
- Understanding the Square Root Property
- Step-by-Step Process of Solving Quadratic Equations
- Solving Perfect Square Equations
- Solving Non-Perfect Square Equations
- Examples and Practice Problems
- Special Cases in Solving Square Root Equations
- Checking Your Solutions
- Common Mistakes to Avoid
- Advanced Techniques and Tips
- Applications of Square Root Solving in Real Life
- Additional Resources and References
- YOUTUBE: Video hướng dẫn sử dụng phương pháp căn bậc hai để giải phương trình bậc hai, giúp người xem dễ dàng nắm bắt kiến thức cơ bản.
Steps to Solve Quadratic Equations Using Square Roots
- Isolate the quadratic term: Ensure that the term with the variable squared is alone on one side of the equation.
- Apply the Square Root Property: Take the square root of both sides of the equation. Remember to consider both the positive and negative square roots.
- Simplify the results: If necessary, simplify the expression under the square root.
- Check the solutions: Substitute the solutions back into the original equation to verify they are correct.
Example 1: Solving \(x^2 = 9\)
- Isolate the quadratic term: \(x^2 = 9\)
- Apply the Square Root Property: \(x = \pm \sqrt{9}\)
- Simplify: \(x = \pm 3\)
- Check the solutions: Substitute \(x = 3\) and \(x = -3\) back into the original equation.
Example 2: Solving \(x^2 = 7\)
- Isolate the quadratic term: \(x^2 = 7\)
- Apply the Square Root Property: \(x = \pm \sqrt{7}\)
- Simplify: Since \(\sqrt{7}\) cannot be simplified further, the solutions are \(x = \sqrt{7}\) and \(x = -\sqrt{7}\)
- Check the solutions: Substitute \(x = \sqrt{7}\) and \(x = -\sqrt{7}\) back into the original equation.
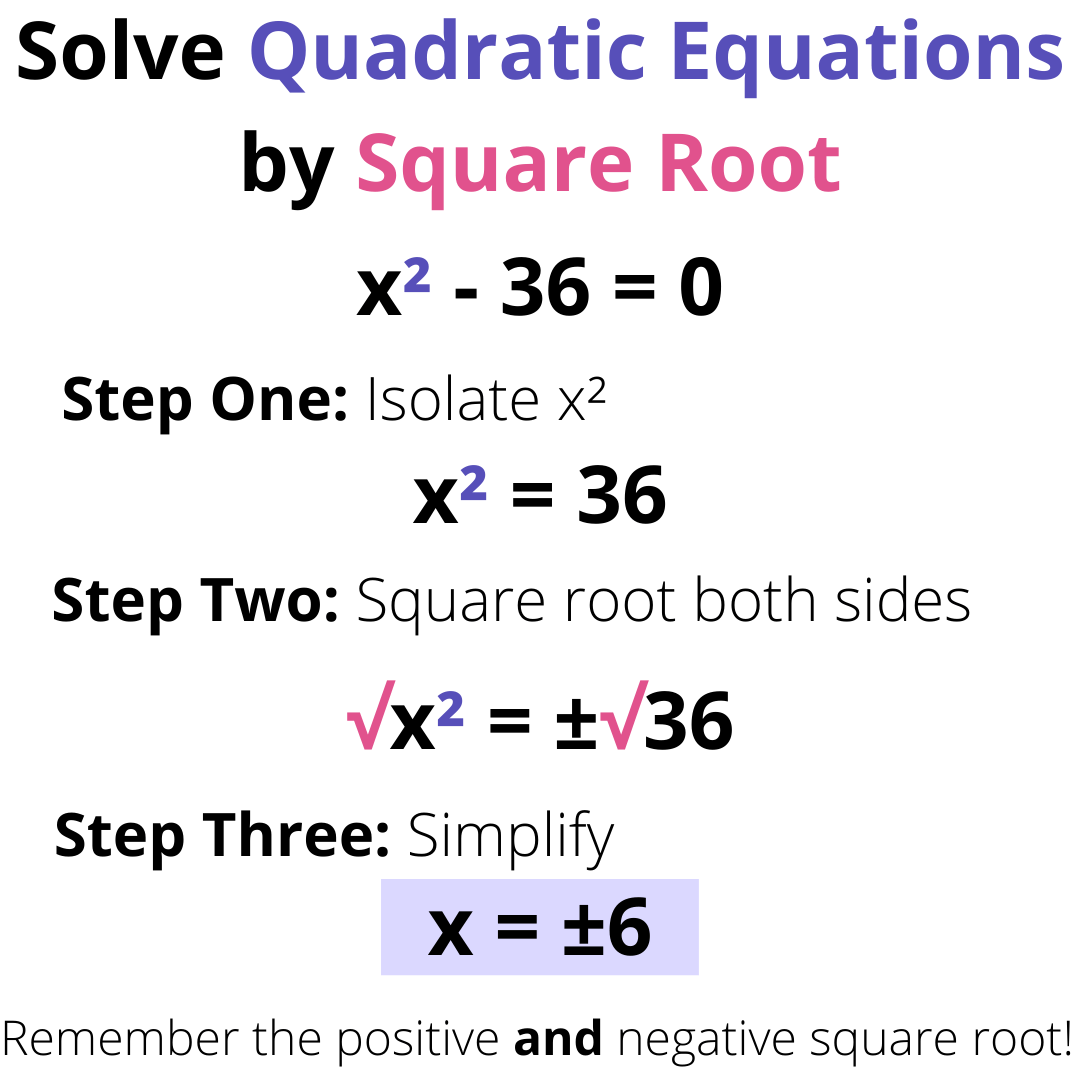
Example 3: Solving \(x^2 - 50 = 0\)
- Isolate the quadratic term: \(x^2 = 50\)
- Apply the Square Root Property: \(x = \pm \sqrt{50}\)
- Simplify: \(x = \pm 5\sqrt{2}\)
- Check the solutions: Substitute \(x = 5\sqrt{2}\) and \(x = -5\sqrt{2}\) back into the original equation.

READ MORE:
Additional Examples
- Example 4: Solve \(3z^2 = 108\)
- Isolate the quadratic term: \(z^2 = 36\)
- Apply the Square Root Property: \(z = \pm \sqrt{36}\)
- Simplify: \(z = \pm 6\)
- Check the solutions: Substitute \(z = 6\) and \(z = -6\) back into the original equation.
- Example 5: Solve \(y^2 - 27 = 0\)
- Isolate the quadratic term: \(y^2 = 27\)
- Apply the Square Root Property: \(y = \pm \sqrt{27}\)
- Simplify: \(y = \pm 3\sqrt{3}\)
- Check the solutions: Substitute \(y = 3\sqrt{3}\) and \(y = -3\sqrt{3}\) back into the original equation.
Additional Examples
- Example 4: Solve \(3z^2 = 108\)
- Isolate the quadratic term: \(z^2 = 36\)
- Apply the Square Root Property: \(z = \pm \sqrt{36}\)
- Simplify: \(z = \pm 6\)
- Check the solutions: Substitute \(z = 6\) and \(z = -6\) back into the original equation.
- Example 5: Solve \(y^2 - 27 = 0\)
- Isolate the quadratic term: \(y^2 = 27\)
- Apply the Square Root Property: \(y = \pm \sqrt{27}\)
- Simplify: \(y = \pm 3\sqrt{3}\)
- Check the solutions: Substitute \(y = 3\sqrt{3}\) and \(y = -3\sqrt{3}\) back into the original equation.
Introduction to Solving Equations Using Square Roots
Solving equations using square roots is a fundamental technique in algebra that allows us to find the roots of equations involving squared terms. This method is particularly useful for solving quadratic equations that can be rewritten in the form \( ax^2 + c = 0 \). The process involves isolating the squared term and then taking the square root of both sides of the equation.
Here are the general steps to solve an equation using square roots:
- Isolate the squared term: Move all other terms to the opposite side of the equation to have the squared term alone on one side. For example, if the equation is \( 4x^2 - 9 = 0 \), rewrite it as \( 4x^2 = 9 \).
- Take the square root of both sides: Apply the square root operation to both sides of the equation. Remember to include both the positive and negative roots. For example, \( \sqrt{4x^2} = \sqrt{9} \) gives \( 2x = \pm 3 \).
- Solve for the variable: Isolate the variable by dividing both sides by the coefficient of the variable term. For example, \( 2x = \pm 3 \) becomes \( x = \pm \frac{3}{2} \).
Let's look at a few examples:
| Equation | Steps | Solution |
|---|---|---|
| \( 4x^2 - 9 = 0 \) |
|
\( x = \pm \frac{3}{2} \) |
| \( 3x^2 + 7 = 55 \) |
|
\( x = \pm 4 \) |
| \( -2x^2 + 15 = x^2 - 12 \) |
|
\( x = \pm 3 \) |
Using these steps, you can solve any quadratic equation that can be rewritten in the form \( ax^2 + c = 0 \). Practice these techniques with various equations to become more proficient in solving equations using square roots.
Understanding the Square Root Property
The Square Root Property is a powerful tool for solving quadratic equations. It states that if \(x^2 = k\), then \(x = \pm \sqrt{k}\). This property allows us to find solutions to equations by isolating the squared term and taking the square root of both sides. Here's a detailed step-by-step process:
- Isolate the quadratic term and make its coefficient one. For example, in the equation \(x^2 - 50 = 0\), we add 50 to both sides to get \(x^2 = 50\).
- Use the Square Root Property: \(x = \pm \sqrt{50}\).
- Simplify the radical if possible. Since \( \sqrt{50} = \sqrt{25 \cdot 2} = 5\sqrt{2}\), the solution is \(x = \pm 5\sqrt{2}\).
- Check the solutions by substituting back into the original equation to ensure they satisfy it.
Let's see another example:
- Consider the equation \( (x - 5)^2 - 100 = 0 \).
- Isolate the squared term: \( (x - 5)^2 = 100 \).
- Apply the Square Root Property: \( x - 5 = \pm \sqrt{100} \).
- Simplify: \( x - 5 = \pm 10 \).
- Solve for \( x \): \( x = 5 \pm 10 \), giving \( x = 15 \) or \( x = -5 \).
By following these steps, you can effectively solve quadratic equations using the Square Root Property.

Step-by-Step Process of Solving Quadratic Equations
Solving quadratic equations using the square root method involves a systematic approach that can simplify the process significantly. Follow these steps to solve quadratic equations efficiently:
- Isolate the quadratic term:
Ensure the quadratic term is on one side of the equation and all other terms are on the opposite side. For example, in the equation \(x^2 - 50 = 0\), add 50 to both sides to isolate the quadratic term: \(x^2 = 50\).
- Make the coefficient of the quadratic term equal to one:
If the coefficient is not one, divide the entire equation by the coefficient. For example, if you have \(4x^2 = 100\), divide by 4 to get \(x^2 = 25\).
- Apply the square root property:
Take the square root of both sides of the equation, remembering to include the ± symbol. For instance, \(\sqrt{x^2} = \pm\sqrt{50}\) simplifies to \(x = \pm\sqrt{50}\).
- Simplify the radical:
If possible, simplify the square root. For example, \(\sqrt{50} = \sqrt{25 \cdot 2} = 5\sqrt{2}\), thus \(x = \pm 5\sqrt{2}\).
- Check the solutions:
Substitute the solutions back into the original equation to verify they are correct. For \(x = 5\sqrt{2}\) and \(x = -5\sqrt{2}\), check if they satisfy \(x^2 - 50 = 0\).
Using these steps, you can solve any quadratic equation that is suitable for the square root method. This method is particularly useful for equations that do not factor easily.
Solving Perfect Square Equations
Perfect square equations are quadratic equations where one side is a perfect square trinomial. These equations can be solved efficiently using the square root property. Below is a step-by-step guide to solving perfect square equations:
-
Identify the Perfect Square: Rewrite the equation in the form \( (ax + b)^2 = c \). For example, the equation \( x^2 + 6x + 9 = 25 \) can be rewritten as \( (x + 3)^2 = 25 \).
-
Apply the Square Root Property: Take the square root of both sides of the equation. Remember to include both the positive and negative solutions. Using the example above, \( (x + 3)^2 = 25 \) becomes \( x + 3 = \pm \sqrt{25} \).
-
Simplify the Roots: Calculate the square root of the constant on the right side. In this case, \( \sqrt{25} = 5 \), so the equation becomes \( x + 3 = \pm 5 \).
-
Solve for the Variable: Separate the equation into two cases to solve for the variable \( x \):
- Case 1: \( x + 3 = 5 \)
- Case 2: \( x + 3 = -5 \)
Solving these gives:
- Case 1: \( x = 5 - 3 = 2 \)
- Case 2: \( x = -5 - 3 = -8 \)
-
Verify the Solutions: Substitute the solutions back into the original equation to ensure they are correct.
Thus, the solutions to the perfect square equation \( x^2 + 6x + 9 = 25 \) are \( x = 2 \) and \( x = -8 \).
Solving Non-Perfect Square Equations
Non-perfect square equations can be more challenging, but with a systematic approach, they can be solved effectively. The steps are as follows:
- First, isolate the term with the square. For example, consider the equation \(3x^2 + 7 = 55\). Subtract 7 from both sides to get \(3x^2 = 48\).
- Next, divide by the coefficient of \(x^2\). For the example above, divide both sides by 3 to obtain \(x^2 = 16\).
- Now, take the square root of both sides. Remember to include both the positive and negative roots. Thus, \(x = \pm \sqrt{16}\) which simplifies to \(x = \pm 4\).
- If the equation involves a binomial squared, for instance, \((x - 5)^2 = 100\), take the square root of both sides to get \(x - 5 = \pm 10\). Solve for \(x\) to get \(x = 15\) or \(x = -5\).
For more complex equations involving multiple terms or constants, combine like terms and apply the square root method iteratively. Consistency in following these steps will lead to correct solutions.
Examples and Practice Problems
Understanding how to solve equations using square roots is essential for mastering various types of quadratic equations. Below are detailed examples and practice problems to help you grasp the concept step-by-step.
Example 1: Solving a Simple Quadratic Equation
Solve the equation \(x^2 = 25\).
- Isolate the quadratic term: \(x^2 = 25\).
- Apply the square root to both sides: \(x = \pm \sqrt{25}\).
- Simplify the square root: \(x = \pm 5\).
Thus, the solutions are \(x = 5\) and \(x = -5\).
Example 2: Solving a Quadratic Equation with a Non-Perfect Square
Solve the equation \(x^2 = 20\).
- Isolate the quadratic term: \(x^2 = 20\).
- Apply the square root to both sides: \(x = \pm \sqrt{20}\).
- Simplify the square root: \(x = \pm \sqrt{4 \times 5} = \pm 2\sqrt{5}\).
Thus, the solutions are \(x = 2\sqrt{5}\) and \(x = -2\sqrt{5}\).
Example 3: Solving a Quadratic Equation with Binomials
Solve the equation \((x - 3)^2 = 9\).
- Isolate the quadratic term: \((x - 3)^2 = 9\).
- Apply the square root to both sides: \(x - 3 = \pm \sqrt{9}\).
- Simplify the square root: \(x - 3 = \pm 3\).
- Solve for \(x\):
- When \(x - 3 = 3\), \(x = 6\).
- When \(x - 3 = -3\), \(x = 0\).
Thus, the solutions are \(x = 6\) and \(x = 0\).
Practice Problems
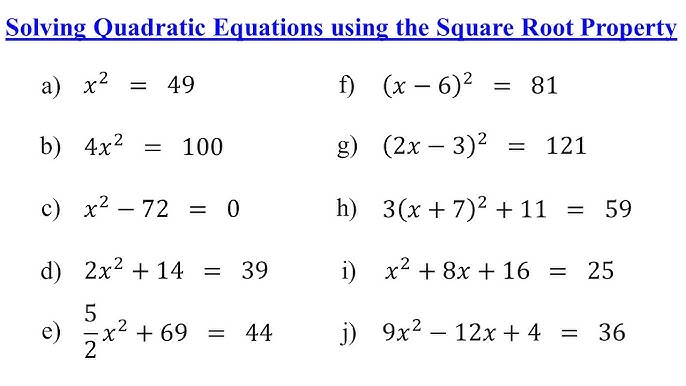
- Solve \(x^2 = 49\).
- Solve \(x^2 = 8\).
- Solve \((x + 4)^2 = 16\).
- Solve \(x^2 - 4 = 0\).
Attempt these practice problems to reinforce your understanding of solving equations using square roots.
Special Cases in Solving Square Root Equations
When solving square root equations, there are some special cases that require careful consideration to ensure accurate solutions. Here are a few of these special cases:
- Extraneous Solutions: These are solutions that arise from the process of solving the equation but do not satisfy the original equation. This typically occurs when both sides of an equation are squared, introducing potential false solutions. To handle extraneous solutions, always substitute back into the original equation to verify each solution.
- Negative Radicands: If the radicand (the number under the square root) is negative, the equation has no real solutions because the square root of a negative number is not a real number. This situation can be identified early in the problem-solving process.
- Perfect Square Equations: When solving an equation where both sides are perfect squares, such as \( (x+3)^2 = 16 \), the solutions can be found by taking the square root of both sides. For instance:
\[
(x+3)^2 = 16
\]
Taking the square root of both sides:
\[
x + 3 = \pm 4
\]
Thus, the solutions are:
\[
x = 4 - 3 = 1 \quad \text{or} \quad x = -4 - 3 = -7
\] - Non-Perfect Square Equations: For equations where the radicand is not a perfect square, the solution will involve irrational numbers. For example, solving \( x^2 = 7 \) gives:
\[
x = \pm \sqrt{7}
\]
Since \( \sqrt{7} \) cannot be simplified further, the solutions are left in this form. - Quadratic Equations with Zero: When solving equations like \( x^2 = 0 \), the only solution is \( x = 0 \) because the square root of zero is zero.
Let's consider an example to illustrate these special cases:
Example: Solve \( (x-2)^2 = 9 \)
- Take the square root of both sides:
\[
\sqrt{(x-2)^2} = \sqrt{9}
\]
Which simplifies to:
\[
x - 2 = \pm 3
\] - Solve for \( x \):
\[
x - 2 = 3 \quad \Rightarrow \quad x = 5
\]
\[
x - 2 = -3 \quad \Rightarrow \quad x = -1
\] - Check for extraneous solutions by substituting back into the original equation:
For \( x = 5 \):
\[
(5-2)^2 = 9 \quad \Rightarrow \quad 3^2 = 9 \quad \Rightarrow \quad 9 = 9 \quad \text{(Valid solution)}
\]
For \( x = -1 \):
\[
(-1-2)^2 = 9 \quad \Rightarrow \quad (-3)^2 = 9 \quad \Rightarrow \quad 9 = 9 \quad \text{(Valid solution)}
\]
By following these steps and considering special cases, you can solve square root equations accurately and efficiently.
Checking Your Solutions
After solving an equation using square roots, it is crucial to verify that your solutions are correct. Here are the steps to check your solutions:
-
Substitute the solutions back into the original equation:
Take each solution and plug it back into the original equation to ensure both sides of the equation are equal.
-
Consider extraneous solutions:
Extraneous solutions are false solutions that may arise when both sides of an equation are squared during the solving process. Therefore, it's important to check if each solution truly satisfies the original equation.
Example
Solve and check the equation \( \sqrt{x+1} = 4 \):
-
Solve the equation:
\[
\sqrt{x+1} = 4 \implies x + 1 = 16 \implies x = 15
\] -
Check the solution:
Substitute \( x = 15 \) back into the original equation:
\[
\sqrt{15+1} = 4 \implies \sqrt{16} = 4 \implies 4 = 4
\]Since both sides are equal, \( x = 15 \) is a valid solution.
Example with Quadratic Equation
Solve and check the equation \( \sqrt{3x+1} = x - 3 \):
-
Solve the equation:
\[
\sqrt{3x+1} = x - 3 \implies ( \sqrt{3x+1} )^2 = (x - 3)^2 \implies 3x + 1 = x^2 - 6x + 9
\]\[
x^2 - 9x + 8 = 0 \implies (x - 8)(x - 1) = 0 \implies x = 8 \text{ or } x = 1
\] -
Check the solutions:
For \( x = 8 \):
\[
\sqrt{3(8)+1} = 8 - 3 \implies \sqrt{24+1} = 5 \implies \sqrt{25} = 5 \implies 5 = 5
\]Since both sides are equal, \( x = 8 \) is a valid solution.
For \( x = 1 \):
\[
\sqrt{3(1)+1} = 1 - 3 \implies \sqrt{3+1} = -2 \implies \sqrt{4} = -2 \implies 2 \neq -2
\]Since both sides are not equal, \( x = 1 \) is not a valid solution.
By following these steps, you can ensure that your solutions are accurate and valid, helping you to avoid common pitfalls in solving equations using square roots.
Common Mistakes to Avoid
When solving equations using square roots, it is essential to be aware of common mistakes that can lead to incorrect solutions. Here are some of the most frequent errors and how to avoid them:
-
Forgetting the Negative Solution:
When taking the square root of both sides of an equation, remember that there are both positive and negative solutions. For example, if \(x^2 = 9\), then \(x = 3\) and \(x = -3\). Always check for both solutions.
-
Misinterpreting the Square Root Symbol:
The square root symbol (√) typically represents the principal (positive) square root. Ensure you account for the negative root separately when solving equations.
-
Incorrectly Simplifying Radicals:
Ensure that you simplify square roots correctly. For instance, \(\sqrt{18}\) should be simplified to \(3\sqrt{2}\) by breaking it down into \(\sqrt{9 \cdot 2} = \sqrt{9} \cdot \sqrt{2} = 3\sqrt{2}\).
-
Distributing Square Roots Improperly:
Square roots do not distribute over addition or subtraction. For example, \(\sqrt{a+b} \ne \sqrt{a} + \sqrt{b}\). This only works for multiplication and division, such as \(\sqrt{ab} = \sqrt{a} \cdot \sqrt{b}\).
-
Errors in Exponents:
Be careful with exponent rules, such as \((x^2)^3 \ne x^5\). Instead, \((x^2)^3 = x^{2 \cdot 3} = x^6\).
-
Fraction Errors:
When working with fractions, remember that \(\frac{a}{b+c} \ne \frac{a}{b} + \frac{a}{c}\). Always handle the entire denominator together.
-
Distributing Negative Signs:
When distributing a negative sign, ensure it applies to all terms inside the parentheses. For example, \(-(a+b) = -a - b\).
-
Parentheses Mistakes:
Be cautious when squaring terms inside parentheses. For example, \((x+a)^2 \ne x^2 + a^2\). Instead, use the formula \((x+a)^2 = x^2 + 2xa + a^2\).
By keeping these common mistakes in mind and practicing regularly, you can improve your accuracy in solving square root equations.
Advanced Techniques and Tips
Solving equations using square roots can be made more efficient and accurate by employing advanced techniques and tips. Below are some useful strategies to enhance your problem-solving skills:
- Completing the Square
Completing the square transforms a quadratic equation into a perfect square trinomial, making it easier to solve by taking the square root of both sides. Here's the step-by-step process:
- Start with the quadratic equation in the form \(ax^2 + bx + c = 0\).
- Divide all terms by \(a\) (if \(a \neq 1\)) to get \(x^2 + \frac{b}{a}x + \frac{c}{a} = 0\).
- Move the constant term to the other side of the equation: \(x^2 + \frac{b}{a}x = -\frac{c}{a}\).
- Add the square of half the coefficient of \(x\) to both sides: \(x^2 + \frac{b}{a}x + \left(\frac{b}{2a}\right)^2 = \left(\frac{b}{2a}\right)^2 - \frac{c}{a}\).
- Rewrite the left side as a square: \(\left(x + \frac{b}{2a}\right)^2 = \left(\frac{b}{2a}\right)^2 - \frac{c}{a}\).
- Solve for \(x\) by taking the square root of both sides and isolating \(x\).
- Using the Discriminant
The discriminant (\(\Delta = b^2 - 4ac\)) of a quadratic equation \(ax^2 + bx + c = 0\) can help determine the nature of the solutions before solving:
- If \(\Delta > 0\), there are two distinct real solutions.
- If \(\Delta = 0\), there is one real solution (a repeated root).
- If \(\Delta < 0\), there are no real solutions (the solutions are complex).
- Utilizing Technology
Graphing calculators and computer algebra systems (CAS) can be extremely helpful for visualizing the solutions and verifying your work. These tools can provide numerical solutions, handle complex algebraic manipulations, and graph the functions to show intersections.
- Linear Approximation for Square Roots
For a quick estimate of square roots, you can use linear approximation. If \(y\) is a number close to \(x\), then \(\sqrt{x} \approx \frac{x + y}{2\sqrt{y}}\). This method works best when \(y\) is a perfect square close to \(x\).
- Handling Complex Numbers
When the discriminant is negative, the solutions to the quadratic equation will be complex. In such cases, use the imaginary unit \(i\) where \(i^2 = -1\) to express the solutions. For example, the solutions will be in the form \(x = a \pm bi\).
Applications of Square Root Solving in Real Life
Square roots have numerous applications in various fields, demonstrating their practical significance. Here are some key areas where square roots are applied:
- Engineering: Engineers often use square roots in calculations involving right-angled triangles, such as determining the length of diagonals and supports. The Pythagorean theorem, which includes square roots, is fundamental in these calculations.
- Finance: In finance, square roots are used to calculate the rate of return over multiple periods. For example, to find the rate of return on an asset over two years, the square root of the return over the first year is used.
- Physics: Square roots are essential in physics for calculations involving distances and velocities. For instance, the distance between two points in a plane or space is found using the distance formula, which incorporates square roots.
- Probability and Statistics: In probability theory, square roots are used in the calculation of standard deviation and variance, which are measures of data dispersion. The normal distribution curve, a key concept in statistics, also relies on square roots.
- Architecture and Construction: Architects and builders use square roots to determine various measurements, such as the slope of roofs and the dimensions of different structures. Accurate calculations ensure the stability and aesthetic appeal of buildings.
- Computer Graphics: In computer graphics, square roots are used to calculate distances between points, which is essential for rendering images and animations. This includes applications in video game development and 3D modeling.
- Astronomy: Astronomers use square roots to calculate distances between celestial objects. The inverse square law, which describes how light and gravity diminish with distance, involves square roots.
Below are some specific examples demonstrating these applications:
- Finding the Diagonal of a Square:
Given a square with side length \( a \), the diagonal \( d \) can be found using the formula:
\[ d = \sqrt{2}a \]
This is derived from the Pythagorean theorem applied to the right-angled triangle formed by the sides and the diagonal of the square.
- Distance Between Two Points:
In a 2-dimensional plane, the distance \( D \) between two points \((x_1, y_1)\) and \((x_2, y_2)\) is given by:
\[ D = \sqrt{(x_2 - x_1)^2 + (y_2 - y_1)^2} \]
In a 3-dimensional space, the formula extends to include the \( z \)-coordinates:
\[ D = \sqrt{(x_2 - x_1)^2 + (y_2 - y_1)^2 + (z_2 - z_1)^2} \]
- Quadratic Equations:
Square roots are used to solve quadratic equations using the quadratic formula:
\[ x = \frac{-b \pm \sqrt{b^2 - 4ac}}{2a} \]
This formula provides solutions for any quadratic equation of the form \( ax^2 + bx + c = 0 \).
- Kinetic Energy:
In physics, the kinetic energy \( KE \) of an object can be found using the square root when given the mass \( m \) and velocity \( v \):
\[ KE = \frac{1}{2}mv^2 \]
To solve for velocity given the kinetic energy and mass, square roots are necessary.
These examples illustrate the diverse and essential roles that square roots play in real-world applications, highlighting their importance beyond basic mathematical exercises.
Additional Resources and References
For further exploration and deeper understanding of solving equations using square roots, consider the following resources:
- : Comprehensive lessons and practice problems.
- : Step-by-step tutorials and explanations.
- : Simplified explanations and interactive examples.
- : Free resources including textbooks and videos.
These resources will help solidify your understanding and provide additional practice opportunities to master solving equations using square roots.
Video hướng dẫn sử dụng phương pháp căn bậc hai để giải phương trình bậc hai, giúp người xem dễ dàng nắm bắt kiến thức cơ bản.
Sử dụng phương pháp căn bậc hai để giải phương trình bậc hai với một biến
READ MORE:
Video hướng dẫn cách giải phương trình bậc hai bằng cách sử dụng tính chất căn bậc hai, cung cấp kiến thức chi tiết cho người học.
Cách Giải Phương Trình Bậc Hai Sử Dụng Tính Chất Căn Bậc Hai | Đại Số