Simplify Square Root of 70: A Step-by-Step Guide

When simplifying the square root of a number, we look for perfect square factors. In this case, we will attempt to simplify $$

70

. However, 70 is not a perfect square, and it does not have any perfect square factors other than 1.

Prime Factorization

First, we perform the prime factorization of 70:

• 70 ÷ 2 = 35
• 35 ÷ 5 = 7
• 7 ÷ 7 = 1

So, the prime factorization of 70 is:

$$
70
=
2
×
5
×
7

Checking for Perfect Squares

Since none of the factors 2, 5, or 7 are perfect squares, we conclude that the square root of 70 cannot be simplified further. Thus:

$$

70

≈
8.37

The square root of 70 remains $$

70

or approximately 8.37 when expressed in decimal form.

Square roots are a fundamental concept in mathematics, used to find a value that, when multiplied by itself, gives the original number. Simplifying square roots involves expressing the root in its simplest radical form. This process makes it easier to work with roots in various mathematical problems. In this section, we will cover the basic steps and principles of simplifying square roots, using examples to illustrate each step clearly.

To simplify a square root:

1. Identify the factors of the number under the square root.
2. Look for perfect square factors.
3. Rewrite the square root as a product of the square root of the perfect square and the square root of the remaining factor.
4. Simplify the expression by taking the square root of the perfect square.

For example, consider the square root of 70:

• Factors of 70: 1, 2, 5, 7, 10, 14, 35, 70
• The perfect square among these factors is 1.
• Since 1 is the only perfect square, the square root of 70 cannot be simplified further in terms of perfect squares.
• Therefore, √70 remains as it is.

Although we cannot simplify √70 in radical form, we can express it in decimal and exponent forms:

• Decimal form: √70 ≈ 8.3666
• Exponent form: 70^1/2

Understanding how to simplify square roots is essential for solving more complex algebraic expressions and equations. By mastering these basic steps, you can tackle a variety of mathematical problems with confidence.

Square roots and perfect squares are fundamental concepts in mathematics that help simplify expressions and solve equations. A square root of a number is a value that, when multiplied by itself, gives the original number. The symbol for the square root is √. For example, √9 equals 3 because 3 x 3 = 9.

A perfect square is a number that has an integer as its square root. Examples of perfect squares include 1, 4, 9, 16, 25, etc. Identifying perfect squares is crucial when simplifying square roots.

To simplify a square root, you need to factorize the number inside the radical into its prime factors and then pair the prime factors. Here are the steps:

• Step 1: Find the prime factors of the number.
• Step 2: Pair the prime factors.
• Step 3: Move each pair of prime factors outside the radical.
• Step 4: Multiply the numbers outside the radical and leave any unpaired factors inside.

Let's look at an example:

Simplify √72:

1. Prime factorization of 72: 2 × 2 × 2 × 3 × 3.
2. Pair the factors: (2 × 2) and (3 × 3).
3. Move pairs outside the radical: 2 × 3.
4. Result: 6√2.

However, not all numbers can be simplified in this way. For example, √70 is already in its simplest form because 70 = 2 × 5 × 7 and there are no pairs of factors. Therefore, √70 cannot be simplified further.

Understanding these basics can make it easier to work with square roots and identify when a number is a perfect square or if it can be simplified.

To simplify the square root of 70 using the prime factorization method, follow these detailed steps:

1. Start by finding the prime factors of 70. The prime factorization of 70 is:

   \[
   70 = 2 \times 5 \times 7
   \]

2. Express the square root of 70 in terms of its prime factors:

   \[
   \sqrt{70} = \sqrt{2 \times 5 \times 7}
   \]

3. Since none of the prime factors are repeated, we cannot simplify the square root further by pairing any factors. Therefore, the square root of 70 is already in its simplest form:

   \[
   \sqrt{70} = \sqrt{2 \times 5 \times 7}
   \]

4. Although we cannot simplify the radical form, we can represent the square root of 70 in decimal form:

   \[
   \sqrt{70} \approx 8.3666
   \]

Therefore, using the prime factorization method, we find that \(\sqrt{70}\) is already in its simplest radical form.

The process of simplifying the square root of 70 involves determining if it can be broken down into simpler radical terms. Follow these steps to understand the simplification:

1. List the factors of 70: Identify all the factors of 70. The factors are:

   • 1
   • 2
   • 5
   • 7
   • 10
   • 14
   • 35
   • 70

2. Identify the perfect squares: From the list of factors, identify any perfect squares. The perfect squares are numbers like 1, 4, 9, 16, etc. In this case, the only perfect square factor is 1.

3. Prime Factorization: Write 70 as a product of its prime factors:

   \[70 = 2 \times 5 \times 7\]

   Since none of these factors are repeated (to form a perfect square), the square root of 70 cannot be simplified further.

4. Conclusion: Because there are no pairs of prime factors, the square root of 70 remains in its simplest form as:

   \[\sqrt{70}\]

5. Decimal Approximation: While the radical form is \(\sqrt{70}\), its decimal approximation can be useful in practical applications. Using a calculator, we find:

   \[\sqrt{70} \approx 8.3666\]

Thus, the square root of 70 is already in its simplest form, but its decimal approximation is approximately 8.3666.

To simplify a square root, it's crucial to check for perfect square factors within the number. Perfect squares are numbers that can be expressed as the square of an integer, such as 1, 4, 9, 16, 25, and so on. Here’s how you can check for perfect square factors in the number 70:

1. List the factors of 70: Start by listing all the factors of 70. The factors are:

   • 1
   • 2
   • 5
   • 7
   • 10
   • 14
   • 35
   • 70

2. Identify perfect squares: From the list of factors, identify which are perfect squares. Perfect squares from the list include only:

   • 1

   Since 1 is the only perfect square factor of 70, it indicates that there are no larger perfect square factors that can be used to simplify \(\sqrt{70}\).

3. Prime factorization: Another method is to use prime factorization. Write 70 as a product of its prime factors:

   \[70 = 2 \times 5 \times 7\]

   None of these prime factors are perfect squares or can be grouped into pairs to form perfect squares. Therefore, no further simplification can be made using perfect square factors.

4. Conclusion: Since 70 has no perfect square factors other than 1, the square root of 70 cannot be simplified further and remains as:

   \[\sqrt{70}\]

Understanding perfect square factors is essential in simplifying square roots, but in the case of \(\sqrt{70}\), it remains in its simplest form because there are no significant perfect square factors other than 1.

When simplifying square roots, there are several common mistakes that can lead to incorrect results. Here are some key errors to watch out for when simplifying \(\sqrt{70}\) and how to avoid them:

1. Forgetting to Check for Perfect Squares: Ensure you identify all perfect square factors. In the case of 70, the perfect squares are limited to 1. Missing this step can lead to incorrect simplification attempts.

2. Incorrect Prime Factorization: Always perform prime factorization accurately. For 70, the correct prime factors are:

   \[70 = 2 \times 5 \times 7\]

   Mistakes in this step can lead to erroneous simplification.

3. Mishandling Radicals: Be cautious when handling multiplication or division of radicals. Remember the rules:

   \[\sqrt{a \times b} = \sqrt{a} \times \sqrt{b}\]

   Incorrect application of these rules can change the value of the expression.

4. Overcomplicating Simplification: Not all square roots can be simplified beyond their original form. For instance, \(\sqrt{70}\) does not simplify further since its prime factors do not form pairs.

5. Decimal Approximation Errors: When converting to a decimal, ensure precision. For \(\sqrt{70}\), the approximate value is:

   \[\sqrt{70} \approx 8.3666\]

   Rounding errors can lead to inaccuracies in practical applications.

Avoiding these common mistakes will help ensure accurate and reliable results when simplifying square roots.

To find the decimal approximation of \( \sqrt{70} \), we can use various methods such as estimation and long division. Here, we will go through a detailed step-by-step guide for both methods.

Estimation Method

1. Identify two consecutive perfect squares between which 70 lies. In this case, it is 64 (which is \( 8^2 \)) and 81 (which is \( 9^2 \)).
2. Take the whole number part as the smaller perfect square, which is 8.
3. For the decimal part, use the formula: \[ \text{Decimal part} = \frac{70 - 64}{81 - 64} = \frac{6}{17} \approx 0.35 \]
4. Combine these to get the estimated square root of 70: \[ \sqrt{70} \approx 8 + 0.35 = 8.35 \]

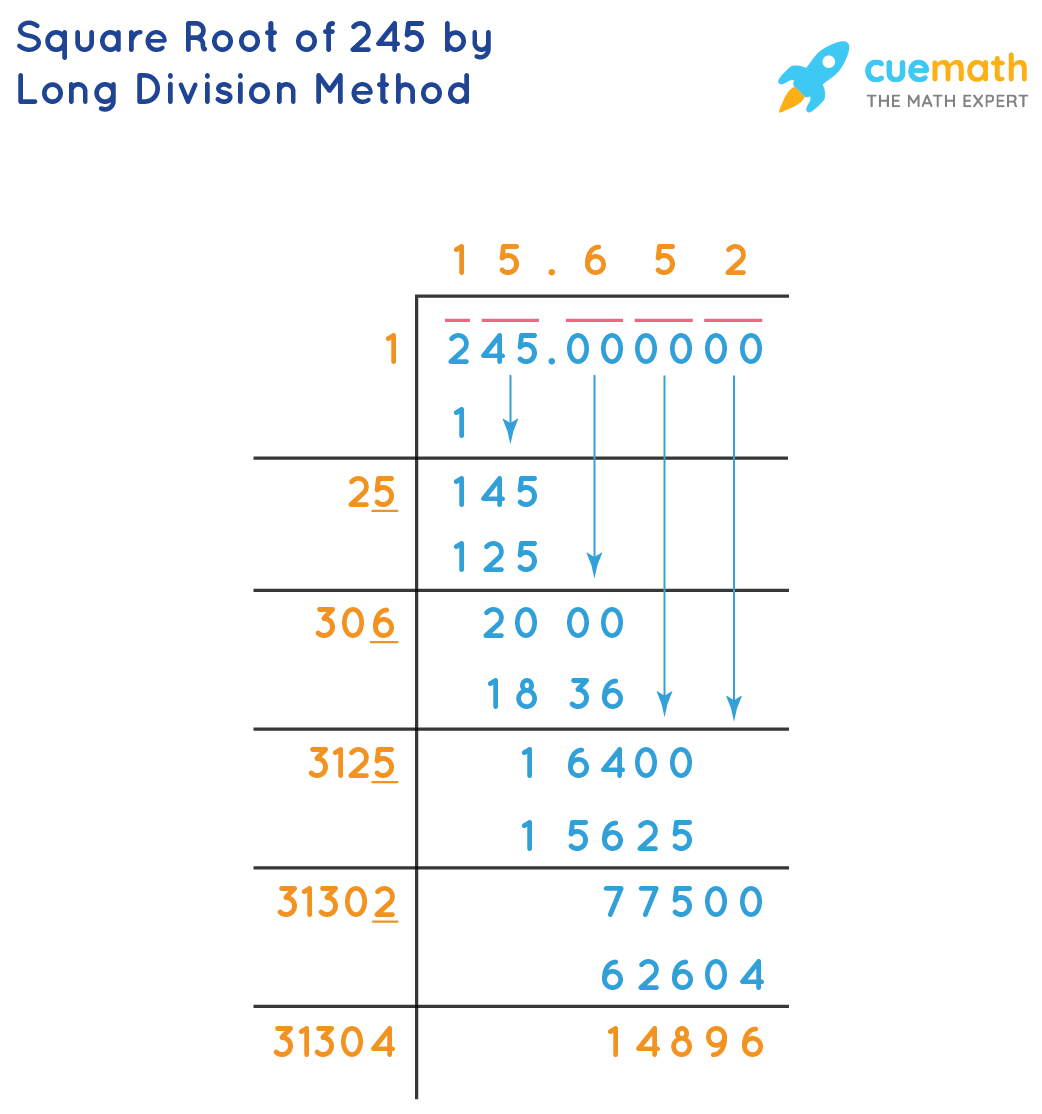
Long Division Method

For a more precise approximation, the long division method is effective. Here’s how you can apply it:

1. Set up 70 as the number to be square-rooted. Begin by grouping the digits in pairs from the decimal point.
2. Find the largest number whose square is less than or equal to the first group. Here, the largest number is 8 (since \( 8^2 = 64 \leq 70 \)). Write 8 above the radical sign.
3. Subtract the square of this number (64) from 70, giving a remainder of 6. Bring down a pair of zeros to make the remainder 600.
4. Double the number above the radical sign (8), getting 16. Determine the largest digit \( x \) such that \( 16x \times x \leq 600 \). The largest digit here is 3 (since \( 163 \times 3 = 489 \leq 600 \)). Write 3 next to 8 above the radical sign.
5. Subtract 489 from 600, getting a new remainder of 111. Bring down another pair of zeros to make it 11100. Continue this process to get a more precise value.
6. The long division process will yield \( \sqrt{70} \approx 8.3666 \) as you continue refining the digits.

Conclusion

Both methods provide a way to approximate \( \sqrt{70} \). The estimation method gives a quick but rough approximation, while the long division method offers a more accurate result. The value of \( \sqrt{70} \) is approximately 8.3666.

Simplifying square roots is a fundamental mathematical skill that has numerous practical applications in various fields. Understanding how to simplify square roots can be beneficial in the following areas:

• Mathematics and Education:

  In higher-level mathematics, simplifying square roots is essential for solving algebraic equations, calculus problems, and geometry tasks. It is a foundational skill taught in schools to help students understand more complex mathematical concepts.

• Science and Engineering:

  Engineers and scientists often encounter square roots in their work, particularly in physics, chemistry, and biology. Simplifying square roots can make it easier to work with formulas and equations, leading to more accurate and efficient calculations.

• Computer Science:

  In computer graphics, algorithms involving square roots are used for tasks such as calculating distances, angles, and optimizing rendering processes. Simplifying these calculations can improve computational efficiency.

• Finance and Economics:

  In finance, square roots are used in various models, including risk assessment and pricing options. Simplifying these expressions can help in understanding and communicating financial data more clearly.

• Everyday Life:

  Simplifying square roots can also be useful in everyday situations, such as when measuring dimensions, constructing objects, or understanding proportions. It helps in making quick mental calculations and estimations.

Overall, mastering the simplification of square roots enhances problem-solving abilities and analytical skills, making it a valuable tool in both academic and professional settings.

Simplifying the square root of 70 is a straightforward yet insightful mathematical process. While √70 cannot be simplified into a smaller radical form due to the lack of perfect square factors, understanding its properties and approximations broadens our comprehension of square roots.

In this article, we explored several key aspects:

• The definition and fundamental properties of square roots.
• How to check for perfect square factors to simplify square roots.
• Common mistakes to avoid in the simplification process.
• The decimal approximation of √70 for practical applications.
• Various real-life applications of simplifying square roots.

Mastering the simplification of square roots, including those that cannot be reduced further, equips us with valuable skills for tackling more complex mathematical problems and practical scenarios in fields such as engineering, physics, and computer science. Keep practicing and exploring different methods, and you will continue to enhance your mathematical proficiency.