Topic simplify square root of negative 100: Discover how to simplify the square root of negative 100 using the imaginary unit \(i\). This guide will walk you through the steps, common mistakes, and practical applications of imaginary numbers, ensuring you understand and master this concept. Perfect for students and math enthusiasts looking to deepen their knowledge of complex numbers.
Table of Content
- Simplifying the Square Root of Negative 100
- Introduction to Imaginary Numbers
- Definition and Properties of the Imaginary Unit \(i\)
- Steps to Simplify the Square Root of Negative Numbers
- Detailed Simplification Process for \( \sqrt{-100} \)
- Common Mistakes and How to Avoid Them
- Practical Applications of Imaginary Numbers
- Complex Numbers: Combining Real and Imaginary Parts
- Examples and Practice Problems
- Further Reading and Resources
- YOUTUBE:
Simplifying the Square Root of Negative 100
When dealing with the square root of a negative number, we need to use the concept of imaginary numbers. The imaginary unit \( i \) is defined as \( \sqrt{-1} \). Using this definition, we can simplify the square root of -100 as follows:
Steps to Simplify \( \sqrt{-100} \)
- Start with the expression \( \sqrt{-100} \).
- Rewrite \( -100 \) as \( -1 \times 100 \).
- Use the property of square roots: \( \sqrt{a \times b} = \sqrt{a} \times \sqrt{b} \). Thus, \( \sqrt{-100} = \sqrt{-1 \times 100} \).
- Separate the square roots: \( \sqrt{-1 \times 100} = \sqrt{-1} \times \sqrt{100} \).
- Recognize that \( \sqrt{-1} = i \) and \( \sqrt{100} = 10 \).
- Combine the results: \( i \times 10 \) or \( 10i \).
Therefore, the simplified form of \( \sqrt{-100} \) is:
\( \sqrt{-100} = 10i \)
This result falls within the realm of complex numbers, where \( i \) is the imaginary unit representing \( \sqrt{-1} \).
Understanding Imaginary Numbers
Imaginary numbers are essential in various fields of mathematics and engineering. They provide solutions to equations that do not have real number solutions. For instance, the equation \( x^2 + 100 = 0 \) does not have any real solutions because you cannot square a real number to get a negative result. However, using imaginary numbers, we can find solutions in the form of complex numbers.
To learn more about imaginary numbers and their applications, consider exploring further resources on algebra and complex numbers.

READ MORE:
Introduction to Imaginary Numbers
Imaginary numbers are a fundamental concept in mathematics, often used to extend the real number system to solve equations that do not have real solutions. The core of imaginary numbers is the imaginary unit \(i\), which is defined as \(i = \sqrt{-1}\). This definition allows us to simplify the square roots of negative numbers.
For example, to simplify the square root of -100, we start by recognizing that \(\sqrt{-100} = \sqrt{100 \cdot -1}\). This can be further broken down using the property of square roots:
- \(\sqrt{100 \cdot -1} = \sqrt{100} \cdot \sqrt{-1}\)
- \(\sqrt{100} = 10\)
- \(\sqrt{-1} = i\)
Combining these results, we find that \(\sqrt{-100} = 10i\). This approach can be applied to any negative number, allowing us to work with square roots that would otherwise be undefined within the realm of real numbers.
The introduction of imaginary numbers leads to the broader concept of complex numbers, which are numbers of the form \(a + bi\), where \(a\) and \(b\) are real numbers. These complex numbers have wide applications in engineering, physics, and applied mathematics, providing a comprehensive framework for solving problems that involve square roots of negative numbers.
Definition and Properties of the Imaginary Unit \(i\)
The imaginary unit, denoted as \(i\), is defined as the square root of -1. It is a fundamental component of complex numbers, allowing us to extend the real number system to solve equations that have no real solutions. Here are some key properties and uses of the imaginary unit \(i\):
- Basic Definition: \(i = \sqrt{-1}\). This definition allows us to represent the square roots of negative numbers.
- Multiplication: When multiplying \(i\) by itself, we get \(i^2 = -1\). This property is crucial in simplifying expressions involving square roots of negative numbers.
- Higher Powers: The powers of \(i\) cycle every four steps:
- \(i^1 = i\)
- \(i^2 = -1\)
- \(i^3 = -i\)
- \(i^4 = 1\)
- The cycle repeats: \(i^5 = i\), \(i^6 = -1\), and so on.
- Complex Numbers: A complex number is a combination of a real number and an imaginary number, written in the form \(a + bi\), where \(a\) and \(b\) are real numbers. For example, \(3 + 4i\) is a complex number.
- Arithmetic with Imaginary Numbers: Operations such as addition, subtraction, multiplication, and division can be performed with imaginary numbers using similar rules as real numbers, with the addition of the property \(i^2 = -1\).
Understanding the imaginary unit \(i\) is essential for working with complex numbers and simplifying expressions involving square roots of negative numbers.
Steps to Simplify the Square Root of Negative Numbers
To simplify the square root of a negative number, we introduce the imaginary unit \(i\), where \(i = \sqrt{-1}\). Follow these steps to simplify such expressions:
- Identify the Negative Sign: Recognize that the number under the square root is negative. For example, in \(\sqrt{-100}\), the negative sign indicates the need for \(i\).
- Rewrite the Expression: Express the square root of the negative number in terms of \(i\). This means rewriting \(\sqrt{-100}\) as \(\sqrt{100} \cdot \sqrt{-1}\).
- Separate the Components: Break down the expression using the properties of square roots: \(\sqrt{100} \cdot \sqrt{-1} = \sqrt{100} \cdot i\).
- Simplify the Square Root: Find the square root of the positive number. For \(\sqrt{100}\), this simplifies to 10.
- Combine Results: Multiply the simplified square root by \(i\). Thus, \(\sqrt{-100} = 10i\).
This process can be applied to any negative number under the square root, simplifying the expression by introducing the imaginary unit \(i\).
Detailed Simplification Process for \( \sqrt{-100} \)
To simplify the square root of a negative number such as \( \sqrt{-100} \), follow these detailed steps:
- Identify the Negative Sign: Recognize that the number under the square root is negative. Here, it is \(-100\).
- Express the Square Root in Terms of \(i\): Recall that \(i\) is the imaginary unit, defined as \( \sqrt{-1} \). Therefore, rewrite the expression as \( \sqrt{100} \cdot \sqrt{-1} \).
- Simplify the Positive Square Root: Find the square root of the positive part of the number. For \( \sqrt{100} \), the result is 10.
- Combine with \(i\): Multiply the simplified square root by \(i\), the square root of \(-1\). So, \( \sqrt{-100} = 10 \cdot i \), which simplifies to \(10i\).
Thus, the square root of \(-100\) is \(10i\), utilizing the imaginary unit \(i\) for the negative component.

Common Mistakes and How to Avoid Them
Simplifying the square root of negative numbers can be tricky, but being aware of common mistakes can help you avoid errors. Here are some typical pitfalls and how to steer clear of them:
- Incorrectly Simplifying Negative Radicands: Remember that the square root of a negative number involves the imaginary unit \(i\). For example, \(\sqrt{-100}\) should be simplified as \(\sqrt{100} \cdot \sqrt{-1} = 10i\), not as a real number.
- Forgetting to Use \(i\): It's easy to forget the imaginary unit. Always include \(i\) when dealing with the square root of a negative number. For instance, \(\sqrt{-25}\) should be \(5i\), not \(5\).
- Mixing Up Rules: Be careful not to mix up the rules for real and imaginary numbers. The property \(\sqrt{a \cdot b} = \sqrt{a} \cdot \sqrt{b}\) does not hold if both \(a\) and \(b\) are negative. Always separate the imaginary unit first.
- Incorrect Assumptions: Do not assume that the square root of a negative number can be simplified in the same way as positive numbers without considering the imaginary unit. For example, \(\sqrt{-4}\) is not the same as \(\sqrt{4}\).
By keeping these common mistakes in mind and understanding the proper steps to simplify the square root of negative numbers, you can avoid errors and simplify expressions accurately.
Practical Applications of Imaginary Numbers
Imaginary numbers, despite their name, have a wide range of practical applications in various fields. Here are some notable examples:
Electrical Engineering
In electrical engineering, imaginary numbers are essential for analyzing and designing AC (alternating current) circuits. The concept of impedance, which combines resistance (real part) and reactance (imaginary part), is crucial. The impedance \( Z \) is represented as:
\[
Z = R + jX
\]
where \( R \) is the resistance and \( X \) is the reactance. Engineers use \( j \) instead of \( i \) to avoid confusion with current.
Signal Processing
Imaginary numbers are used in signal processing for Fourier transforms, which decompose signals into their frequency components. This is vital in audio and image processing, telecommunications, and more.
Control Systems
Control systems engineers use imaginary numbers for stability analysis. The poles of the transfer function in the complex plane determine the system's stability. A pole with a positive imaginary part indicates oscillatory behavior.
Quantum Mechanics
In quantum mechanics, the wave function, which describes the state of a particle, is often represented as a complex function. Imaginary numbers help describe the probabilities of different outcomes and the behavior of particles at quantum scales.
Fractals and Chaos Theory
Imaginary numbers are used to generate fractals, such as the Mandelbrot set, which are complex and infinitely detailed structures. These concepts are also applied in chaos theory to model dynamic systems.
Practical Example: AC Circuit Analysis
Consider an AC circuit with a resistor \( R \) and an inductor \( L \). The impedance \( Z \) is given by:
\[
Z = R + j\omega L
\]
where \( \omega \) is the angular frequency. The voltage \( V \) and current \( I \) are related by \( V = IZ \), allowing engineers to analyze and design circuits efficiently.
Visualizing Complex Numbers
Complex numbers, written as \( a + bi \), can be visualized on the complex plane with the real part \( a \) on the x-axis and the imaginary part \( b \) on the y-axis. This visualization aids in understanding the behavior of systems described by complex numbers.
- Real-world applications of imaginary numbers demonstrate their utility in solving practical problems across various domains.
- Imaginary numbers help bridge theoretical concepts and practical implementations in science and engineering.
Complex Numbers: Combining Real and Imaginary Parts
Complex numbers are numbers that have both a real part and an imaginary part, expressed in the form \(a + bi\), where \(a\) and \(b\) are real numbers, and \(i\) is the imaginary unit with the property that \(i^2 = -1\). Here, \(a\) is the real part, and \(b\) is the imaginary part.
Adding and Subtracting Complex Numbers
To add or subtract complex numbers, we simply combine the real parts and the imaginary parts separately:
- Given two complex numbers \( (a + bi) \) and \( (c + di) \), their sum is: \[ (a + bi) + (c + di) = (a + c) + (b + d)i \]
- Their difference is: \[ (a + bi) - (c + di) = (a - c) + (b - d)i \]
Multiplying Complex Numbers
When multiplying two complex numbers, we use the distributive property, similar to multiplying polynomials, and simplify using \( i^2 = -1 \):
- Given \( (a + bi) \) and \( (c + di) \), their product is: \[ (a + bi)(c + di) = ac + adi + bci + bdi^2 \]
- Since \( i^2 = -1 \), the product simplifies to: \[ (ac - bd) + (ad + bc)i \]
Dividing Complex Numbers
Division of complex numbers involves multiplying the numerator and the denominator by the complex conjugate of the denominator to eliminate the imaginary part in the denominator:
- Given \(\dfrac{a + bi}{c + di}\), the complex conjugate of \(c + di\) is \(c - di\).
- Multiply the numerator and the denominator by the complex conjugate: \[ \dfrac{(a + bi)(c - di)}{(c + di)(c - di)} \]
- Simplify using \(i^2 = -1\): \[ \dfrac{(ac + bd) + (bc - ad)i}{c^2 + d^2} \]
Practical Example
Let's consider an example where we combine the real and imaginary parts:
- Given the complex numbers \( (5 + 3i) \) and \( (2 - 4i) \):
- Their sum is: \[ (5 + 3i) + (2 - 4i) = 7 - i \]
- Their product is: \[ (5 + 3i)(2 - 4i) = 10 - 20i + 6i - 12i^2 = 10 - 14i + 12 = 22 - 14i \]
Conclusion
Combining the real and imaginary parts of complex numbers allows for a wide range of operations and applications, especially in fields such as engineering, physics, and applied mathematics.
Examples and Practice Problems
Understanding the process of simplifying the square root of negative numbers is crucial for mastering complex numbers. Below are some examples and practice problems to help solidify these concepts:
Example 1: Simplify \( \sqrt{-16} \)
- Rewrite the expression as \( \sqrt{-1 \cdot 16} \).
- Separate into \( \sqrt{-1} \cdot \sqrt{16} \).
- Simplify to \( i \cdot 4 \).
- The final answer is \( 4i \).
Example 2: Simplify \( \sqrt{-25} \)
- Rewrite the expression as \( \sqrt{-1 \cdot 25} \).
- Separate into \( \sqrt{-1} \cdot \sqrt{25} \).
- Simplify to \( i \cdot 5 \).
- The final answer is \( 5i \).
Example 3: Simplify \( \sqrt{-48} \)
- Rewrite the expression as \( \sqrt{-1 \cdot 48} \).
- Factorize 48: \( 48 = 16 \cdot 3 \).
- Separate into \( \sqrt{-1} \cdot \sqrt{16} \cdot \sqrt{3} \).
- Simplify to \( i \cdot 4 \cdot \sqrt{3} \).
- The final answer is \( 4i \sqrt{3} \).
Practice Problems
- Problem 1: Simplify \( \sqrt{-9} \)
- Problem 2: Simplify \( \sqrt{-36} \)
- Problem 3: Simplify \( \sqrt{-100} \)
- Problem 4: Simplify \( \sqrt{-72} \)
- Problem 5: Simplify \( \sqrt{-200} \)
Solution: \( 3i \)
Solution: \( 6i \)
Solution: \( 10i \)
Solution: \( 6i \sqrt{2} \)
Solution: \( 10i \sqrt{2} \)
Working through these examples and practice problems will help reinforce your understanding of simplifying square roots of negative numbers and handling imaginary units.
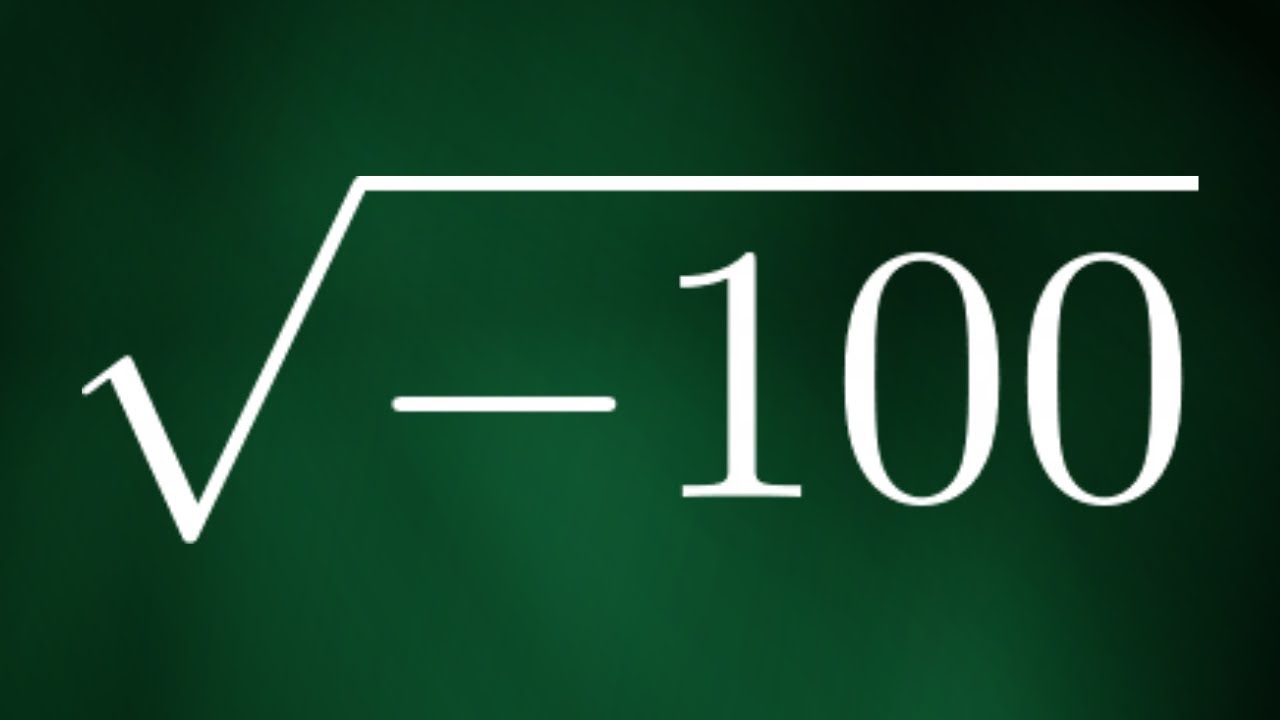
Further Reading and Resources
To deepen your understanding of simplifying the square root of negative numbers and exploring imaginary numbers, consider the following resources:
- Online Tutorials and Guides
- - A comprehensive guide on using the product and quotient rules to simplify square roots, including detailed examples and practice problems.
- - An interactive resource explaining the rules and steps for simplifying square roots, with examples and visual aids.
- - A detailed textbook section covering the principles of simplifying square roots, with numerous examples and exercises to practice.
- Books
- The Joy of x: A Guided Tour of Math, from One to Infinity by Steven Strogatz - A popular math book that provides insight into various mathematical concepts, including complex numbers and their applications.
- Visual Complex Analysis by Tristan Needham - A visually-oriented book that covers complex numbers and their geometric interpretations, suitable for those interested in a deeper understanding.
- Courses
- - A series of free online courses that cover imaginary and complex numbers, from basic concepts to advanced applications.
- - Comprehensive courses that include modules on complex numbers, offered by various universities and institutions.
- Practice Problems
- - Provides worksheets and practice problems on simplifying square roots and complex numbers.
- - An interactive learning platform offering practice problems and immediate feedback to reinforce your understanding.
These resources will provide you with a thorough understanding and ample practice in simplifying square roots and working with imaginary and complex numbers.
Đơn giản hóa căn bậc hai của -100
READ MORE:
Hướng dẫn - Cách đơn giản hóa căn bậc hai của số âm bằng đơn vị ảo i ví dụ 8