Topic simplify square root of 384: Unlock the secrets of simplifying the square root of 384 with our easy-to-follow guide. Discover the step-by-step process to break down and simplify square roots, making complex calculations a breeze. Perfect for students and math enthusiasts, this article will boost your confidence in tackling similar problems effortlessly.
Table of Content
- Simplifying the Square Root of 384
- Introduction to Simplifying Square Roots
- Understanding Square Roots
- What is the Square Root of 384?
- Prime Factorization Method
- Steps to Simplify the Square Root of 384
- Finding the Prime Factors of 384
- Breaking Down the Factors
- Simplifying the Radical Expression
- Square Roots of Prime Numbers
- Perfect Squares and Their Role
- Combining Like Terms
- Using Factor Trees for Simplification
- Alternative Methods to Simplify Square Roots
- Common Mistakes to Avoid
- Practice Problems
- YOUTUBE: Hướng dẫn từng bước để đơn giản hóa căn bậc hai của √(384x^4y^3), giúp bạn nắm vững các kỹ năng toán học cơ bản.
Simplifying the Square Root of 384
To simplify the square root of 384, we need to find the prime factorization of 384 and then simplify the square root expression using those factors.
Step-by-Step Process
- Find the prime factorization of 384.
- 384 is even, so divide by 2: \( 384 \div 2 = 192 \)
- 192 is even, so divide by 2: \( 192 \div 2 = 96 \)
- 96 is even, so divide by 2: \( 96 \div 2 = 48 \)
- 48 is even, so divide by 2: \( 48 \div 2 = 24 \)
- 24 is even, so divide by 2: \( 24 \div 2 = 12 \)
- 12 is even, so divide by 2: \( 12 \div 2 = 6 \)
- 6 is even, so divide by 2: \( 6 \div 2 = 3 \)
- 3 is a prime number.
- Rewrite the square root of 384 using its prime factorization.
- Simplify the expression by grouping the factors in pairs.
- Take the square root of the perfect squares out of the radical.
Therefore, the prime factorization of 384 is \( 2^7 \times 3 \).
\[ \sqrt{384} = \sqrt{2^7 \times 3} \]
\[ \sqrt{2^7 \times 3} = \sqrt{(2^6 \times 2) \times 3} = \sqrt{2^6} \times \sqrt{2 \times 3} \]
\[ \sqrt{2^6} = 2^3 = 8 \]
\[ \sqrt{2^7 \times 3} = 8 \sqrt{6} \]
Conclusion
The simplified form of \( \sqrt{384} \) is \( 8\sqrt{6} \).

READ MORE:
Introduction to Simplifying Square Roots
Simplifying square roots involves breaking down the number under the square root into its prime factors and simplifying the expression by removing perfect squares. This process helps in making calculations easier and understanding the properties of numbers better.
For example, to simplify the square root of 384, we can follow these steps:
- Find the prime factorization of 384.
- Rewrite the square root using the prime factorization.
- Group the factors in pairs.
- Simplify the expression by taking the square root of the perfect squares.
The prime factorization of 384 is \( 2^7 \times 3 \).
\[ \sqrt{384} = \sqrt{2^7 \times 3} \]
\[ \sqrt{2^7 \times 3} = \sqrt{(2^6 \times 2) \times 3} = \sqrt{2^6} \times \sqrt{2 \times 3} \]
\[ \sqrt{2^6} = 2^3 = 8 \]
Therefore, \[ \sqrt{384} = 8 \sqrt{6} \]
By understanding and applying these steps, you can simplify square roots of any number, making it easier to work with radical expressions in various mathematical problems.
Understanding Square Roots
Square roots are a fundamental concept in mathematics, involving finding a number which, when multiplied by itself, gives the original number. The symbol for the square root is \( \sqrt{} \), known as the radical sign. For example, the square root of 9 is 3, because \( 3 \times 3 = 9 \).
In general, for any non-negative number \( x \), there exists a non-negative number \( y \) such that \( y \times y = x \). This number \( y \) is denoted as \( \sqrt{x} \). Square roots are particularly useful in various fields of science, engineering, and mathematics, especially in solving quadratic equations and working with areas and volumes.
When simplifying square roots, the goal is to express the root in its simplest form. This often involves factoring the number under the radical sign into its prime factors and then simplifying.
Example: Simplifying the Square Root of 384
Let's consider the square root of 384 as an example:
Step 1: Find the prime factorization of 384:
- 384 can be factored into \( 2^7 \times 3 \).
Step 2: Group the prime factors into pairs:
- \( 384 = 2^2 \times 2^2 \times 2^2 \times 2 \times 3 \)
Step 3: Simplify by taking one factor from each pair outside the radical:
- \( \sqrt{384} = \sqrt{(2^2 \times 2^2 \times 2^2) \times (2 \times 3)} \)
- \( \sqrt{384} = 2 \times 2 \times 2 \times \sqrt{6} \)
- \( \sqrt{384} = 8 \sqrt{6} \)
Therefore, the simplified form of the square root of 384 is \( 8 \sqrt{6} \).
This method can be applied to any number to simplify its square root by breaking it down into its prime factors, grouping them, and simplifying accordingly.
Understanding square roots and how to simplify them is a crucial skill in mathematics, enabling more straightforward solutions to various problems.
What is the Square Root of 384?
The square root of a number is a value that, when multiplied by itself, gives the original number. For the number 384, its square root is a number that, when squared, equals 384. We denote the square root of 384 as \( \sqrt{384} \).
To find the square root of 384, we can use the method of prime factorization and simplify the radical expression. Here's how we do it step by step:
- Prime Factorization:
First, we find the prime factors of 384. We repeatedly divide 384 by the smallest prime numbers until we are left with 1.
- 384 ÷ 2 = 192
- 192 ÷ 2 = 96
- 96 ÷ 2 = 48
- 48 ÷ 2 = 24
- 24 ÷ 2 = 12
- 12 ÷ 2 = 6
- 6 ÷ 2 = 3
- 3 ÷ 3 = 1
So, the prime factorization of 384 is \( 2^7 \times 3 \).
- Grouping the Factors:
Next, we group the prime factors in pairs.
\( 384 = 2^7 \times 3 = (2^2 \times 2^2 \times 2^2) \times (2 \times 3) \)
- Taking Square Roots of the Groups:
We take one number out of each pair and multiply them together, while the remaining factors stay under the square root sign.
- \( \sqrt{384} = \sqrt{(2^2 \times 2^2 \times 2^2) \times (2 \times 3)} \)
- \( \sqrt{384} = 2 \times 2 \times 2 \times \sqrt{6} \)
- \( \sqrt{384} = 8 \sqrt{6} \)
Therefore, the square root of 384 in its simplest radical form is \( 8 \sqrt{6} \). This means that 384 can be broken down into the product of \( 8 \) and \( \sqrt{6} \), providing a simpler expression for mathematical calculations.
In decimal form, the square root of 384 is approximately 19.595917942265. This value is useful in various applications where an exact radical form is not required, and a numerical approximation is sufficient.
Prime Factorization Method
Prime factorization is a systematic method for simplifying square roots by breaking down the number into its prime factors. Here are the detailed steps to simplify the square root of 384 using the prime factorization method:
- Find Prime Factors: Start by finding the prime factors of 384.
384 can be written as:
\(384 = 2 \times 2 \times 2 \times 2 \times 2 \times 2 \times 3\)
- Group the Prime Factors: Group the prime factors into pairs.
\(384 = (2 \times 2) \times (2 \times 2) \times (2 \times 2) \times 3\)
- Take One Factor from Each Pair: For each pair of prime factors, take one factor out of the square root sign.
\(\sqrt{384} = \sqrt{(2 \times 2) \times (2 \times 2) \times (2 \times 2) \times 3}\)
\(\sqrt{384} = 2 \times 2 \times 2 \times \sqrt{3}\)
- Multiply the Factors: Multiply the factors taken out of the square root sign.
\(\sqrt{384} = 8\sqrt{3}\)
Therefore, the simplified form of the square root of 384 is \(8\sqrt{3}\).
This process shows how breaking down the number into its prime factors can help in simplifying the square root effectively.
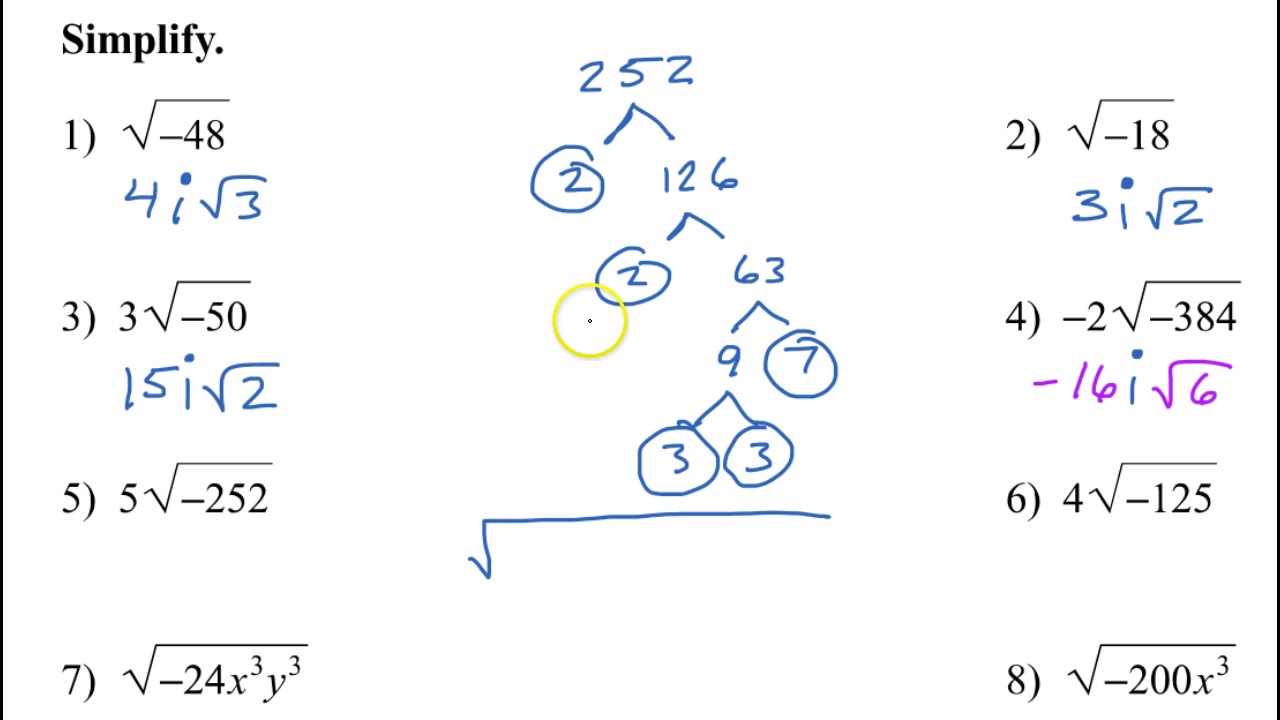
Steps to Simplify the Square Root of 384
To simplify the square root of 384, follow these detailed steps:
- Prime Factorization:
Start by finding the prime factors of 384. The prime factorization of 384 is:
\[ 384 = 2^7 \times 3^1 \]
- Group the Factors:
Group the prime factors in pairs of two:
\[ 384 = (2^2 \times 2^2 \times 2^2) \times (2 \times 3) \]
- Extract the Square Roots:
Take the square root of each group of pairs and bring them outside the radical:
\[ \sqrt{384} = \sqrt{(2^2 \times 2^2 \times 2^2) \times (2 \times 3)} = 2 \times 2 \times 2 \times \sqrt{6} = 8\sqrt{6} \]
- Simplify:
The simplest radical form of the square root of 384 is:
\[ \sqrt{384} = 8\sqrt{6} \]
So, the simplified form of \(\sqrt{384}\) is \(8\sqrt{6}\).
Finding the Prime Factors of 384
Prime factorization is a method used to express a number as the product of its prime factors. To simplify the square root of 384, we first need to find its prime factors. Here are the steps to determine the prime factors of 384:
- Divide by the smallest prime number: Begin with the smallest prime number, which is 2. Since 384 is even, it is divisible by 2.
- \(384 \div 2 = 192\)
- Repeat the process: Continue dividing the quotient by 2 until it is no longer divisible by 2.
- \(192 \div 2 = 96\)
- \(96 \div 2 = 48\)
- \(48 \div 2 = 24\)
- \(24 \div 2 = 12\)
- \(12 \div 2 = 6\)
- \(6 \div 2 = 3\)
- Identify the remaining prime factor: The last quotient, 3, is a prime number and cannot be divided further by 2.
- Therefore, \(3\) is also a prime factor of 384.
By following these steps, we can express 384 as a product of prime factors:
\(384 = 2 \times 2 \times 2 \times 2 \times 2 \times 2 \times 2 \times 3 = 2^7 \times 3^1\)
Thus, the prime factors of 384 are:
- Seven 2s: \(2 \times 2 \times 2 \times 2 \times 2 \times 2 \times 2\)
- One 3: \(3\)
Understanding these prime factors is essential for simplifying the square root of 384, which involves grouping these factors appropriately.
Breaking Down the Factors
To simplify the square root of 384, we need to break it down into its prime factors. Here are the steps to achieve this:
- First, find the prime factors of 384. Start by dividing 384 by the smallest prime number, which is 2:
- 384 ÷ 2 = 192
- 192 ÷ 2 = 96
- 96 ÷ 2 = 48
- 48 ÷ 2 = 24
- 24 ÷ 2 = 12
- 12 ÷ 2 = 6
- 6 ÷ 2 = 3
- Now, 3 is a prime number, so we stop here. The prime factors of 384 are:
- Next, pair the prime factors in groups of two:
- Simplify the pairs:
- Since the square root of a product is the product of the square roots, we can separate the terms:
- Therefore, the simplified form of the square root of 384 is:
\[ 384 = 2^7 \times 3^1 \]
\[ 384 = (2^2 \times 2^2 \times 2^2) \times (2 \times 3) \]
\[ \sqrt{384} = \sqrt{(2^2 \times 2^2 \times 2^2) \times (2 \times 3)} \]
\[ \sqrt{384} = \sqrt{2^2 \times 2^2 \times 2^2} \times \sqrt{2 \times 3} \]
\[ \sqrt{384} = 2 \times 2 \times 2 \times \sqrt{6} \]
\[ \sqrt{384} = 8 \sqrt{6} \]
\[ \sqrt{384} = 8 \sqrt{6} \]
This method ensures that the square root of 384 is expressed in its simplest radical form.
Simplifying the Radical Expression
To simplify the square root of 384, we need to break it down into its prime factors and then apply the properties of square roots to simplify the expression. Here are the steps to simplify the radical expression:
Start by finding the prime factorization of 384.
Write the number under the radical as a product of its prime factors.
Combine the factors into pairs of identical factors to use the product property of roots.
Use the product property of roots to separate the perfect square factors from the radical.
Simplify the square root of the perfect square factor.
Combine the simplified root with the remaining factors under the radical.
\(384 = 2^7 \times 3\)
\(\sqrt{384} = \sqrt{2^7 \times 3}\)
\(\sqrt{2^7 \times 3} = \sqrt{(2^6 \times 2) \times 3} = \sqrt{(2^3)^2 \times 2 \times 3}\)
\(\sqrt{(2^3)^2 \times 2 \times 3} = \sqrt{(2^3)^2} \times \sqrt{2 \times 3}\)
\(\sqrt{(2^3)^2} = 2^3 = 8\)
\(\sqrt{384} = 8 \sqrt{6}\)
Therefore, the simplified form of \(\sqrt{384}\) is \(8 \sqrt{6}\).

Square Roots of Prime Numbers
Understanding the square roots of prime numbers is essential for simplifying radical expressions. A prime number is a natural number greater than 1 that has no positive divisors other than 1 and itself. Prime numbers are fundamental in mathematics because they are the building blocks of all numbers. When dealing with square roots of prime numbers, it is important to note that they often result in irrational numbers.
Here are some key points about the square roots of prime numbers:
- Irrational Numbers: The square root of a prime number is always irrational. This means it cannot be expressed as a simple fraction and its decimal form is non-repeating and non-terminating.
- Prime Factorization: Prime factorization is the process of breaking down a number into its prime factors. For instance, the number 384 can be broken down into its prime factors to simplify its square root.
- Perfect Squares: A perfect square is a number that is the square of an integer. The square root of a perfect square is always an integer. However, the square root of a non-perfect square, including primes, is irrational.
Let's consider the prime factorization of 384 to understand its simplification:
| Step | Prime Factorization |
| 1 | 384 ÷ 2 = 192 |
| 2 | 192 ÷ 2 = 96 |
| 3 | 96 ÷ 2 = 48 |
| 4 | 48 ÷ 2 = 24 |
| 5 | 24 ÷ 2 = 12 |
| 6 | 12 ÷ 2 = 6 |
| 7 | 6 ÷ 2 = 3 |
The prime factorization of 384 is \(2^7 \times 3\). Using this factorization, we can simplify the square root of 384:
\[
\sqrt{384} = \sqrt{2^7 \times 3} = \sqrt{(2^6 \times 2) \times 3} = \sqrt{2^6 \times 2 \times 3} = \sqrt{2^6} \times \sqrt{2 \times 3} = 2^3 \times \sqrt{6} = 8\sqrt{6}
\]
This shows that the square root of 384 simplifies to \(8\sqrt{6}\), demonstrating the use of prime factorization and understanding the properties of square roots of prime numbers.
Perfect Squares and Their Role
Perfect squares play a crucial role in simplifying square roots. A perfect square is a number that can be expressed as the product of an integer with itself. For instance, 1, 4, 9, 16, 25, etc., are perfect squares. Recognizing perfect squares within a number allows us to simplify square roots efficiently.
When simplifying the square root of 384, we first identify the perfect squares that are factors of 384. Here’s a step-by-step breakdown:
- List the factors of 384:
- 1, 2, 3, 4, 6, 8, 12, 16, 24, 32, 48, 64, 96, 128, 192, 384
- Identify the perfect squares among these factors:
- 1, 4, 16, 64
- Divide 384 by the largest perfect square:
- 384 ÷ 64 = 6
- Simplify the square root:
The square root of the largest perfect square is √64 = 8. Therefore, we can rewrite √384 as follows:
\[\sqrt{384} = \sqrt{64 \times 6} = \sqrt{64} \times \sqrt{6} = 8\sqrt{6}\]
This method leverages the property of square roots which states that the square root of a product is the product of the square roots of the factors. Using perfect squares simplifies the expression significantly.
By understanding and utilizing perfect squares, the process of simplifying square roots becomes more manageable and straightforward. This is a fundamental technique in algebra that aids in breaking down complex radicals into simpler, more understandable forms.
Combining Like Terms
Combining like terms is a crucial step in simplifying expressions involving square roots. Here are the steps to combine like terms effectively:
- Identify Like Terms:
Like terms are those that contain the same radical part. For example, \(3\sqrt{2}\) and \(5\sqrt{2}\) are like terms because they both contain \(\sqrt{2}\).
- Simplify Each Term:
Ensure that each radical is in its simplest form before combining. For example, simplify \( \sqrt{50} \) to \( 5\sqrt{2} \) before attempting to combine it with other terms.
- Add or Subtract Coefficients:
Once the radicals are in their simplest form, add or subtract the coefficients. For instance, if you have \(3\sqrt{2}\) and \(5\sqrt{2}\), you can combine them to get \( (3 + 5)\sqrt{2} = 8\sqrt{2} \).
- Handle Unlike Terms Separately:
If terms have different radical parts, they cannot be combined directly. For example, \(3\sqrt{2} + 4\sqrt{3}\) cannot be simplified further because \(\sqrt{2}\) and \(\sqrt{3}\) are different radicals.
- Example:
Let's simplify the expression \(2\sqrt{6} + 3\sqrt{6} - \sqrt{24}\).
- First, simplify \(\sqrt{24}\) to \(2\sqrt{6}\).
- The expression becomes \(2\sqrt{6} + 3\sqrt{6} - 2\sqrt{6}\).
- Combine the like terms: \((2 + 3 - 2)\sqrt{6} = 3\sqrt{6}\).
Combining like terms allows us to simplify expressions and make them easier to work with. Practice this technique with various problems to master the process.
Using Factor Trees for Simplification
Simplifying the square root of 384 using a factor tree is an effective method to break down the number into its prime factors and then simplify the radical expression. Here are the steps to follow:
-
Start with the number 384 and create branches to its factors:
- 384 can be divided by 2, giving us 192.
- 192 can be divided by 2, giving us 96.
- 96 can be divided by 2, giving us 48.
- 48 can be divided by 2, giving us 24.
- 24 can be divided by 2, giving us 12.
- 12 can be divided by 2, giving us 6.
- 6 can be divided by 2, giving us 3.
- 3 is a prime number.
-
Write down all the prime factors of 384:
\[
384 = 2 \times 2 \times 2 \times 2 \times 2 \times 2 \times 3
\] -
Group the prime factors into pairs:
- \((2 \times 2), (2 \times 2), (2 \times 2), (3)\)
-
For each pair of prime factors, take one factor out of the square root:
\[
\sqrt{384} = \sqrt{2^2 \times 2^2 \times 2^2 \times 3} = 2 \times 2 \times 2 \times \sqrt{3} = 8\sqrt{3}
\] -
The simplified form of the square root of 384 is:
\[
8\sqrt{3}
\]
By following these steps, you can simplify the square root of any number using factor trees, ensuring each step is clear and methodical. This approach helps to visualize the factorization process and makes it easier to handle more complex numbers.

Alternative Methods to Simplify Square Roots
Simplifying square roots can be done using various methods. While the prime factorization and factor tree methods are popular, there are other techniques that can also be effective. Here, we explore several alternative methods to simplify square roots.
Method 1: Using the Product Property of Square Roots
The product property of square roots states that \(\sqrt{a \times b} = \sqrt{a} \times \sqrt{b}\). This property can be used to break down a square root into simpler components.
- Identify two factors of the number under the square root such that one of them is a perfect square.
- Apply the product property.
- Simplify the perfect square.
Example:
\(\sqrt{50} = \sqrt{25 \times 2} = \sqrt{25} \times \sqrt{2} = 5\sqrt{2}\)
Method 2: Rationalizing the Denominator
When dealing with square roots in the denominator, rationalizing can be an effective method. This involves eliminating the square root from the denominator by multiplying both the numerator and the denominator by a suitable value.
- Identify the square root in the denominator.
- Multiply both the numerator and the denominator by the same square root.
- Simplify the resulting expression.
Example:
\(\frac{1}{\sqrt{2}} \times \frac{\sqrt{2}}{\sqrt{2}} = \frac{\sqrt{2}}{2}\)
Method 3: Estimation and Approximation
In some cases, estimating the square root can be helpful, especially when dealing with large numbers or when an exact value is not necessary. This method involves finding the nearest perfect squares.
- Identify the perfect squares nearest to the given number.
- Estimate the square root based on these perfect squares.
Example:
\(\sqrt{52}\) is between \(\sqrt{49}\) and \(\sqrt{64}\), so \(\sqrt{52} \approx 7.2\)
Method 4: Using Exponents
Expressing square roots as exponents can also simplify the process, particularly when dealing with fractional exponents.
- Rewrite the square root using exponent notation.
- Simplify the expression using exponent rules.
Example:
\(\sqrt[3]{8} = 8^{1/3} = 2\)
Method 5: Simplifying Radicals with Variables
When simplifying square roots that involve variables, the same principles apply. However, it's important to consider the properties of exponents and ensure that variables are simplified appropriately.
- Factor the expression inside the square root.
- Simplify each factor separately.
Example:
\(\sqrt{x^4y^2} = x^2y\)
Practice Problems
Try simplifying these square roots using the alternative methods discussed:
- \(\sqrt{75}\)
- \(\frac{3}{\sqrt{5}}\)
- \(\sqrt{72}\)
- \(\sqrt[4]{16}\)
- \(\sqrt{a^2b^4}\)
Common Mistakes to Avoid
When simplifying square roots, it is crucial to be aware of common mistakes that can lead to incorrect results. Here are some key errors to watch out for and how to avoid them:
- Skipping Prime Factorization: Ensure to fully factor the radicand into its prime factors. Missing this step can result in incomplete simplification.
- Misidentifying Perfect Squares: Always double-check to correctly identify perfect squares within the radicand. Confusing these can significantly affect your simplification.
- Incorrectly Simplifying Variables: When dealing with variables, apply the rules accurately. For example, remember that \\( \sqrt{x^2} = x \\), not \\( x^2 \\).
- Overlooking Rationalization: If your simplified expression includes a square root in the denominator, make sure to rationalize it by multiplying the numerator and denominator by the appropriate square root.
- Miscalculating Non-Perfect Squares: Approximations should be checked for accuracy. Verify your calculations to ensure precision.
- Forgetting Absolute Values: When variables are involved, remember to include absolute value signs where necessary, as this affects the meaning of the solution.
By keeping these common mistakes in mind and following a careful, step-by-step approach, you can enhance your accuracy and proficiency in simplifying square roots.
Practice Problems
Try simplifying the following square roots using the methods discussed above:
- Simplify \( \sqrt{128} \).
- Simplify \( \sqrt{250} \).
- Simplify \( \sqrt{72} \).
- Simplify \( \sqrt{98} \).
- Simplify \( \sqrt{450} \).
Solutions:
-
\( \sqrt{128} \)
- Prime factorization: \( 128 = 2^7 \)
- Breaking it down: \( \sqrt{2^7} = \sqrt{2^6 \cdot 2} = 2^3 \sqrt{2} = 8\sqrt{2} \)
-
\( \sqrt{250} \)
- Prime factorization: \( 250 = 2 \cdot 5^3 \)
- Breaking it down: \( \sqrt{2 \cdot 5^3} = 5\sqrt{10} \)
-
\( \sqrt{72} \)
- Prime factorization: \( 72 = 2^3 \cdot 3^2 \)
- Breaking it down: \( \sqrt{2^3 \cdot 3^2} = 6\sqrt{2} \)
-
\( \sqrt{98} \)
- Prime factorization: \( 98 = 2 \cdot 7^2 \)
- Breaking it down: \( \sqrt{2 \cdot 7^2} = 7\sqrt{2} \)
-
\( \sqrt{450} \)
- Prime factorization: \( 450 = 2 \cdot 3^2 \cdot 5^2 \)
- Breaking it down: \( \sqrt{2 \cdot 3^2 \cdot 5^2} = 15\sqrt{2} \)
Remember to check your answers by squaring them to ensure you return to the original numbers under the square roots.
Hướng dẫn từng bước để đơn giản hóa căn bậc hai của √(384x^4y^3), giúp bạn nắm vững các kỹ năng toán học cơ bản.
Đơn giản hóa √(384x^4y^3)
READ MORE:
Khám phá cách đơn giản hóa căn bậc hai bằng cách sử dụng các căn bậc hai hoàn hảo trong video này.
Ví dụ: Đơn giản hóa Căn Bậc Hai - Căn Bậc Hai Hoàn Hảo