Topic simplify square root of 432: Welcome to our comprehensive guide on simplifying the square root of 432. This article will take you through the process step-by-step, using prime factorization and other methodologies to make the concept easy to understand. Whether you're a student or a math enthusiast, this guide will help you master simplifying square roots.
Table of Content
Simplify Square Root of 432
To simplify the square root of 432, we start by finding its prime factors.
| 432 | ÷ 2 = 216 |
| 216 | ÷ 2 = 108 |
| 108 | ÷ 2 = 54 |
| 54 | ÷ 2 = 27 |
| 27 | ÷ 3 = 9 |
| 9 | ÷ 3 = 3 |
| 3 | ÷ 3 = 1 |
Prime factors of 432 are 2, 2, 2, 2, 3, 3, 3.
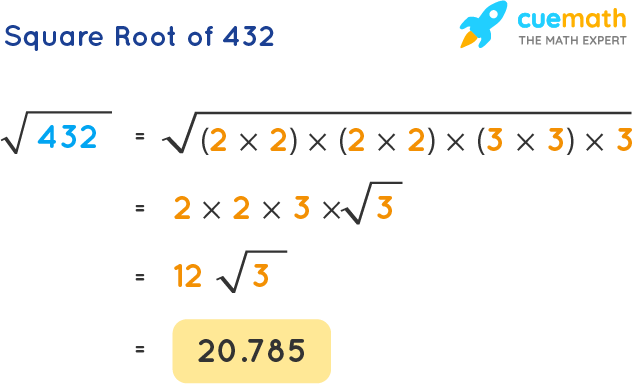
Therefore, √432 = √(24 × 33) = 12√3.
READ MORE:
Introduction
Simplifying the square root of 432 might seem challenging at first, but with a clear understanding of prime factorization and basic algebraic rules, the process becomes straightforward. This guide will walk you through each step to simplify √432 into its simplest form, making it easier to handle in various mathematical contexts.
To simplify √432, we need to find its prime factors and group them appropriately. By the end of this guide, you will be able to simplify the square root of any number using similar techniques.
Understanding Square Roots
The concept of a square root is fundamental in mathematics. The square root of a number is a value that, when multiplied by itself, gives the original number. It is represented by the radical symbol √. For example, the square root of 9 is 3, because 3 multiplied by 3 equals 9. Square roots are used in various fields such as geometry, algebra, and calculus.
There are several key points to understand about square roots:
- Positive and Negative Roots: Every positive number has two square roots: a positive root and a negative root. For instance, both 3 and -3 are square roots of 9.
- Perfect Squares: Numbers like 1, 4, 9, 16, and 25 are perfect squares because their square roots are whole numbers.
- Irrational Numbers: Some numbers do not have a perfect square root. These roots are irrational numbers, meaning they cannot be expressed as a simple fraction. For example, the square root of 2 is approximately 1.414, a non-repeating, non-terminating decimal.
When simplifying square roots, especially for non-perfect squares like 432, we often use prime factorization to make the process easier. Let's delve into the methodologies for simplifying square roots in the next section.
Methodologies to Simplify Square Roots
Simplifying square roots involves breaking down the number under the square root into its prime factors and identifying perfect squares. Here are the key steps to simplify square roots:
-
Identify the Prime Factors: Break down the number into its prime factors. For example, \(432 = 2^4 \times 3^3\).
-
Pair the Prime Factors: Group the prime factors into pairs. Each pair will be simplified outside the square root.
-
Simplify Using Pairs: For every pair of prime factors, one factor comes out of the square root. This reduces the square root to a simpler form.
For instance, with the square root of \(432\):
- \(432 = 2^4 \times 3^3\)
- Pairs: \(2^4\) becomes \(2^2\) outside the square root, and \(3^2\) from \(3^3\) becomes \(3\) outside the square root.
- The simplified form: \(2^2 \times 3 \times \sqrt{3} = 12\sqrt{3}\).
Using these methodologies helps to simplify square roots efficiently.
Step-by-Step Guide to Simplify √432
To simplify \( \sqrt{432} \), follow these detailed steps:
-
Find the Prime Factorization: Start by finding the prime factors of 432.
- Divide by 2: \(432 \div 2 = 216\)
- Divide by 2: \(216 \div 2 = 108\)
- Divide by 2: \(108 \div 2 = 54\)
- Divide by 2: \(54 \div 2 = 27\)
- Divide by 3: \(27 \div 3 = 9\)
- Divide by 3: \(9 \div 3 = 3\)
- Divide by 3: \(3 \div 3 = 1\)
The prime factorization of 432 is \(2^4 \times 3^3\).
-
Group the Prime Factors: Pair the prime factors to simplify the square root.
- Pairs of 2s: \(2^4\) results in two pairs of 2.
- Pairs of 3s: \(3^3\) results in one pair of 3 and one 3 left inside the square root.
-
Simplify the Expression: For each pair, one number comes out of the square root.
- Two 2s come out as \(2 \times 2 = 4\).
- One 3 comes out as 3.
Combine these: \(4 \times 3 = 12\).
-
Final Simplified Form: The remaining number inside the square root is 3.
Thus, \( \sqrt{432} = 12\sqrt{3} \).
This method ensures an accurate and simplified result for the square root of 432.

Prime Factorization of 432
Prime factorization involves breaking down a number into its prime components. Here's how to find the prime factorization of 432:
-
Start with the Smallest Prime: Begin with 2, the smallest prime number.
- \(432 \div 2 = 216\)
- \(216 \div 2 = 108\)
- \(108 \div 2 = 54\)
- \(54 \div 2 = 27\)
-
Move to the Next Prime: Since 27 is odd, proceed to the next prime number, which is 3.
- \(27 \div 3 = 9\)
- \(9 \div 3 = 3\)
- \(3 \div 3 = 1\)
-
Compile the Prime Factors: After all divisions, the prime factors are identified.
The prime factorization of 432 is \(2^4 \times 3^3\).
This breakdown demonstrates that 432 consists of four 2's and three 3's, providing a clear and organized factorization.
Calculating Square Roots Using Prime Factors
Calculating square roots using prime factors is an effective method to simplify the expression. Here’s how to apply this method to \( \sqrt{432} \):
-
Determine the Prime Factorization: First, express 432 as a product of its prime factors.
- Prime factorization of 432: \(2^4 \times 3^3\)
-
Identify Pairs of Factors: Group the prime factors into pairs, as pairs can be taken out of the square root.
- Pairs of 2s: \(2^4\) has two pairs of 2.
- Pairs of 3s: \(3^3\) has one pair of 3, with one 3 remaining.
-
Simplify the Square Root: For each pair, remove one factor from the square root.
- From \(2^4\), two 2s come out as \(2 \times 2 = 4\).
- From \(3^2\), one 3 comes out as 3.
Combine the factors outside the square root: \(4 \times 3 = 12\).
-
Write the Simplified Form: The remaining factor inside the square root is 3.
Thus, \( \sqrt{432} = 12\sqrt{3} \).
This method of using prime factorization not only simplifies the square root but also provides clarity on the factors involved.
Examples of Simplifying Square Root of 432
Simplifying the square root of 432 can be approached in several ways. Here are a few examples:
-
Using Prime Factorization:
-
Find the prime factorization: \(432 = 2^4 \times 3^3\).
-
Pair the prime factors: \(2^4\) gives two pairs of 2, and \(3^3\) gives one pair of 3 with one 3 remaining.
-
Take the pairs out of the square root: \(2^2\) and \(3\) come out as 4 and 3, respectively.
-
Simplify: \(4 \times 3 = 12\), with \( \sqrt{3} \) remaining.
-
Final result: \( \sqrt{432} = 12\sqrt{3} \).
-
-
Using a Calculator:
-
Input \( \sqrt{432} \) into a calculator.
-
The approximate decimal value is about 20.7846.
-
Using the simplified form \(12\sqrt{3}\), verify that it equals approximately 20.7846 as well.
-
These examples illustrate how to simplify the square root of 432 through different methods, emphasizing the clarity and accuracy of using prime factorization.
Conclusion
Simplifying the square root of 432 involves breaking down the number into its prime factors and extracting the square factors from under the radical sign. This process allows us to express the square root in its simplest radical form, making it easier to understand and utilize in various mathematical applications.
By following the prime factorization method, we find that:
- 432 = 2^4 × 3^3
- Grouping the prime factors in pairs gives us 2^2 and 3^1.
- Taking one number from each pair and multiplying them together outside the square root gives us 12.
- The simplified form of √432 is therefore 12√3.
This simplified form, 12√3, is much more manageable for further calculations and provides a clear understanding of the square root's structure.
Understanding and simplifying square roots is a fundamental skill in mathematics, enabling us to solve equations more efficiently and accurately. The process of simplification also reveals the underlying properties of numbers, offering insights into their composition and relationships.
In conclusion, the square root of 432, simplified to 12√3, exemplifies the importance of prime factorization and simplification techniques in making complex mathematical concepts more accessible and practical. Whether for academic purposes, practical applications, or further mathematical exploration, mastering these techniques is invaluable.

Đơn Giản Hóa [3]√(432) - 5*[3]√(2)
READ MORE:
Cách Đơn Giản Hóa Căn Bậc Hai Của Một Số Không Phải Số Chính Phương