Topic simplify square root of 245: Welcome to our comprehensive guide on how to simplify the square root of 245. This article will walk you through each step of the simplification process, making it easy to understand and apply. Whether you're a student, teacher, or math enthusiast, you'll find this guide helpful and informative.
Table of Content
- Simplifying the Square Root of 245
- Introduction
- Understanding Square Roots
- Prime Factorization Method
- Step-by-Step Guide to Simplifying √245
- Finding and Using Perfect Squares
- Applying the Product Rule for Radicals
- Converting Between Radical and Exponent Forms
- Decimal Form and Approximations
- Common Mistakes to Avoid
- Practice Problems and Solutions
- FAQs on Simplifying Square Roots
- YOUTUBE: Video hướng dẫn cách đơn giản hóa căn bậc hai của 245. Tìm hiểu các bước đơn giản để đơn giản hóa căn bậc hai của 245 một cách dễ hiểu.
Simplifying the Square Root of 245
The process of simplifying the square root of 245 involves breaking down the number into its prime factors and then simplifying the radical. Here are the steps to follow:
Step 1: Prime Factorization
First, we need to find the prime factors of 245. We can start by dividing 245 by the smallest prime number, which is 5:
\[
245 \div 5 = 49
\]
Next, we find the prime factors of 49. Since 49 is a perfect square, it can be expressed as:
\[
49 = 7 \times 7
\]
So, the prime factorization of 245 is:
\[
245 = 5 \times 7^2
\]
Step 2: Grouping Prime Factors
To simplify the square root, we group the prime factors into pairs:
\[
\sqrt{245} = \sqrt{5 \times 7^2}
\]
Step 3: Simplifying the Radical
We can simplify the square root by taking the square root of each group of prime factors. In this case, we have a pair of 7's:
\[
\sqrt{245} = \sqrt{5 \times 7^2} = 7 \sqrt{5}
\]
Thus, the simplified form of the square root of 245 is:
\[
\sqrt{245} = 7 \sqrt{5}
\]
Conclusion
By breaking down 245 into its prime factors and grouping them appropriately, we have simplified the square root of 245 to \(7 \sqrt{5}\). This process makes it easier to work with the radical in various mathematical contexts.
READ MORE:
Introduction
Understanding how to simplify the square root of 245 can greatly enhance your mathematical skills. Simplifying square roots involves breaking down the number under the radical into its prime factors and identifying any perfect squares. This guide will take you through the process step by step, ensuring you grasp the fundamental concepts and techniques required to simplify √245.
Here are the steps we will cover:
- Identifying the prime factors of 245.
- Finding the perfect squares among the factors.
- Applying the product rule for radicals.
- Expressing the simplified form using Mathjax code.
Understanding Square Roots
Square roots are mathematical operations that determine what number, when multiplied by itself, will equal the original number. The symbol for the square root is √. For example, the square root of 9 is 3 because 3 * 3 = 9.
In general, the square root of a number 'x' is written as √x. It can also be expressed in exponent form as x^(1/2).
Square roots can be either positive or negative because both (positive) and (negative) values squared will result in a positive number. However, by convention, when we refer to the square root of a number, we typically mean the principal (non-negative) square root.
Properties of Square Roots
- Square roots of perfect squares are integers. For example,
√16 = 4because 4 * 4 = 16. - Square roots of non-perfect squares are irrational numbers. For example,
√2cannot be expressed as a simple fraction and its decimal form is non-terminating and non-repeating. - The product rule of square roots states that
√(a * b) = √a * √b. - The quotient rule of square roots states that
√(a / b) = √a / √bas long as b ≠ 0.
Examples
Let's look at a few examples to understand these properties better:
- Simplifying
√12:- First, factor 12 into its prime factors:
12 = 2 * 2 * 3. - Use the product rule:
√12 = √(4 * 3) = √4 * √3. - Since
√4 = 2, we get√12 = 2√3.
- First, factor 12 into its prime factors:
- Simplifying
√50:- First, factor 50 into its prime factors:
50 = 2 * 5 * 5. - Use the product rule:
√50 = √(25 * 2) = √25 * √2. - Since
√25 = 5, we get√50 = 5√2.
- First, factor 50 into its prime factors:
Understanding and simplifying square roots is a foundational skill in algebra and higher mathematics. This process involves recognizing perfect squares and applying the product and quotient rules effectively.
Prime Factorization Method
The prime factorization method is a systematic approach to simplifying square roots by breaking down the number into its prime factors. Here’s a detailed step-by-step guide to simplify the square root of 245 using this method:
-
Step 1: Prime Factorization
First, we need to find the prime factors of 245. A prime factor is a factor that is a prime number. We start by dividing 245 by the smallest prime number, 5:
\[ 245 \div 5 = 49 \]
Next, we find the prime factors of 49:
\[ 49 = 7 \times 7 \]
Therefore, the prime factorization of 245 is:
\[ 245 = 5 \times 7 \times 7 \]
-
Step 2: Grouping the Pairs
Identify and group the pairs of prime factors. In the case of 245, we have one pair of 7s:
\[ 245 = 5 \times (7 \times 7) \]
-
Step 3: Simplifying the Radical
For each pair of prime factors, take one factor out of the square root:
\[ \sqrt{245} = \sqrt{5 \times 7 \times 7} \]
The pair of 7s comes out of the square root as a single 7:
\[ \sqrt{245} = 7 \sqrt{5} \]
Therefore, the simplified form of the square root of 245 is:
\[ \sqrt{245} = 7 \sqrt{5} \]
This process of using the prime factorization method allows us to simplify the square root of a number efficiently by leveraging the properties of prime numbers and square roots.
Step-by-Step Guide to Simplifying √245
To simplify the square root of 245, follow these detailed steps:
-
List the Factors: Identify all the factors of 245.
\[ 245 = 1, 5, 7, 35, 49, 245 \]
-
Find the Perfect Squares: From the list of factors, find the perfect squares. The perfect squares in the list are 1 and 49.
-
Divide by the Largest Perfect Square: Divide 245 by the largest perfect square, which is 49.
\[ 245 \div 49 = 5 \]
-
Calculate the Square Root of the Perfect Square: Find the square root of 49.
\[ \sqrt{49} = 7 \]
-
Combine the Results: Rewrite the original square root expression using the square root of the perfect square and the remaining factor.
\[ \sqrt{245} = \sqrt{49 \times 5} = \sqrt{49} \times \sqrt{5} = 7\sqrt{5} \]
Thus, the simplified form of \(\sqrt{245}\) is \(7\sqrt{5}\).

Finding and Using Perfect Squares
When simplifying square roots, recognizing and using perfect squares can greatly simplify the process. Perfect squares are numbers that are the product of an integer multiplied by itself. For instance, 1, 4, 9, 16, 25, and 36 are all perfect squares.
What Are Perfect Squares?
A perfect square is a number that can be expressed as \( n^2 \), where \( n \) is an integer. Here are some examples of perfect squares:
- \(1 = 1^2\)
- \(4 = 2^2\)
- \(9 = 3^2\)
- \(16 = 4^2\)
- \(25 = 5^2\)
- \(36 = 6^2\)
- \(49 = 7^2\)
- \(64 = 8^2\)
- \(81 = 9^2\)
- \(100 = 10^2\)
Using Perfect Squares to Simplify Square Roots
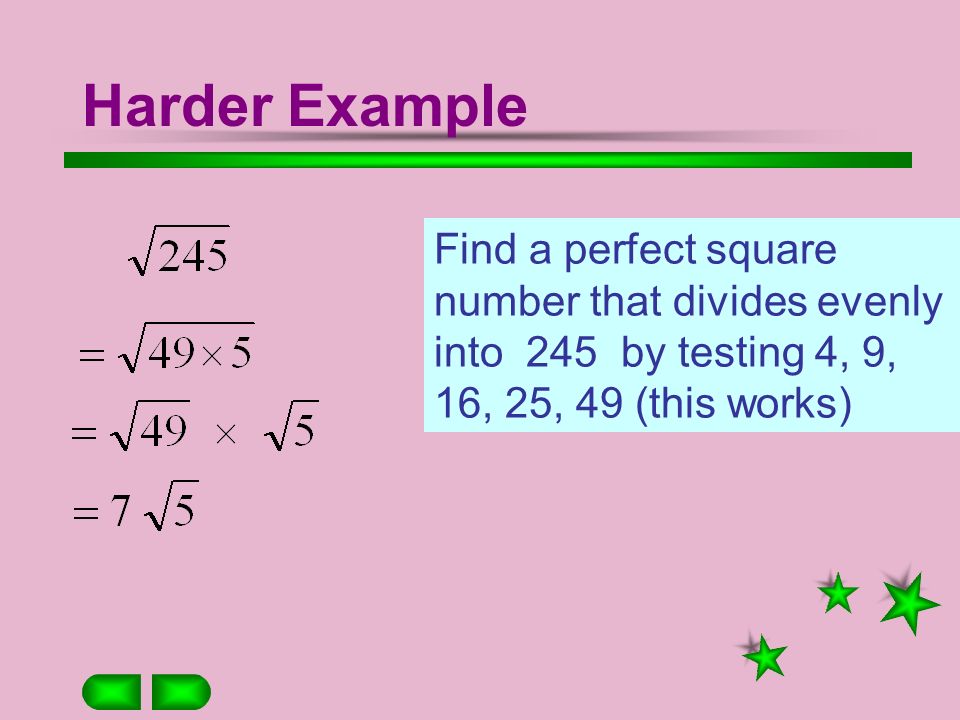
To simplify the square root of a number, we look for the largest perfect square factor. Let's apply this to \(\sqrt{245}\):
- First, identify the factors of 245. The prime factorization of 245 is \(5 \times 7 \times 7\).
- Group the prime factors into pairs: \(7 \times 7 = 49\).
- Recognize that 49 is a perfect square, since \(49 = 7^2\).
- Rewrite the original expression using the perfect square: \(\sqrt{245} = \sqrt{49 \times 5}\).
- Use the property of square roots that states \(\sqrt{a \times b} = \sqrt{a} \times \sqrt{b}\):
- \(\sqrt{245} = \sqrt{49} \times \sqrt{5} = 7\sqrt{5}\).
Thus, the simplified form of \(\sqrt{245}\) is \(7\sqrt{5}\).
Benefits of Recognizing Perfect Squares
Understanding perfect squares can help streamline the process of simplifying square roots, making it easier to work with radical expressions in mathematics.
Applying the Product Rule for Radicals
The product rule for radicals is a fundamental concept in simplifying square roots. It states that the square root of a product is equal to the product of the square roots of the factors. Mathematically, this is expressed as:
\[\sqrt{ab} = \sqrt{a} \cdot \sqrt{b}\]
Let's apply this rule to simplify \(\sqrt{245}\).
- Factor the radicand into its prime factors:
First, we find the prime factorization of 245:
- 245 is divisible by 5: \(245 \div 5 = 49\)
- 49 is a perfect square: \(49 = 7 \times 7\)
Thus, \(245 = 5 \times 7^2\).
- Apply the product rule:
Using the product rule for radicals:
\[\sqrt{245} = \sqrt{5 \times 7^2} = \sqrt{5} \cdot \sqrt{7^2}\] - Simplify the expression:
We know that \(\sqrt{7^2} = 7\), so:
\[\sqrt{245} = \sqrt{5} \cdot 7\]
Therefore:
\[\sqrt{245} = 7\sqrt{5}\]
By applying the product rule for radicals, we have simplified \(\sqrt{245}\) to \(7\sqrt{5}\).
Converting Between Radical and Exponent Forms
Converting between radical and exponent forms is a useful skill in algebra and calculus. This process allows for easier manipulation and simplification of expressions. Here, we'll explore the steps to convert radicals to exponents and vice versa.
Radicals to Exponents
A radical expression can be converted to an exponent by understanding the relationship between the radical sign and fractional exponents. The general form is:
\(\sqrt[n]{a^m} = a^{\frac{m}{n}}\)
- \(a\) is the base.
- \(m\) is the power of the base inside the radical.
- \(n\) is the index of the radical.
For example:
\(\sqrt[3]{x^2} = x^{\frac{2}{3}}\)
\(\sqrt[4]{y^5} = y^{\frac{5}{4}}\)
Exponents to Radicals
Converting exponents back to radicals involves the inverse process. The exponent is split into the power and the root:
\(a^{\frac{m}{n}} = \sqrt[n]{a^m}\)
For example:
\(x^{\frac{2}{3}} = \sqrt[3]{x^2}\)
\(y^{\frac{5}{4}} = \sqrt[4]{y^5}\)
Step-by-Step Example
Let's apply these conversions to an example:
- Convert \(\sqrt[5]{32}\) to exponent form:
- Recognize that \(\sqrt[5]{32} = 32^{\frac{1}{5}}\)
- Convert \(49^{\frac{3}{2}}\) to radical form:
- Identify the base (49), the exponent's numerator (3), and the denominator (2).
- Rewrite as \(\sqrt{49^3}\).
- Simplify inside the radical: \(\sqrt{49^3} = \sqrt{343}\).
Benefits of Conversion
- Allows for easier manipulation of algebraic expressions.
- Facilitates operations like differentiation and integration in calculus.
- Simplifies the process of solving equations involving roots and exponents.
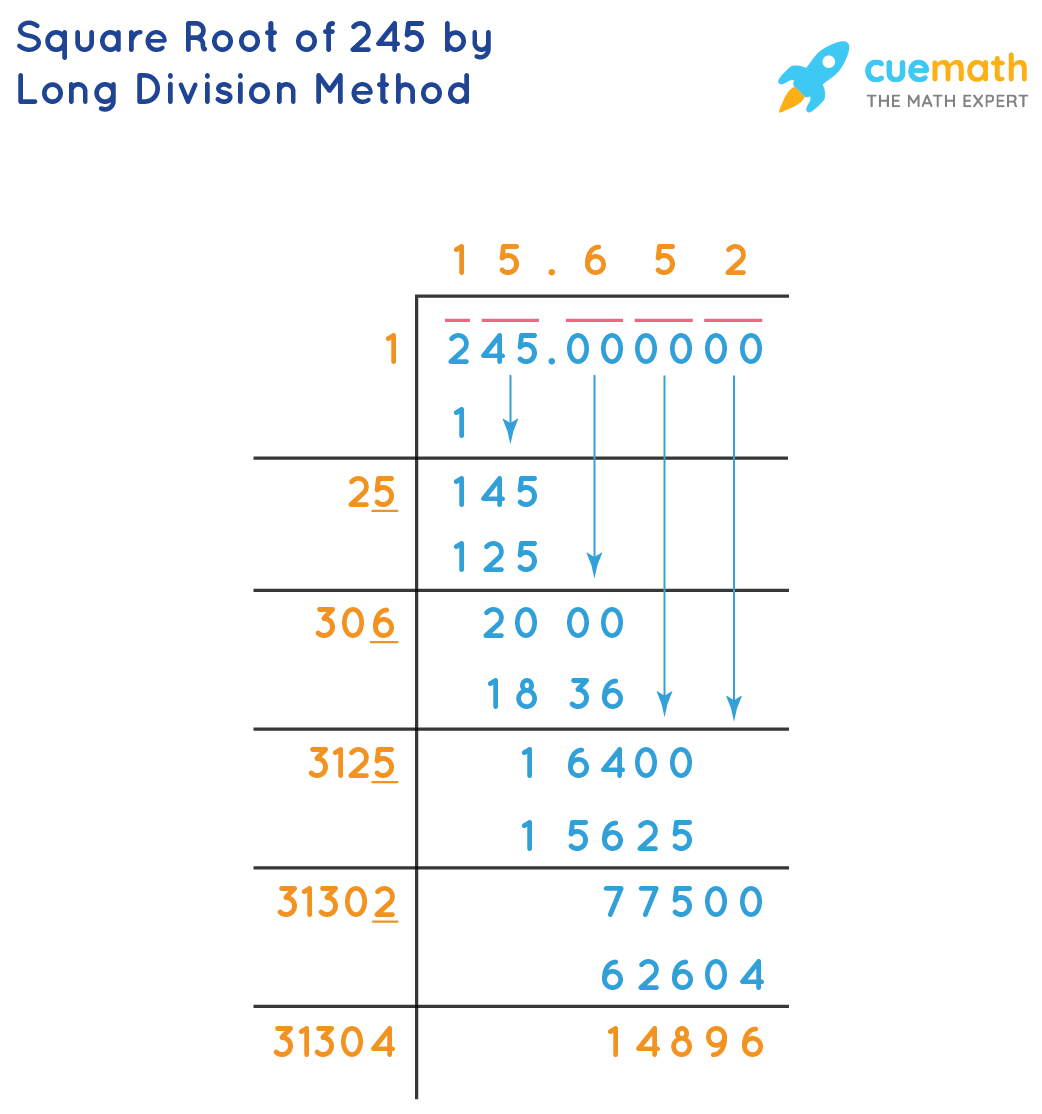
Decimal Form and Approximations
When working with square roots, it's often useful to approximate their values in decimal form. The square root of 245 (\(\sqrt{245}\)) can be approximated using a calculator to provide a decimal value.
The exact value of \(\sqrt{245}\) in decimal form is approximately:
\(\sqrt{245} \approx 15.652475\)
To better understand how we arrive at this approximation, let's break down the steps:
- Identify the nearest perfect squares:
- The square root of 225 (\(15^2\)) is 15.
- The square root of 256 (\(16^2\)) is 16.
- Locate \(\sqrt{245}\) between these perfect squares:
Since 245 is between 225 and 256, \(\sqrt{245}\) will be between 15 and 16.
- Use a calculator for precision:
By entering 245 into a square root calculator, we get the precise decimal value: 15.652475.
This decimal form is useful for many practical applications, such as engineering, physics, and everyday calculations where an approximate value is sufficient.
Additionally, understanding how to find decimal approximations of square roots helps in estimating and comparing the magnitudes of different numbers.
Common Mistakes to Avoid
When simplifying square roots, it's easy to make mistakes that can lead to incorrect results. Here are some common pitfalls to be aware of and tips to avoid them:
-
Forgetting to Check for Perfect Squares:
Before simplifying, always check if the number under the square root is a perfect square. For example, \( \sqrt{245} \) can be broken down because 245 is not a perfect square, but it contains a perfect square factor.
-
Incorrect Prime Factorization:
Ensure you correctly factorize the number into its prime components. For \( \sqrt{245} \), the prime factors are 5 and 7 (since \( 245 = 7^2 \times 5 \)). Missing or misidentifying factors will result in errors.
-
Ignoring the Product Rule for Radicals:
Use the product rule \( \sqrt{a \times b} = \sqrt{a} \times \sqrt{b} \) correctly. For instance, \( \sqrt{245} \) can be simplified to \( \sqrt{49 \times 5} = \sqrt{49} \times \sqrt{5} = 7\sqrt{5} \).
-
Rushing Through Calculations:
Take your time to work through each step carefully. Rushing can lead to missed factors or miscalculations.
-
Misplacing the Radical Sign:
Ensure the radical sign is correctly placed and extends over all terms that are supposed to be under the square root.
-
Inaccurate Simplification:
Double-check your simplified form. For example, \( \sqrt{245} \) correctly simplifies to \( 7\sqrt{5} \). Always verify your final answer by squaring it to see if it returns the original number.
By being mindful of these common mistakes and following the proper steps, you can confidently simplify square roots and avoid errors.
Practice Problems and Solutions
Here are some practice problems to help you master simplifying square roots, specifically focusing on the square root of 245:
Problem 1
Simplify \( \sqrt{245} \).
- Factor 245 into its prime factors: \( 245 = 5 \times 49 \).
- Recognize that 49 is a perfect square: \( 49 = 7^2 \).
- Rewrite the expression: \( \sqrt{245} = \sqrt{5 \times 49} = \sqrt{5 \times 7^2} \).
- Apply the product rule for radicals: \( \sqrt{5 \times 7^2} = \sqrt{5} \times \sqrt{7^2} \).
- Simplify: \( \sqrt{7^2} = 7 \), thus \( \sqrt{245} = 7\sqrt{5} \).
Solution: \( \sqrt{245} = 7\sqrt{5} \).
Problem 2
Simplify \( \sqrt{180} \).
- Factor 180 into its prime factors: \( 180 = 2^2 \times 3^2 \times 5 \).
- Rewrite the expression: \( \sqrt{180} = \sqrt{2^2 \times 3^2 \times 5} \).
- Apply the product rule for radicals: \( \sqrt{2^2 \times 3^2 \times 5} = \sqrt{2^2} \times \sqrt{3^2} \times \sqrt{5} \).
- Simplify: \( \sqrt{2^2} = 2 \) and \( \sqrt{3^2} = 3 \), thus \( \sqrt{180} = 2 \times 3 \times \sqrt{5} \).
Solution: \( \sqrt{180} = 6\sqrt{5} \).
Problem 3
Simplify \( \sqrt{98} \).
- Factor 98 into its prime factors: \( 98 = 2 \times 49 \).
- Recognize that 49 is a perfect square: \( 49 = 7^2 \).
- Rewrite the expression: \( \sqrt{98} = \sqrt{2 \times 49} = \sqrt{2 \times 7^2} \).
- Apply the product rule for radicals: \( \sqrt{2 \times 7^2} = \sqrt{2} \times \sqrt{7^2} \).
- Simplify: \( \sqrt{7^2} = 7 \), thus \( \sqrt{98} = 7\sqrt{2} \).
Solution: \( \sqrt{98} = 7\sqrt{2} \).
Problem 4
Simplify \( \sqrt{50} \).
- Factor 50 into its prime factors: \( 50 = 2 \times 25 \).
- Recognize that 25 is a perfect square: \( 25 = 5^2 \).
- Rewrite the expression: \( \sqrt{50} = \sqrt{2 \times 25} = \sqrt{2 \times 5^2} \).
- Apply the product rule for radicals: \( \sqrt{2 \times 5^2} = \sqrt{2} \times \sqrt{5^2} \).
- Simplify: \( \sqrt{5^2} = 5 \), thus \( \sqrt{50} = 5\sqrt{2} \).
Solution: \( \sqrt{50} = 5\sqrt{2} \).
FAQs on Simplifying Square Roots
-
What is the square root of 245 in simplest form?
The square root of 245 in its simplest form is \( 7\sqrt{5} \). This is obtained by finding the largest perfect square factor of 245, which is 49, and simplifying \( \sqrt{245} \) to \( \sqrt{49 \times 5} = 7\sqrt{5} \).
-
How do you simplify the square root of 245?
To simplify \( \sqrt{245} \), follow these steps:
- Find the prime factors of 245: \( 245 = 5 \times 7^2 \).
- Rewrite the square root: \( \sqrt{245} = \sqrt{5 \times 7^2} \).
- Simplify by taking the square root of 7 squared out of the radical: \( \sqrt{5} \times \sqrt{7^2} = 7\sqrt{5} \).
-
What is the approximate decimal value of \( \sqrt{245} \)?The approximate decimal value of \( \sqrt{245} \) is 15.6525.
-
Is the square root of 245 a rational number?
No, \( \sqrt{245} \) is an irrational number because it cannot be expressed as a simple fraction.
-
Is the square root of 245 a real number?
Yes, \( \sqrt{245} \) is a real number.
Video hướng dẫn cách đơn giản hóa căn bậc hai của 245. Tìm hiểu các bước đơn giản để đơn giản hóa căn bậc hai của 245 một cách dễ hiểu.
Cách đơn giản hóa căn bậc hai của 245?
READ MORE:
Video hướng dẫn cách đơn giản hóa căn bậc hai của 245. Tìm hiểu cách thức đơn giản hóa một cách hiệu quả và dễ hiểu.
Đơn giản hóa căn bậc hai của 245
















