Topic how to simplify square roots: Learn how to simplify square roots with our easy-to-follow guide. Whether you're a student tackling homework or just curious about math, this article breaks down the process into simple steps, making it accessible for everyone. Discover tips, tricks, and practice problems to master square root simplification in no time!
Table of Content
- How to Simplify Square Roots
- Introduction to Square Roots
- Understanding the Basics of Square Roots
- Prime Factorization Method for Simplifying Square Roots
- Extracting Perfect Squares from the Radicand
- Step-by-Step Process to Simplify Square Roots
- Special Cases in Simplifying Square Roots
- Working with Square Roots of Perfect Squares
- Simplifying Square Roots of Non-Perfect Squares
- Handling Square Roots of Fractions
- Simplifying Square Roots with Variables
- Practice Problems and Examples
- Common Mistakes to Avoid
- Advanced Techniques for Simplifying Square Roots
- Using Technology to Simplify Square Roots
- Applications of Simplifying Square Roots in Real Life
- Frequently Asked Questions
- Additional Resources and References
- YOUTUBE: Xem video 'Đơn Giản Hóa Căn Bậc Hai' với Thầy J để học cách đơn giản hóa căn bậc hai một cách dễ dàng và chi tiết. Phù hợp cho học sinh và người yêu thích toán học.
How to Simplify Square Roots
Simplifying square roots involves expressing a square root in its simplest form. This can often be done by identifying and extracting perfect square factors from the radicand (the number under the square root symbol).
Steps to Simplify Square Roots
-
Identify the prime factors of the number under the square root. For example, for √72, determine the prime factors of 72.
-
Group the prime factors into pairs. For instance, the prime factors of 72 are 2, 2, 2, 3, and 3. Grouping them gives us (2 × 2), (2), and (3 × 3).
-
Extract the pairs of prime factors from the square root. Each pair of primes can be taken out of the square root as a single number. So, for √72:
\(\sqrt{72} = \sqrt{(2 \times 2) \times 2 \times (3 \times 3)} = 2 \times 3 \times \sqrt{2} = 6\sqrt{2}\)
-
Simplify the expression by multiplying the numbers outside the square root. This gives the simplest form of the square root.
Additional Tips
- Remember that any square root of a perfect square (e.g., √4, √9, √16) simplifies to an integer (2, 3, 4, respectively).
- If the number under the square root is a prime number, it cannot be simplified further.
- Practice with different numbers to become more comfortable with the process.
Examples
| \(\sqrt{50}\) | = \(\sqrt{25 \times 2}\) | = \(5\sqrt{2}\) |
| \(\sqrt{18}\) | = \(\sqrt{9 \times 2}\) | = \(3\sqrt{2}\) |
| \(\sqrt{45}\) | = \(\sqrt{9 \times 5}\) | = \(3\sqrt{5}\) |
| \(\sqrt{32}\) | = \(\sqrt{16 \times 2}\) | = \(4\sqrt{2}\) |

READ MORE:
Introduction to Square Roots
Square roots are a fundamental concept in mathematics, representing a value that, when multiplied by itself, gives the original number. The square root of a number \( x \) is denoted as \( \sqrt{x} \). For example, \( \sqrt{16} = 4 \) because \( 4 \times 4 = 16 \).
To understand square roots, let's explore their properties and how to simplify them step-by-step.
-
Factorization: Identify and factorize the number inside the square root into its prime factors. For example, \( 72 = 2 \times 2 \times 2 \times 3 \times 3 \).
-
Pairing Factors: Group the factors into pairs. Each pair of the same number can be taken out of the square root. For instance, \( \sqrt{72} \) becomes \( \sqrt{(2 \times 2) \times (3 \times 3) \times 2} = 2 \times 3 \times \sqrt{2} = 6\sqrt{2} \).
-
Simplifying Fractions: When dealing with square roots in fractions, simplify using the property \( \sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}} \). For example, \( \sqrt{\frac{16}{9}} = \frac{\sqrt{16}}{\sqrt{9}} = \frac{4}{3} \).
Understanding these steps and practicing with various examples will help you master the simplification of square roots. Simplifying square roots not only makes calculations easier but also helps in solving more complex mathematical problems.
Understanding the Basics of Square Roots
Square roots are a fundamental concept in mathematics, representing a number that, when multiplied by itself, gives the original number. For example, the square root of 25 is 5, because \(5 \times 5 = 25\). The square root symbol is denoted as \(\sqrt{}\).
There are a few key points to understand about square roots:
- Every positive number has two square roots: one positive (called the principal square root) and one negative. For example, the square roots of 9 are 3 and -3.
- The square root of 0 is 0.
- Negative numbers do not have real square roots because no real number squared gives a negative result.
To simplify a square root, follow these steps:
- Factor the number: Break down the number inside the square root into its prime factors. For example, for \(\sqrt{72}\), we factor it as \(72 = 2^3 \times 3^2\).
- Pair the factors: Identify pairs of prime factors. For \(\sqrt{72}\), the pairs are \((2 \times 2) \times (3 \times 3) \times 2\).
- Simplify: Take one number from each pair out of the square root. For \(\sqrt{72}\), we get \(2 \times 3 \times \sqrt{2} = 6\sqrt{2}\).
Let's look at an example:
| Example | Simplification |
| \(\sqrt{50}\) |
|
| \(\sqrt{18}\) |
|
Understanding these basics will help in simplifying more complex square roots and working with radicals in various mathematical contexts.
Prime Factorization Method for Simplifying Square Roots
The prime factorization method is a systematic way to simplify square roots by breaking down the number under the square root (the radicand) into its prime factors. Here is a step-by-step guide to simplifying square roots using this method:
- Start by finding the prime factors of the radicand. Prime factors are the prime numbers that multiply together to give the original number. For example, to find the prime factors of 72, you would break it down as follows:
- 72 = 2 × 36
- 36 = 2 × 18
- 18 = 2 × 9
- 9 = 3 × 3
- Group the prime factors into pairs. Each pair of prime factors can be taken out of the square root as a single factor. For example:
- \(\sqrt{72} = \sqrt{2 \times 2 \times 2 \times 3 \times 3}\)
- Group the pairs: \(\sqrt{(2 \times 2) \times (3 \times 3) \times 2}\)
- Take out each pair as a single factor: \(2 \times 3 \times \sqrt{2}\)
- If there are any prime factors left unpaired inside the square root, they remain inside the square root. Using the previous example, the unpaired 2 remains inside as \(\sqrt{2}\).
By following these steps, you can simplify any square root using the prime factorization method. This method is particularly useful for large numbers or numbers that have a clear set of prime factors.
Extracting Perfect Squares from the Radicand
Simplifying square roots involves extracting perfect square factors from the radicand. This method reduces the radicand to its simplest form, making it easier to handle in mathematical operations. Follow these steps to extract perfect squares from the radicand:
-
Identify the largest perfect square factor of the radicand. A perfect square is a number like 1, 4, 9, 16, 25, etc., which can be expressed as the square of an integer.
-
Express the radicand as a product of the perfect square factor and another factor.
For example, to simplify \(\sqrt{72}\), identify the largest perfect square factor, which is 36. Thus, \(\sqrt{72} = \sqrt{36 \times 2}\).
-
Rewrite the expression as the product of two square roots.
\(\sqrt{72} = \sqrt{36} \times \sqrt{2}\)
-
Simplify the square root of the perfect square factor.
\(\sqrt{36} = 6\), so \(\sqrt{72} = 6 \times \sqrt{2}\)
-
If the remaining factor is not a perfect square, it stays under the square root symbol.
The final simplified form of \(\sqrt{72}\) is \(6\sqrt{2}\).
Here's another example for clarity:
-
Simplify \(\sqrt{200}\):
-
Identify the largest perfect square factor of 200, which is 100.
-
Express 200 as a product of 100 and 2: \(200 = 100 \times 2\).
-
Rewrite as a product of square roots: \(\sqrt{200} = \sqrt{100} \times \sqrt{2}\).
-
Simplify the perfect square root: \(\sqrt{100} = 10\).
-
The simplified form is \(10\sqrt{2}\).
This method can also be applied to radicands containing variables. Consider \(\sqrt{48x^4y^2}\):
- Identify the perfect square factors for both numerical and variable parts: \(48 = 16 \times 3\), \(x^4\) and \(y^2\) are perfect squares.
- Rewrite as the product of square roots: \(\sqrt{48x^4y^2} = \sqrt{16 \times 3 \times x^4 \times y^2}\).
- Simplify each square root: \(\sqrt{16} = 4\), \(\sqrt{x^4} = x^2\), \(\sqrt{y^2} = y\).
- The simplified form is \(4x^2y\sqrt{3}\).
By extracting perfect squares from the radicand, you simplify square roots, making calculations more manageable and understandable.

Step-by-Step Process to Simplify Square Roots
To simplify square roots, follow these steps:
- Identify the radicand (the number under the square root symbol).
- Factorize the radicand into its prime factors.
- Extract any perfect square factors from under the square root.
- Multiply the square root of the perfect square factors by any remaining factors left under the square root.
- If applicable, simplify further by combining like terms or reducing fractions.
For example, to simplify \( \sqrt{48} \):
| \( \sqrt{48} \) | Original expression |
| \( \sqrt{16 \times 3} \) | Factorize \( 48 \) into \( 16 \times 3 \) |
| \( \sqrt{16} \times \sqrt{3} \) | Extract perfect square \( 16 \) and simplify |
| \( 4 \sqrt{3} \) | Multiply and simplify |
Following these steps will help simplify square roots efficiently.
Special Cases in Simplifying Square Roots
Simplifying square roots can sometimes involve special cases that require different approaches. Below are some common special cases and how to handle them:
-
Square Roots of Negative Numbers: When dealing with the square root of a negative number, the result involves imaginary numbers.
- Example: \(\sqrt{-9}\) is simplified as \(3i\), where \(i\) is the imaginary unit (\(i = \sqrt{-1}\)).
-
Square Roots of Fractions: Use the quotient property to simplify the square root of a fraction.
- Example: \(\sqrt{\frac{25}{16}} = \frac{\sqrt{25}}{\sqrt{16}} = \frac{5}{4}\)
-
Square Roots with Variables: When variables are involved, simplify by factoring out perfect squares.
- Example: \(\sqrt{x^4y^2} = x^2y\)
-
Multiplying Square Roots: Use the property \(\sqrt{a} \cdot \sqrt{b} = \sqrt{ab}\).
- Example: \(\sqrt{2} \cdot \sqrt{8} = \sqrt{16} = 4\)
-
Adding and Subtracting Square Roots: Combine like terms only if the radicands are the same.
- Example: \(2\sqrt{3} + 3\sqrt{3} = 5\sqrt{3}\)
- Non-example: \(\sqrt{2} + \sqrt{3}\) cannot be simplified further.
-
Square Roots of Large Numbers: Factor the number into primes and extract the perfect squares.
- Example: \(\sqrt{72} = \sqrt{36 \times 2} = 6\sqrt{2}\)
-
Square Roots of Products: Simplify each square root separately, then multiply.
- Example: \(\sqrt{50 \cdot 18} = \sqrt{900} = 30\)
Understanding these special cases can help in efficiently simplifying square roots in various mathematical problems.
Working with Square Roots of Perfect Squares
Perfect squares are numbers that are the square of an integer. When dealing with the square roots of perfect squares, the process is straightforward because the result is always an integer.
Here are some steps and examples to help you understand:
- Identify the perfect square.
- Take the square root of the perfect square.
Let's look at some examples:
| Perfect Square | Square Root |
|---|---|
| \(4\) | \(\sqrt{4} = 2\) |
| \(9\) | \(\sqrt{9} = 3\) |
| \(16\) | \(\sqrt{16} = 4\) |
| \(25\) | \(\sqrt{25} = 5\) |
Here is the general rule for any perfect square \(n^2\):
\[
\sqrt{n^2} = n
\]
For example:
- Consider the perfect square \(36\).
- Recognize that \(36 = 6^2\).
- Thus, \(\sqrt{36} = 6\).
Another example:
- Consider the perfect square \(49\).
- Recognize that \(49 = 7^2\).
- Thus, \(\sqrt{49} = 7\).
Working with perfect squares simplifies many mathematical operations, as it allows us to remove the radical and work with integers. This is particularly useful in algebra and other areas of mathematics where simplifying expressions is crucial.
Understanding and recognizing perfect squares can also help in estimating and simplifying more complex square root problems that are not perfect squares.
Simplifying Square Roots of Non-Perfect Squares
Simplifying the square roots of non-perfect squares involves breaking down the number inside the radical into its prime factors and identifying the largest perfect square factor. Here is a step-by-step guide:
Steps to Simplify:
- List all factors of the number inside the radical.
- Identify the largest perfect square factor.
- Rewrite the radical as a product of two radicals: one with the perfect square factor and one with the remaining factor.
- Simplify the radical containing the perfect square.
- Multiply the simplified radical by the remaining radical.
Example 1: Simplify \(\sqrt{18}\)
- Factors of 18: 1, 2, 3, 6, 9, 18
- The largest perfect square factor is 9.
- Rewrite: \(\sqrt{18} = \sqrt{9 \times 2} = \sqrt{9} \times \sqrt{2}\)
- Simplify: \(\sqrt{9} = 3\)
- Result: \(\sqrt{18} = 3\sqrt{2}\)
Example 2: Simplify \(\sqrt{50}\)
- Factors of 50: 1, 2, 5, 10, 25, 50
- The largest perfect square factor is 25.
- Rewrite: \(\sqrt{50} = \sqrt{25 \times 2} = \sqrt{25} \times \sqrt{2}\)
- Simplify: \(\sqrt{25} = 5\)
- Result: \(\sqrt{50} = 5\sqrt{2}\)
Example 3: Simplify \(\sqrt{72}\)
- Factors of 72: 1, 2, 3, 4, 6, 8, 9, 12, 18, 24, 36, 72
- The largest perfect square factor is 36.
- Rewrite: \(\sqrt{72} = \sqrt{36 \times 2} = \sqrt{36} \times \sqrt{2}\)
- Simplify: \(\sqrt{36} = 6\)
- Result: \(\sqrt{72} = 6\sqrt{2}\)
These steps can be applied to any non-perfect square to simplify the square root, making it easier to work with in further calculations.

Handling Square Roots of Fractions
When dealing with the square roots of fractions, the key is to simplify the fraction first if possible, and then apply the square root to both the numerator and the denominator. Here is a step-by-step guide to handling square roots of fractions:
-
Simplify the Fraction: If the fraction can be simplified, do that first. For example, consider the fraction \(\frac{18}{50}\):
\[
\frac{18}{50} = \frac{9}{25}
\] -
Apply the Square Root to the Simplified Fraction: Now apply the square root to both the numerator and the denominator separately:
\[
\sqrt{\frac{9}{25}} = \frac{\sqrt{9}}{\sqrt{25}} = \frac{3}{5}
\] -
Handling Mixed Numbers: Convert any mixed numbers to improper fractions before applying the square root. For example, consider \(1\frac{13}{36}\):
\[
\sqrt{1\frac{13}{36}} = \sqrt{\frac{49}{36}} = \frac{\sqrt{49}}{\sqrt{36}} = \frac{7}{6} = 1\frac{1}{6}
\] -
Rationalizing the Denominator: If you have a fraction with a square root in the denominator, you can rationalize it by multiplying both the numerator and the denominator by the square root in the denominator. For example, consider \(\frac{5}{\sqrt{2}}\):
\[
\frac{5}{\sqrt{2}} \times \frac{\sqrt{2}}{\sqrt{2}} = \frac{5\sqrt{2}}{2}
\]
Here are a few more examples to illustrate these steps:
| Example | Solution |
|---|---|
| \(\sqrt{\frac{25}{36}}\) | \[ \frac{\sqrt{25}}{\sqrt{36}} = \frac{5}{6} \] |
| \(\sqrt{\frac{18}{32}}\) | \[ \frac{\sqrt{\frac{9}{16}}} = \frac{\sqrt{9}}{\sqrt{16}} = \frac{3}{4} \] |
| \(\sqrt{1\frac{11}{25}}\) | \[ \sqrt{\frac{36}{25}} = \frac{\sqrt{36}}{\sqrt{25}} = \frac{6}{5} = 1\frac{1}{5} \] |
By following these steps, you can effectively handle square roots of fractions and simplify them in a systematic manner.
Simplifying Square Roots with Variables
When simplifying square roots that contain variables, we follow a process similar to simplifying square roots of numbers. The goal is to factor the expression under the square root into perfect squares, which can then be simplified.
Here is a step-by-step guide:
- Identify and separate the coefficients and variables inside the radical.
- Factor the variables into pairs of identical factors.
- Simplify the pairs into their respective variables outside the radical.
Let's look at some examples:
Example 1
Simplify \(\sqrt{9x^6}\).
- Factor the expression under the radical: \(\sqrt{9 \cdot x^6}\).
- Rewrite the factors as perfect squares: \(\sqrt{3^2 \cdot (x^3)^2}\).
- Separate the radicals: \(\sqrt{3^2} \cdot \sqrt{(x^3)^2}\).
- Simplify each radical: \(3 \cdot x^3\).
Thus, \(\sqrt{9x^6} = 3x^3\).
Example 2
Simplify \(\sqrt{50x^4y^2}\).
- Factor the expression under the radical: \(\sqrt{50 \cdot x^4 \cdot y^2}\).
- Rewrite the coefficients and variables as perfect squares: \(\sqrt{25 \cdot 2 \cdot (x^2)^2 \cdot y^2}\).
- Separate the radicals: \(\sqrt{25} \cdot \sqrt{2} \cdot \sqrt{(x^2)^2} \cdot \sqrt{y^2}\).
- Simplify each radical: \(5 \cdot x^2 \cdot y \cdot \sqrt{2}\).
Thus, \(\sqrt{50x^4y^2} = 5x^2y\sqrt{2}\).
Example 3
Simplify \(\sqrt{72a^3b^5}\).
- Factor the expression under the radical: \(\sqrt{72 \cdot a^3 \cdot b^5}\).
- Rewrite the coefficients and variables as perfect squares: \(\sqrt{36 \cdot 2 \cdot a^2 \cdot a \cdot b^4 \cdot b}\).
- Separate the radicals: \(\sqrt{36} \cdot \sqrt{2} \cdot \sqrt{a^2} \cdot \sqrt{a} \cdot \sqrt{b^4} \cdot \sqrt{b}\).
- Simplify each radical: \(6 \cdot a \cdot b^2 \cdot \sqrt{2ab}\).
Thus, \(\sqrt{72a^3b^5} = 6ab^2\sqrt{2ab}\).
By following these steps, you can simplify any square root expression that contains variables, ensuring each part is simplified to its most basic form.
Practice Problems and Examples
Practicing simplifying square roots helps solidify the concepts. Here are some practice problems and examples to work through:
Example 1: Simplifying a Basic Square Root
Simplify \( \sqrt{50} \).
- Identify the largest perfect square factor of 50, which is 25.
- Rewrite 50 as \( 25 \times 2 \).
- Use the property \( \sqrt{a \times b} = \sqrt{a} \times \sqrt{b} \):
- \( \sqrt{50} = \sqrt{25 \times 2} = \sqrt{25} \times \sqrt{2} \).
- Simplify \( \sqrt{25} \) to 5:
- Final answer: \( 5\sqrt{2} \).
Example 2: Simplifying a Square Root with Variables
Simplify \( \sqrt{18x^2} \).
- Identify the largest perfect square factor of 18, which is 9.
- Rewrite 18 as \( 9 \times 2 \).
- Use the property \( \sqrt{a \times b} = \sqrt{a} \times \sqrt{b} \):
- \( \sqrt{18x^2} = \sqrt{9 \times 2 \times x^2} = \sqrt{9} \times \sqrt{2} \times \sqrt{x^2} \).
- Simplify \( \sqrt{9} \) to 3 and \( \sqrt{x^2} \) to \( x \):
- Final answer: \( 3x\sqrt{2} \).
Example 3: Simplifying the Square Root of a Fraction
Simplify \( \sqrt{\frac{49}{4}} \).
- Use the property \( \sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}} \):
- \( \sqrt{\frac{49}{4}} = \frac{\sqrt{49}}{\sqrt{4}} \).
- Simplify \( \sqrt{49} \) to 7 and \( \sqrt{4} \) to 2:
- Final answer: \( \frac{7}{2} \) or 3.5.
Practice Problems
- Simplify \( \sqrt{75} \).
- Simplify \( \sqrt{32} \).
- Simplify \( \sqrt{45x^4} \).
- Simplify \( \sqrt{8y^6} \).
- Simplify \( \sqrt{\frac{81}{16}} \).
Use the steps outlined in the examples to solve these problems. Check your answers with a calculator or algebra software if needed.
Common Mistakes to Avoid
When simplifying square roots, it's crucial to avoid common mistakes that can lead to incorrect results. Here are some key points to keep in mind:
- Forgetting to check for perfect squares: Always identify perfect squares within the radicand before simplifying.
- Rushing through the process: Take your time and simplify step by step to avoid errors.
- Ignoring prime factorization: Breaking down the radicand into its prime factors helps in identifying perfect square factors.
- Mistaking addition for multiplication: Remember that \(\sqrt{a \cdot b} = \sqrt{a} \cdot \sqrt{b}\), but \(\sqrt{a + b} \neq \sqrt{a} + \sqrt{b}\).
- Incorrectly handling variables: Apply exponent rules correctly when variables are involved, ensuring that only even exponents can be taken out of the square root.
- Failing to rationalize the denominator: Ensure that the denominator of a fraction does not contain a radical by multiplying by the conjugate if necessary.
- Combining unlike terms: Only like radicals can be combined. For example, \(\sqrt{2} + \sqrt{3}\) cannot be simplified further.
By being mindful of these mistakes, you can improve your accuracy in simplifying square roots and enhance your overall mathematical skills.

Advanced Techniques for Simplifying Square Roots
Simplifying square roots can become more sophisticated when dealing with complex expressions. Here, we will explore some advanced techniques to handle such cases efficiently.
1. Using Rational Exponents
Expressing square roots as rational exponents can simplify the manipulation of these expressions. For example:
\(\sqrt{x} = x^{\frac{1}{2}}\)
This method is particularly useful when multiplying and dividing radicals:
- \(\sqrt{x} \cdot \sqrt{y} = x^{\frac{1}{2}} \cdot y^{\frac{1}{2}} = (xy)^{\frac{1}{2}} = \sqrt{xy}\)
- \(\frac{\sqrt{x}}{\sqrt{y}} = \frac{x^{\frac{1}{2}}}{y^{\frac{1}{2}}} = \left(\frac{x}{y}\right)^{\frac{1}{2}} = \sqrt{\frac{x}{y}}\)
2. Prime Factorization
Prime factorization is a powerful method to simplify square roots, especially when the radicand has multiple factors:
For example, simplify \(\sqrt{180}\):
- Find the prime factors: \(180 = 2^2 \times 3^2 \times 5\)
- Group the pairs of prime factors: \(\sqrt{2^2 \times 3^2 \times 5} = 2 \cdot 3 \cdot \sqrt{5}\)
- Simplify: \(\sqrt{180} = 6\sqrt{5}\)
3. Rationalizing the Denominator
When a radical appears in the denominator, rationalizing is necessary to simplify the expression:
For example, simplify \(\frac{1}{\sqrt{2}}\):
- Multiply numerator and denominator by \(\sqrt{2}\): \(\frac{1}{\sqrt{2}} \cdot \frac{\sqrt{2}}{\sqrt{2}} = \frac{\sqrt{2}}{2}\)
For more complex denominators, use the conjugate:
For example, simplify \(\frac{1}{1+\sqrt{3}}\):
- Multiply by the conjugate: \(\frac{1}{1+\sqrt{3}} \cdot \frac{1-\sqrt{3}}{1-\sqrt{3}} = \frac{1-\sqrt{3}}{1-3} = \frac{\sqrt{3}-1}{2}\)
4. Combining Like Terms
Combining like terms is essential when simplifying expressions with multiple radicals:
- For example: \(2\sqrt{3} + 4\sqrt{3} = 6\sqrt{3}\)
- For variables: \(\sqrt{x^2 \cdot y} = x\sqrt{y}\)
5. Advanced Variable Simplification
Handling variables under the radical involves treating them similarly to numerical factors:
- For example: \(\sqrt{a^4 b^2} = a^2 b\)
These advanced techniques not only simplify the process but also enhance the ability to handle more complex algebraic expressions involving square roots.
Using Technology to Simplify Square Roots
Technology offers various tools to simplify square roots efficiently. Here, we'll explore some of the most popular methods and devices:
1. Scientific Calculators
Most scientific calculators have a square root function that allows for quick simplification:
- Turn on the calculator and press the square root button (√).
- Enter the number you wish to simplify.
- Press the equals button (=) to get the simplified square root.
For example, to find the square root of 25, you would enter √25=5.
2. Graphing Calculators
Graphing calculators like the TI-83 or TI-84 offer more advanced features:
- Press the square root button (√).
- Input the number or expression inside the parentheses.
- Press enter to get the result.
These calculators also allow you to simplify expressions with variables, such as √(4x^2) = 2x.
3. Online Calculators
Numerous online calculators can simplify square roots quickly and accurately. Websites like Symbolab and WolframAlpha provide step-by-step solutions:
- Go to the calculator's website.
- Enter the square root expression in the provided input box.
- Click the 'Simplify' or 'Calculate' button to see the step-by-step solution.
4. Software Tools
Software like Microsoft Excel can also be used to simplify square roots:
- Open Excel and select a cell.
- Type
=SQRT(number)in the cell and press Enter. - For example,
=SQRT(25)will return 5.
5. Mobile Apps
There are various mobile apps available for both iOS and Android that can simplify square roots. Apps like Photomath and Mathway allow you to take a picture of the expression, and they provide a step-by-step simplification:
- Download and install the app from your app store.
- Open the app and use the camera feature to capture the square root expression.
- The app will process the image and provide the simplified result.
6. Online Learning Resources
Websites like Khan Academy and Coursera offer tutorials and interactive exercises to practice simplifying square roots using technology. These resources help reinforce your understanding and improve your skills:
- Visit the educational website.
- Search for courses or tutorials on simplifying square roots.
- Follow the interactive exercises and tutorials to practice.
Using these technological tools can significantly enhance your efficiency and accuracy when simplifying square roots, making complex calculations more manageable and accessible.
Applications of Simplifying Square Roots in Real Life
Simplifying square roots is not just an academic exercise; it has numerous practical applications across various fields. Here are some real-life examples where simplifying square roots is essential:
1. Engineering
In engineering, simplified square roots are crucial for precise measurements and calculations. For instance, they are used in determining the natural frequencies of structures, which helps predict how buildings and bridges will respond to different forces, such as wind or traffic loads.
2. Finance
Square roots play a significant role in finance, particularly in calculating stock market volatility. Investors use the square root of the variance of stock returns to assess the risk associated with investments, aiding in making informed financial decisions.
3. Science
In scientific calculations, square roots are used to determine various physical properties. For example, they help calculate the intensity of sound waves, the velocity of moving objects, and the absorption of radiation by materials, aiding scientists in understanding and developing new technologies.
4. Statistics
Statisticians use square roots to compute standard deviation, a measure of data dispersion around the mean. This is essential for analyzing data sets and drawing accurate conclusions in research and surveys.
5. Geometry
Square roots are fundamental in geometry for solving problems involving right triangles and other polygons. The Pythagorean theorem, which uses square roots, helps calculate the lengths of sides in triangles, essential for various architectural and design tasks.
6. Computer Science
In computer science, square roots are used in algorithms for encryption, image processing, and game physics. For example, encryption algorithms utilize square roots to generate secure keys for data protection.
7. Navigation
Pilots and navigators use square roots to calculate distances between points on a map or globe. This helps in plotting accurate courses and ensuring safe and efficient travel routes.
8. Electrical Engineering
Electrical engineers use square roots to determine power, voltage, and current in circuits. These calculations are vital for designing and developing electrical systems, such as power grids and electronic devices.
9. Photography
The f-number of a camera lens, which controls the amount of light entering the camera, is related to the square root of the lens's area. Understanding this relationship helps photographers adjust their settings to capture the perfect shot.
10. Telecommunication
In telecommunication, signal strength decreases with the square of the distance from the transmitter. Understanding this inverse square law is crucial for optimizing signal transmission and reception in wireless communication networks.
By mastering the simplification of square roots, professionals in these fields can perform critical calculations more efficiently, leading to advancements and innovations in their respective areas.
Frequently Asked Questions
Below are some frequently asked questions about simplifying square roots:
What is a square root?
A square root of a number is a value that, when multiplied by itself, gives the original number. It is represented by the symbol \( \sqrt{} \). For example, \( \sqrt{16} = 4 \) because \( 4 \times 4 = 16 \).
How do you simplify square roots?
To simplify a square root, you need to factor the number inside the radical sign into its prime factors and then extract the square roots of any perfect squares. For example, to simplify \( \sqrt{72} \):
- Factor 72 into its prime factors: \( 72 = 2^3 \times 3^2 \).
- Extract the square roots of the perfect squares: \( \sqrt{72} = \sqrt{2^3 \times 3^2} = \sqrt{(2^2 \times 3^2) \times 2} = 6\sqrt{2} \).
What are perfect squares?
Perfect squares are numbers that are the square of an integer. Examples include 1, 4, 9, 16, 25, etc. These numbers can be factored into pairs of the same number, which can be extracted from under the radical sign.
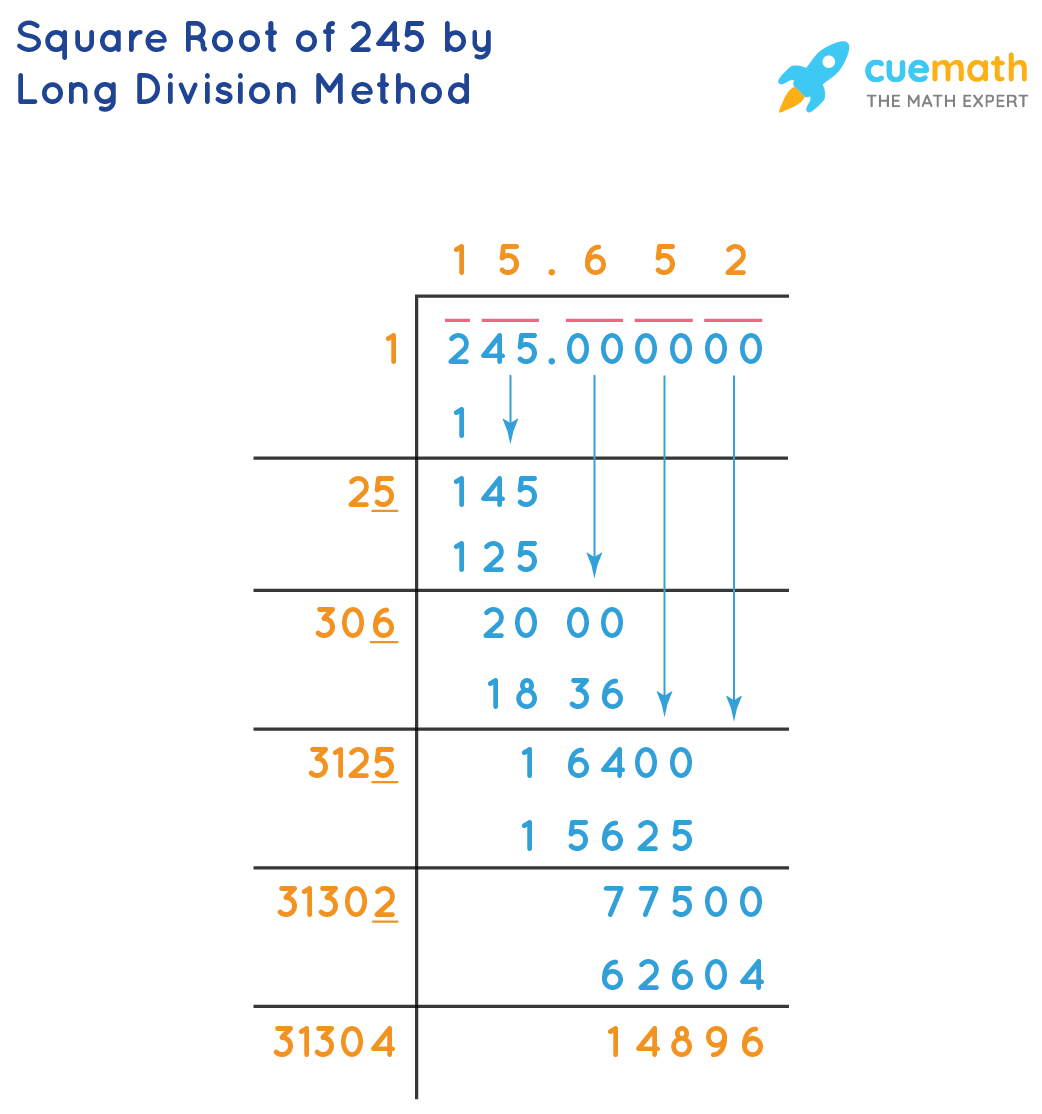
How can I find square roots without a calculator?
You can find square roots without a calculator by using methods such as prime factorization or the long division method. These methods involve breaking down the number into simpler components and solving step by step.
What is the square root of a negative number?
The square root of a negative number is not a real number but an imaginary number. It involves the imaginary unit \( i \), where \( i = \sqrt{-1} \). For example, \( \sqrt{-4} = 2i \).
Is the square root of 2 a rational number?
No, the square root of 2 is an irrational number. It cannot be expressed as a fraction of two integers. Its decimal representation is non-terminating and non-repeating, approximately equal to 1.41421356.
What are some common mistakes to avoid when simplifying square roots?
Common mistakes include:
- Forgetting to check for perfect squares before simplifying.
- Rushing through the simplification process without careful factorization.
- Not fully simplifying the radical expression.
Can I simplify square roots with variables?
Yes, square roots with variables can be simplified by applying the same principles. For example, \( \sqrt{18x^2} = \sqrt{9 \times 2 \times x^2} = 3x\sqrt{2} \).
Are there any tools available to help simplify square roots?
Yes, there are many online calculators and apps that can help you simplify square roots quickly and accurately. These tools can be very helpful for checking your work and understanding the process.

Additional Resources and References
Here are some valuable resources and references to help you further understand and master the process of simplifying square roots:
-
Textbooks and Online Lessons
- Comprehensive explanations and examples on simplifying square roots, including special cases and applications in various fields.
- Detailed lesson plans and downloadable resources specifically tailored for Grade 8 students on simplifying square roots.
- Free, high-quality video tutorials and practice exercises on simplifying square roots.
-
Practice and Interactive Tools
- Interactive practice problems and immediate feedback on simplifying square roots, tailored to different grade levels.
- An online calculator that not only provides solutions but also step-by-step explanations for simplifying square roots.
- A powerful calculator and problem solver for algebra, including detailed steps for simplifying square roots.
-
Academic Papers and Research Articles
- Access to scholarly articles and papers on mathematical methods, including the simplification of square roots.
- A network where researchers publish and discuss their work, offering in-depth papers on advanced techniques for simplifying square roots.
-
Educational Institutions and Online Courses
- Online courses from top universities that include modules on algebra and the simplification of square roots.
- Offers courses from institutions like MIT and Harvard, covering algebra and square root simplification.
These resources provide a wealth of information and practice opportunities to enhance your understanding and skills in simplifying square roots. Whether you are a student, educator, or simply looking to brush up on your math skills, these references will be invaluable.
Xem video 'Đơn Giản Hóa Căn Bậc Hai' với Thầy J để học cách đơn giản hóa căn bậc hai một cách dễ dàng và chi tiết. Phù hợp cho học sinh và người yêu thích toán học.
Đơn Giản Hóa Căn Bậc Hai | Toán Học với Thầy J
READ MORE:
Xem video 'Toán Học Vui - Đơn Giản Hóa Căn Bậc Hai' để học cách đơn giản hóa căn bậc hai một cách dễ hiểu và thú vị. Phù hợp cho học sinh và người yêu thích toán học.
Toán Học Vui - Đơn Giản Hóa Căn Bậc Hai