Topic 1/9 squared: When you square 1/9, you get a fascinating result that demonstrates basic principles of fractions and exponents. In this article, we'll explore how to calculate and simplify this expression, along with its significance in various mathematical contexts.
Table of Content
- Understanding 1/9 Squared
- Introduction
- Understanding Squaring
- Mathematical Calculation of 1/9 Squared
- Step-by-Step Solution
- Fraction and Decimal Forms
- Importance in Algebra
- Applications in Real Life
- Related Mathematical Concepts
- Conclusion
- YOUTUBE: Cách bình phương một số | Ý nghĩa của việc bình phương một số | Lũy thừa | Toán với Thầy J
Understanding 1/9 Squared
To calculate the square of a fraction like \( \frac{1}{9} \), we multiply the fraction by itself:
\( \left(\frac{1}{9}\right)^2 = \frac{1}{9} \times \frac{1}{9} = \frac{1}{81} \)
Fraction Form
In fraction form, the result is:
\( \frac{1}{81} \)
Decimal Form
In decimal form, the result is:
\( 0.0123 \)
Steps to Calculate
- Multiply the numerators: \( 1 \times 1 = 1 \)
- Multiply the denominators: \( 9 \times 9 = 81 \)
- Combine the results: \( \frac{1}{81} \)
Square Root of 1/9
The square root of \( \frac{1}{9} \) is found by taking the square root of both the numerator and the denominator:
\( \sqrt{\frac{1}{9}} = \frac{\sqrt{1}}{\sqrt{9}} = \frac{1}{3} \)
Perfect Squares
Perfect squares are the squares of whole numbers:
- 0² = 0
- 1² = 1
- 2² = 4
- 3² = 9
- 4² = 16
- 5² = 25
- 6² = 36
- 7² = 49
- 8² = 64
- 9² = 81
- 10² = 100
Applications
Understanding how to square fractions is important in various fields of mathematics, including algebra and calculus. It is also useful in solving real-world problems involving ratios and proportions.

READ MORE:
Introduction
The concept of squaring fractions, such as \( \left(\frac{1}{9}\right)^2 \), involves multiplying the fraction by itself. In this case, \( \left(\frac{1}{9}\right)^2 = \frac{1}{81} \). Squaring fractions follows the same mathematical principles as squaring whole numbers but requires careful attention to the properties of fractions and their operations.
Understanding the mathematical steps to square a fraction involves recognizing that both the numerator and the denominator are squared separately. For \( \left(\frac{a}{b}\right)^2 \), this becomes \( \frac{a^2}{b^2} \). In our specific example, this means:
- Square the numerator: \(1^2 = 1\)
- Square the denominator: \(9^2 = 81\)
Thus, \( \left(\frac{1}{9}\right)^2 = \frac{1}{81} \).
This process is fundamental in various mathematical applications, including algebra and calculus, where understanding the properties of squared terms can simplify complex equations and expressions.
Understanding Squaring
Squaring a number means multiplying the number by itself. It is denoted mathematically as \( x^2 \), where \( x \) is the number being squared. For instance, squaring 2 (written as \( 2^2 \)) results in 4 because \( 2 \times 2 = 4 \).
Here are key points to understand about squaring numbers:
- Positive and Negative Numbers: The square of both positive and negative numbers is always positive because multiplying two negative numbers results in a positive number.
- Perfect Squares: These are squares of integers, such as \( 1, 4, 9, 16, \) etc. For example, 9 is a perfect square because it is \( 3^2 \) (3 multiplied by itself).
- Visualization: A useful way to visualize squaring is by imagining a square shape where each side length is the number being squared. For example, a square with side length 2 has an area of \( 2^2 = 4 \).
- Applications: Squaring is used in various mathematical and real-world applications, including calculating areas, solving algebraic equations, and understanding exponential growth.
To summarize, squaring is a fundamental operation in mathematics with wide-ranging applications. Understanding how to square numbers and the properties of squared numbers is essential for further mathematical learning and problem-solving.
Mathematical Calculation of 1/9 Squared
To understand the process of squaring a fraction like , follow these steps:
- Identify the fraction: .
- Square both the numerator and the denominator separately:
- Square the numerator:
- Square the denominator:
- Combine the squared numerator and denominator into a new fraction: .
- Express the final result: or in decimal form as .
These steps show that squaring the fraction
results in
.
Step-by-Step Solution
To find \( \left(\frac{1}{9}\right)^2 \), follow these steps:
- Start with the expression \( \left(\frac{1}{9}\right)^2 \).
- Apply the square of a fraction rule: \( \left(\frac{a}{b}\right)^2 = \frac{a^2}{b^2} \).
- Substitute \( a = 1 \) and \( b = 9 \) into the formula.
- Calculate \( 1^2 = 1 \) and \( 9^2 = 81 \).
- Thus, \( \left(\frac{1}{9}\right)^2 = \frac{1^2}{9^2} = \frac{1}{81} \).
Therefore, \( \left(\frac{1}{9}\right)^2 = \frac{1}{81} \).
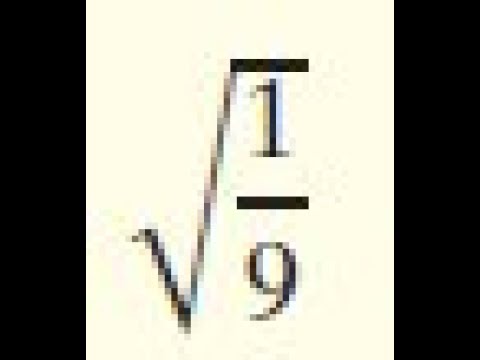
Fraction and Decimal Forms
The fraction \( \left(\frac{1}{9}\right)^2 \) can be expressed as:
- In fractional form: \( \left(\frac{1}{9}\right)^2 = \frac{1}{81} \).
- In decimal form: To convert \( \frac{1}{81} \) to a decimal, perform the division:
| Divide 1 by 81: | \( 1 \div 81 = 0.012345679 \ldots \) |
Therefore, \( \left(\frac{1}{9}\right)^2 \) in decimal form is approximately \( 0.0123 \).
Importance in Algebra
The calculation \( \left(\frac{1}{9}\right)^2 \) holds significance in algebra due to several reasons:
- It demonstrates the application of exponentiation rules in algebraic expressions.
- It illustrates the concept of squaring a fraction and how to handle powers of fractions.
- Understanding \( \left(\frac{1}{9}\right)^2 \) helps in grasping the relationship between fractions and their squares.
- It provides a basis for understanding higher-level algebraic manipulations involving exponents and fractions.
Applications in Real Life
The concept of \( \left(\frac{1}{9}\right)^2 \) finds practical applications in various real-life scenarios:
- Calculating areas and volumes where fractions of measurements are involved, such as in architecture and engineering.
- Understanding probabilities and statistical distributions where fractions play a crucial role.
- In finance, especially when dealing with interest rates and percentages that involve fractional calculations.
- In physics and chemistry, particularly in calculations involving concentrations, dilutions, and specific measurements.
- In computer science and information technology, for precision calculations and algorithms involving fractions.
Related Mathematical Concepts
Several related mathematical concepts are associated with \( \left(\frac{1}{9}\right)^2 \):
- Exponentiation: Understanding the general rules and properties of raising fractions to powers.
- Fractional Powers: Exploring how fractional exponents behave and are manipulated in algebraic expressions.
- Decimal Conversion: Converting fractions to decimals and understanding the implications in calculations.
- Inverse Operations: Relating squaring a fraction to finding its reciprocal and the implications in solving equations.
- Geometric Series: Connecting fractional powers to series and sequences in mathematics.
Conclusion
In conclusion, exploring \( \left(\frac{1}{9}\right)^2 \) enhances mathematical understanding in various aspects:
- It reinforces the fundamental rules of exponentiation and fractional powers.
- Understanding its calculation in both fraction and decimal forms aids in practical applications.
- Its relevance in algebraic manipulations underscores its importance in higher-level mathematics.
- Real-life applications demonstrate its utility across diverse fields such as engineering, finance, and science.
- Related mathematical concepts provide a broader context for its application and manipulation.
READ MORE:
Cách bình phương một số | Ý nghĩa của việc bình phương một số | Lũy thừa | Toán với Thầy J
Cách bình phương một số | Ý nghĩa của việc bình phương một số | Lũy thừa | Toán với Thầy J