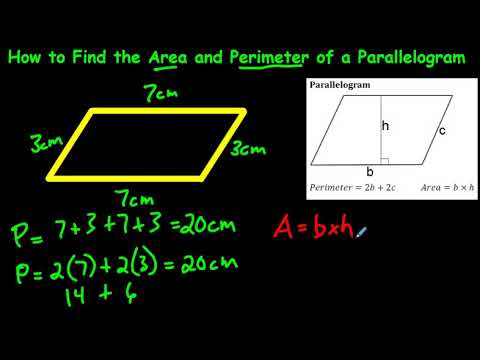
Topic formula for perimeter of a semicircle: The formula for the perimeter of a semicircle is essential for students and professionals in mathematics and engineering. This guide simplifies the concept, providing a clear understanding of how to calculate the perimeter with practical examples. Learn the steps and apply the formula confidently in various applications.
Table of Content
- Perimeter of a Semicircle
- Introduction to the Semicircle
- Understanding the Perimeter
- Derivation of the Perimeter Formula
- Mathematical Representation
- Components of the Formula
- Step-by-Step Calculation
- Example Calculations
- Applications of the Perimeter Formula
- Common Mistakes to Avoid
- Advanced Topics Related to Semicircles
- Summary and Conclusion
- YOUTUBE:
Perimeter of a Semicircle
The perimeter of a semicircle includes the length of the curved edge (half of the circumference of a full circle) and the diameter of the circle. The formula to calculate the perimeter \( P \) of a semicircle is given by:
\[ P = \pi r + 2r \]
Where:
- \( r \) is the radius of the semicircle
- \( \pi \) is a constant approximately equal to 3.14159
The term \( \pi r \) represents the curved edge of the semicircle, and \( 2r \) represents the diameter of the circle. The combined length of these two components gives the total perimeter of the semicircle.
Example Calculation
For example, if the radius of a semicircle is 5 units, the perimeter can be calculated as follows:
\[ P = \pi \times 5 + 2 \times 5 \]
\[ P = 5\pi + 10 \]
Thus, the perimeter of the semicircle is \( 5\pi + 10 \) units.

READ MORE:
Introduction to the Semicircle
A semicircle is a two-dimensional geometric shape that represents half of a full circle. It is formed by cutting a whole circle along its diameter, resulting in a shape with a curved edge and a straight edge.
Key characteristics of a semicircle include:
- Radius (\(r\)): The distance from the center of the semicircle to any point on the curved edge.
- Diameter (\(d\)): The straight edge of the semicircle, which is twice the radius (\(d = 2r\)).
- Curved Edge: This is half of the circumference of the original circle.
In mathematical terms, the perimeter of a semicircle consists of the curved edge and the diameter. Understanding how to calculate the perimeter is crucial for various practical applications, such as engineering, architecture, and design.
To derive the formula for the perimeter of a semicircle, consider the following steps:
- Calculate the circumference of a full circle using the formula \( C = 2\pi r \).
- Divide the circumference by 2 to get the length of the curved edge: \( \frac{C}{2} = \pi r \).
- Add the diameter to the length of the curved edge to obtain the total perimeter: \( P = \pi r + 2r \).
Thus, the formula for the perimeter \( P \) of a semicircle is:
\[ P = \pi r + 2r \]
This formula helps in calculating the boundary length of the semicircle, which is essential for solving various geometric problems.
Understanding the Perimeter
The perimeter of a semicircle is the total distance around its boundary, which includes the curved edge (half of the circumference of the original circle) and the straight edge (the diameter). To fully understand how to calculate the perimeter, it is important to grasp the relationship between the radius, diameter, and circumference of a circle.
Key concepts:
- Radius (\(r\)): The distance from the center of the circle to any point on its boundary.
- Diameter (\(d\)): The straight line passing through the center, connecting two points on the boundary, which is twice the radius (\(d = 2r\)).
- Circumference (\(C\)): The total distance around a full circle, calculated as \( C = 2\pi r \).
The perimeter of a semicircle involves two main parts:
- Curved Edge: This is half the circumference of the original circle. Since the circumference of a full circle is \( 2\pi r \), the curved edge of a semicircle is \( \pi r \).
- Straight Edge (Diameter): This is the diameter of the circle, which is \( 2r \).
To calculate the perimeter \( P \) of a semicircle, we sum these two parts:
\[ P = \pi r + 2r \]
This formula combines the curved portion and the straight line to give the total perimeter of the semicircle.
For a better understanding, consider an example:
If the radius of a semicircle is 4 units:
- Calculate the curved edge: \( \pi r = \pi \times 4 = 4\pi \).
- Calculate the diameter: \( 2r = 2 \times 4 = 8 \).
- Add the two parts to get the perimeter: \( P = 4\pi + 8 \).
Thus, the perimeter of the semicircle is \( 4\pi + 8 \) units.
Understanding this formula is crucial for solving geometric problems involving semicircles and applying it in various real-world scenarios.
Derivation of the Perimeter Formula
Deriving the formula for the perimeter of a semicircle involves understanding the geometric properties of a circle and how they relate to a semicircle. Here is a step-by-step process to derive the formula:
- Understanding the Circle:
The perimeter (circumference) of a full circle is given by the formula:
\[ C = 2\pi r \]
where \( r \) is the radius of the circle.
- Identifying the Semicircle:
A semicircle is half of a full circle. Therefore, the curved edge of a semicircle is half of the circumference of the full circle.
\[ \text{Curved Edge} = \frac{C}{2} = \frac{2\pi r}{2} = \pi r \]
- Adding the Diameter:
In addition to the curved edge, a semicircle includes the diameter of the circle as its straight edge. The diameter \( d \) is twice the radius:
\[ d = 2r \]
- Combining the Parts:
To find the total perimeter \( P \) of the semicircle, we add the length of the curved edge to the diameter:
\[ P = \pi r + 2r \]
This step-by-step derivation shows how the perimeter of a semicircle is composed of half the circumference of the original circle plus the diameter.
For example, if the radius of a semicircle is 6 units:
- Calculate the curved edge: \( \pi r = \pi \times 6 = 6\pi \).
- Calculate the diameter: \( 2r = 2 \times 6 = 12 \).
- Add the two parts to get the perimeter: \( P = 6\pi + 12 \).
Thus, the perimeter of the semicircle is \( 6\pi + 12 \) units.
Understanding this derivation helps in accurately applying the formula for various mathematical and practical applications involving semicircles.
Mathematical Representation
To accurately calculate the perimeter of a semicircle, it's essential to understand its mathematical representation. The formula for the perimeter combines the lengths of the curved edge and the straight edge (diameter).
The perimeter \( P \) of a semicircle with radius \( r \) can be represented as:
\[ P = \pi r + 2r \]
Here’s a detailed breakdown of each component of this formula:
- Curved Edge:
The curved edge of the semicircle is half the circumference of a full circle. The circumference \( C \) of a full circle is calculated using the formula:
\[ C = 2\pi r \]
Since a semicircle is half of a full circle, its curved edge is:
\[ \text{Curved Edge} = \frac{C}{2} = \frac{2\pi r}{2} = \pi r \]
- Diameter:
The straight edge of the semicircle is the diameter of the original circle, which is twice the radius:
\[ d = 2r \]
- Total Perimeter:
Adding the length of the curved edge and the diameter gives the total perimeter of the semicircle:
\[ P = \pi r + 2r \]
This formula encapsulates the entire boundary length of the semicircle.
For better understanding, consider an example with a specific radius:
Suppose the radius of the semicircle is 7 units:
- Calculate the curved edge: \( \pi r = \pi \times 7 = 7\pi \).
- Calculate the diameter: \( 2r = 2 \times 7 = 14 \).
- Add the two parts to get the perimeter: \( P = 7\pi + 14 \).
Thus, the perimeter of the semicircle is \( 7\pi + 14 \) units.
This mathematical representation is crucial for accurately determining the perimeter and applying it to various geometric problems and real-world applications.
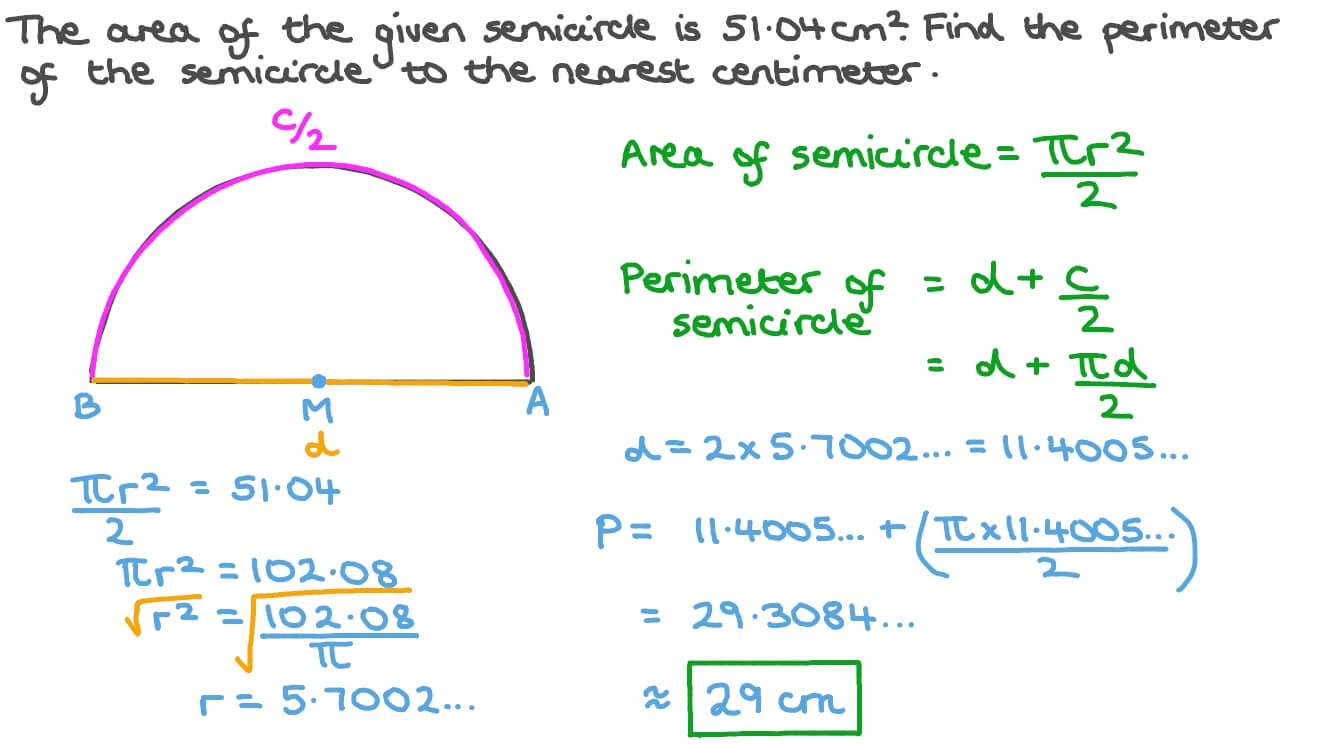
Components of the Formula
The formula for the perimeter of a semicircle is derived by combining the lengths of its curved edge and its straight edge (diameter). Understanding each component of the formula is crucial for accurate calculations. The formula is expressed as:
\[ P = \pi r + 2r \]
Here are the detailed components of this formula:
- Radius (\( r \)):
The radius is the distance from the center of the semicircle to any point on the curved edge. It is a fundamental measure in circular geometry and is used to determine both the curved edge and the diameter.
- Curved Edge (\( \pi r \)):
The curved edge of the semicircle is half the circumference of the full circle. The circumference \( C \) of a full circle is given by:
\[ C = 2\pi r \]
Since the semicircle is half of the full circle, the length of the curved edge is:
\[ \text{Curved Edge} = \frac{C}{2} = \frac{2\pi r}{2} = \pi r \]
- Diameter (\( 2r \)):
The diameter is the straight edge of the semicircle, stretching from one end of the curved edge to the other, passing through the center. It is twice the radius:
\[ d = 2r \]
- Total Perimeter:
By adding the length of the curved edge and the diameter, we obtain the total perimeter of the semicircle:
\[ P = \pi r + 2r \]
To illustrate, consider a semicircle with a radius of 3 units:
- Calculate the curved edge: \( \pi r = \pi \times 3 = 3\pi \).
- Calculate the diameter: \( 2r = 2 \times 3 = 6 \).
- Add the two parts to get the perimeter: \( P = 3\pi + 6 \).
Therefore, the perimeter of the semicircle is \( 3\pi + 6 \) units.
Understanding these components allows for the accurate application of the formula in various mathematical and practical scenarios involving semicircles.
Step-by-Step Calculation
The perimeter of a semicircle is the sum of the straight edge (diameter) and the curved edge (half the circumference of a full circle). Follow these steps to calculate the perimeter of a semicircle:
-
Identify the radius (r) of the semicircle:
The radius is the distance from the center of the semicircle to any point on its curved edge.
Example: Let the radius (r) be 5 cm.
-
Calculate the diameter (d):
The diameter is twice the radius.
Formula: \( d = 2r \)
Example: \( d = 2 \times 5 \, \text{cm} = 10 \, \text{cm} \)
-
Calculate the circumference of the full circle:
The circumference is given by the formula \( 2\pi r \).
Formula: \( C = 2\pi r \)
Example: \( C = 2 \pi \times 5 \, \text{cm} = 10\pi \, \text{cm} \)
-
Calculate the length of the curved edge (half the circumference):
Since the semicircle is half of a full circle, the curved edge is half the circumference.
Formula: \( \text{Curved Edge} = \frac{1}{2} \times 2\pi r = \pi r \)
Example: \( \text{Curved Edge} = \pi \times 5 \, \text{cm} = 5\pi \, \text{cm} \)
-
Add the diameter and the curved edge to get the perimeter:
Formula: \( \text{Perimeter} = d + \pi r \)
Example: \( \text{Perimeter} = 10 \, \text{cm} + 5\pi \, \text{cm} \)
-
Final Calculation:
Combine the values to find the perimeter.
Example: \( \text{Perimeter} = 10 + 5\pi \, \text{cm} \)
Thus, the perimeter of a semicircle with a radius of 5 cm is \( 10 + 5\pi \, \text{cm} \).
Example Calculations
Here are a few example calculations to demonstrate how to find the perimeter of a semicircle:
-
Example 1: Radius = 3 cm
- Identify the radius: \( r = 3 \, \text{cm} \)
- Calculate the diameter: \( d = 2r = 2 \times 3 \, \text{cm} = 6 \, \text{cm} \)
- Calculate the curved edge (half the circumference): \( \text{Curved Edge} = \pi r = \pi \times 3 \, \text{cm} = 3\pi \, \text{cm} \)
- Calculate the perimeter: \( \text{Perimeter} = d + \text{Curved Edge} = 6 \, \text{cm} + 3\pi \, \text{cm} \)
- Final result: \( \text{Perimeter} = 6 + 3\pi \, \text{cm} \)
-
Example 2: Radius = 7 cm
- Identify the radius: \( r = 7 \, \text{cm} \)
- Calculate the diameter: \( d = 2r = 2 \times 7 \, \text{cm} = 14 \, \text{cm} \)
- Calculate the curved edge (half the circumference): \( \text{Curved Edge} = \pi r = \pi \times 7 \, \text{cm} = 7\pi \, \text{cm} \)
- Calculate the perimeter: \( \text{Perimeter} = d + \text{Curved Edge} = 14 \, \text{cm} + 7\pi \, \text{cm} \)
- Final result: \( \text{Perimeter} = 14 + 7\pi \, \text{cm} \)
-
Example 3: Radius = 10 cm
- Identify the radius: \( r = 10 \, \text{cm} \)
- Calculate the diameter: \( d = 2r = 2 \times 10 \, \text{cm} = 20 \, \text{cm} \)
- Calculate the curved edge (half the circumference): \( \text{Curved Edge} = \pi r = \pi \times 10 \, \text{cm} = 10\pi \, \text{cm} \)
- Calculate the perimeter: \( \text{Perimeter} = d + \text{Curved Edge} = 20 \, \text{cm} + 10\pi \, \text{cm} \)
- Final result: \( \text{Perimeter} = 20 + 10\pi \, \text{cm} \)
These examples illustrate the step-by-step process to calculate the perimeter of a semicircle given different radii. Remember, the formula is \( \text{Perimeter} = 2r + \pi r \) or \( \text{Perimeter} = r(2 + \pi) \).
Applications of the Perimeter Formula
The formula for the perimeter of a semicircle, \( P = r(\pi + 2) \), has several practical applications in various fields. Understanding and using this formula can help solve real-world problems effectively. Below are some common applications:
- Architecture and Construction: In designing arches, domes, and other semicircular structures, the perimeter formula helps determine the length of materials needed for construction. This is crucial for planning and budgeting.
- Landscaping: When creating garden paths, borders, or semicircular seating areas, the formula aids in calculating the perimeter, ensuring accurate placement and material usage.
- Manufacturing: In industries where semicircular components are produced, such as pipes or semi-cylindrical tanks, the perimeter is essential for determining the material length required for fabrication.
- Education: Teachers and students use this formula in geometry classes to solve problems related to semicircles, enhancing their understanding of mathematical concepts.
- Art and Design: Artists and designers apply the perimeter formula when creating semicircular frames, patterns, or layouts, ensuring precise and aesthetically pleasing results.
- Sports and Recreation: Designing tracks, playgrounds, or sports equipment that includes semicircular shapes relies on the perimeter formula for accurate measurements and constructions.
- Engineering: In mechanical and civil engineering, the perimeter of semicircular components is crucial for stress analysis, load distribution, and structural integrity assessments.
These applications demonstrate the versatility and importance of understanding the perimeter of a semicircle. Accurate calculations ensure efficiency, cost-effectiveness, and precision in various professional and academic activities.

Common Mistakes to Avoid
Calculating the perimeter of a semicircle is straightforward, but there are several common mistakes to watch out for. Here are some key errors and tips to avoid them:
- Confusing Radius and Diameter:
The radius (\(r\)) is half of the diameter (\(d\)). Ensure you are using the radius when applying the formula \(P = r(\pi + 2)\). If given the diameter, remember to divide by two to find the radius.
- Incorrect Value of Pi:
Using an overly simplified value of \(\pi\) can lead to inaccuracies. For most calculations, use at least \(\pi \approx 3.14\) or use the \(\pi\) button on your calculator for more precision.
- Forgetting the Straight Edge:
The perimeter of a semicircle includes both the curved edge (half the circumference) and the straight edge (diameter). Ensure you add both parts together: \(P = r(\pi + 2)\).
- Mathematical Errors:
Simple arithmetic mistakes, such as incorrect addition or multiplication, can skew results. Double-check your calculations to avoid these errors.
- Unit Inconsistencies:
Ensure all measurements are in the same unit before starting your calculations. Mixing units (e.g., centimeters and meters) will lead to incorrect results.
- Rounding Errors:
Be cautious with rounding intermediate steps. Keep calculations as precise as possible and only round the final result to the desired number of decimal places.
By being mindful of these common mistakes, you can ensure accurate and reliable results when calculating the perimeter of a semicircle.
Advanced Topics Related to Semicircles
The study of semicircles extends beyond basic geometry into various advanced topics and applications. Here are some key advanced topics related to semicircles:
-
Thales' Theorem:
This theorem states that any triangle inscribed in a semicircle with the diameter as one side is a right-angled triangle. This is a foundational concept in geometry that has implications in various geometric constructions and proofs.
-
Parbelos:
Inspired by the arbelos, a parbelos involves segments of parabolas instead of semicircles. This geometric figure is studied for its unique properties and applications in advanced geometric problems.
-
Equation of a Semicircle:
The equation of a semicircle can vary depending on its orientation. For a semicircle centered at the origin with radius \( r \), the equations are:
- Upper Semicircle: \( y = \sqrt{r^2 - x^2} \)
- Lower Semicircle: \( y = -\sqrt{r^2 - x^2} \)
These equations are fundamental in mathematical modeling and computer graphics.
-
Semicircles in Architecture and Engineering:
Semicircles play a significant role in architecture and engineering. Semicircular arches are common in historical and modern structures due to their aesthetic appeal and structural efficiency. Similarly, semicircular designs are used in bridges and tunnels to optimize load distribution.
-
Optics and Reflection:
In optics, the principles of reflection in concave mirrors, which are semicircular in shape, are crucial. Understanding the properties of semicircles helps in designing optical instruments and studying light behavior.
-
Trigonometry and Unit Circle:
The unit circle, a fundamental concept in trigonometry, involves the use of semicircles to define sine and cosine functions. This application is crucial in various fields, including signal processing and engineering.
-
Semicircles in Nature and Design:
Natural phenomena such as rainbows and horizons often appear semicircular. In design, semicircular patterns and motifs are popular in art and architecture for their symmetry and aesthetic appeal.
These advanced topics highlight the versatility and importance of semicircles in various fields, from theoretical mathematics to practical engineering and design.
Summary and Conclusion
In conclusion, the formula for the perimeter of a semicircle is a vital concept in geometry, combining fundamental aspects of circles and linear measurement. The perimeter of a semicircle is given by the formula:
\( P = \pi r + 2r \) or \( P = r(\pi + 2) \)
where \( r \) is the radius of the semicircle. This formula effectively adds the half-circumference of the circle (the curved part) and the diameter (the straight part).
To summarize the key points:
- The perimeter of a semicircle includes the length of the curved arc plus the diameter of the circle.
- The formula \( P = \pi r + 2r \) captures both these components, ensuring accurate calculation of the total boundary length.
- This formula is derived by halving the circumference of a full circle and adding the diameter: \( \frac{1}{2} \times 2\pi r + 2r \).
- Understanding this derivation helps in visualizing why the perimeter includes both the curved and straight segments.
Practical applications of the semicircle perimeter formula are found in various fields such as architecture, engineering, and design, where precise measurements of curved boundaries are necessary.
When using this formula, it is important to ensure the radius is accurately measured and the units are consistent to avoid common mistakes.
In more advanced topics, variations of this formula can be applied to sectors and other partial circles, expanding its utility in solving complex geometrical problems.
In conclusion, mastering the formula for the perimeter of a semicircle equips students and professionals with a fundamental tool for accurate measurement and design in various practical applications.
Cách Tính Chu Vi Nửa Đường Tròn
READ MORE:
Tìm hiểu về công thức tính chu vi của nửa hình tròn qua video này. Video hướng dẫn chi tiết và dễ hiểu.
Chu vi của Nửa Hình Tròn