Topic simplifying radical expressions worksheet: Unlock the secrets of simplifying radical expressions with our comprehensive worksheet. Designed for students of all levels, this worksheet covers key concepts and offers a variety of practice problems to enhance your understanding. Dive in and master the art of simplifying radicals with ease and confidence!
Table of Content
- Understanding Radical Expressions
- Introduction to Radical Expressions
- Understanding Radicals and Radicands
- Perfect Squares and Perfect Cubes
- Basic Rules of Simplifying Radicals
- Methods for Simplifying Radical Expressions
- Simplifying Radicals with Variables
- Adding and Subtracting Radical Expressions
- Multiplying Radical Expressions
- Dividing Radical Expressions
- Rationalizing the Denominator
- Solving Radical Equations
- Using the Distance Formula with Radicals
- Using the Midpoint Formula with Radicals
- Practice Worksheets and Quizzes
- Additional Resources and References
- YOUTUBE: Xem video 'Bài Tập Giản Lược Biểu Thức Chứa Căn' để học cách giản lược biểu thức chứa căn một cách hiệu quả. Video này cung cấp các bài tập và hướng dẫn chi tiết, phù hợp cho người học toán ở mọi trình độ.
Understanding Radical Expressions
Radical expressions involve roots, such as square roots or cube roots. Simplifying these expressions is an essential skill in algebra. Here, we will guide you through the process of simplifying radical expressions with clear explanations and examples.
Key Concepts
- Radicand: The number inside the radical symbol.
- Index: The small number outside the radical symbol indicating the root (e.g., 3 for cube root).
- Perfect Squares: Numbers like 4, 9, 16, which are the square of integers.
Steps to Simplify Radical Expressions
- Identify and factor the radicand into its prime factors.
- Look for pairs of factors (for square roots) or groups (for cube roots) inside the radicand.
- Move each pair or group outside the radical as a single number.
- Multiply the numbers outside the radical together.
- Simplify the expression inside the radical if possible.
Examples
| \(\sqrt{50}\) | Factor 50: \(2 \times 5^2\). Move 5 outside: \(5\sqrt{2}\). |
| \(\sqrt{72}\) | Factor 72: \(2^3 \times 3^2\). Move 6 outside: \(6\sqrt{2}\). |
| \(\sqrt[3]{54}\) | Factor 54: \(2 \times 3^3\). Move 3 outside: \(3\sqrt[3]{2}\). |
Practice Problems
Try simplifying the following radical expressions:
- \(\sqrt{32}\)
- \(\sqrt{45}\)
- \(\sqrt[3]{81}\)
- \(\sqrt[4]{256}\)
Additional Resources
For more practice and detailed worksheets on simplifying radical expressions, consider these resources:
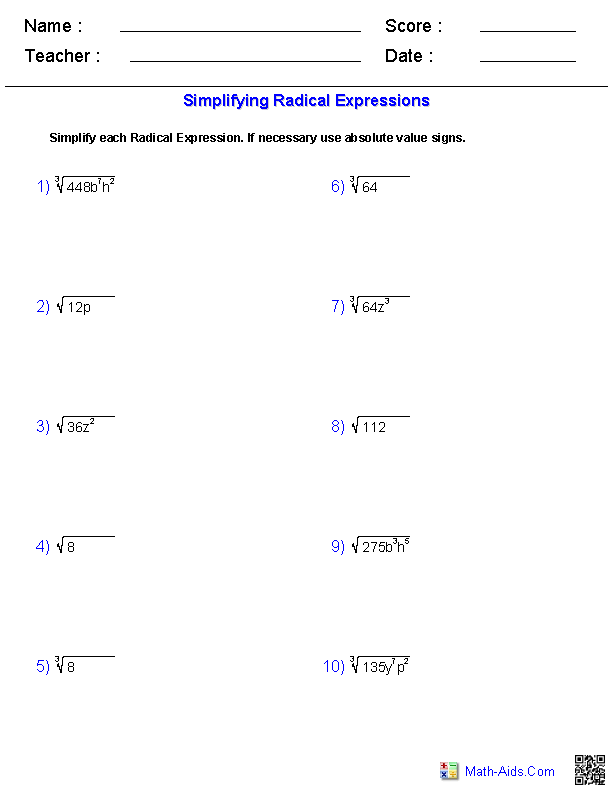
READ MORE:
Introduction to Radical Expressions
Radical expressions are mathematical expressions that include a square root, cube root, or higher-order root symbol. Understanding and simplifying these expressions is essential for solving many algebraic problems. Here, we will introduce the basic concepts and rules for working with radical expressions.
In a radical expression, the number inside the radical symbol is called the radicand, and the number outside is called the index. For example, in the expression , the radicand is 16, and the index is 2, indicating a square root. If the index is 3, it is a cube root, and so on.
To simplify a radical expression, follow these steps:
- Identify and factor the radicand into its prime factors. For example, the prime factors of 18 are 2 and 32 (since 18 = 2 × 3 × 3).
- Look for pairs of factors (for square roots) or groups of three (for cube roots) within the prime factors. For the square root of 18, we have one pair of 3's.
- Move each pair (or group) outside the radical, simplifying the expression. For , we take out a 3, resulting in 3.
Let's look at a specific example to illustrate these steps:
In this example, 50 is factored into 25 and 2. Since 25 is a perfect square (5 × 5), it is taken out of the radical, leaving us with 5.
Practicing these steps with different radicands will help you become proficient in simplifying radical expressions. Use worksheets and practice problems to reinforce your understanding and improve your skills.
Understanding Radicals and Radicands
A radical expression includes a radical symbol (√) and a radicand, which is the number or expression inside the radical symbol. Radicals are used to denote roots of numbers, with the most common being the square root (√) and cube root (∛).
The radicand is the value under the radical sign. For example, in the expression \( \sqrt{25} \), 25 is the radicand. If the number under the radical can be expressed as the product of two equal integers, it is called a perfect square. For instance, 16 is a perfect square because it can be written as \( 4 \times 4 \).
Here are the steps to simplify a radical expression:
-
Identify Perfect Squares: Check if the radicand is a perfect square. If it is, you can simplify the radical. For example, \( \sqrt{36} = 6 \) because 36 is a perfect square (6 x 6). -
Factor the Radicand: If the radicand is not a perfect square, factor it to find any perfect square factors. For example, to simplify \( \sqrt{72} \):- Factor 72 into its prime factors: \( 72 = 2^3 \times 3^2 \).
- Separate the perfect squares: \( 72 = (2^2 \times 3^2) \times 2 \).
- Take the square root of the perfect squares: \( \sqrt{2^2 \times 3^2 \times 2} = 2 \times 3 \times \sqrt{2} = 6\sqrt{2} \).
-
Simplify Radicals with Variables: Apply the same principles to variables. For example, to simplify \( \sqrt{50x^2} \):- Factor the radicand: \( 50x^2 = 25 \times 2 \times x^2 \).
- Take the square root of the perfect squares: \( \sqrt{25 \times 2 \times x^2} = 5x\sqrt{2} \).
Understanding these steps will help you simplify radical expressions efficiently and accurately.
Perfect Squares and Perfect Cubes
Understanding perfect squares and perfect cubes is essential for simplifying radical expressions. Here are some key concepts and examples to help you master this topic.
Perfect Squares
A perfect square is a number that can be expressed as the product of an integer with itself. For example, 16 is a perfect square because it equals 4 × 4.
Common perfect squares include:
- 1 = 1 × 1
- 4 = 2 × 2
- 9 = 3 × 3
- 16 = 4 × 4
- 25 = 5 × 5
- 36 = 6 × 6
- 49 = 7 × 7
- 64 = 8 × 8
- 81 = 9 × 9
- 100 = 10 × 10
Perfect Cubes
A perfect cube is a number that can be expressed as the product of an integer with itself twice. For example, 27 is a perfect cube because it equals 3 × 3 × 3.
Common perfect cubes include:
- 1 = 1 × 1 × 1
- 8 = 2 × 2 × 2
- 27 = 3 × 3 × 3
- 64 = 4 × 4 × 4
- 125 = 5 × 5 × 5
- 216 = 6 × 6 × 6
- 343 = 7 × 7 × 7
- 512 = 8 × 8 × 8
- 729 = 9 × 9 × 9
- 1000 = 10 × 10 × 10
Simplifying Radicals Involving Perfect Squares and Cubes
When simplifying radicals, recognizing perfect squares and cubes can make the process much easier. For example:
√16 = √(4 × 4) = 4
³√27 = ³√(3 × 3 × 3) = 3
To simplify a radical expression:
- Identify any perfect square or perfect cube factors of the radicand (the number under the radical sign).
- Rewrite the radicand as the product of these factors.
- Take the square root (or cube root) of the perfect square (or cube) factor out of the radical.
- Simplify the remaining expression.
Examples
| Expression | Simplified Form |
|---|---|
| √50 | √(25 × 2) = 5√2 |
| √72 | √(36 × 2) = 6√2 |
| ³√54 | ³√(27 × 2) = 3³√2 |
| ³√128 | ³√(64 × 2) = 4³√2 |
Practice these steps to become proficient in identifying and simplifying radicals involving perfect squares and cubes. This will make solving more complex radical expressions much more manageable.
Basic Rules of Simplifying Radicals
When simplifying radicals, it's essential to follow certain rules to ensure expressions are in their simplest form. Here are the basic rules and steps for simplifying radical expressions:
-
Identify Perfect Squares: Check if the radicand (the number under the radical) has any perfect square factors. For example, in the expression \(\sqrt{50}\), the number 50 can be factored into \(25 \times 2\), where 25 is a perfect square.
-
Factor the Radicand: Rewrite the radicand as the product of its factors. Using the example above, \(\sqrt{50} = \sqrt{25 \times 2}\).
-
Simplify the Radical: Take the square root of the perfect square factor. Continuing with the example, \(\sqrt{25 \times 2} = \sqrt{25} \times \sqrt{2} = 5\sqrt{2}\).
-
Combine Like Terms: If you have multiple radical terms, combine them if they have the same radicand. For example, \(2\sqrt{3} + 3\sqrt{3} = 5\sqrt{3}\).
-
Rationalize the Denominator: If the radical expression is in the denominator, multiply the numerator and the denominator by the conjugate or a suitable radical to eliminate the radical from the denominator. For instance, to rationalize \(\frac{1}{\sqrt{2}}\), multiply by \(\frac{\sqrt{2}}{\sqrt{2}}\) to get \(\frac{\sqrt{2}}{2}\).
Let's look at a few examples to understand these rules better:
-
Example 1: Simplify \(\sqrt{72}\)
Step 1: Identify perfect square factors of 72. \(72 = 36 \times 2\)
Step 2: Rewrite the radicand. \(\sqrt{72} = \sqrt{36 \times 2}\)
Step 3: Simplify the radical. \(\sqrt{36 \times 2} = \sqrt{36} \times \sqrt{2} = 6\sqrt{2}\)
-
Example 2: Simplify \(\sqrt{18x^3}\)
Step 1: Factor the expression. \(18x^3 = 9 \times 2 \times x^2 \times x\)
Step 2: Simplify the perfect square factors. \(\sqrt{9 \times 2 \times x^2 \times x} = \sqrt{9} \times \sqrt{x^2} \times \sqrt{2x} = 3x\sqrt{2x}\)
Practice these steps with various expressions to become proficient in simplifying radicals. This foundation will be essential for more advanced topics involving radicals.
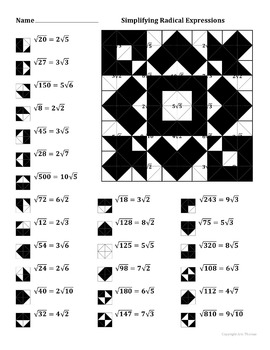
Methods for Simplifying Radical Expressions
To simplify radical expressions, follow these methods step by step:
-
Factor the Radicand:
Break down the number or expression under the radical sign into its prime factors.
Example: \( \sqrt{72} \)
Factor 72: \( 72 = 2^3 \times 3^2 \)
-
Pair Factors and Move Them Outside the Radical:
For every pair of the same factor, move one factor outside the radical sign.
Example: \( \sqrt{72} = \sqrt{2^3 \times 3^2} = 3 \sqrt{2^3} \)
Simplify further: \( 3 \sqrt{8} = 3 \sqrt{4 \times 2} = 3 \times 2 \sqrt{2} = 6 \sqrt{2} \)
-
Simplify Radicals with Variables:
Apply the same method to variables, treating their exponents like numbers.
Example: \( \sqrt{50x^4} \)
Factor 50 and \( x^4 \): \( 50 = 2 \times 5^2 \), \( x^4 = (x^2)^2 \)
Simplify: \( \sqrt{50x^4} = \sqrt{2 \times 5^2 \times (x^2)^2} = 5x^2 \sqrt{2} \)
-
Combine Like Terms:
Add or subtract radicals with the same radicand.
Example: \( 2\sqrt{3} + 4\sqrt{3} = 6\sqrt{3} \)
-
Multiply Radicals:
Multiply the coefficients and the radicands separately.
Example: \( \sqrt{3} \times \sqrt{12} = \sqrt{3 \times 12} = \sqrt{36} = 6 \)
-
Divide Radicals:
Divide the coefficients and the radicands separately.
Example: \( \frac{\sqrt{18}}{\sqrt{2}} = \sqrt{\frac{18}{2}} = \sqrt{9} = 3 \)
By mastering these methods, you will be able to simplify any radical expression efficiently.
Simplifying Radicals with Variables
When simplifying radicals that contain variables, the process is similar to simplifying numerical radicals. However, there are additional considerations when dealing with variables. Here is a step-by-step guide to simplify radicals with variables:
-
Identify and Separate: Start by separating the numerical part and the variable part of the radical. For example, in , separate the number 50 and the variable .
-
Simplify the Numerical Part: Factor the numerical part into its prime factors and identify any perfect squares. For , we get . Thus, simplifies to .
-
Simplify the Variable Part: Apply the property to the variable part. For , we get .
-
Combine the Simplified Parts: Combine the simplified numerical and variable parts. For , we get .
Here are some additional examples to illustrate the process:
By following these steps, you can simplify any radical expression that includes variables.
Adding and Subtracting Radical Expressions
When adding or subtracting radical expressions, it is important to remember that you can only combine like terms. Like terms are radical expressions that have the same radicand (the number or expression inside the radical) and the same index (the root being taken).
Steps for Adding and Subtracting Radical Expressions:
- Simplify the Radicals: Ensure all radical expressions are in their simplest form. This might involve factoring out squares or other powers.
- Identify Like Terms: Look for radicals with the same radicand and index. Only these can be combined.
- Combine Like Terms: Add or subtract the coefficients of the like terms while keeping the radical part the same.
Examples:
Consider the following examples to understand the process:
- Example 1:
- Example 2:
- Example 3:
Simplify \( 3\sqrt{5} + 2\sqrt{5} \)
Since both terms have the same radicand (\( \sqrt{5} \)), you can combine them:
\( 3\sqrt{5} + 2\sqrt{5} = (3 + 2)\sqrt{5} = 5\sqrt{5} \)
Simplify \( 4\sqrt{3} - \sqrt{3} \)
Both terms have the same radicand (\( \sqrt{3} \)), so you can subtract them:
\( 4\sqrt{3} - \sqrt{3} = (4 - 1)\sqrt{3} = 3\sqrt{3} \)
Simplify \( 2\sqrt{2} + 3\sqrt{3} \)
Since the terms have different radicands, they cannot be combined. The expression remains:
\( 2\sqrt{2} + 3\sqrt{3} \)
Practice Problems:
- Simplify \( 5\sqrt{7} + 3\sqrt{7} \)
- Simplify \( 6\sqrt{2} - 2\sqrt{2} \)
- Simplify \( 4\sqrt{3} + 3\sqrt{5} \)
- Simplify \( \sqrt{18} + 2\sqrt{8} - \sqrt{2} \) (Hint: Simplify each radical first)
Multiplying Radical Expressions
When multiplying radical expressions, you can use the product rule of square roots to simplify your calculations. The product rule states that the square root of a product is equal to the product of the square roots of the factors:
$$\sqrt{a \cdot b} = \sqrt{a} \cdot \sqrt{b}$$
Here are the steps to follow when multiplying radical expressions:
- Simplify each radical expression if possible.
- Multiply the radicands (the numbers inside the radical symbols) together.
- Combine the coefficients (the numbers outside the radical symbols) together.
- Simplify the resulting radical expression if necessary.
Let's look at a few examples:
Example 1: $$\sqrt{3} \cdot \sqrt{12}$$
- Step 1: Multiply the radicands: $$3 \cdot 12 = 36$$
- Step 2: Take the square root of the product: $$\sqrt{36} = 6$$
Example 2: $$2\sqrt{5} \cdot 3\sqrt{2}$$
- Step 1: Multiply the coefficients: $$2 \cdot 3 = 6$$
- Step 2: Multiply the radicands: $$5 \cdot 2 = 10$$
- Step 3: Combine them: $$6\sqrt{10}$$
Example 3: $$\sqrt{8} \cdot \sqrt{2}$$
- Step 1: Multiply the radicands: $$8 \cdot 2 = 16$$
- Step 2: Take the square root of the product: $$\sqrt{16} = 4$$
When working with variables under the radicals, you can apply the same principles:
Example 4: $$\sqrt{x^2y} \cdot \sqrt{xy^3}$$
- Step 1: Multiply the radicands: $$x^2y \cdot xy^3 = x^{2+1}y^{1+3} = x^3y^4$$
- Step 2: Take the square root of the product: $$\sqrt{x^3y^4} = x^{\frac{3}{2}}y^2 = x^{1.5}y^2$$
Practice these steps to become proficient in multiplying radical expressions!

Dividing Radical Expressions
When dividing radical expressions, the goal is to simplify the expression while ensuring no radicals remain in the denominator. Here are the steps involved in dividing radical expressions:
-
Divide the coefficients: Start by dividing the coefficients (the numbers outside the radical) as you would with any other numbers.
Example: \(\frac{12\sqrt{18}}{6\sqrt{3}} \rightarrow \frac{12}{6} = 2\)
-
Divide the radicands: Divide the radicands (the numbers inside the radical) by one another.
Example: \(\frac{\sqrt{18}}{\sqrt{3}} = \sqrt{\frac{18}{3}} = \sqrt{6}\)
-
Simplify the radical: Simplify the resulting radical if possible.
Example: \(\frac{12\sqrt{18}}{6\sqrt{3}} \rightarrow 2\sqrt{6}\)
-
Rationalize the denominator (if necessary): If the final expression has a radical in the denominator, multiply the numerator and denominator by the conjugate to remove the radical from the denominator.
Example: \(\frac{3}{\sqrt{2}} \rightarrow \frac{3}{\sqrt{2}} \times \frac{\sqrt{2}}{\sqrt{2}} = \frac{3\sqrt{2}}{2}\)
Let's look at some examples to illustrate these steps:
-
Example 1: \(\frac{\sqrt{50}}{\sqrt{2}}\)
- Divide the radicands: \(\frac{\sqrt{50}}{\sqrt{2}} = \sqrt{\frac{50}{2}} = \sqrt{25} = 5\)
Result: \(5\)
-
Example 2: \(\frac{7\sqrt{45}}{5\sqrt{5}}\)
- Divide the coefficients: \(\frac{7}{5}\)
- Divide the radicands: \(\frac{\sqrt{45}}{\sqrt{5}} = \sqrt{\frac{45}{5}} = \sqrt{9} = 3\)
Result: \(\frac{7 \cdot 3}{5} = \frac{21}{5}\)
By following these steps, you can simplify any radical division problem effectively. Practice with various examples to master these techniques.
Rationalizing the Denominator
Rationalizing the denominator is the process of eliminating any radical expressions from the denominator of a fraction. This makes the expression simpler and easier to work with. Here are the steps to rationalize the denominator:
Case 1: Single Term in the Denominator
If the denominator consists of a single radical term, multiply both the numerator and the denominator by the same radical to remove the radical from the denominator.
- Identify the radical in the denominator.
- Multiply both the numerator and the denominator by this radical.
- Simplify the resulting expression.
Example:
\[
\frac{1}{\sqrt{5}} \times \frac{\sqrt{5}}{\sqrt{5}} = \frac{\sqrt{5}}{5}
\]
Case 2: Binomial in the Denominator
If the denominator is a binomial containing a radical, multiply both the numerator and the denominator by the conjugate of the denominator. The conjugate of a binomial \( a + b \) is \( a - b \).
- Identify the binomial in the denominator.
- Form the conjugate of the binomial.
- Multiply both the numerator and the denominator by the conjugate.
- Simplify the resulting expression.
Example:
\[
\frac{1}{\sqrt{3} + 1} \times \frac{\sqrt{3} - 1}{\sqrt{3} - 1} = \frac{\sqrt{3} - 1}{3 - 1} = \frac{\sqrt{3} - 1}{2}
\]
Additional Tips
- Always check if the radicals can be simplified before rationalizing.
- Combine like terms where possible to further simplify the expression.
Solving Radical Equations
Solving radical equations involves isolating the radical on one side of the equation and then eliminating the radical by raising both sides of the equation to the power that matches the index of the radical. Here are the detailed steps:
-
Isolate the Radical:
Ensure the radical expression is isolated on one side of the equation.
- Example: \( \sqrt{x+3} = 5 \)
-
Eliminate the Radical:
Raise both sides of the equation to the power that matches the index of the radical to eliminate it.
- Example: \( (\sqrt{x+3})^2 = 5^2 \) becomes \( x + 3 = 25 \)
-
Solve the Resulting Equation:
Once the radical is eliminated, solve the resulting equation.
- Example: \( x + 3 = 25 \) simplifies to \( x = 22 \)
-
Check for Extraneous Solutions:
Substitute your solutions back into the original equation to verify if they are true solutions, as raising both sides to a power can introduce extraneous solutions.
- Example: Verify \( x = 22 \) in \( \sqrt{x+3} = 5 \) gives \( \sqrt{22+3} = 5 \) which simplifies to \( 5 = 5 \), so it is a valid solution.
Let's consider a more complex example:
Example: Solve \( \sqrt{2x + 7} = x - 3 \)
- Isolate the radical:
- The radical \( \sqrt{2x + 7} \) is already isolated.
- Eliminate the radical by squaring both sides:
- \( (\sqrt{2x + 7})^2 = (x - 3)^2 \)
- Results in: \( 2x + 7 = x^2 - 6x + 9 \)
- Solve the resulting quadratic equation:
- Rearrange: \( x^2 - 8x + 2 = 0 \)
- Use the quadratic formula: \( x = \frac{-b \pm \sqrt{b^2 - 4ac}}{2a} \)
- Where \( a = 1 \), \( b = -8 \), \( c = 2 \)
- \( x = \frac{8 \pm \sqrt{64 - 8}}{2} = \frac{8 \pm \sqrt{56}}{2} = \frac{8 \pm 2\sqrt{14}}{2} = 4 \pm \sqrt{14} \)
- Check for extraneous solutions:
- Substitute \( x = 4 + \sqrt{14} \) and \( x = 4 - \sqrt{14} \) back into the original equation to verify.
- Only the valid solutions will satisfy the original equation.
Using the Distance Formula with Radicals
The distance formula is used to find the distance between two points in a coordinate plane. The formula is derived from the Pythagorean theorem and involves radicals. The distance \(d\) between two points \((x_1, y_1)\) and \((x_2, y_2)\) is given by:
\[ d = \sqrt{(x_2 - x_1)^2 + (y_2 - y_1)^2} \]
Here are the steps to use the distance formula:
- Identify the coordinates of the two points. Let's denote them as \((x_1, y_1)\) and \((x_2, y_2)\).
- Subtract the x-coordinates and y-coordinates of the points: \( (x_2 - x_1) \) and \( (y_2 - y_1) \).
- Square the differences: \( (x_2 - x_1)^2 \) and \( (y_2 - y_1)^2 \).
- Add the squares of the differences: \( (x_2 - x_1)^2 + (y_2 - y_1)^2 \).
- Take the square root of the sum to get the distance: \( d = \sqrt{(x_2 - x_1)^2 + (y_2 - y_1)^2} \).
Let's work through an example to illustrate these steps:
Example: Find the distance between the points \((3, 4)\) and \((7, 1)\).
- Identify the coordinates: \( (x_1, y_1) = (3, 4) \) and \( (x_2, y_2) = (7, 1) \).
- Calculate the differences: \( x_2 - x_1 = 7 - 3 = 4 \) and \( y_2 - y_1 = 1 - 4 = -3 \).
- Square the differences: \( 4^2 = 16 \) and \( (-3)^2 = 9 \).
- Add the squares: \( 16 + 9 = 25 \).
- Take the square root: \( d = \sqrt{25} = 5 \).
Thus, the distance between the points \((3, 4)\) and \((7, 1)\) is \( 5 \) units.
Practice using the distance formula with these exercises:
- Find the distance between \((2, -1)\) and \((5, 3)\).
- Find the distance between \((-4, 0)\) and \((0, -3)\).
- Find the distance between \((1, 1)\) and \((4, 5)\).
Using the distance formula helps in various mathematical and real-world applications, such as finding the shortest path between two locations on a map.

Using the Midpoint Formula with Radicals
The midpoint formula is used to find the exact center point between two defined points in a coordinate plane. This formula is particularly useful in geometry and various applications of algebra. The formula for the midpoint \( M \) between two points \((x_1, y_1)\) and \((x_2, y_2)\) is given by:
\[ M = \left( \frac{x_1 + x_2}{2}, \frac{y_1 + y_2}{2} \right) \]
When dealing with radicals, you follow the same steps but need to simplify the radicals as part of the process. Let's go through a detailed step-by-step example:
Consider the points \( A ( \sqrt{3}, \sqrt{5} ) \) and \( B ( 2\sqrt{3}, \sqrt{20} ) \).
First, apply the midpoint formula:
Simplify the x-coordinate:
Simplify the y-coordinate:
Combine the simplified coordinates to find the midpoint:
\[
M = \left( \frac{\sqrt{3} + 2\sqrt{3}}{2}, \frac{\sqrt{5} + \sqrt{20}}{2} \right)
\]
\[
\frac{\sqrt{3} + 2\sqrt{3}}{2} = \frac{3\sqrt{3}}{2}
\]
\[
\sqrt{20} = 2\sqrt{5}
\]
\[
\frac{\sqrt{5} + 2\sqrt{5}}{2} = \frac{3\sqrt{5}}{2}
\]
\[
M = \left( \frac{3\sqrt{3}}{2}, \frac{3\sqrt{5}}{2} \right)
\]
Thus, the midpoint \( M \) of the points \( A ( \sqrt{3}, \sqrt{5} ) \) and \( B ( 2\sqrt{3}, \sqrt{20} ) \) is \( \left( \frac{3\sqrt{3}}{2}, \frac{3\sqrt{5}}{2} \right) \).
This method can be applied to any set of points with radicals. Simplifying the radicals at each step ensures the coordinates are in their simplest form.
Practice Worksheets and Quizzes
Here, you'll find a collection of practice worksheets and quizzes to help reinforce your understanding of simplifying radical expressions. These resources are designed to offer a variety of problems, ranging from basic to advanced, ensuring that you can practice and master each concept thoroughly.
Worksheet 1: Simplifying Radicals
This worksheet focuses on simplifying basic radical expressions. It includes a series of problems that guide you through the process step-by-step, starting with simpler problems and gradually increasing in difficulty.
- Simplify \( \sqrt{16} \)
- Simplify \( \sqrt{50} \)
- Simplify \( \sqrt{72} \)
- Simplify \( 2\sqrt{18} \)
Worksheet 2: Adding and Subtracting Radicals
This worksheet provides practice problems for adding and subtracting radical expressions. Each problem is designed to help you apply the rules for combining like radicals.
- Simplify \( 2\sqrt{3} + 4\sqrt{3} \)
- Simplify \( 5\sqrt{5} - \sqrt{5} \)
- Simplify \( 3\sqrt{2} + 2\sqrt{8} \)
- Simplify \( \sqrt{27} + 2\sqrt{12} \)
Worksheet 3: Multiplying and Dividing Radicals
These problems focus on multiplying and dividing radical expressions, including those with variables. This worksheet helps you practice applying the distributive property and rationalizing the denominator.
- Simplify \( \sqrt{2} \times \sqrt{8} \)
- Simplify \( \frac{\sqrt{50}}{\sqrt{2}} \)
- Simplify \( (3\sqrt{5})(2\sqrt{2}) \)
- Simplify \( \frac{4\sqrt{3}}{\sqrt{12}} \)
Quiz: Test Your Skills
After practicing with the worksheets, take this quiz to test your skills. The quiz includes a mix of problems from all topics covered, providing a comprehensive review.
- Simplify \( \sqrt{45} \)
- Simplify \( 3\sqrt{18} + 2\sqrt{2} \)
- Simplify \( \frac{5\sqrt{10}}{\sqrt{2}} \)
- Solve \( \sqrt{x} + 3 = 7 \)
Answer Keys
Answer keys are provided for each worksheet and quiz, ensuring that you can check your work and understand any mistakes. Detailed solutions help you learn the correct methods.
Additional Resources and References
Below are some valuable resources and references to aid in mastering the simplification of radical expressions. These resources include printable worksheets, online tutorials, and comprehensive guides:
- Effortless Math
- - Offers detailed worksheets and examples on simplifying radical expressions.
- Example exercises:
- \(\sqrt{121x^4}\)
- \(\sqrt{225x^6}\)
- \(\sqrt{36x^2} \times \sqrt{64}\)
- Math Worksheets 4 Kids
- - Provides a variety of printable worksheets covering topics such as identifying the radicand and index, converting between radical and exponential forms, and simplifying radical expressions.
- Cuemath
- - Features worksheets and practice problems for different grade levels, helping students to solidify their understanding of radical expressions.
- Worksheets include:
- Radical Expression Worksheet - 1
- Radical Expression Worksheet - 2
- Radical Expression Worksheet - 3
- Khan Academy
- - Offers video tutorials, interactive exercises, and detailed explanations on simplifying radical expressions.
For additional practice, consider exploring these worksheets and guides to strengthen your understanding and proficiency in simplifying radical expressions. Regular practice with these resources will ensure a solid grasp of the concepts and techniques involved.
Xem video 'Bài Tập Giản Lược Biểu Thức Chứa Căn' để học cách giản lược biểu thức chứa căn một cách hiệu quả. Video này cung cấp các bài tập và hướng dẫn chi tiết, phù hợp cho người học toán ở mọi trình độ.
Bài Tập Giản Lược Biểu Thức Chứa Căn
READ MORE:
Giảm biểu thức căn bậc hai với biến số, mũ, phân số, căn bậc ba - Đại số
Giảm biểu thức căn bậc hai với biến số, mũ, phân số, căn bậc ba - Đại số










