Simplify Radical Expressions Solver: Your Ultimate Guide to Mastering Radicals

Simplifying radical expressions involves breaking down the expression into its simplest form. This process includes factoring the radicand, identifying perfect squares, and applying properties of radicals.

Steps to Simplify Radical Expressions

1. Factor the radicand into its prime factors.
2. Identify and extract perfect squares from the radicand.
3. Apply the property \( \sqrt{a \cdot b} = \sqrt{a} \cdot \sqrt{b} \).
4. Simplify the expression by multiplying the numbers outside the radical.

Example: Simplifying \( \sqrt{72} \)

1. Factor 72 into prime factors: \( 72 = 2^3 \cdot 3^2 \).
2. Identify perfect squares: \( 72 = (2^2 \cdot 3^2) \cdot 2 = 36 \cdot 2 \).
3. Apply the property: \( \sqrt{72} = \sqrt{36 \cdot 2} = \sqrt{36} \cdot \sqrt{2} \).
4. Simplify: \( \sqrt{36} = 6 \), so \( \sqrt{72} = 6\sqrt{2} \).

Example: Simplifying \( \sqrt{200} \)

1. Factor 200 into prime factors: \( 200 = 2^3 \cdot 5^2 \).
2. Identify perfect squares: \( 200 = (2^2 \cdot 5^2) \cdot 2 = 100 \cdot 2 \).
3. Apply the property: \( \sqrt{200} = \sqrt{100 \cdot 2} = \sqrt{100} \cdot \sqrt{2} \).
4. Simplify: \( \sqrt{100} = 10 \), so \( \sqrt{200} = 10\sqrt{2} \).

Using an Online Radical Expressions Solver

Online solvers can help simplify radical expressions quickly. These tools typically require you to input the radicand and then provide a step-by-step solution. Popular features of these solvers include:

• Detailed step-by-step simplification process.
• Ability to handle complex expressions.
• Support for various types of radicals, including square roots and higher-order roots.
• Instant results with visual representation of each step.

Benefits of Simplifying Radical Expressions

• Makes complex calculations more manageable.
• Helps in solving algebraic equations more efficiently.
• Facilitates better understanding of mathematical properties and relationships.

Practice Problems

Try simplifying the following radical expressions:

1. \( \sqrt{50} \)
2. \( \sqrt{98} \)
3. \( \sqrt{288} \)
4. \( \sqrt{45} \)

Use the steps outlined above to simplify these expressions and check your answers with an online solver for accuracy.

Radical expressions are mathematical expressions that contain a radical symbol (√). They are used to represent the roots of numbers and variables. Simplifying radical expressions involves reducing them to their simplest form, making calculations easier and more understandable.

Understanding the basics of radical expressions is crucial for mastering algebra and higher-level math. Here's a detailed introduction to the key concepts:

• Radicand: The number or expression inside the radical symbol. For example, in \( \sqrt{25} \), the radicand is 25.
• Index: The small number written just above and to the left of the radical symbol indicating which root is taken. For example, in \( \sqrt[3]{8} \), the index is 3.

Radical expressions follow specific properties that help in their simplification:

• \( \sqrt{a \cdot b} = \sqrt{a} \cdot \sqrt{b} \)
• \( \sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}} \)
• \( (\sqrt{a})^2 = a \)

To simplify a radical expression, follow these steps:

1. Factor the radicand into its prime factors.
2. Identify and extract perfect squares or cubes from the radicand.
3. Apply the properties of radicals to simplify the expression.

Let's consider an example to illustrate the simplification process:

Example: Simplify \( \sqrt{72} \)

1. Factor 72 into its prime factors: \( 72 = 2^3 \cdot 3^2 \).
2. Identify perfect squares: \( 72 = (2^2 \cdot 3^2) \cdot 2 = 36 \cdot 2 \).
3. Apply the properties: \( \sqrt{72} = \sqrt{36 \cdot 2} = \sqrt{36} \cdot \sqrt{2} \).
4. Simplify: \( \sqrt{36} = 6 \), so \( \sqrt{72} = 6\sqrt{2} \).

By understanding and applying these steps, you can simplify any radical expression efficiently. Mastering this skill will greatly enhance your mathematical abilities and prepare you for more advanced topics.

Radical expressions involve roots, which are represented by the radical symbol (√). To understand radical expressions, it's important to know the components: the radicand and the index. The radicand is the number or expression inside the radical symbol, and the index is the small number outside the radical that indicates the degree of the root. If no index is shown, it is assumed to be a square root.

For example, in the expression \( \sqrt[3]{8} \), 8 is the radicand and 3 is the index, indicating a cube root.

The process of simplifying radical expressions involves:

• Identifying the radicand and the index.
• Factoring the radicand into prime factors.
• Applying the product rule for radicals: \( \sqrt[n]{a \cdot b} = \sqrt[n]{a} \cdot \sqrt[n]{b} \).
• Simplifying the expression by taking out perfect n-th powers from under the radical.

Here is a step-by-step example:

1. Consider \( \sqrt{50} \).
2. Factor 50 into prime factors: \( 50 = 2 \cdot 5^2 \).
3. Apply the product rule: \( \sqrt{50} = \sqrt{2 \cdot 5^2} \).
4. Simplify by taking the square root of 25 out of the radical: \( \sqrt{50} = \sqrt{2} \cdot 5 = 5\sqrt{2} \).

Understanding these basics allows you to simplify any radical expression with confidence. Whether working with square roots, cube roots, or higher-order roots, the principles remain the same.

Prime factorization is a crucial step in simplifying radical expressions. It involves breaking down a composite number into its prime factors. Here is a detailed step-by-step guide:

1. Identify the radicand (the number under the radical sign) that you want to simplify. For example, let's consider the number 72 under a square root: \( \sqrt{72} \).

2. Perform prime factorization of the radicand. Begin with the smallest prime number, which is 2, and divide the radicand by 2 until you can't anymore:

   • \( 72 \div 2 = 36 \)
   • \( 36 \div 2 = 18 \)
   • \( 18 \div 2 = 9 \)
   • \( 9 \div 3 = 3 \)
   • \( 3 \div 3 = 1 \)

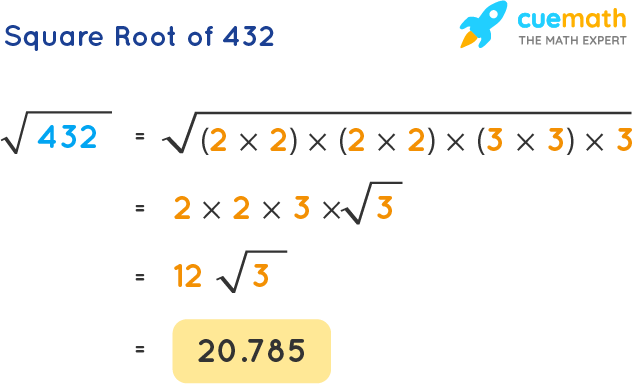
   So, the prime factors of 72 are \( 2 \times 2 \times 2 \times 3 \times 3 \) or \( 2^3 \times 3^2 \).

3. Rewrite the radicand using its prime factors:

   \( \sqrt{72} = \sqrt{2^3 \times 3^2} \)

4. Group the prime factors into pairs (since we are dealing with square roots, we group in pairs of two):

   \( \sqrt{2^3 \times 3^2} = \sqrt{(2^2 \times 2) \times 3^2} = \sqrt{2^2 \times 3^2 \times 2} \)

5. Take the square root of each pair of prime factors and move them outside the radical:

   \( \sqrt{2^2} \times \sqrt{3^2} \times \sqrt{2} = 2 \times 3 \times \sqrt{2} = 6\sqrt{2} \)

6. The simplified form of \( \sqrt{72} \) is \( 6\sqrt{2} \).

This method can be applied to any radicand to simplify it using prime factorization, making it easier to handle in mathematical expressions and calculations.

Identifying perfect squares is a crucial step in simplifying radical expressions. Perfect squares are numbers that can be expressed as the square of an integer. For example, 1, 4, 9, 16, and 25 are all perfect squares because they can be written as \(1^2, 2^2, 3^2, 4^2, \) and \(5^2\) respectively. Understanding and identifying these perfect squares helps in breaking down and simplifying radical expressions efficiently.

To identify and utilize perfect squares in simplifying radicals, follow these steps:

1. Find the prime factorization of the radicand.
2. Group the factors into pairs.
3. Move each pair of factors outside the radical as a single number.
4. Multiply the numbers outside the radical.
5. Leave any unpaired factors inside the radical.

Here’s a detailed example to illustrate the process:

• Consider the radical expression \( \sqrt{72} \).
• First, find the prime factorization of 72: \( 72 = 2 \times 2 \times 2 \times 3 \times 3 \).
• Group the factors into pairs: \( 72 = (2 \times 2) \times 2 \times (3 \times 3) \).
• Move each pair of factors outside the radical: \( \sqrt{72} = 2 \times 3 \times \sqrt{2} \).
• Multiply the numbers outside the radical: \( 2 \times 3 = 6 \).
• The simplified form is: \( 6\sqrt{2} \).

Recognizing perfect squares and applying these steps will make simplifying radical expressions straightforward and efficient. By practicing these techniques, you can handle more complex expressions with confidence.

Understanding the properties of radicals is crucial in simplifying radical expressions. Here are the key properties:

• Product Property: The radical of a product is the product of the radicals. For example, \( \sqrt{a \cdot b} = \sqrt{a} \cdot \sqrt{b} \).
• Quotient Property: The radical of a quotient is the quotient of the radicals. For example, \( \sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}} \) (for \( b \neq 0 \)).
• Power Property: The radical of a power can be simplified by dividing the exponent by the index. For example, \( \sqrt[n]{a^m} = a^{\frac{m}{n}} \).

These properties are essential when working with radical expressions, enabling easier manipulation and simplification.

Here are some examples demonstrating the simplification of radical expressions:

• Example 1: Simplify \( \sqrt{72} \)
  1. Find a perfect square factor of 72. The largest perfect square factor is 36.
  2. Rewrite \( 72 \) as \( 36 \times 2 \).
  3. Apply the property \( \sqrt{ab} = \sqrt{a} \times \sqrt{b} \):
  4. \( \sqrt{72} = \sqrt{36 \times 2} = \sqrt{36} \times \sqrt{2} = 6\sqrt{2} \)
• Example 2: Simplify \( \sqrt{48} \)
  1. Find a perfect square factor of 48. The largest perfect square factor is 16.
  2. Rewrite \( 48 \) as \( 16 \times 3 \).
  3. Apply the property \( \sqrt{ab} = \sqrt{a} \times \sqrt{b} \):
  4. \( \sqrt{48} = \sqrt{16 \times 3} = \sqrt{16} \times \sqrt{3} = 4\sqrt{3} \)
• Example 3: Simplify \( \sqrt{200} \)
  1. Find a perfect square factor of 200. The largest perfect square factor is 100.
  2. Rewrite \( 200 \) as \( 100 \times 2 \).
  3. Apply the property \( \sqrt{ab} = \sqrt{a} \times \sqrt{b} \):
  4. \( \sqrt{200} = \sqrt{100 \times 2} = \sqrt{100} \times \sqrt{2} = 10\sqrt{2} \)
• Example 4: Simplify \( \sqrt{12x^2y^4} \)
  1. Separate the numbers and variables. The largest perfect square factor of 12 is 4.
  2. Rewrite \( 12 \) as \( 4 \times 3 \) and express variables in pairs:
  3. \( \sqrt{12x^2y^4} = \sqrt{4 \times 3 \times x^2 \times y^4} = \sqrt{4} \times \sqrt{3} \times \sqrt{x^2} \times \sqrt{y^4} \)
  4. Simplify each square root:
  5. \( \sqrt{12x^2y^4} = 2 \times \sqrt{3} \times x \times y^2 = 2xy^2\sqrt{3} \)

Online radical expression solvers are powerful tools that can simplify complex radicals quickly and accurately. These solvers utilize algorithms to perform operations and simplify expressions, making them valuable for students and professionals alike.

Here are the steps to use an online radical expressions solver:

1. Choose a reliable solver: Select a trusted online tool such as CalculatorSoup, Microsoft Math Solver, or Calculator-Online.
2. Input the expression: Enter the radical expression you want to simplify. Ensure you input the correct format, such as \(\sqrt[3]{54}\) or \(2\sqrt{50}\).
3. Select the operation: Depending on the tool, you may need to select the type of simplification or operation you want to perform, such as simplifying, adding, or multiplying radicals.
4. Calculate: Click on the 'Calculate' or 'Solve' button to process the expression. The solver will then perform the necessary calculations and display the simplified form.
5. Review the steps: Many solvers provide a step-by-step breakdown of the simplification process. This helps you understand how the final result was obtained.

For example, using the CalculatorSoup Simplify Radical Expressions Calculator:

• Input: \( \sqrt{75} + \sqrt{12} \)
• Steps:
  • Factorize the radicands: \( \sqrt{25 \times 3} + \sqrt{4 \times 3} \)
  • Simplify: \( 5\sqrt{3} + 2\sqrt{3} \)
  • Combine like terms: \( 7\sqrt{3} \)
• Output: \( 7\sqrt{3} \)

These solvers are beneficial as they save time, reduce errors, and provide educational value by showing the steps involved in simplifying radicals.

When simplifying radical expressions, there are several common mistakes that can lead to incorrect results. Here are some of the most frequent errors and tips on how to avoid them:

• Not Simplifying Completely:

  Ensure that all factors of the radicand that can be simplified are fully simplified. For example, in \(\sqrt{50}\), the correct simplification should be \(5\sqrt{2}\), not just \(\sqrt{50}\).

• Incorrect Application of the Product Property:

  The product property of radicals states that \(\sqrt{ab} = \sqrt{a} \cdot \sqrt{b}\). Misapplying this property can lead to incorrect results. For example, \(\sqrt{12}\) should be simplified to \(2\sqrt{3}\) by recognizing that \(12 = 4 \times 3\).

• Misunderstanding the Quotient Property:

  The quotient property of radicals states that \(\sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}}\). It is essential to apply this property correctly. For example, \(\sqrt{\frac{9}{16}} = \frac{3}{4}\).

• Failing to Rationalize the Denominator:

  Leaving radicals in the denominator is generally considered improper. For instance, \(\frac{1}{\sqrt{2}}\) should be rationalized to \(\frac{\sqrt{2}}{2}\).

• Ignoring the Principal Root:

  Remember that \(\sqrt{x^2} = |x|\) to account for the absolute value, especially when dealing with variables. For example, \(\sqrt{x^2} = |x|\) and not just \(x\).

• Incorrect Combination of Radicals:

  Only like radicals can be combined. For instance, \(\sqrt{2} + \sqrt{3}\) cannot be simplified further, but \(2\sqrt{5} + 3\sqrt{5} = 5\sqrt{5}\).

• Forgetting to Check for Negative Radicands:

  In real numbers, the radicand must be non-negative. For example, \(\sqrt{-4}\) is not defined in the set of real numbers.

Simplifying radicals can sometimes involve advanced techniques to handle more complex expressions. Below are several advanced methods to consider:

• Recognizing Perfect Powers:

  When simplifying radicals, look for perfect power factors within the radicand. For example:

  To simplify \( \sqrt[3]{54} \):

  1. Factor 54 into \( 2 \times 3^3 \).
  2. Recognize that \( 3^3 \) is a perfect cube.
  3. Rewrite as \( \sqrt[3]{2 \times 3^3} = 3 \sqrt[3]{2} \).
• Combining Like Terms:

  When you have multiple radical terms, combine like terms to simplify the expression. For example:

  Combine \( 3\sqrt{2} + 2\sqrt{2} \):

  1. Recognize that both terms have the same radicand.
  2. Add the coefficients: \( 3 + 2 = 5 \).
  3. The simplified form is \( 5\sqrt{2} \).
• Rationalizing the Denominator:

  Rationalizing involves eliminating radicals from the denominator of a fraction. For example:

  To rationalize \( \frac{5}{\sqrt{3}} \):

  1. Multiply the numerator and denominator by \( \sqrt{3} \).
  2. The result is \( \frac{5 \sqrt{3}}{3} \).
• Using Conjugates:

  When rationalizing denominators with binomials, use the conjugate. For example:

  To rationalize \( \frac{1}{1 + \sqrt{2}} \):

  1. Multiply by the conjugate \( 1 - \sqrt{2} \): \( \frac{1}{1 + \sqrt{2}} \times \frac{1 - \sqrt{2}}{1 - \sqrt{2}} \).
  2. Expand the numerator and the denominator: \( \frac{1 - \sqrt{2}}{1 - 2} = \frac{1 - \sqrt{2}}{-1} \).
  3. Simplify the expression to \( -1 + \sqrt{2} \).
• Simplifying Radicals with Variables:

  To simplify expressions involving variables, apply the rules of exponents. For example:

  To simplify \( \sqrt{x^{12}} \):

  1. Recognize that \( x^{12} \) is a perfect square: \( (x^6)^2 \).
  2. The simplified form is \( x^6 \).

  For expressions with odd exponents, separate the factors. For example, to simplify \( \sqrt{x^{11}} \):

  1. Rewrite \( x^{11} \) as \( x^{10} \times x \).
  2. Recognize that \( x^{10} \) is a perfect square: \( (x^5)^2 \).
  3. The simplified form is \( x^5\sqrt{x} \).

Simplified radicals play a significant role in various algebraic applications. Here are some key areas where simplified radicals are used:

• Simplifying Expressions:

  One of the primary uses of simplified radicals is in simplifying algebraic expressions. By reducing radicals to their simplest form, it becomes easier to perform operations such as addition, subtraction, multiplication, and division.

• Solving Equations:

  Simplified radicals are often used to solve algebraic equations. This is particularly useful in quadratic equations where the solutions can be expressed in terms of radicals. For example, the quadratic formula:

  
  \[
  x = \frac{{-b \pm \sqrt{{b^2 - 4ac}}}}{2a}
  \]

  In this formula, simplifying the radical part can provide clearer and more precise solutions.

• Rationalizing Denominators:

  In algebra, it's often necessary to rationalize denominators that contain radicals. This involves rewriting the expression so that the denominator is a rational number. For example:

  
  \[
  \frac{1}{\sqrt{2}} = \frac{\sqrt{2}}{2}
  \]

  Rationalizing the denominator makes it easier to perform further algebraic operations.

• Geometry and Trigonometry:

  In geometry, simplified radicals are used to express lengths, areas, and other measurements. In trigonometry, they help in simplifying expressions involving trigonometric identities and functions.

• Calculus:

  Simplified radicals appear in calculus, especially when dealing with limits, derivatives, and integrals. For example, simplifying the radical part of an integral can make the integration process more straightforward.

Overall, the ability to simplify radicals efficiently can significantly enhance problem-solving skills and mathematical understanding in various algebraic contexts.

Simplifying radical expressions is a fundamental skill in algebra that can greatly enhance your ability to solve complex mathematical problems. By mastering the techniques of simplification, you can make radical expressions more manageable and easier to work with. Throughout this guide, we have explored various methods to simplify radicals, from basic steps to advanced techniques.

Understanding the applications of simplified radicals in algebra helps to underscore their importance. Whether you are solving quadratic equations, working with polynomial expressions, or tackling geometry problems, the ability to simplify radicals is invaluable.

To further deepen your knowledge, consider exploring additional resources and practice problems. These will not only reinforce what you've learned but also introduce new strategies and concepts. Below are some recommended resources for further reading:

By continuously practicing and applying these techniques, you'll become proficient in handling radical expressions, making your algebraic journey smoother and more rewarding. Keep exploring and practicing, and you'll see significant improvement in your mathematical skills.