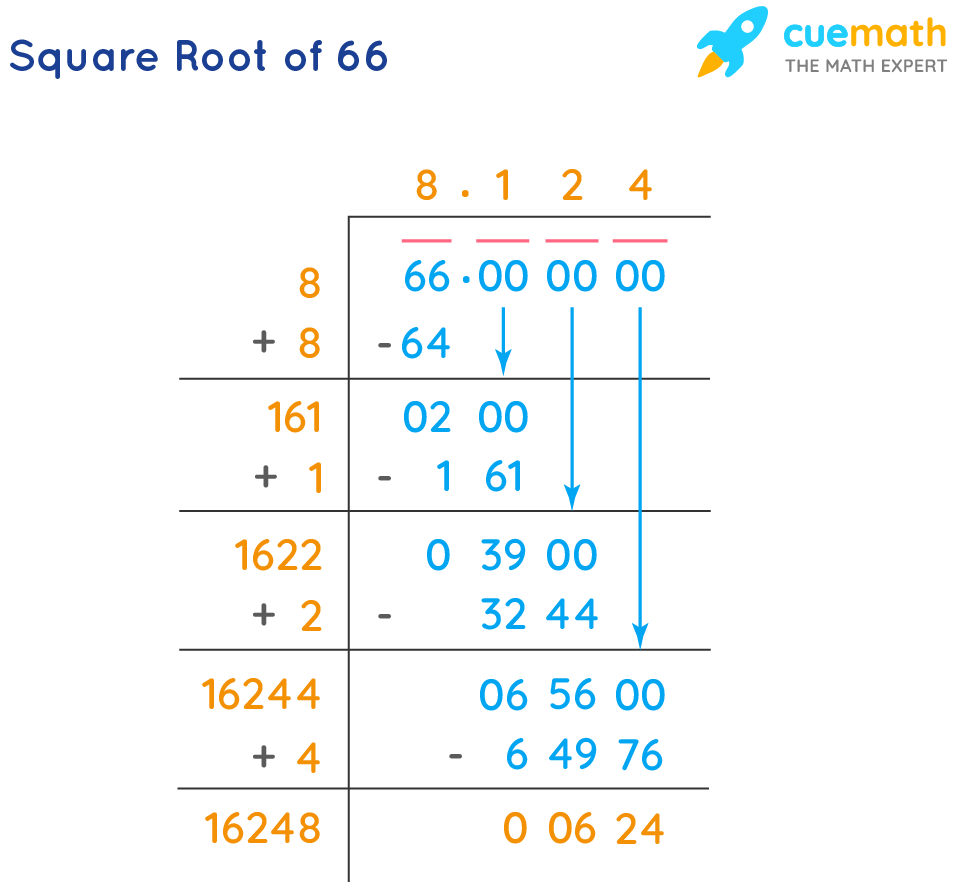
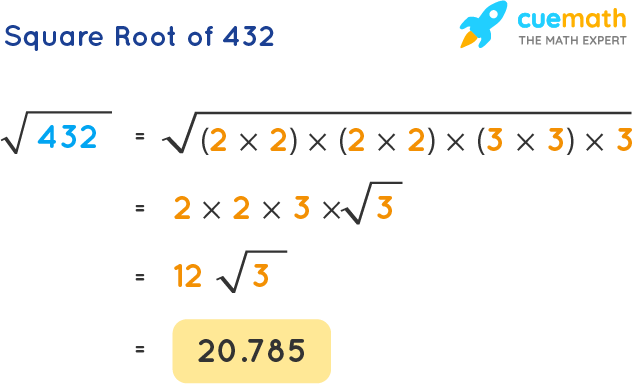
Topic simplify radical expressions with variables calculator: Discover the easiest way to simplify radical expressions with variables using our calculator. This tool not only simplifies your calculations but also provides a step-by-step guide, making complex radicals manageable. Perfect for students and math enthusiasts, it ensures accuracy and efficiency, enhancing your understanding and skills in simplifying radicals.
Table of Content
- Simplifying Radical Expressions with Variables
- Introduction to Radical Expressions
- Understanding Radicals
- Rules for Simplifying Radicals
- Using a Calculator for Simplification
- Step-by-Step Simplification Process
- Examples of Simplifying Radicals
- Special Cases in Simplification
- Applications of Radical Simplification
- Common Mistakes to Avoid
- Conclusion and Further Resources
- YOUTUBE: Hướng dẫn chi tiết về cách đơn giản hóa biểu thức căn với biến, phù hợp cho người học toán.
Simplifying Radical Expressions with Variables
Simplifying radical expressions with variables can often seem challenging, but using a calculator can make the process much easier. These calculators help in reducing expressions to their simplest form by following mathematical rules and using prime factorization.
Key Features of Radical Expression Calculators
- Handles various forms of radicals, including square roots, cube roots, and higher orders.
- Supports operations such as addition, subtraction, multiplication, and division of radicals.
- Provides step-by-step solutions for better understanding.
Basic Rules for Simplifying Radicals
- The radicand must be positive, and the root order should be 2 or greater.
- Combine like terms when possible to simplify the expression further.
- Use prime factorization to break down numbers under the radical.
Examples of Simplified Radical Expressions
| Expression | Simplified Form |
|---|---|
| \(\sqrt{75} + \sqrt{12}\) | \(7\sqrt{3}\) |
| \(\sqrt{18} \times \sqrt{14}\) | \(6\sqrt{7}\) |
These calculators not only simplify expressions but also help in learning the underlying concepts of radicals and their properties.
How to Use a Simplify Radical Expressions Calculator
- Select the desired operation from the options provided.
- Input the required values into the designated fields.
- Click the calculate button to receive the simplified form and detailed steps.
Using these tools can significantly enhance your understanding and efficiency when working with radical expressions.

READ MORE:
Introduction to Radical Expressions
Radical expressions involve roots of numbers or variables, commonly represented using the radical symbol (√). Understanding the basics of radicals is crucial for simplifying complex expressions in algebra.
Radicals can be simplified by applying the product and quotient rules. For example, to simplify a radical expression, you can factor the radicand into its prime components and extract any perfect squares or cubes.
Using calculators can greatly assist in simplifying these expressions step-by-step, providing solutions for addition, subtraction, multiplication, and division of radicals efficiently.
Understanding Radicals
Radicals are expressions that involve roots, such as square roots or cube roots. A radical expression is composed of a radicand, which is the number or expression inside the radical symbol, and an index, which indicates the degree of the root. For example, in the expression \( \sqrt[3]{x^2} \), \( x^2 \) is the radicand and 3 is the index.
The most common type of radical is the square root, where the index is 2. For example, \( \sqrt{9} = 3 \) because \( 3^2 = 9 \). Similarly, \( \sqrt[3]{8} = 2 \) because \( 2^3 = 8 \). Radicals can also include variables, such as \( \sqrt{x} \) or \( \sqrt[3]{x^2} \).
Simplifying radicals involves rewriting them in their simplest form. This process includes:
- Factoring the Radicand: Break down the number or expression inside the radical into its prime factors. For instance, \( \sqrt{50} \) can be factored into \( \sqrt{25 \times 2} \).
- Using Properties of Exponents: Apply properties such as \( \sqrt{a \times b} = \sqrt{a} \times \sqrt{b} \). For example, \( \sqrt{25 \times 2} = \sqrt{25} \times \sqrt{2} = 5\sqrt{2} \).
Here are some steps to simplify radical expressions with variables:
- Factor the Radicand: Identify and factor the radicand into its prime factors, considering any variables. For example, \( \sqrt{32x^4} = \sqrt{16 \times 2 \times x^4} \).
- Separate the Radicands: Split the radical into separate radicals for each factor. For instance, \( \sqrt{16 \times 2 \times x^4} = \sqrt{16} \times \sqrt{2} \times \sqrt{x^4} \).
- Simplify Each Radical: Simplify each radical individually. Using the example above, \( \sqrt{16} = 4 \), \( \sqrt{2} \) remains \( \sqrt{2} \), and \( \sqrt{x^4} = x^2 \). Therefore, \( \sqrt{32x^4} = 4x^2\sqrt{2} \).
The simplified form of a radical expression should have no perfect square factors other than 1 under the radical, and no fractions under the radical sign. For instance, \( \frac{\sqrt{50}}{5} = \sqrt{2} \).
Understanding these fundamental concepts will aid in simplifying more complex radical expressions and applying these techniques to solve equations involving radicals.
Rules for Simplifying Radicals
To simplify radical expressions, follow these fundamental rules and steps:
- Identify the Radicand and the Index:
The radicand is the number or expression inside the radical sign, and the index is the root (e.g., square root, cube root).
- Prime Factorization:
Break down the radicand into its prime factors.
Example: \( \sqrt{75} = \sqrt{3 \cdot 5^2} \).
- Apply the Product Rule for Radicals:
The product rule states that \( \sqrt{a \cdot b} = \sqrt{a} \cdot \sqrt{b} \).
Example: \( \sqrt{75} = \sqrt{25 \cdot 3} = \sqrt{25} \cdot \sqrt{3} = 5\sqrt{3} \).
- Simplify the Radicals:
Take the square root of any perfect squares out of the radical.
Example: \( \sqrt{75} = 5\sqrt{3} \).
- Combine Like Terms:
If you have multiple radicals with the same radicand, combine them.
Example: \( 5\sqrt{3} + 2\sqrt{3} = 7\sqrt{3} \).
- Rationalize the Denominator:
If there is a radical in the denominator, multiply the numerator and the denominator by the radical to eliminate it.
Example: \( \frac{1}{\sqrt{2}} = \frac{1}{\sqrt{2}} \cdot \frac{\sqrt{2}}{\sqrt{2}} = \frac{\sqrt{2}}{2} \).
Here are some additional rules that can help in simplifying radicals:
- The nth root must be larger than or equal to 2.
- The radicand must be a non-negative number.
- Square roots are the radicals of order 2 and can be simplified by finding the square of a number.
For more complex expressions, consider using a calculator to simplify the radicals. These tools can break down the steps and provide accurate results efficiently.
Using a Calculator for Simplification
Using a calculator to simplify radical expressions, especially those with variables, can be incredibly helpful. These calculators perform various operations, ensuring accurate and simplified results quickly. Below are detailed steps on how to use an online calculator to simplify radical expressions:
-
Select the Operation: Start by choosing the specific operation you want to perform. Many online calculators offer options such as addition, subtraction, multiplication, division, and simplification of radical expressions.
-
Input the Expression: Enter the radical expression into the designated fields. For instance, if you are working with an expression like
\(3\sqrt{18x^2}\), input it correctly to ensure accurate simplification. -
Prime Factorization: The calculator will often perform prime factorization of the radicand (the number inside the radical). This process breaks down the radicand into its prime factors to simplify the expression. For example,
\(\sqrt{18x^2}\)becomes\(\sqrt{9 \cdot 2 \cdot x^2}\). -
Simplify the Expression: The calculator will then simplify the expression by taking out the perfect squares from under the radical. Using our example,
\(\sqrt{9 \cdot 2 \cdot x^2}\)simplifies to3x\sqrt{2}\). -
Result Display: After simplification, the calculator displays the simplified form of the expression. This can be very useful for checking your work or for performing quick calculations when dealing with complex expressions.
Online calculators such as Symbolab, Calculator Soup, and Calculator-Online.net provide user-friendly interfaces and detailed steps, making the simplification process straightforward. They also handle special cases and more complex operations efficiently, ensuring that your expressions are simplified correctly every time.

Step-by-Step Simplification Process
Simplifying radical expressions with variables can be done efficiently by following a systematic process. Below is a detailed step-by-step guide to simplify these expressions:
-
Identify and Factorize the Radicand: Start by breaking down the number or expression inside the radical into its prime factors. For example, if you have \( \sqrt{50} \), factorize 50 into \( 2 \times 5^2 \). If the radicand contains variables, factor them as well. For instance, \( \sqrt{50x^4} \) becomes \( \sqrt{2 \times 5^2 \times x^4} \).
-
Apply the Square Root to the Factors: Use the property of square roots that \( \sqrt{a \times b} = \sqrt{a} \times \sqrt{b} \). For our example, \( \sqrt{50x^4} = \sqrt{2} \times \sqrt{5^2} \times \sqrt{x^4} \).
-
Simplify the Square Roots: Take out the square roots of any perfect squares. \( \sqrt{5^2} \) simplifies to 5, and \( \sqrt{x^4} \) simplifies to \( x^2 \). Therefore, \( \sqrt{50x^4} = 5x^2\sqrt{2} \).
-
Combine Like Terms: If you have multiple radical terms, combine them if they have the same radicand. For instance, \( 3\sqrt{2} + 4\sqrt{2} \) becomes \( 7\sqrt{2} \).
-
Rationalize the Denominator: If the expression is a fraction and the denominator contains a radical, multiply the numerator and denominator by a suitable radical to remove the radical from the denominator. For example, to rationalize \( \frac{1}{\sqrt{2}} \), multiply by \( \frac{\sqrt{2}}{\sqrt{2}} \) to get \( \frac{\sqrt{2}}{2} \).
By following these steps, you can simplify any radical expression, making it easier to handle in further calculations or applications.
Examples of Simplifying Radicals
To effectively understand the process of simplifying radical expressions with variables, let's go through several examples step-by-step:
Example 1: Simplify \(\sqrt{27x^3}\)
- Identify the factors inside the radical that are perfect squares: \(\sqrt{27x^3} = \sqrt{9 \cdot 3 \cdot x^2 \cdot x}\).
- Apply the property \(\sqrt{a \cdot b} = \sqrt{a} \cdot \sqrt{b}\): \(\sqrt{9} \cdot \sqrt{3} \cdot \sqrt{x^2} \cdot \sqrt{x}\).
- Simplify each radical: \(3 \cdot \sqrt{3} \cdot x \cdot \sqrt{x} = 3x \sqrt{3x}\).
Example 2: Simplify \(\sqrt{50y^4}\)
- Break down the radical into factors: \(\sqrt{50y^4} = \sqrt{25 \cdot 2 \cdot y^4}\).
- Separate into individual radicals: \(\sqrt{25} \cdot \sqrt{2} \cdot \sqrt{y^4}\).
- Simplify each term: \(5 \cdot \sqrt{2} \cdot y^2 = 5y^2 \sqrt{2}\).
Example 3: Simplify \(\sqrt{72x^5y^3}\)
- Factor inside the radical: \(\sqrt{72x^5y^3} = \sqrt{36 \cdot 2 \cdot x^4 \cdot x \cdot y^2 \cdot y}\).
- Use the property to split the radicals: \(\sqrt{36} \cdot \sqrt{2} \cdot \sqrt{x^4} \cdot \sqrt{x} \cdot \sqrt{y^2} \cdot \sqrt{y}\).
- Simplify each part: \(6 \cdot \sqrt{2} \cdot x^2 \cdot \sqrt{x} \cdot y \cdot \sqrt{y} = 6x^2y \sqrt{2xy}\).
Example 4: Simplify \(\sqrt{125a^6b^2}\)
- Decompose the expression: \(\sqrt{125a^6b^2} = \sqrt{25 \cdot 5 \cdot a^6 \cdot b^2}\).
- Split into separate radicals: \(\sqrt{25} \cdot \sqrt{5} \cdot \sqrt{a^6} \cdot \sqrt{b^2}\).
- Simplify: \(5 \cdot \sqrt{5} \cdot a^3 \cdot b = 5a^3b \sqrt{5}\).
Example 5: Simplify \(\sqrt{32x^8y^2}\)
- Identify the perfect square factors: \(\sqrt{32x^8y^2} = \sqrt{16 \cdot 2 \cdot x^8 \cdot y^2}\).
- Break it into separate radicals: \(\sqrt{16} \cdot \sqrt{2} \cdot \sqrt{x^8} \cdot \sqrt{y^2}\).
- Simplify each radical: \(4 \cdot \sqrt{2} \cdot x^4 \cdot y = 4x^4y \sqrt{2}\).
Special Cases in Simplification
In simplifying radical expressions, there are several special cases to consider. These special cases often involve variables and coefficients that have particular properties which allow for unique simplification techniques.
Case 1: Radicals with Perfect Powers
When the radicand (the number inside the radical) is a perfect power of the same degree as the root, the expression simplifies directly. For example:
- \(\sqrt[3]{27x^3}\) simplifies to \(3x\).
- \(\sqrt[4]{16y^4}\) simplifies to \(2|y|\) or \(2y\) if \(y\) is known to be positive.
Case 2: Variables with Even Exponents
When dealing with variables raised to even exponents under a square root or higher even root, the absolute value is used to ensure non-negative results. For instance:
- \(\sqrt{x^2} = |x|\) because \(x\) can be positive or negative.
- \(\sqrt[4]{y^4} = |y|\) for similar reasons.
Case 3: Radicals in the Denominator
When simplifying expressions with radicals in the denominator, rationalizing the denominator is often necessary. This involves multiplying the numerator and denominator by a suitable radical to eliminate the radical in the denominator. For example:
- Consider \(\frac{1}{\sqrt{2}}\). Multiply by \(\frac{\sqrt{2}}{\sqrt{2}}\) to get \(\frac{\sqrt{2}}{2}\).
- For \(\frac{3}{\sqrt{5} + \sqrt{3}}\), multiply by the conjugate \(\sqrt{5} - \sqrt{3}\) to rationalize the denominator.
Case 4: Complex Radicals
When dealing with complex radicals, such as those involving sums or differences of radicals, it is crucial to simplify each radical individually first before performing any addition or subtraction. Example:
- Simplify \(\sqrt{75} + \sqrt{12}\) by breaking them into their prime factors: \(\sqrt{75} = 5\sqrt{3}\) and \(\sqrt{12} = 2\sqrt{3}\), then combine to get \(7\sqrt{3}\).
Case 5: Nested Radicals
Nested radicals require an understanding of the properties of exponents and radicals. Simplifying these often involves converting the nested radical into an exponential form and then simplifying. For example:
- \(\sqrt{2 + \sqrt{3}}\) can sometimes be simplified by recognizing patterns or using special identities.
These special cases highlight the importance of recognizing patterns and applying the appropriate rules for simplifying radical expressions efficiently.
Applications of Radical Simplification
Radical simplification has a wide range of applications in various fields, making it an essential skill to master. Here are some key areas where simplified radicals are utilized:
-
Mathematics and Algebra:
Simplified radicals are frequently used in algebra to make expressions more manageable. This is particularly important when solving equations, performing arithmetic operations, and factoring. For example, simplifying expressions such as \( \sqrt{50} \) to \( 5\sqrt{2} \) helps in combining like terms and solving algebraic equations more efficiently.
-
Geometry:
In geometry, radicals are often used to express lengths and areas. For instance, the length of the diagonal of a square with side length \( s \) is given by \( s\sqrt{2} \). Simplifying these expressions aids in precise calculations and clearer communication of geometric properties.
-
Physics:
Physics problems frequently involve radicals, especially when dealing with equations of motion, energy, and wave functions. Simplified radicals allow for easier manipulation of formulas and clearer interpretation of physical quantities. For example, the expression for the period of a pendulum, \( T = 2\pi\sqrt{\frac{L}{g}} \), involves the square root, and simplifying any radicals in the calculation process can lead to more straightforward results.
-
Engineering:
In engineering, radicals appear in calculations involving dimensions, forces, and other physical properties. Simplified radicals are crucial for making these calculations more manageable and reducing the potential for errors. For example, in electrical engineering, the impedance of a circuit containing inductors and capacitors is often expressed with radicals, and simplifying these expressions helps in designing and analyzing circuits.
-
Computer Science:
Radical simplification is used in algorithms and computer graphics. For example, in computer graphics, calculating distances and transformations often involves radicals, and simplifying these expressions can optimize performance and accuracy in rendering images and animations.
By understanding and applying radical simplification, one can solve complex problems more effectively across various disciplines, making it a valuable skill in both academic and professional contexts.

Common Mistakes to Avoid
Simplifying radical expressions can be tricky, and it's easy to make mistakes. Here are some common errors and how to avoid them:
- Not Fully Simplifying Radicals: Ensure all factors are simplified to their smallest possible form. For example, \(\sqrt{18}\) should be simplified to \(3\sqrt{2}\), not left as is.
- Incorrectly Handling Variables: Remember to apply the same simplification rules to variables. For instance, \(\sqrt{x^4} = x^2\), not \(x\sqrt{x}\).
- Adding/Subtracting Radicals Incorrectly: Only like radicals can be added or subtracted. For example, \(\sqrt{2} + \sqrt{8}\) should be simplified to \(\sqrt{2} + 2\sqrt{2} = 3\sqrt{2}\).
- Ignoring Absolute Values: When dealing with even roots, consider the absolute value. For instance, \(\sqrt{x^2} = |x|\), not just \(x\).
- Forgetting to Rationalize the Denominator: Always rationalize the denominator if it contains a radical. For example, \(\frac{1}{\sqrt{2}}\) should be simplified to \(\frac{\sqrt{2}}{2}\).
By keeping these common mistakes in mind and double-checking your work, you can avoid errors and ensure your radical expressions are correctly simplified.
Conclusion and Further Resources
Simplifying radical expressions with variables is a fundamental skill in algebra that aids in understanding more complex mathematical concepts. By mastering the step-by-step simplification process, recognizing special cases, and using calculators effectively, you can handle a wide range of problems involving radicals.
As we've seen, the process involves breaking down the radicand, applying the rules of exponents, and combining like terms to achieve the simplest form. This not only makes the expressions more manageable but also reveals deeper insights into their properties and relationships.
For those who want to explore further, here are some additional resources and tools:
- : An online tool and resource for understanding and simplifying radical expressions.
- : A calculator that simplifies radical expressions quickly and accurately.
- : Video tutorials and practice problems to reinforce your understanding.
Continued practice and use of these resources will help solidify your skills in simplifying radical expressions. Remember, mathematics is a journey, and every step you take in mastering these concepts brings you closer to a deeper appreciation and understanding of the subject.
Happy learning!
Hướng dẫn chi tiết về cách đơn giản hóa biểu thức căn với biến, phù hợp cho người học toán.
Đơn giản hóa biểu thức căn với biến
READ MORE:
Hướng dẫn cách đơn giản hóa căn bậc hai với biến, phù hợp cho người học toán.
Căn bậc hai với biến (Đơn giản hóa Toán học)