5 Square Root 2: Unlocking Its Secrets and Applications

The square root of 2, denoted as \( \sqrt{2} \), is an irrational number approximately equal to 1.414. When multiplied by 5, \( 5\sqrt{2} \) equals approximately 7.071. Here's a summary of the search results:

• Mathematical Definition: \( 5\sqrt{2} = 5 \times \sqrt{2} \).
• Numerical Value: Approximately \( 7.071 \).
• Explanation: \( 5\sqrt{2} \) represents 5 times the square root of 2, often used in mathematical calculations involving geometry, physics, and engineering.

For more detailed information or specific applications, further exploration may be required.

Square roots are mathematical functions that determine the value which, when multiplied by itself, equals the original number. The square root is symbolized by the radical sign (√). For example, the square root of 9 is 3 because 3 x 3 = 9. Square roots are essential in various areas of mathematics, including algebra, geometry, and calculus.

The concept of square roots can be traced back to ancient civilizations, where it was used to solve geometric problems and equations. The Pythagoreans of ancient Greece are among the earliest known groups to study square roots in detail. Today, square roots are fundamental in numerous mathematical applications and are widely taught in schools and universities.

Here are some key points about square roots:

• Every positive real number has two square roots: one positive and one negative. For example, the square roots of 16 are 4 and -4.
• The square root of zero is zero.
• Negative numbers do not have real square roots, as no real number multiplied by itself can result in a negative number. Instead, they have complex square roots.

Square roots are used in various real-life applications, such as:

• Calculating distances in geometry, such as the length of the diagonal of a square.
• Determining standard deviations in statistics, which measure the amount of variation or dispersion in a set of values.
• Solving quadratic equations in algebra, which are equations of the form ax² + bx + c = 0.

In summary, square roots are a crucial mathematical tool that helps us understand and solve problems involving areas, distances, and equations. As we delve deeper into the topic, we will explore specific applications and calculations involving square roots, including the intriguing example of 5√2.

In mathematics, the square root of a number \( x \) is a value that, when multiplied by itself, gives \( x \). The square root is denoted by the radical symbol \( \sqrt{} \). For example, the square root of 25 is 5, written as \( \sqrt{25} = 5 \), because \( 5 \times 5 = 25 \).

More formally, for any non-negative number \( x \), its square root is a number \( y \) such that:

\[ y^2 = x \]

There are several important properties and characteristics of square roots:

• Every positive real number \( x \) has two square roots: a positive square root \( \sqrt{x} \) and a negative square root \( -\sqrt{x} \). For example, the square roots of 9 are \( \sqrt{9} = 3 \) and \( -\sqrt{9} = -3 \).
• The principal square root is the non-negative root, often referred to as \( \sqrt{x} \).
• The square root of zero is zero: \( \sqrt{0} = 0 \).
• Negative numbers do not have real square roots since no real number squared will result in a negative number. Instead, they have imaginary square roots. For example, the square root of -1 is \( i \), where \( i \) is the imaginary unit.

Square roots are not only applicable in pure mathematics but also in practical contexts, including geometry, algebra, and various scientific calculations. Here is a brief overview of square roots in different areas:

• Geometry: Square roots are used to determine lengths of sides in right-angled triangles via the Pythagorean theorem. For instance, if the legs of a right triangle are of lengths 3 and 4, the hypotenuse \( c \) can be found using \( c = \sqrt{3^2 + 4^2} = \sqrt{25} = 5 \).
• Algebra: Solving quadratic equations often requires finding the square roots of numbers. For example, the solutions to \( x^2 = 16 \) are \( x = \pm\sqrt{16} = \pm4 \).
• Physics: Calculations involving kinetic energy, where the speed of an object is derived using square roots, e.g., \( v = \sqrt{\frac{2E}{m}} \), where \( E \) is kinetic energy and \( m \) is mass.

In our specific case, we are interested in the expression \( 5\sqrt{2} \). Here, \( \sqrt{2} \) represents the square root of 2, which is an irrational number approximately equal to 1.414. Thus, \( 5\sqrt{2} \approx 5 \times 1.414 = 7.07 \).

Understanding the square root function and its properties is fundamental to many areas of mathematics and science, providing a foundation for more advanced topics and practical applications.

Square roots possess several important properties that are fundamental to their behavior in mathematics. Understanding these properties helps in simplifying expressions and solving equations involving square roots. Here are some key properties of square roots:

• Non-Negativity: The principal square root of a non-negative number is always non-negative. For any \( x \geq 0 \), \( \sqrt{x} \geq 0 \).
• Product Property: The square root of a product is the product of the square roots. For any non-negative numbers \( a \) and \( b \): \[ \sqrt{a \cdot b} = \sqrt{a} \cdot \sqrt{b} \] This property allows for the simplification of square roots involving multiplication.
• Quotient Property: The square root of a quotient is the quotient of the square roots. For any non-negative numbers \( a \) and \( b \) where \( b \neq 0 \): \[ \sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}} \] This property is useful in simplifying expressions involving division.
• Exponent Property: The square root of a number can be expressed as an exponent. For any non-negative number \( x \): \[ \sqrt{x} = x^{\frac{1}{2}} \] This property is helpful in calculus and algebra when working with powers and roots.
• Square Property: Squaring a square root returns the original number. For any non-negative number \( x \): \[ (\sqrt{x})^2 = x \] This property ensures that taking the square root and then squaring a number returns the original value.
• Addition and Subtraction: Unlike multiplication and division, the square root of a sum or difference is not equal to the sum or difference of the square roots. For example: \[ \sqrt{a + b} \neq \sqrt{a} + \sqrt{b} \] \[ \sqrt{a - b} \neq \sqrt{a} - \sqrt{b} \] Therefore, special techniques are needed to handle addition and subtraction under the radical sign.

Let's apply some of these properties to the expression \( 5\sqrt{2} \). Here, we have a product of 5 and \( \sqrt{2} \). Using the properties, we can understand that:

• The expression \( 5\sqrt{2} \) can be viewed as \( 5 \times \sqrt{2} \).
• The value of \( \sqrt{2} \) is approximately 1.414, so: \[ 5\sqrt{2} \approx 5 \times 1.414 = 7.07 \]

These properties of square roots are essential for simplifying mathematical expressions and solving complex equations. By mastering these properties, we can handle a wide range of mathematical problems involving square roots with confidence and precision.

The expression \( 5\sqrt{2} \) represents a number that is five times the square root of 2. To understand this expression, we need to break it down into its components and then combine them according to the rules of arithmetic and properties of square roots.

First, let's consider the square root of 2:

• \( \sqrt{2} \) is an irrational number, meaning it cannot be expressed as a simple fraction. Its approximate decimal value is 1.414.
• The exact value of \( \sqrt{2} \) continues infinitely without repeating, which is characteristic of irrational numbers.

Next, we multiply this square root by 5:

• Using the approximation \( \sqrt{2} \approx 1.414 \), we can calculate \( 5\sqrt{2} \): \[ 5 \times 1.414 \approx 7.07 \]
• Therefore, \( 5\sqrt{2} \approx 7.07 \) in decimal form.

To better understand the value and implications of \( 5\sqrt{2} \), consider the following steps:

1. Exact Value: The exact value of \( 5\sqrt{2} \) is best represented in its original radical form, as any decimal approximation will be inexact: \[ 5\sqrt{2} \]
2. Decimal Approximation: For practical purposes, we often use a decimal approximation. Using a calculator or software, we can find: \[ 5\sqrt{2} \approx 7.07106781187 \]
3. Visual Representation: Imagine a right triangle with legs of length 1. The hypotenuse of such a triangle is \( \sqrt{2} \). If we scale this triangle so the hypotenuse is 5 times longer, its length becomes \( 5\sqrt{2} \).

Understanding \( 5\sqrt{2} \) also involves recognizing its applications in various fields:

• Geometry: In geometry, \( 5\sqrt{2} \) might represent a length, diagonal, or other measurement in a geometric shape, especially in problems involving right triangles and Pythagorean theorem.
• Algebra: In algebra, expressions like \( 5\sqrt{2} \) frequently appear in the solutions of quadratic equations and other algebraic problems.
• Physics: In physics, this value could represent a magnitude of a vector, distance, or another physical quantity, scaled by the factor of the square root of 2.

By examining both the exact and approximate values, as well as understanding its application in various contexts, we gain a comprehensive understanding of \( 5\sqrt{2} \). This expression exemplifies how mathematical concepts can be both abstract and highly practical.

Calculating \( 5\sqrt{2} \) involves a straightforward process. We'll break it down step by step, ensuring clarity at each stage of the calculation.

1. Identify the Components:
   • The expression \( 5\sqrt{2} \) consists of two parts: the coefficient 5 and the square root of 2.
   • \( \sqrt{2} \) is an irrational number approximately equal to 1.414213562.
2. Calculate the Square Root of 2:
   • Using a calculator, find the value of \( \sqrt{2} \): \[ \sqrt{2} \approx 1.414213562 \]
3. Multiply by the Coefficient:
   • Next, multiply the value of \( \sqrt{2} \) by the coefficient 5: \[ 5 \times \sqrt{2} \approx 5 \times 1.414213562 \]
   • Perform the multiplication: \[ 5 \times 1.414213562 \approx 7.07106781 \]

To summarize, the steps are:

1. Identify the components of the expression \( 5\sqrt{2} \).
2. Calculate \( \sqrt{2} \) using a calculator or approximation.
3. Multiply the result by 5 to obtain the final value.

The exact value of \( 5\sqrt{2} \) is best left in its radical form:
\[ 5\sqrt{2} \]

The approximate decimal value of \( 5\sqrt{2} \) is:
\[ 5\sqrt{2} \approx 7.07106781 \]

This calculation can be verified with a calculator or software to ensure accuracy. Understanding these steps is fundamental to performing similar operations with other square root expressions.

Square roots are used extensively in various real-life applications across different fields, making them an essential mathematical concept. Here are some notable applications:

• Geometry and Trigonometry:
  • Calculating distances: The distance between two points in a plane can be determined using the distance formula, which involves square roots: \[ \text{Distance} = \sqrt{(x_2 - x_1)^2 + (y_2 - y_1)^2} \]
  • Right triangles: The Pythagorean theorem uses square roots to find the length of the hypotenuse: \[ c = \sqrt{a^2 + b^2} \]
• Physics:
  • Kinematics: Square roots are used to calculate the magnitude of velocity and displacement in kinematic equations.
  • Wave speed: The speed of waves on a string can be determined using the square root of the tension divided by the linear mass density: \[ v = \sqrt{\frac{T}{\mu}} \]
• Engineering:
  • Stress and strain: Calculations involving material stress and strain often use square roots to determine critical values.
  • Electrical engineering: The root mean square (RMS) value of an alternating current (AC) is calculated using square roots: \[ I_{RMS} = \sqrt{\frac{1}{T} \int_0^T i(t)^2 \, dt} \]
• Statistics:
  • Standard deviation: A key measure of variability in a data set, standard deviation, is calculated using the square root of the variance: \[ \sigma = \sqrt{\frac{1}{N} \sum_{i=1}^N (x_i - \mu)^2} \]
• Finance:
  • Compound interest: The formula for compound interest involves square roots when solving for the rate or time period: \[ A = P \left(1 + \frac{r}{n}\right)^{nt} \]
  • Volatility: The volatility of an asset, which indicates the risk, is often expressed as the square root of the variance of the asset's returns.

These applications demonstrate the versatility and importance of square roots in solving practical problems across various disciplines. Understanding square roots not only enhances mathematical proficiency but also provides valuable tools for real-world problem-solving.

The expression \( 5\sqrt{2} \) frequently appears in geometric contexts, particularly when dealing with right triangles, diagonal lengths, and scaling figures. Understanding \( 5\sqrt{2} \) in geometry can be achieved through several key examples and applications:

1. Diagonal of a Square:
   • The diagonal of a square can be calculated using the Pythagorean theorem. For a square with side length \( s \), the diagonal \( d \) is: \[ d = s\sqrt{2} \]
   • For a square with a side length of 5 units, the diagonal is: \[ d = 5\sqrt{2} \]
   • This diagonal measures approximately: \[ d \approx 5 \times 1.414 = 7.07 \text{ units} \]
2. Right Triangles:
   • In an isosceles right triangle (45-45-90 triangle), the legs are equal, and the hypotenuse is \( \sqrt{2} \) times the length of each leg.
   • If each leg of the triangle is 5 units, the hypotenuse \( h \) is: \[ h = 5\sqrt{2} \]
   • This hypotenuse length is: \[ h \approx 7.07 \text{ units} \]
3. Scaling Figures:
   • When scaling geometric figures, dimensions often involve square roots. For example, scaling a shape's sides by \( \sqrt{2} \) results in new dimensions proportional to \( \sqrt{2} \) times the original.
   • Scaling a line segment of 5 units by \( \sqrt{2} \) gives a new length of: \[ 5\sqrt{2} \]
4. Coordinate Geometry:
   • In coordinate geometry, the distance between two points \((x_1, y_1)\) and \((x_2, y_2)\) is given by: \[ d = \sqrt{(x_2 - x_1)^2 + (y_2 - y_1)^2} \]
   • For points such that the squared differences sum to \( 50 \): \[ d = \sqrt{50} = 5\sqrt{2} \]
   • This distance is: \[ d \approx 7.07 \text{ units} \]

These examples illustrate how \( 5\sqrt{2} \) is used in various geometric scenarios. Understanding this expression enables accurate calculations and deeper insights into geometric relationships and properties.

The expression \( 5\sqrt{2} \) is commonly encountered in algebraic problems, particularly those involving radicals, quadratic equations, and simplifications. Understanding its use in algebra involves various operations and concepts:

1. Simplifying Radicals:
   • The expression \( 5\sqrt{2} \) is already in its simplest radical form, where 5 is the coefficient and \( \sqrt{2} \) is the radical.
2. Operations with Radicals:
   • When adding or subtracting like radicals, you combine the coefficients. For example: \[ 5\sqrt{2} + 3\sqrt{2} = (5 + 3)\sqrt{2} = 8\sqrt{2} \] \[ 5\sqrt{2} - 2\sqrt{2} = (5 - 2)\sqrt{2} = 3\sqrt{2} \]
   • When multiplying radicals, you multiply the coefficients and the radicals separately: \[ 5\sqrt{2} \times 2\sqrt{3} = (5 \times 2)(\sqrt{2} \times \sqrt{3}) = 10\sqrt{6} \]
3. Quadratic Equations:
   • In quadratic equations, solutions often involve square roots. For instance, consider the equation: \[ x^2 = 50 \]
   • The solutions are: \[ x = \pm \sqrt{50} = \pm 5\sqrt{2} \]
   • Thus, \( 5\sqrt{2} \) and \( -5\sqrt{2} \) are roots of the quadratic equation \( x^2 - 50 = 0 \).
4. Rationalizing the Denominator:
   • When a denominator contains a square root, it can be rationalized by multiplying the numerator and denominator by the conjugate. For example: \[ \frac{1}{5\sqrt{2}} \] \[ \frac{1}{5\sqrt{2}} \times \frac{\sqrt{2}}{\sqrt{2}} = \frac{\sqrt{2}}{5 \times 2} = \frac{\sqrt{2}}{10} \]
5. Solving Radical Equations:
   • Equations involving radicals can be solved by isolating the radical and then squaring both sides. For instance: \[ 5\sqrt{2x} = 10 \]
   • First, isolate the radical: \[ \sqrt{2x} = 2 \]
   • Then square both sides to eliminate the square root: \[ 2x = 4 \]
   • Finally, solve for \( x \): \[ x = 2 \]

These examples highlight the versatility of \( 5\sqrt{2} \) in algebraic operations. Mastering these concepts enables efficient handling of various algebraic problems involving radicals.

Understanding square roots is fundamental in mathematics, yet there are several common misconceptions that often arise. Clarifying these can help solidify your comprehension.

• Misconception 1: Square Roots Are Always Positive

  While the principal square root is positive, every positive number actually has two square roots: one positive and one negative. For example, the square roots of 9 are 3 and -3.

  \[\sqrt{9} = 3 \quad \text{and} \quad -\sqrt{9} = -3\]

• Misconception 2: Square Roots of Negative Numbers Don't Exist

  In the set of real numbers, negative numbers don't have real square roots. However, in the set of complex numbers, they do. The square root of -1 is represented by the imaginary unit \(i\).

  \[\sqrt{-1} = i\]

• Misconception 3: The Square Root of a Sum Is the Sum of the Square Roots

  This is not true. The square root of a sum is not the same as the sum of the square roots.

  \[\sqrt{a + b} \neq \sqrt{a} + \sqrt{b}\]

  For example, \(\sqrt{4 + 9} = \sqrt{13} \neq \sqrt{4} + \sqrt{9} = 2 + 3 = 5\).

• Misconception 4: All Square Roots Are Rational Numbers

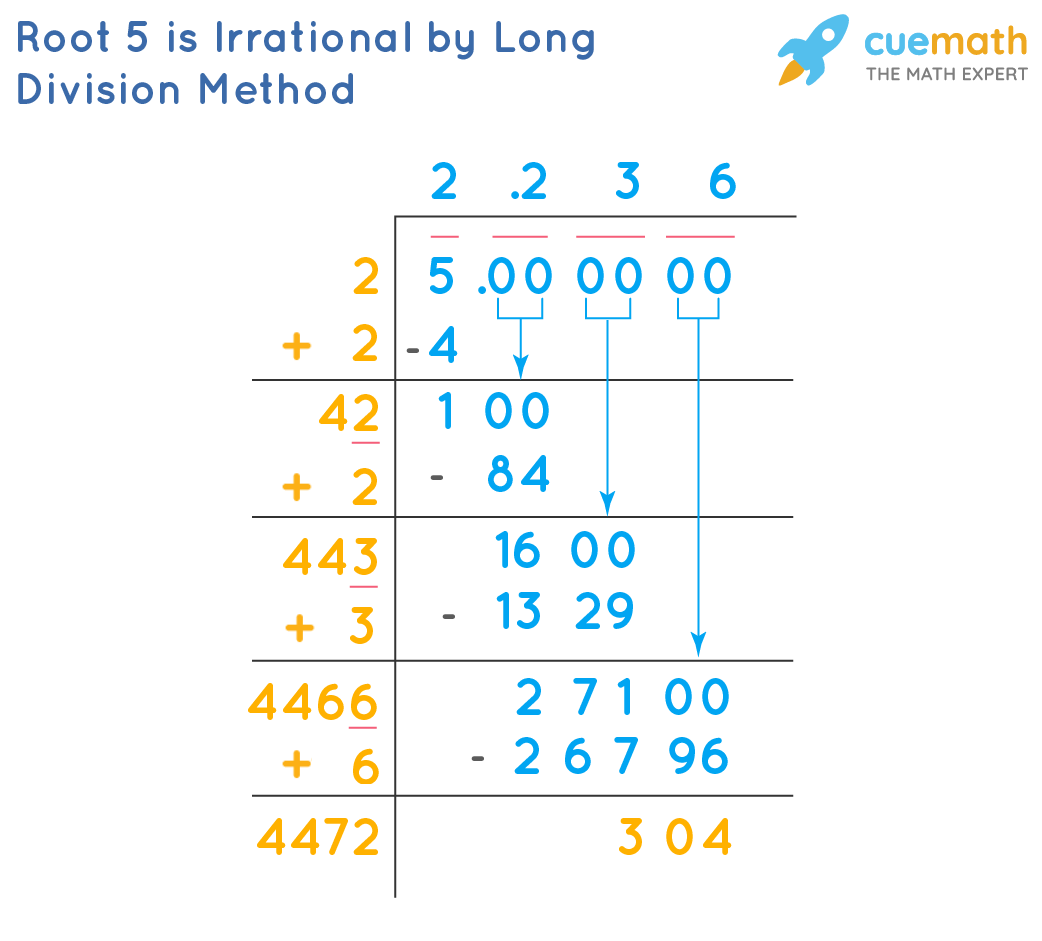
  Not all square roots are rational. A rational number can be expressed as the quotient of two integers, but many square roots are irrational. For instance, \(\sqrt{2}\) is an irrational number.

  \[\sqrt{2} \approx 1.414213\ldots\]

• Misconception 5: Simplifying Square Roots Always Results in a Smaller Number

  Simplifying a square root means expressing it in its simplest radical form, but this doesn't always result in a smaller numerical value. For example, \(\sqrt{50}\) simplifies to \(5\sqrt{2}\), where both expressions are different forms of the same value.

  \[\sqrt{50} = 5\sqrt{2}\]

Understanding square roots can be challenging. Here are some frequently asked questions and detailed answers to help you grasp the concept better:

• What is a square root?

  The square root of a number is a value that, when multiplied by itself, gives the original number. For example, the square root of 25 is 5, because \(5 \times 5 = 25\).

• What is the square root of 2?

  The square root of 2 is an irrational number, approximately equal to 1.414. It cannot be expressed exactly as a fraction or a terminating or repeating decimal.

• How do you calculate 5 times the square root of 2?

  To calculate \(5 \sqrt{2}\), you multiply 5 by the approximate value of \(\sqrt{2}\). This is \(5 \times 1.414 = 7.07\).

• Why is \(\sqrt{2}\) considered irrational?

  A number is considered irrational if it cannot be expressed as a ratio of two integers. The decimal expansion of \(\sqrt{2}\) is non-terminating and non-repeating, which means it cannot be written as a simple fraction.

• What are some properties of square roots?
  • The square root of a product: \(\sqrt{ab} = \sqrt{a} \times \sqrt{b}\)
  • The square root of a quotient: \(\sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}}\)
  • Square root of a square: \(\sqrt{a^2} = |a|\)
• How are square roots used in real life?

  Square roots are used in various fields including engineering, physics, and finance. For example, they are used in calculating areas, standard deviations, and in solving quadratic equations.

• What are common misconceptions about square roots?

  One common misconception is that the square root of a negative number is not possible. In fact, it leads to imaginary numbers. Another misconception is that all square roots are rational, which is not true.

• What is an imaginary number?

  An imaginary number is a number that when squared gives a negative result. The most basic imaginary number is \(i\), where \(i = \sqrt{-1}\).

• What is the difference between a perfect square and a non-perfect square?

  A perfect square is a number that has an integer as its square root, such as 1, 4, 9, 16, etc. A non-perfect square does not have an integer as its square root, and its square root is an irrational number.

The concept of square roots is fundamental in mathematics, offering insight into various areas such as algebra, geometry, and real-life applications. Throughout this guide, we have explored the mathematical definition of square roots, their properties, and specific applications of the expression \(5\sqrt{2}\).

To summarize, the square root of 2 is an irrational number, approximately equal to 1.414, and when multiplied by 5, it results in \(5\sqrt{2}\), which is roughly 7.071. This expression appears frequently in various mathematical contexts, especially in simplifying radicals and solving equations.

Understanding how to manipulate and apply square roots can greatly enhance one's ability to solve complex mathematical problems. We discussed the process of calculating square roots, including using tools like calculators and iterative methods such as Heron's method for approximating roots.

Moreover, \(5\sqrt{2}\) has practical applications in fields like engineering and physics, where precise measurements and calculations are crucial. For instance, it often emerges in calculations involving diagonal lengths in geometry, where the Pythagorean theorem applies.

In conclusion, mastering square roots and their properties is essential for anyone looking to deepen their mathematical knowledge. The expression \(5\sqrt{2}\) serves as a prime example of how understanding square roots can simplify and solve real-world problems.

We hope this comprehensive guide has provided you with a clear understanding of square roots and the specific intricacies of \(5\sqrt{2}\). Continue exploring and applying these concepts to enhance your mathematical skills and problem-solving abilities.