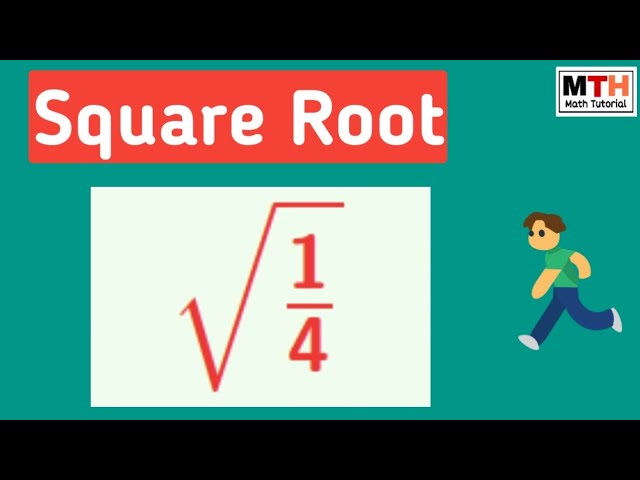
Topic 1/4 square root: The 1/4 square root is a fundamental mathematical concept with diverse applications in geometry, finance, and statistics. This article delves into the properties, calculations, and practical uses of the 1/4 square root, providing readers with a comprehensive understanding of its significance and how it can be applied to solve real-world problems.
Table of Content
- Understanding the Concept of 1/4 and the Square Root
- Introduction to 1/4 and Square Roots
- Definition and Properties of the Fraction 1/4
- Understanding Square Roots
- Mathematical Calculation of the Square Root of 1/4
- Properties of the Square Root of 1/4
- Geometric Interpretation of 1/4 and Its Square Root
- Practical Applications in Various Fields
- 1/4 and Its Square Root in Geometry
- 1/4 and Its Square Root in Statistics
- 1/4 and Its Square Root in Finance
- Comparative Analysis of 1/4 with Other Fractions and Their Roots
- Visual Representations and Graphs
- Common Misconceptions and Clarifications
- Exercises and Practice Problems
- Conclusion and Summary
- References and Further Reading
- YOUTUBE:
Understanding the Concept of 1/4 and the Square Root
In mathematics, fractions and roots are fundamental concepts that frequently appear in various calculations and problem-solving scenarios. This guide explores the relationship and properties of the fraction 1/4 and the square root function.
What is 1/4?
The fraction 1/4 represents one part of a whole divided into four equal parts. It is equivalent to 0.25 in decimal form. It is often used in various fields such as finance, measurement, and statistics.
Square Root
The square root of a number x is a value that, when multiplied by itself, gives the number x. It is denoted by the symbol √. For example, the square root of 16 is 4, because 4 × 4 = 16.
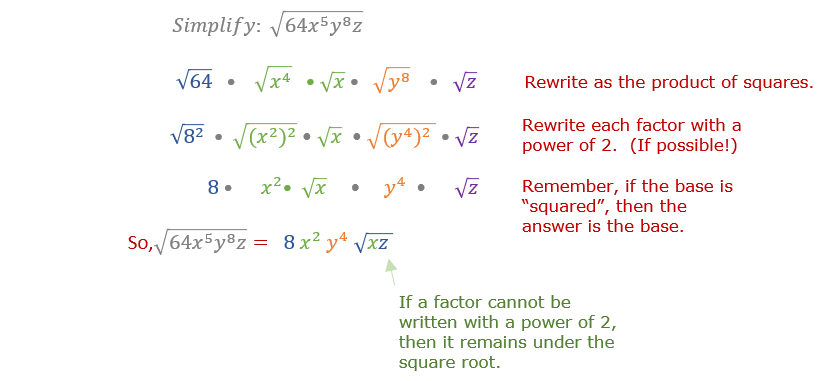
Calculating the Square Root of 1/4
To find the square root of 1/4, you can use the property of square roots:
\[
\sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}}
\]
Applying this property to 1/4:
\[
\sqrt{\frac{1}{4}} = \frac{\sqrt{1}}{\sqrt{4}} = \frac{1}{2} = 0.5
\]
Properties of 1/4 and Its Square Root
- The fraction
1/4is less than 1, representing a small portion of a whole. - The square root of
1/4is0.5, indicating that taking the square root of a fraction less than 1 results in a larger number than the original fraction. - Both
1/4and its square root,0.5, are commonly used in practical applications, including geometry and probability.
Practical Applications
Understanding fractions and their square roots is essential in various fields:
- Geometry: In geometry, understanding the square root of areas and other quantities is crucial for solving problems related to shapes and sizes.
- Statistics: Fractions and square roots are used to calculate probabilities, averages, and standard deviations.
- Finance: In finance, fractions like
1/4are used to represent interest rates, growth rates, and portions of investments.
Conclusion
The fraction 1/4 and its square root are basic yet powerful mathematical concepts. Understanding how to manipulate and apply these values is fundamental to mastering more complex mathematical and real-world problems.
READ MORE:
Introduction to 1/4 and Square Roots
The concepts of fractions and square roots are foundational in mathematics. Understanding these concepts is crucial for tackling more complex mathematical problems and real-world applications. This section provides an introduction to the fraction 1/4 and the concept of square roots, explaining their definitions, properties, and calculations.
Fraction 1/4:
- The fraction 1/4 represents one part of a whole divided into four equal parts.
- In decimal form, 1/4 is equivalent to 0.25.
- It is commonly used in various fields such as finance, measurement, and everyday life to represent quarters.
Square Roots:
- The square root of a number x is a value that, when multiplied by itself, gives the number x.
- It is denoted by the symbol \(\sqrt{}\).
- For example, the square root of 16 is 4 because \(4 \times 4 = 16\).
Calculating the Square Root of 1/4:
To find the square root of 1/4, we can use the property of square roots that states:
\[
\sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}}
\]
Applying this property to 1/4:
\[
\sqrt{\frac{1}{4}} = \frac{\sqrt{1}}{\sqrt{4}} = \frac{1}{2} = 0.5
\]
Properties of 1/4 and Its Square Root:
- The fraction 1/4 is less than 1, representing a small portion of a whole.
- The square root of 1/4 is 0.5, indicating that the square root of a fraction less than 1 results in a larger value than the original fraction.
- Both 1/4 and its square root, 0.5, are frequently used in practical applications, including geometry and probability.
Definition and Properties of the Fraction 1/4
The fraction 1/4, also known as one-quarter, represents a division of a whole into four equal parts. It is a fundamental concept in mathematics and is widely used in various applications. Understanding its definition and properties is essential for grasping more complex mathematical ideas.
Definition of 1/4:
- The fraction 1/4 is written as \(\frac{1}{4}\).
- It signifies one part of a whole that is divided into four equal sections.
- In decimal form, 1/4 is represented as 0.25.
- In percentage terms, 1/4 is equivalent to 25%.
Properties of 1/4:
- Equality: The fraction 1/4 is equal to other fractions such as 2/8 and 3/12 when they are simplified. This is due to the property of equivalent fractions.
- Addition: When adding fractions with a common denominator, 1/4 + 1/4 = 2/4, which simplifies to 1/2.
- Subtraction: Similarly, subtracting fractions with a common denominator, 1/4 - 1/4 = 0.
- Multiplication: Multiplying 1/4 by another fraction involves multiplying the numerators and denominators: \(\frac{1}{4} \times \frac{1}{2} = \frac{1 \times 1}{4 \times 2} = \frac{1}{8}\).
- Division: Dividing by 1/4 involves multiplying by the reciprocal: \(\frac{1}{4} \div \frac{1}{2} = \frac{1}{4} \times \frac{2}{1} = \frac{1 \times 2}{4 \times 1} = \frac{2}{4} = \frac{1}{2}\).
Visualization of 1/4:
The fraction 1/4 can be visualized using various methods:
- In a circle, 1/4 represents one quarter of the circle, typically illustrated as one of the four equal sectors.
- In a rectangle or square, 1/4 can be shown as one of four equal smaller rectangles or squares.
- In a set of objects, 1/4 of the set would mean selecting one object from every four.
These properties and visualizations of the fraction 1/4 demonstrate its importance and utility in mathematics. By understanding these fundamental concepts, one can apply 1/4 in various mathematical and real-world scenarios with confidence.
Understanding Square Roots
The square root of a number is a value that, when multiplied by itself, gives the original number. This operation is the inverse of squaring a number. The symbol for the square root is √, known as the radical sign.
For example, the square root of 9 is 3, since 3 × 3 = 9. This can be written as:
\[ \sqrt{9} = 3 \]
Square roots have several important properties and applications in mathematics:
- Principal Square Root: For any non-negative number \( x \), the principal square root is the non-negative root. For example, the principal square root of 25 is 5.
- Two Roots: Every positive number has two square roots: one positive and one negative. For instance, both 5 and -5 are square roots of 25 because \( 5^2 = 25 \) and \( (-5)^2 = 25 \). However, by convention, the square root symbol (√) refers to the principal (positive) root.
Mathematically, this is represented as:
\[ \sqrt{a^2} = |a| \]
where \( |a| \) denotes the absolute value of \( a \).
When dealing with fractions, the square root can be applied to both the numerator and the denominator separately. For example, the square root of \( \frac{1}{4} \) is calculated as follows:
\[ \sqrt{\frac{1}{4}} = \sqrt{\frac{1^2}{2^2}} = \frac{\sqrt{1^2}}{\sqrt{2^2}} = \frac{1}{2} \]
This simplification utilizes the property:
\[ \sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}} \]
Understanding square roots is fundamental in solving quadratic equations, performing geometric calculations, and analyzing various real-world scenarios where relationships between squared quantities are involved.
Mathematical Calculation of the Square Root of 1/4
Calculating the square root of a fraction, such as \( \frac{1}{4} \), involves a few straightforward steps. Here is the detailed process:
- Express the Fraction:
We start with the fraction \( \frac{1}{4} \).
- Apply the Square Root to Both Numerator and Denominator:
According to the properties of square roots, the square root of a fraction can be written as the square root of the numerator divided by the square root of the denominator:
\[
\sqrt{\frac{1}{4}} = \frac{\sqrt{1}}{\sqrt{4}}
\] - Calculate the Square Roots:
Next, we find the square roots of the numerator and the denominator separately:
- The square root of 1 is 1, since \( 1 \times 1 = 1 \).
- The square root of 4 is 2, since \( 2 \times 2 = 4 \).
So, we have:
\[
\frac{\sqrt{1}}{\sqrt{4}} = \frac{1}{2}
\] - Conclusion:
The square root of \( \frac{1}{4} \) simplifies to \( \frac{1}{2} \). Thus,
\[
\sqrt{\frac{1}{4}} = \frac{1}{2}
\]
This calculation shows that the principal square root of \( \frac{1}{4} \) is \( \frac{1}{2} \). This method can be applied to any fraction where both the numerator and the denominator are perfect squares.

Properties of the Square Root of 1/4
The square root of \( \frac{1}{4} \), denoted as \( \sqrt{\frac{1}{4}} \), has several important mathematical properties. Here is a detailed exploration:
- Principal Square Root:
The principal (positive) square root of \( \frac{1}{4} \) is \( \frac{1}{2} \), since:
\[
\sqrt{\frac{1}{4}} = \frac{\sqrt{1}}{\sqrt{4}} = \frac{1}{2}
\] - Positive and Negative Roots:
Every positive number has two square roots: one positive and one negative. Thus, \( \frac{1}{4} \) has two square roots:
\[
\sqrt{\frac{1}{4}} = \pm \frac{1}{2}
\]This means \( \frac{1}{2} \) and \( -\frac{1}{2} \) are both roots of \( \frac{1}{4} \).
- Property of Fractions:
For any fraction \( \frac{a}{b} \), the square root can be expressed as the quotient of the square roots of the numerator and the denominator:
\[
\sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}}
\] - Exponential Form:
The square root can be expressed in exponential form. For \( \frac{1}{4} \), this is:
\[
\left(\frac{1}{4}\right)^{\frac{1}{2}} = \left(1^2 \cdot \frac{1}{2^2}\right)^{\frac{1}{2}} = \left(\frac{1}{2}\right)^2 = \frac{1}{2}
\] - Rational Number:
The square root of \( \frac{1}{4} \) is a rational number because both the numerator and the denominator are perfect squares. This results in a rational square root, \( \frac{1}{2} \).
- Multiplication and Division:
The properties of multiplication and division apply to square roots. For example:
- Multiplication: \[ \sqrt{\frac{1}{4} \times 4} = \sqrt{1} = 1 \]
- Division: \[ \sqrt{\frac{\frac{1}{4}}{4}} = \sqrt{\frac{1}{16}} = \frac{1}{4} \]
These properties highlight the versatility and applicability of square roots in mathematical operations and problem-solving.
Geometric Interpretation of 1/4 and Its Square Root
The geometric interpretation of the fraction \( \frac{1}{4} \) and its square root can be visualized through areas and lengths in geometry. Here's a detailed, step-by-step explanation:
- Representation of \( \frac{1}{4} \) as an Area:
Consider a unit square with side length 1. The area of this square is \( 1 \times 1 = 1 \) square unit. To represent \( \frac{1}{4} \), we need a square whose area is \( \frac{1}{4} \) of the unit square. This means dividing the unit square into four equal smaller squares.
\[
\text{Area of one smaller square} = \frac{1}{4} \times 1 = \frac{1}{4} \text{ square unit}
\] - Finding the Side Length of the Smaller Square:
Since the area of the smaller square is \( \frac{1}{4} \), the side length of this smaller square can be found by taking the square root of the area.
\[
\text{Side length} = \sqrt{\frac{1}{4}} = \frac{1}{2}
\]This means each side of the smaller square is \( \frac{1}{2} \) unit long.
- Geometric Construction:
To construct this geometrically, draw a unit square. Then, divide this square into four equal smaller squares by drawing lines through the midpoints of the sides of the unit square.
The side length of each smaller square, as calculated, will be \( \frac{1}{2} \) unit. This provides a visual representation of both \( \frac{1}{4} \) as an area and \( \frac{1}{2} \) as the side length of a square with area \( \frac{1}{4} \).
- Geometric Mean Interpretation:
In a broader sense, the square root can be seen as a geometric mean. If you have two segments of lengths \( a \) and \( b \), the geometric mean is given by \( \sqrt{a \cdot b} \). For our case, with \( a = 1 \) and \( b = \frac{1}{4} \), the geometric mean is:
\[
\sqrt{1 \cdot \frac{1}{4}} = \sqrt{\frac{1}{4}} = \frac{1}{2}
This geometric interpretation not only helps in visualizing the relationship between \( \frac{1}{4} \) and its square root but also reinforces the concept of square roots in a tangible way.
Practical Applications in Various Fields
The fraction 1/4 and its square root have numerous practical applications across various fields. Here are some of the key areas where these mathematical concepts are utilized:
-
1. Engineering
In engineering, the fraction 1/4 is often used in calculations involving proportions and scales. For instance, when designing models or prototypes, engineers may use a 1/4 scale to create a smaller, manageable version of a larger structure.
The square root of 1/4, which is 1/2, is also significant in engineering, particularly in areas such as signal processing, where it may be used in algorithms for data compression and noise reduction.
-
2. Physics
In physics, the fraction 1/4 appears in formulas related to energy and force. For example, in electrostatics, the force between two point charges is inversely proportional to the square of the distance between them, which can be represented using fractions like 1/4.
The square root of 1/4 is used in equations related to wave functions and probabilities, particularly in quantum mechanics.
-
3. Statistics
In statistics, 1/4, or 25%, is commonly used to represent the first quartile, which divides the lowest 25% of data from the rest. This is crucial for understanding the distribution of data and identifying outliers.
The square root of 1/4 is used in the calculation of standard deviations and variances, especially when working with normalized data sets.
-
4. Finance
In finance, the fraction 1/4 is frequently used in interest rate calculations, payment schedules, and financial ratios. For example, quarterly interest rates are often calculated as a quarter of the annual rate.
The square root of 1/4 can be applied in risk assessment and portfolio diversification strategies to understand the volatility and correlation between different assets.
-
5. Medicine
In the medical field, the fraction 1/4 is used in dosing calculations, especially when prescribing medication. Physicians often prescribe doses that are a fraction of a standard dose, such as 1/4, to ensure safety and efficacy.
The square root of 1/4 is relevant in statistical analyses of medical data, such as calculating confidence intervals and understanding the variability of clinical trial results.
1/4 and Its Square Root in Geometry
The fraction 1/4 and its square root, 1/2, have several interesting geometric interpretations and applications. Understanding these concepts can enhance comprehension of both fundamental geometry and more advanced geometric principles.
1. Square Area and Side Length
A square with an area of 1/4 square units has sides of length 1/2 units. This relationship is derived from the formula for the area of a square:
\[
\text{Area} = a^2
\]
where \( a \) is the side length. Thus,
\[
\frac{1}{4} = \left( \frac{1}{2} \right)^2
\]
2. Geometric Mean Construction
The square root of a fraction can be constructed geometrically. For example, the square root of 1/4 can be visualized by constructing a right triangle with legs of length 1/2. The hypotenuse of this triangle will have a length of 1, illustrating the geometric mean.
3. Circle and Diameter
Consider a circle with a diameter of 1 unit. A chord of this circle that is 1/2 units long will correspond to the side of a square with area 1/4 when the circle's diameter is used to form the square.
4. Square's Diagonals
The diagonal of a square relates to the square root of its area. For a square with side length \( \frac{1}{2} \):
\[
\text{Diagonal} = \frac{1}{2} \sqrt{2}
\]
This illustrates how the square root of the area influences the diagonal length in geometric constructions.
5. Practical Examples
- Scaling Models: When scaling geometric models, knowing the side lengths and their square roots helps maintain proportional relationships.
- Design and Architecture: Architects use these principles to calculate dimensions in design, ensuring structural integrity and aesthetic harmony.
- Construction: Accurate measurements involving square roots are crucial in construction projects for precise cutting and fitting of materials.
6. Visualizing Square Roots
Visual tools such as grid paper or geometric drawing software can help students and professionals visualize the relationship between a fraction and its square root in geometry.
7. Educational Tools
Using physical tools like compasses and straightedges, one can construct geometric figures to understand the square roots of fractions, fostering a deeper understanding of geometric properties and theorems.
In summary, the geometric interpretation of 1/4 and its square root 1/2 is fundamental in various fields, providing a foundation for more complex geometric and algebraic concepts.
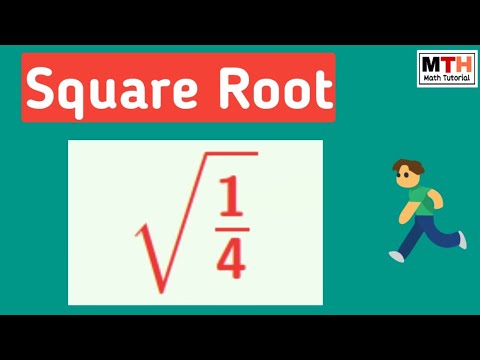
1/4 and Its Square Root in Statistics
The fraction \( \frac{1}{4} \) and its square root \( \frac{1}{2} \) have various practical applications in the field of statistics. These concepts are essential in understanding statistical measures, data transformations, and interpretations.
Variance Stabilizing Transformation
In statistical analysis, particularly with count data, the square root transformation is commonly used to stabilize variance. For instance, if we have a dataset with counts, the variance of these counts often increases with the mean. Applying the square root transformation reduces this dependency, making the data more suitable for analysis.
The transformation is given by:
\[ y' = \sqrt{y} \]
For a count of \( \frac{1}{4} \), its square root is \( \frac{1}{2} \). This transformation helps in normalizing the distribution of the data, making it closer to a normal distribution, which is a common assumption in many statistical methods.
Geometric Mean Calculation
The geometric mean is another statistical measure where the concepts of \( \frac{1}{4} \) and its square root are useful. The geometric mean is appropriate for data that involves multiplication or exponential growth, such as interest rates or growth rates.
To find the geometric mean of a set of values, we use the formula:
\[ \text{Geometric Mean} = \left( \prod_{i=1}^{n} x_i \right)^{\frac{1}{n}} \]
For example, if we have values \( \frac{1}{4}, 1, 4 \), the geometric mean is:
\[ \left( \frac{1}{4} \times 1 \times 4 \right)^{\frac{1}{3}} = (1)^{\frac{1}{3}} = 1 \]
Confidence Intervals
When constructing confidence intervals for proportions or rates, the square root of \( \frac{1}{4} \) plays a role in the formulas used. The standard error of a proportion can be approximated using the square root of the proportion times one minus the proportion, divided by the sample size.
For example, if we are estimating the proportion \( p = \frac{1}{4} \) with a sample size \( n \), the standard error (SE) is:
\[ SE = \sqrt{\frac{p(1-p)}{n}} = \sqrt{\frac{\frac{1}{4} \times \frac{3}{4}}{n}} = \sqrt{\frac{3}{16n}} \]
This standard error is essential for constructing confidence intervals around the estimate of the proportion.
Hypothesis Testing
In hypothesis testing, particularly in tests involving proportions, the square root transformation can help meet the assumptions of the test. For example, when using a chi-square test for independence in a contingency table, the expected frequencies are sometimes transformed using the square root to stabilize the variance and meet the test assumptions.
For a cell with an expected frequency of \( \frac{1}{4} \), the transformed value is \( \sqrt{\frac{1}{4}} = \frac{1}{2} \).
These applications demonstrate the importance and utility of the fraction \( \frac{1}{4} \) and its square root in various statistical contexts, enhancing the accuracy and reliability of statistical analyses.
1/4 and Its Square Root in Finance
Understanding the role of fractions and their square roots is crucial in finance, where precise calculations are essential for investment decisions and risk management. The fraction 1/4 (or 0.25) and its square root (1/2 or 0.5) find several practical applications in various financial contexts.
Investment Return Rate Calculation
The square root function is commonly used to calculate the return rate of an investment over a given period. This is particularly useful for understanding the performance of an investment compounded over multiple years.
The formula to calculate the return rate (R) over two years using the square root is:
\( R = \sqrt{\frac{V_2}{V_0}} - 1 \)
Where:
- \( R \) = Return rate
- \( V_2 \) = Ending value of the investment
- \( V_0 \) = Starting value of the investment
For example, if an investor initially invests $100 (V0) and the investment grows to $196 (V2) after two years, the return rate can be calculated as follows:
\( R = \sqrt{\frac{196}{100}} - 1 = \sqrt{1.96} - 1 = 1.4 - 1 = 0.4 \) or 40%
Volatility and Risk Management
In finance, the square root of time is often used in models to calculate the volatility or risk of an investment over different time periods. This is a key component in the Black-Scholes model for option pricing and the calculation of the Value at Risk (VaR).
For instance, if the annual volatility of a stock is 16%, the volatility over a quarter (3 months) can be approximated using the square root of time rule:
\( \text{Quarterly Volatility} = \text{Annual Volatility} \times \sqrt{\frac{1}{4}} = 0.16 \times \frac{1}{2} = 0.08 \) or 8%
Loan Amortization
The concept of square roots is also applied in the amortization of loans, where understanding the reduction of principal and the accumulation of interest over time requires precise mathematical calculations. The time value of money can often involve square roots when calculating the present and future value of cash flows.
Practical Example: Mortgage Calculation
Consider a scenario where an individual takes a mortgage with quarterly compounding interest. Understanding how payments reduce over time involves square root calculations, especially when transitioning from annual to quarterly or monthly compounding periods.
For a mortgage with an annual interest rate of 4%, compounded quarterly, the effective quarterly interest rate is:
\( \text{Quarterly Interest Rate} = \sqrt[4]{1.04} - 1 \approx 0.00985 \) or 0.985%
Conclusion
The square root of 1/4, which is 1/2, may seem simple but plays a significant role in complex financial calculations. Whether it's determining the return rate of investments, assessing volatility, or calculating loan amortizations, understanding and applying these mathematical concepts is essential for accurate financial analysis and decision-making.
Comparative Analysis of 1/4 with Other Fractions and Their Roots
Understanding the square root of fractions involves comparing the square roots of different fractions to illustrate their properties and simplification methods. This section explores various fractions and their square roots, providing insights into their mathematical behaviors.
-
Fraction: 1/4
The square root of 1/4 can be calculated as follows:
\[\sqrt{\frac{1}{4}} = \frac{\sqrt{1}}{\sqrt{4}} = \frac{1}{2}\]
This simplification shows that the square root of 1/4 is 1/2.
-
Fraction: 9/16
The square root of 9/16 can be calculated as:
\[\sqrt{\frac{9}{16}} = \frac{\sqrt{9}}{\sqrt{16}} = \frac{3}{4}\]
Here, both the numerator and the denominator are perfect squares, making the calculation straightforward.
-
Fraction: 1/25
For 1/25, the square root is:
\[\sqrt{\frac{1}{25}} = \frac{\sqrt{1}}{\sqrt{25}} = \frac{1}{5}\]
Again, since 1 and 25 are perfect squares, the simplification is simple.
-
Fraction: 7/18
Finding the square root of 7/18 involves a few more steps:
\[\sqrt{\frac{7}{18}} = \frac{\sqrt{7}}{\sqrt{18}}\]
Since 18 is not a perfect square, it can be simplified as:
\[\frac{\sqrt{7}}{\sqrt{9 \times 2}} = \frac{\sqrt{7}}{3\sqrt{2}}\]
To rationalize the denominator, multiply the numerator and denominator by \(\sqrt{2}\):
\[\frac{\sqrt{7} \cdot \sqrt{2}}{3\sqrt{2} \cdot \sqrt{2}} = \frac{\sqrt{14}}{6}\]
-
Fraction: 30/32
For 30/32, the square root calculation is:
\[\sqrt{\frac{30}{32}} = \frac{\sqrt{30}}{\sqrt{32}} = \frac{\sqrt{30}}{4\sqrt{2}}\]
Rationalizing the denominator gives:
\[\frac{\sqrt{30} \cdot \sqrt{2}}{4\sqrt{2} \cdot \sqrt{2}} = \frac{\sqrt{60}}{8} = \frac{\sqrt{4 \times 15}}{8} = \frac{2\sqrt{15}}{8} = \frac{\sqrt{15}}{4}\]
This comparative analysis highlights how the properties of the numerator and the denominator affect the simplification process. Simplifying square roots of fractions often involves recognizing perfect squares and rationalizing denominators to achieve the simplest form.
Visual Representations and Graphs
Visual representations and graphs are essential tools for understanding the mathematical concepts of fractions and their square roots. They provide a clear and intuitive way to see the relationships and properties of these numbers.
Graphing the Fraction 1/4
Let's start by plotting the fraction \( \frac{1}{4} \) on a number line. This simple visual helps us understand its position relative to other numbers.
- On a number line, \( \frac{1}{4} \) is located between 0 and 0.5.
- It's a way to visualize that \( \frac{1}{4} \) is one part of four equal divisions between 0 and 1.
Graphing the Square Root of 1/4
Next, let's consider the square root of \( \frac{1}{4} \), which is \( \frac{1}{2} \) or 0.5. Plotting this on the number line provides insight into its magnitude.
- \( \sqrt{\frac{1}{4}} = \frac{1}{2} \), which is exactly halfway between 0 and 1.
Graphing Functions
To better understand these values, we can plot their corresponding functions:
- The function \( y = x \) where \( x = \frac{1}{4} \).
- The function \( y = \sqrt{x} \) evaluated at \( x = \frac{1}{4} \).
Using graphing tools like Desmos or GeoGebra, we can visualize these functions:
| Function | Graph |
|---|---|
| \( y = x \) | |
| \( y = \sqrt{x} \) |
Comparative Visualization
By comparing the graphs of \( y = x \) and \( y = \sqrt{x} \), we see how the square root function compresses the values of \( x \) between 0 and 1 into the interval from 0 to 0.5.
Here are some key observations:
- For \( x = \frac{1}{4} \), \( y = \frac{1}{4} \) on the linear graph, but \( y = \frac{1}{2} \) on the square root graph.
- The square root graph rises more slowly than the linear graph, indicating that square roots grow slower than their corresponding base values.
Geometric Interpretations
Geometric shapes can also help visualize these concepts. Consider a square with an area of \( \frac{1}{4} \) square units:
- The side length of this square is \( \sqrt{\frac{1}{4}} = \frac{1}{2} \) units.
- This visual shows that the square root represents the side length of a square whose area is the original number.
Graphs and visual tools are invaluable for understanding and comparing mathematical concepts, providing clarity and insight that complement numerical and algebraic methods.

Common Misconceptions and Clarifications
Understanding fractions and their square roots can often lead to several misconceptions. Here, we address some common misunderstandings and provide clarifications to help solidify these mathematical concepts.
Misconception 1: Larger Denominators Mean Larger Values
One common mistake is assuming that a larger denominator in a fraction indicates a larger value. For example, some may think that \(\frac{1}{4}\) is larger than \(\frac{1}{2}\) because 4 is greater than 2. However, the opposite is true. The larger the denominator, the smaller the fraction. This is because the whole is divided into more parts, making each part smaller.
Clarification: To understand this, consider dividing a pizza into 2 and 4 equal parts. Each part of the pizza when divided into 4 pieces (\(\frac{1}{4}\)) is smaller than each part when divided into 2 pieces (\(\frac{1}{2}\)).
Misconception 2: The Square Root of a Fraction is Smaller
Another misconception is that the square root of a fraction is always smaller than the original fraction. For example, thinking that \(\sqrt{\frac{1}{4}}\) is less than \(\frac{1}{4}\).
Clarification: The square root of \(\frac{1}{4}\) is \(\frac{1}{2}\), which is actually larger than \(\frac{1}{4}\). This occurs because when taking the square root of a fraction less than 1, the result is a fraction larger than the original fraction but still less than 1.
\[
\sqrt{\frac{1}{4}} = \frac{1}{2}
\]
Misconception 3: Fraction Addition and Subtraction
Adding or subtracting fractions directly without finding a common denominator is another frequent mistake. For example, believing that \(\frac{1}{2} + \frac{1}{4} = \frac{2}{6}\).
Clarification: To add or subtract fractions, you must first find a common denominator. In this case, the common denominator for \(\frac{1}{2}\) and \(\frac{1}{4}\) is 4. Thus, \(\frac{1}{2} = \frac{2}{4}\), and \(\frac{2}{4} + \frac{1}{4} = \frac{3}{4}\).
\[
\frac{1}{2} + \frac{1}{4} = \frac{2}{4} + \frac{1}{4} = \frac{3}{4}
\]
Misconception 4: Misinterpreting Division and Multiplication of Fractions
Some learners struggle to understand why dividing by a fraction is the same as multiplying by its reciprocal. For instance, \(\frac{1}{4} \div \frac{1}{2} = \frac{1}{4} \times 2 = \frac{1}{2}\).
Clarification: Division by a fraction involves multiplying by the reciprocal of that fraction. Hence, \(\frac{1}{4} \div \frac{1}{2}\) becomes \(\frac{1}{4} \times \frac{2}{1} = \frac{1 \times 2}{4 \times 1} = \frac{2}{4} = \frac{1}{2}\).
\[
\frac{1}{4} \div \frac{1}{2} = \frac{1}{4} \times \frac{2}{1} = \frac{2}{4} = \frac{1}{2}
\]
Misconception 5: Misunderstanding the Whole
Students often fail to grasp that fractions are always parts of a whole and that the value of a fraction changes with the size of the whole. For example, half of a small cake is different from half of a large cake.
Clarification: The concept of the 'whole' is crucial. When dealing with fractions, always define the whole first. This helps in understanding that \(\frac{1}{2}\) of one quantity may not be equal to \(\frac{1}{2}\) of another.
Misconception 6: Confusing Natural and Rational Numbers
Natural numbers (like 1, 2, 3) have fixed values, while rational numbers (fractions) are relative to the whole. Students often mistakenly apply the properties of natural numbers to rational numbers.
Clarification: Rational numbers represent a relationship between the numerator and the denominator. For example, \(\frac{3}{4}\) means 3 parts out of 4 equal parts of a whole, which is different from the natural number 3.
Addressing these misconceptions early can greatly improve understanding and application of fractions and their square roots in various mathematical contexts.
Exercises and Practice Problems
To deepen your understanding of the square root of 1/4 and related concepts, here are a variety of exercises and practice problems. These problems range from basic square root calculations to more complex applications involving fractions and quadratic equations.
Basic Square Root Calculations
- Find the square root of the following numbers:
- \(\sqrt{1/4}\)
- \(\sqrt{16}\)
- \(\sqrt{81}\)
- \(\sqrt{100}\)
Intermediate Problems
- Simplify the following expressions:
- \(\sqrt{\frac{1}{4}}\)
- \(\frac{\sqrt{9}}{2}\)
- \(\sqrt{\frac{49}{25}}\)
- \(\frac{5}{\sqrt{25}}\)
- Solve for \(x\):
- \(x^2 = \frac{1}{4}\)
- \(x^2 = 4\)
- \(x^2 - 9 = 0\)
- \(4x^2 - 16 = 0\)
Advanced Problems
- Solve the following quadratic equations:
- \(\frac{1}{2}x^2 + \frac{1}{4}x - \frac{25}{8} = 0\)
- \(x^2 + \frac{14}{8}x - \frac{147}{16} = 0\)
- \(16(8x - 1)(x + 1) = -15\)
- \((11x + 2)(x - 17) = 7x\)
- Find the square roots using the prime factorization method:
- \(\sqrt{144}\)
- \(\sqrt{1024}\)
- \(\sqrt{256}\)
- \(\sqrt{2025}\)
Real-World Applications
- Geometry: Find the side length of a square with an area of 1/4 square units.
- Finance: Calculate the annual interest rate if the amount doubles in four years under continuous compounding (hint: use the formula for compound interest and solve for the rate).
- Statistics: Determine the standard deviation of a dataset where the variance is 1/4.
These exercises will help solidify your understanding of square roots and their applications. Practicing these problems regularly will enhance your problem-solving skills and mathematical fluency.
Conclusion and Summary
In this comprehensive guide, we have explored the fraction 1/4 and its square root from various perspectives. The following points summarize the key takeaways:
- Definition and Properties: The fraction 1/4, representing one part of a whole divided into four equal parts, is a fundamental concept in mathematics.
- Square Root: The square root of 1/4 is calculated as √(1/4), which equals 1/2. This value demonstrates how square roots can simplify fractional expressions.
- Geometric Interpretation: Geometrically, 1/4 can be visualized as a quarter of a square or any other shape. The square root of 1/4, or 1/2, can be interpreted as the length of the side of a square that has an area of 1/4.
- Practical Applications: Both 1/4 and its square root are utilized across various fields including geometry, statistics, and finance, showcasing their versatility and importance.
- Comparative Analysis: Comparing 1/4 with other fractions and their square roots reveals interesting patterns and relationships, deepening our understanding of fractional and root values.
- Visual Representations: Graphs and visual aids help in comprehending the properties and applications of 1/4 and its square root, making complex concepts more accessible.
- Common Misconceptions: Addressing misconceptions clarifies misunderstandings and provides accurate knowledge about fractions and square roots.
In conclusion, the study of 1/4 and its square root offers valuable insights into mathematical concepts and their real-world applications. By understanding these basics, one can better appreciate the beauty and utility of mathematics in various disciplines.
We hope this guide has provided a thorough understanding of 1/4 and its square root, equipping you with the knowledge to apply these concepts effectively in both academic and practical contexts.
References and Further Reading
-
- Detailed steps and explanations for calculating the square root of 1/4.
-
- A breakdown of the mathematical process to determine the square root of 1/4.
-
- An introduction to the concepts of squares and square roots with easy-to-understand examples.
-
- Educational videos and exercises on approximating square roots and understanding their properties.
-
- An online tool to calculate the square roots of any number, including fractions like 1/4.

Căn bậc hai của 1/4 | Căn(1/4)
READ MORE:
Tìm Căn Bậc Hai: -√(1/4)