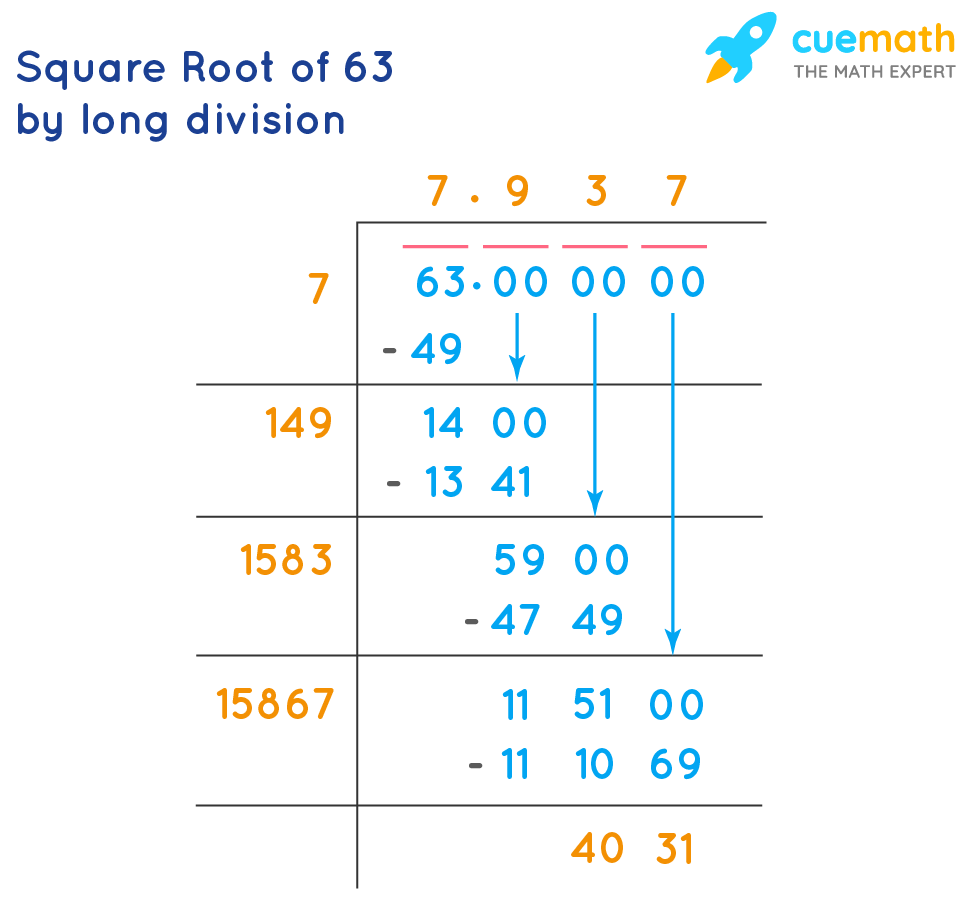
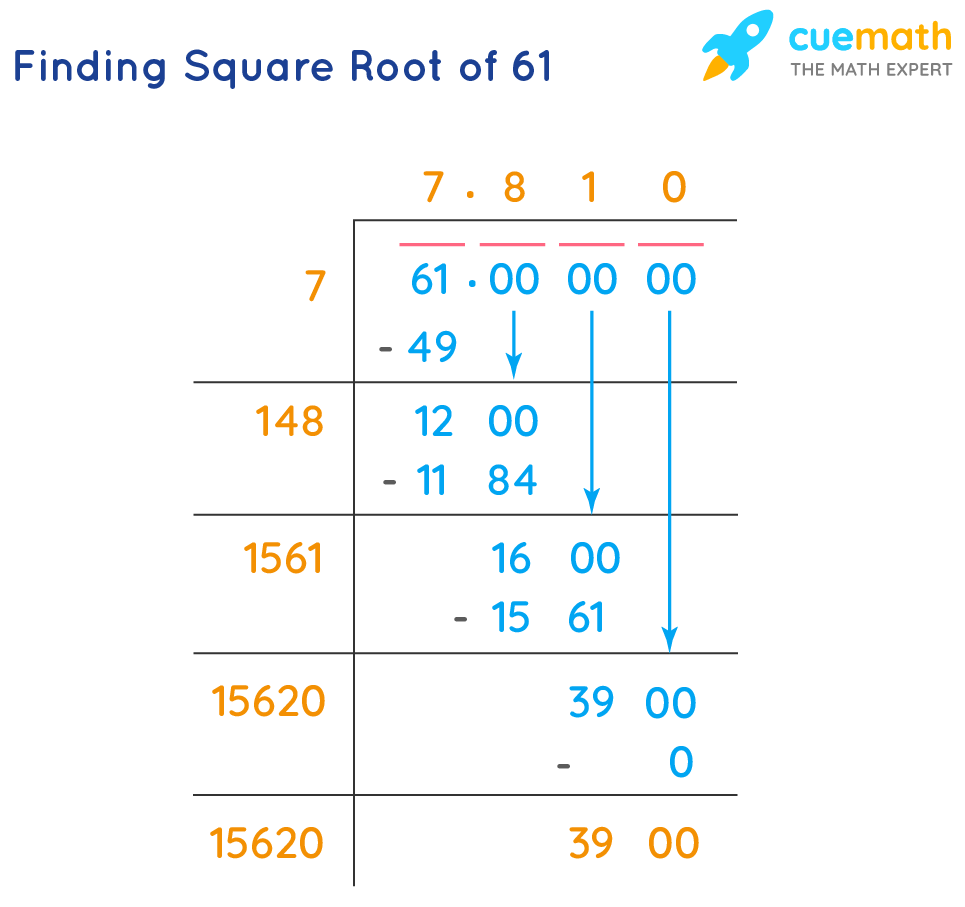
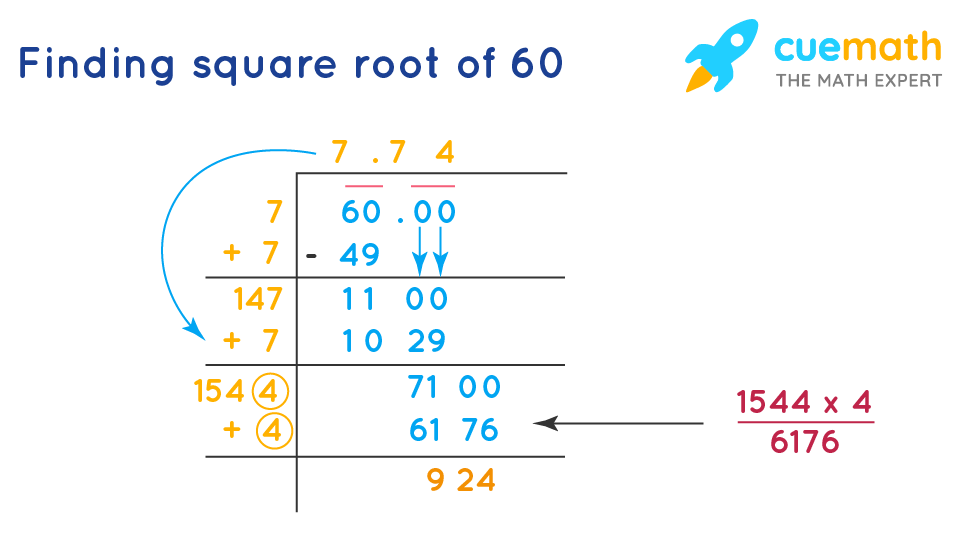
Topic what is the square of 65: Understanding what the square of 65 is can be essential for various mathematical and real-world applications. In this article, we'll explore the calculation, significance, and uses of squaring numbers, with a focus on 65. Join us to uncover why knowing the square of 65 is both interesting and valuable.
Table of Content
- Square Calculation
- Introduction to Squaring Numbers
- Definition of Squaring
- Mathematical Notation
- Basic Calculation of Square of 65
- Step-by-Step Guide to Calculating 65 Squared
- Understanding the Multiplication Process
- Real-World Applications of Squaring Numbers
- Importance of Squaring in Mathematics
- Using a Calculator to Find the Square of 65
- Common Mistakes When Squaring Numbers
- Alternative Methods for Squaring Numbers
- Historical Context of Squaring in Mathematics
- Advanced Concepts Related to Squaring Numbers
- Conclusion on the Square of 65
- YOUTUBE: Video này giới thiệu về cách tính căn bậc hai của 65. Video hướng dẫn chi tiết và dễ hiểu cho người xem.
Square Calculation
The square of a number is the result of multiplying that number by itself. In mathematical notation, the square of a number \( n \) is denoted as \( n^2 \).
Example: Calculating the Square of 65
To find the square of 65, we multiply 65 by itself:
\[
65^2 = 65 \times 65
\]
Performing the multiplication:
\[
65 \times 65 = 4225
\]
Therefore, the square of 65 is 4225.
Step-by-Step Calculation
- Write down the number: 65
- Multiply the number by itself: \( 65 \times 65 \)
- Calculate the result: \( 4225 \)
So, the square of 65 is:
\[
65^2 = 4225
\]

READ MORE:
Introduction to Squaring Numbers
Squaring a number is a fundamental mathematical operation where a number is multiplied by itself. This operation is denoted by raising the number to the power of two. The result of squaring a number is called a "square" and is useful in various mathematical contexts and real-world applications.
Let's break down the concept and steps involved in squaring a number:
- Identify the number you want to square. In our case, it is 65.
- Multiply the number by itself. This can be expressed as:
\[
65^2 = 65 \times 65
\] - Calculate the result of the multiplication:
\[
65 \times 65 = 4225
\]
Thus, the square of 65 is 4225.
Squaring numbers is not only a basic arithmetic skill but also has practical uses in geometry, physics, engineering, and computer science. For instance, calculating the area of a square when the length of one side is known, or determining the variance in statistical data involves squaring numbers.
Understanding and mastering the process of squaring numbers can enhance your problem-solving abilities and provide a strong foundation for more advanced mathematical concepts.
Definition of Squaring
Squaring a number is a mathematical operation that involves multiplying the number by itself. This is an essential concept in mathematics, often used to describe the area of squares, among other applications.
In mathematical terms, the square of a number \( n \) is written as \( n^2 \). The notation \( n^2 \) means \( n \) multiplied by \( n \). For example, the square of 65 is calculated as follows:
- Start with the number: 65.
- Multiply the number by itself:
\[
65^2 = 65 \times 65
\] - Perform the multiplication:
\[
65 \times 65 = 4225
\]
Therefore, the square of 65 is 4225.
Here are some key points to remember about squaring numbers:
- Squaring a positive number results in a positive number.
- Squaring a negative number also results in a positive number, as the product of two negative numbers is positive.
- The square of zero is zero.
Squaring is used in various fields such as physics, engineering, and computer science. For instance, it is crucial for calculating areas, determining quadratic equations, and analyzing statistical data.
Mathematical Notation
In mathematics, notation is used to concisely express operations and relationships. Squaring a number is a common operation, and the notation for this is straightforward and universally understood.
To denote the square of a number \( n \), we write \( n^2 \). This exponentiation indicates that \( n \) is to be multiplied by itself. For example, to represent the square of 65, we write:
\[
65^2
\]
Here's a step-by-step breakdown of the notation and calculation:
- Identify the number to be squared, in this case, 65.
- Use the exponentiation notation, which in this case is:
\[
65^2
\] - Understand that \( 65^2 \) means \( 65 \times 65 \).
- Perform the multiplication:
\[
65 \times 65 = 4225
\]
Therefore, using mathematical notation, we have shown that the square of 65 is 4225.
This notation is used because it is efficient and reduces the complexity of writing out repeated multiplication, especially for larger numbers or more complex expressions. Here are some additional points to note about mathematical notation for squaring:
- Exponents are typically written as superscripts, such as \( n^2 \).
- This notation can be extended to higher powers, such as \( n^3 \) for cubing, \( n^4 \) for the fourth power, and so on.
- The notation is consistent and universally understood in the mathematical community, making it easier to communicate complex ideas succinctly.
Understanding and using mathematical notation correctly is crucial for solving problems efficiently and accurately in mathematics and related fields.
Basic Calculation of Square of 65
The process of squaring a number involves multiplying the number by itself. In this section, we will perform the basic calculation to find the square of 65, step by step.
- Start with the number 65.
- Multiply 65 by itself:
\[
65 \times 65
\] - Break down the multiplication for clarity:
First, multiply the tens place: \( 60 \times 60 = 3600 \)
Next, multiply the units place: \( 5 \times 5 = 25 \)
Now, use the distributive property to combine:
- \( 60 \times 5 = 300 \)
- \( 5 \times 60 = 300 \)
Sum these partial products:
- \( 3600 \) (from \( 60 \times 60 \))
- \( 300 \) (from \( 60 \times 5 \))
- \( 300 \) (from \( 5 \times 60 \))
- \( 25 \) (from \( 5 \times 5 \))
- Add the results together:
\[
3600 + 300 + 300 + 25 = 4225
\]
Therefore, the square of 65 is:
\[
65^2 = 4225
\]
Through this calculation, we have confirmed that the square of 65 is 4225. This method can be applied to square any number, providing a clear and systematic approach to multiplication.

Step-by-Step Guide to Calculating 65 Squared
Calculating the square of a number involves multiplying the number by itself. Here, we will walk through the steps to find the square of 65 in a detailed manner.
- Identify the number to be squared: 65.
- Write the multiplication expression:
\[
65 \times 65
\] - Break down the number into parts to simplify the calculation:
- 65 can be broken down into \( 60 + 5 \).
- Use the distributive property to expand the expression:
\[
(60 + 5) \times (60 + 5)
\]- First, multiply the tens place: \( 60 \times 60 = 3600 \).
- Then, multiply the tens place by the units place twice:
\[
60 \times 5 = 300
\]
\[
5 \times 60 = 300
\] - Finally, multiply the units place: \( 5 \times 5 = 25 \).
- Add the partial products together:
- Sum of squares of tens: \( 3600 \)
- Sum of cross-products: \( 300 + 300 = 600 \)
- Sum of square of units: \( 25 \)
\[
3600 + 600 + 25 = 4225
\]
Therefore, the square of 65 is:
\[
65^2 = 4225
\]
This method of breaking down the number and using the distributive property helps to simplify the calculation and ensures accuracy.
Understanding the Multiplication Process
To fully grasp the concept of squaring a number, it is essential to understand the underlying multiplication process. In this section, we will break down the multiplication process used to calculate the square of 65 in a detailed manner.
- Identify the number to be squared: 65.
- Write the multiplication expression:
\[
65 \times 65
\] - Break down the number into manageable parts to simplify the multiplication:
- Express 65 as the sum of its place values: \( 60 + 5 \).
- Apply the distributive property (FOIL method) to expand the expression:
\[
(60 + 5) \times (60 + 5) = 60 \times 60 + 60 \times 5 + 5 \times 60 + 5 \times 5
\]- First, multiply the tens place by itself:
\[
60 \times 60 = 3600
\] - Next, multiply the tens place by the units place:
\[
60 \times 5 = 300
\] - Then, multiply the units place by the tens place (same as above, but from the other factor):
\[
5 \times 60 = 300
\] - Finally, multiply the units place by itself:
\[
5 \times 5 = 25
\]
- First, multiply the tens place by itself:
- Add all the partial products together to get the final result:
- Sum of the squares of the tens: \( 3600 \)
- Sum of the cross-products: \( 300 + 300 = 600 \)
- Sum of the squares of the units: \( 25 \)
\[
3600 + 600 + 25 = 4225
\]
Therefore, the square of 65 is:
\[
65^2 = 4225
\]
Understanding this multiplication process is crucial as it lays the foundation for more complex arithmetic and algebraic operations. By breaking down the calculation into smaller, more manageable steps, we can ensure accuracy and develop a deeper comprehension of the principles involved.
Real-World Applications of Squaring Numbers
Squaring numbers is a fundamental mathematical operation with numerous real-world applications across various fields. Understanding these applications can highlight the importance and utility of this concept.
- Geometry and Area Calculations:
In geometry, squaring is used to calculate the area of squares. If each side of a square is of length \( s \), the area \( A \) is given by:
\[
A = s^2
\]
For example, if the side length is 65 units, the area is:
\[
65^2 = 4225 \text{ square units}
\] - Physics and Engineering:
In physics, squaring is used in formulas involving energy, force, and other physical quantities. For instance, the kinetic energy (\( KE \)) of an object is given by:
\[
KE = \frac{1}{2} mv^2
\]
where \( m \) is the mass and \( v \) is the velocity. Here, the velocity is squared to calculate the kinetic energy. - Statistics and Data Analysis:
In statistics, squaring is crucial for calculating the variance and standard deviation of a data set, which measure data dispersion. The variance (\( \sigma^2 \)) is calculated as:
\[
\sigma^2 = \frac{1}{N} \sum_{i=1}^{N} (x_i - \mu)^2
\]
where \( x_i \) are data points, \( \mu \) is the mean, and \( N \) is the number of data points. - Financial Calculations:
In finance, squaring is used in various models, such as the calculation of compound interest and risk assessment. For example, the volatility of an asset, which is essential for risk management, often involves squared terms.
- Astronomy:
Astronomers use squaring when calculating gravitational forces and distances. Newton's law of universal gravitation states:
\[
F = G \frac{m_1 m_2}{r^2}
\]
where \( F \) is the force between two masses \( m_1 \) and \( m_2 \), \( r \) is the distance between the centers of the two masses, and \( G \) is the gravitational constant. Here, the distance \( r \) is squared. - Computer Science:
In computer science, algorithms often use squaring for tasks such as cryptography and error detection/correction. Squaring helps in generating keys for encryption algorithms, ensuring secure data transmission.
These examples demonstrate that squaring numbers is not just a basic arithmetic operation, but a critical tool in various scientific, engineering, financial, and technological applications. Mastery of this concept allows for better understanding and problem-solving in these fields.
Importance of Squaring in Mathematics
Squaring numbers is a fundamental operation in mathematics with broad applications across various fields. Here are some key reasons why squaring is important:
- Area Calculations: Squaring is essential in geometry, especially for calculating the area of squares. For instance, a square with a side length of 65 units has an area of \(65^2 = 4,225\) square units.
- Quadratic Equations: Squaring plays a crucial role in solving quadratic equations, which appear frequently in algebra and calculus.
- Exponential Growth: Squaring functions are used to model exponential growth in fields like finance, biology, and physics. Understanding squaring helps in predicting growth patterns and rates.
- Pythagorean Theorem: In trigonometry, squaring is integral to the Pythagorean theorem, which relates the squares of the sides of a right triangle: \(a^2 + b^2 = c^2\).
- Statistics: Squaring is used in statistics to calculate variance and standard deviation, which measure the spread of data points in a dataset.
Mathematically, squaring a number n is represented as \(n^2\) or \(n \times n\). It is a building block for higher mathematical concepts and is utilized in various methods to simplify complex calculations.
Here is a table demonstrating the squares of numbers close to 65:
| Number | Square |
| 60 | 3,600 |
| 61 | 3,721 |
| 62 | 3,844 |
| 63 | 3,969 |
| 64 | 4,096 |
| 65 | 4,225 |
| 66 | 4,356 |
| 67 | 4,489 |
Understanding the importance of squaring enhances mathematical literacy and problem-solving abilities, making it a vital concept in education and practical applications.

Using a Calculator to Find the Square of 65
Calculating the square of 65 using a calculator is a straightforward process. Here are the detailed steps to follow:
-
Turn on the Calculator: Ensure your calculator is powered on and functioning correctly.
-
Enter the Number: Press the key for the number
65. -
Square the Number: Press the square function key, usually denoted as
x²or^2. The calculator will display the result of \(65^2\). -
Read the Result: The calculator should show the result as
4225. Thus, \(65^2 = 4225\).
To summarize, using a calculator to find the square of 65 involves entering the number, using the square function, and reading the result. Here is an example of how it might look on a calculator:
65x²= 4225
Using a calculator ensures accuracy and saves time, especially for larger numbers. This method is not only efficient but also helps in verifying manual calculations. If your calculator supports it, you can also use more advanced functions to explore related mathematical concepts.
For additional methods and more detailed calculations, you can refer to online resources or specialized calculators that provide step-by-step solutions and explanations.
Common Mistakes When Squaring Numbers
Squaring numbers is a fundamental mathematical operation, but it's easy to make mistakes. Here are some common errors and how to avoid them:
-
Incorrect Multiplication:
When squaring a number, you multiply the number by itself. A common mistake is to multiply it by 2 instead. For example, the square of 65 is \(65 \times 65\), not \(65 \times 2\).
-
Misplacing Digits:
During multiplication, especially with larger numbers, it's easy to misplace digits. Carefully align the numbers to avoid errors. Using grid or column methods can help.
-
Forgetting Intermediate Steps:
In manual calculations, skipping steps can lead to mistakes. Write out each step clearly:
- Multiply the units place: \(5 \times 5 = 25\) (write down 5, carry over 2)
- Multiply crosswise and add carry over: \(6 \times 5 + 5 \times 6 = 30 + 30 = 60\), add 2 (carry over) to get 62 (write down 2, carry over 6)
- Multiply the tens place and add carry over: \(6 \times 6 = 36\), add 6 (carry over) to get 42
Combine these results to get \(65^2 = 4225\).
-
Using Incorrect Formula:
Remember that the formula for squaring a sum is \((a + b)^2 = a^2 + 2ab + b^2\). Misapplying algebraic identities can result in incorrect answers.
-
Neglecting Negative Signs:
When squaring negative numbers, the result is always positive. For example, \((-65)^2 = 65^2 = 4225\). Forgetting this can lead to errors.
-
Calculator Input Errors:
When using a calculator, double-check your input. Ensure you are squaring the number, not multiplying by 2. Some calculators have a specific square function to avoid this error.
-
Misunderstanding Perfect Squares:
Recognize perfect squares and their properties to avoid mistakes. For instance, knowing that \(64\) is \(8^2\) and \(81\) is \(9^2\) helps in quickly verifying calculations.
By being mindful of these common mistakes, you can improve accuracy when squaring numbers.
Alternative Methods for Squaring Numbers
While the traditional method of squaring a number involves simple multiplication, there are several alternative methods that can simplify the process, especially for specific types of numbers. Here are some alternative methods for squaring numbers:
-
Using the Algebraic Identity Method:
The algebraic identity for squaring a number is:
\[
(a + b)^2 = a^2 + 2ab + b^2
\]For example, to find the square of 65:
Let \( a = 60 \) and \( b = 5 \)
\[
65^2 = (60 + 5)^2 = 60^2 + 2 \cdot 60 \cdot 5 + 5^2
\]
\[
60^2 = 3600, \quad 2 \cdot 60 \cdot 5 = 600, \quad 5^2 = 25
\]
\[
65^2 = 3600 + 600 + 25 = 4225 -
Using the Difference of Squares Method:
This method involves expressing the number as the difference of two squares. The identity used is:
\[
a^2 - b^2 = (a - b)(a + b)
\]For instance, to find the square of 65, you can rewrite it as:
\[
65^2 = (65)^2 = (64 + 1)^2
\]
\[
= 64^2 + 2 \cdot 64 \cdot 1 + 1^2
\]
\[
64^2 = 4096, \quad 2 \cdot 64 \cdot 1 = 128, \quad 1^2 = 1
\]
\[
65^2 = 4096 + 128 + 1 = 4225 -
Using the Special Case Method for Numbers Ending in 5:
This method leverages a shortcut specifically for numbers ending in 5. The rule is:
\[
(10a + 5)^2 = 100a(a + 1) + 25For example, to find the square of 65:
\[
a = 6
\[
65^2 = 100 \cdot 6 \cdot (6 + 1) + 25 = 100 \cdot 6 \cdot 7 + 25
\]
\[
= 100 \cdot 42 + 25 = 4200 + 25 = 4225 -
Using Vedic Mathematics Techniques:
Vedic mathematics offers various sutras (formulas) for rapid calculations. One of them is the "By one more than the one before" method:
For squaring numbers ending in 5, such as 65:
Identify the tens digit (6) and multiply it by the next higher number (7):
\[
6 \cdot 7 = 42Then append 25 to the result:
\[
65^2 = 4225
These alternative methods can make squaring numbers quicker and easier, especially when dealing with specific types of numbers or when performing mental calculations.
Historical Context of Squaring in Mathematics
Squaring numbers, particularly the problem of squaring geometric shapes, has a rich history in mathematics. This concept has intrigued mathematicians across different civilizations and eras, leading to significant developments in mathematical theory and practice.
Ancient Civilizations
In ancient Babylon, mathematicians developed sophisticated techniques for solving quadratic equations, which included finding squares and square roots using pre-calculated tables. They could efficiently compute these values, showcasing their advanced understanding of algebra and geometry.
The Greeks contributed immensely to the concept of squaring through geometric methods. They used squaring to find areas of various shapes, including the quadrature of the circle, which involved constructing a square with the same area as a given circle using only a compass and straightedge. Although ultimately proven impossible for a perfect circle, this problem spurred much mathematical innovation.
Medieval and Renaissance Developments
During the medieval period, European mathematicians continued to explore squaring techniques, often using methods that were less rigorous but more powerful than those of the Greeks. The method of indivisibles, a precursor to integral calculus, was one such technique that allowed for the calculation of areas under curves and contributed to the understanding of squaring complex figures.
In the Renaissance, mathematicians like Regiomontanus and Leonardo da Vinci further investigated squaring the circle and other geometric problems. These efforts, although not always successful, laid the groundwork for the development of calculus.
The Era of Calculus
With the advent of integral calculus, mathematicians like John Wallis, Isaac Barrow, and James Gregory made significant strides in understanding and calculating areas. The techniques developed during this period provided a universal method for finding areas, effectively solving many problems related to squaring shapes.
Modern Implications
Today, the concept of squaring extends beyond simple geometric shapes to complex applications in various fields such as physics, engineering, and computer science. The historical pursuit of squaring shapes has not only enriched mathematical theory but also provided tools and methods that are fundamental to modern scientific and technological advancements.

Advanced Concepts Related to Squaring Numbers
Squaring numbers extends beyond basic arithmetic into several advanced mathematical concepts. Here, we explore some of these concepts in detail:
- Exponentiation: Squaring a number is a specific case of exponentiation where the exponent is 2. This is written as \( a^2 \), which means \( a \times a \). Understanding this principle is foundational to more complex exponentiation, such as cubic numbers (\( a^3 \)) and higher powers.
- Complex Numbers: In the realm of complex numbers, squaring becomes more intriguing. A complex number \( z \) can be written as \( z = a + bi \), where \( i \) is the imaginary unit. The square of \( z \) is calculated as: \[ z^2 = (a + bi)^2 = a^2 + 2abi + (bi)^2 = a^2 - b^2 + 2abi \] This introduces concepts like the imaginary unit and its properties.
- Matrices: In linear algebra, squaring matrices involves matrix multiplication. For a square matrix \( A \): \[ A^2 = A \times A \] Matrix squaring is used in solving systems of linear equations, transformation geometry, and more.
- Calculus and Differential Equations: Squaring functions is common in calculus. For example, when dealing with parabolic functions, \( f(x) = x^2 \), the square function's derivatives and integrals are foundational concepts. In differential equations, squaring terms appear frequently in nonlinear equations.
- Number Theory: Squares of integers, known as perfect squares, play a significant role in number theory. Concepts like Pythagorean triples and the Fermat's Last Theorem involve squares. For example, Pythagorean triples are sets of three integers \( a \), \( b \), and \( c \) that satisfy \( a^2 + b^2 = c^2 \).
- Optimization: In optimization problems, particularly those involving quadratic forms, squaring functions are used to describe the cost, error, or energy in physical systems. This leads to quadratic programming and least squares methods in data fitting.
Advanced understanding of squaring numbers is crucial in these and many other areas, illustrating its profound impact on various mathematical fields and applications.
Conclusion on the Square of 65
In conclusion, the square of 65, which is 4225, demonstrates the fascinating properties and applications of squaring numbers in mathematics. Squaring is a fundamental arithmetic operation that is used extensively in various fields such as algebra, geometry, and even in everyday problem-solving scenarios.
Understanding how to square a number like 65 provides a foundation for more advanced mathematical concepts. It showcases the importance of mastering basic mathematical operations as they form the building blocks for more complex calculations and theories.
Additionally, squaring numbers has practical applications in real life, from calculating areas in geometry to analyzing quadratic equations in algebra. This operation also appears in many real-world contexts, such as physics, engineering, and computer science, where it helps in modeling and solving problems.
By exploring the square of 65, we appreciate the elegance and utility of mathematics in both theoretical and practical domains. The ability to square numbers accurately and efficiently is a skill that enhances our overall mathematical proficiency and problem-solving capabilities.
Thus, whether you are a student learning the basics or a professional applying advanced concepts, the understanding and application of squaring numbers remain an essential aspect of mathematical literacy.
Video này giới thiệu về cách tính căn bậc hai của 65. Video hướng dẫn chi tiết và dễ hiểu cho người xem.
CĂN BẬC HAI CỦA 65
READ MORE:
Video ngắn này trình bày các số bình phương từ 1 đến 100 một cách trực quan và thú vị.
Bình Phương Từ 1 Đến 100 ll #shorts