Topic is square root of 6 a rational number: Many wonder if the square root of 6 is a rational number. In this article, we delve into the nature of √6, explore its properties, and provide a clear answer to this mathematical question. Join us as we uncover the truth about the square root of 6 and its classification.
Table of Content
- Is the Square Root of 6 a Rational Number?
- Introduction to Rational and Irrational Numbers
- Understanding Rational Numbers
- Understanding Irrational Numbers
- Properties of the Square Root of 6
- Mathematical Proof of Irrationality
- Contradiction Method
- Numerical Approximation of Square Root of 6
- Decimal Expansion of Square Root of 6
- Historical Context and Discovery
- Importance in Mathematics
- Applications of Irrational Numbers
- Common Misconceptions
- YOUTUBE: Video giải thích khái niệm căn bậc hai bằng tiếng Việt. Tìm hiểu cách tính căn bậc hai và ứng dụng của nó trong toán học với Mr. J.
Is the Square Root of 6 a Rational Number?
The question of whether the square root of 6 is a rational number can be addressed through a detailed exploration of rational and irrational numbers.
Definition of Rational and Irrational Numbers
A rational number is any number that can be expressed as the quotient or fraction of two integers, where the numerator is an integer and the denominator is a non-zero integer. In mathematical terms, a rational number can be written as p/q, where p and q are integers, and q ≠ 0.
An irrational number, on the other hand, cannot be expressed as a simple fraction. Irrational numbers have non-repeating, non-terminating decimal expansions.
Square Root of 6
To determine if the square root of 6 is a rational number, we need to analyze its properties:
- The square root of 6 is denoted as √6.
- √6 is approximately equal to 2.449489742783178...
- This decimal representation does not terminate or repeat.
Proof by Contradiction
One common method to determine if √6 is rational or irrational is to use a proof by contradiction:
- Assume √6 is rational. Then it can be written as a/b where a and b are coprime integers (having no common factors other than 1), and b ≠ 0.
- Then, (√6)2 = (a/b)2, which implies 6 = a2/b2.
- By rearranging, we get a2 = 6b2.
- This equation implies that a2 is divisible by 6. Therefore, a must also be divisible by 6 (since 6 is not a perfect square).
- Let a = 6k for some integer k. Then, substituting back, we get (6k)2 = 6b2, which simplifies to 36k2 = 6b2, and further to 6k2 = b2.
- This implies that b2 is divisible by 6, and hence b must also be divisible by 6.
- This contradicts our original assumption that a and b are coprime.
Since assuming that √6 is rational leads to a contradiction, we conclude that √6 is irrational.
Conclusion
Based on the analysis and the proof by contradiction, it is clear that the square root of 6 is not a rational number. It is an irrational number.

READ MORE:
Introduction to Rational and Irrational Numbers
In mathematics, numbers are categorized into various types, among which rational and irrational numbers are fundamental. Understanding these categories helps in comprehending the nature of numbers and their properties.
Rational Numbers are defined as numbers that can be expressed as the quotient of two integers, where the numerator is an integer and the denominator is a non-zero integer. In mathematical notation, a rational number can be written as:
\[ \frac{a}{b} \]
where \( a \) and \( b \) are integers and \( b \neq 0 \).
Examples of rational numbers include:
- \(\frac{1}{2}\)
- \(-\frac{3}{4}\)
- \(5\) (which can be written as \(\frac{5}{1}\))
- \(0\) (which can be written as \(\frac{0}{1}\))
Rational numbers can have finite or repeating decimal expansions. For example, \(\frac{1}{2} = 0.5\) and \(\frac{1}{3} = 0.333...\).
Irrational Numbers, in contrast, are numbers that cannot be expressed as a simple fraction of two integers. Their decimal expansions are non-terminating and non-repeating. This means they continue infinitely without repeating a pattern.
Examples of irrational numbers include:
- \(\pi\) (Pi), approximately 3.14159...
- \(e\) (Euler's number), approximately 2.71828...
- \(\sqrt{2}\), approximately 1.41421...
- \(\sqrt{6}\), approximately 2.44949...
The distinction between rational and irrational numbers is crucial for understanding number theory and various mathematical concepts. Recognizing whether a number is rational or irrational can help in solving equations, understanding functions, and more.
Understanding Rational Numbers
Rational numbers are a fundamental concept in mathematics, defined as numbers that can be expressed as the quotient of two integers. This means any number that can be written in the form:
\[ \frac{a}{b} \]
where \( a \) and \( b \) are integers and \( b \neq 0 \), is considered a rational number. These numbers include both positive and negative fractions, as well as whole numbers and zero.
Some key characteristics of rational numbers include:
- They can be written as fractions: For example, \( \frac{1}{2} \), \( \frac{-3}{4} \), and \( \frac{7}{1} \) are all rational numbers.
- They have either terminating or repeating decimal expansions: For example, \( \frac{1}{4} = 0.25 \) (terminating) and \( \frac{1}{3} = 0.333\ldots \) (repeating).
To further understand rational numbers, consider the following properties:
- Closure: The sum, difference, product, and quotient (except division by zero) of two rational numbers is always a rational number. For instance, \( \frac{1}{2} + \frac{1}{3} = \frac{3}{6} + \frac{2}{6} = \frac{5}{6} \).
- Density: Between any two rational numbers, there exists another rational number. For example, between \( \frac{1}{2} \) and \( \frac{2}{3} \), there is \( \frac{5}{9} \).
- Equivalence: Different fractions can represent the same rational number if they are equivalent. For instance, \( \frac{2}{4} = \frac{1}{2} \).
Rational numbers form a dense subset of the real numbers, meaning there are infinitely many rational numbers between any two real numbers. This property makes them incredibly useful in various mathematical and practical applications.
Understanding rational numbers lays the foundation for more advanced mathematical concepts and helps in solving real-world problems that involve fractional quantities and ratios.
Understanding Irrational Numbers
Irrational numbers are real numbers that cannot be expressed as the quotient of two integers. Unlike rational numbers, which have either terminating or repeating decimal expansions, the decimal expansions of irrational numbers are non-terminating and non-repeating. This unique characteristic sets irrational numbers apart and makes them an intriguing subject of study in mathematics.
Some well-known examples of irrational numbers include:
- \(\pi\) (Pi), approximately 3.14159...
- \(e\) (Euler's number), approximately 2.71828...
- \(\sqrt{2}\), approximately 1.41421...
- \(\sqrt{6}\), approximately 2.44949...
The discovery of irrational numbers dates back to ancient Greece, where mathematicians like Pythagoras initially believed that all numbers were rational. The realization that \(\sqrt{2}\) could not be expressed as a fraction marked the beginning of the study of irrational numbers.
To understand why numbers like \(\sqrt{6}\) are irrational, consider the following properties:
- Non-Terminating, Non-Repeating Decimal Expansion: Irrational numbers cannot be precisely represented as fractions. Their decimal expansions go on infinitely without repeating any pattern. For instance, \(\sqrt{6} \approx 2.44949...\).
- Proof by Contradiction: One common method to prove a number is irrational is by contradiction. For example, assuming \(\sqrt{6}\) is rational leads to a contradiction, as shown in mathematical proofs involving coprime integers and the properties of even and odd numbers.
- Incommensurability: Irrational numbers cannot be measured by the same unit as rational numbers. This means that there is no common measure that can exactly divide both an irrational and a rational length.
Irrational numbers play a crucial role in various branches of mathematics, including algebra, calculus, and number theory. They are essential in understanding the completeness of the real number line, as they fill in the gaps between rational numbers.
Despite their complexity, irrational numbers are fundamental to many real-world applications. For example, \(\pi\) is used in calculating the circumference and area of circles, while \(e\) appears in natural logarithms and compound interest calculations.
In summary, irrational numbers are a fascinating and essential part of mathematics. Their unique properties and applications highlight the richness and depth of the mathematical landscape.
Properties of the Square Root of 6
The square root of 6, denoted as \( \sqrt{6} \), has several important mathematical properties that highlight its significance as an irrational number:
- Non-terminating and Non-repeating Decimal: The decimal expansion of \( \sqrt{6} \) is non-terminating and non-repeating, indicating that it cannot be expressed as a fraction of two integers.
- Approximate Value: The approximate value of \( \sqrt{6} \) is 2.44949. This is useful for practical calculations, though it is not exact.
- Prime Factorization: The number 6 can be factored into prime numbers as \( 2 \times 3 \). Since neither of these primes is a perfect square, their product's square root is irrational.
- Algebraic Property: The square root of 6 satisfies the equation \( x^2 = 6 \). This equation has no rational solutions, further confirming the irrationality of \( \sqrt{6} \).
Visual Representation
A visual representation can help in understanding the properties of \( \sqrt{6} \). Below is a table showing some key approximations:
| Decimal Place | Approximate Value |
|---|---|
| 1st | 2.4 |
| 2nd | 2.45 |
| 3rd | 2.449 |
| 4th | 2.4495 |
| 5th | 2.44949 |
Mathematical Expression
Using the prime factorization, the property of irrationality can be shown as follows:
\[
\sqrt{6} = \sqrt{2 \times 3} = \sqrt{2} \times \sqrt{3}
\]
Since both \( \sqrt{2} \) and \( \sqrt{3} \) are known to be irrational, their product, \( \sqrt{6} \), is also irrational.
Mathematical Proof of Irrationality
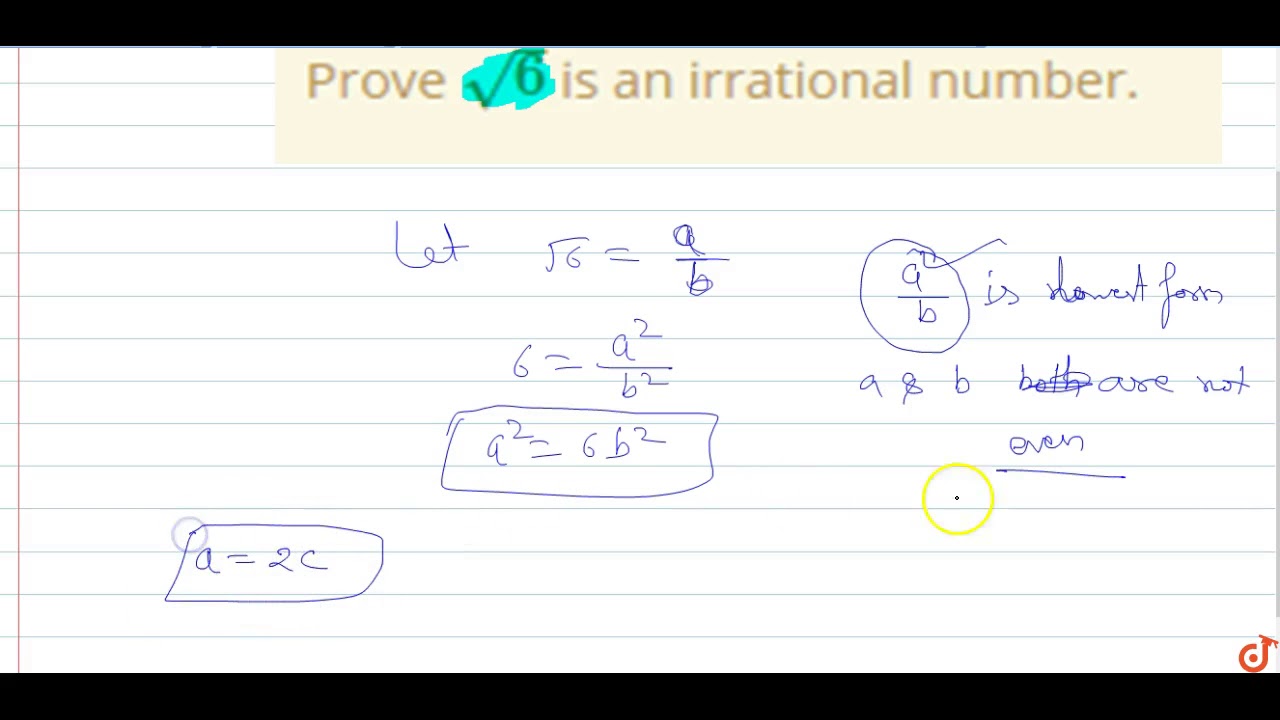
To prove that the square root of 6 is irrational, we will use a proof by contradiction. This method involves assuming the opposite of what we want to prove and then showing that this assumption leads to a contradiction.
- Assume that √6 is a rational number. This means it can be expressed as a fraction of two integers, say \( \frac{a}{b} \), where \(a\) and \(b\) are coprime integers (they have no common factors other than 1), and \(b \neq 0\).
- If \( \sqrt{6} = \frac{a}{b} \), then squaring both sides gives \(6 = \frac{a^2}{b^2}\).
- Rearranging this equation, we get \(6b^2 = a^2\).
- This implies that \(a^2\) is divisible by 6, and hence \(a\) must also be divisible by 6 (since 6 is a product of primes and squares of primes are only divisible by those primes).
- Let \(a = 6k\) for some integer \(k\). Substituting \(a = 6k\) into the equation \(6b^2 = a^2\), we get \(6b^2 = (6k)^2\), which simplifies to \(6b^2 = 36k^2\) or \(b^2 = 6k^2\).
- This implies that \(b^2\) is divisible by 6, and hence \(b\) must also be divisible by 6.
- Therefore, both \(a\) and \(b\) are divisible by 6, which contradicts our initial assumption that \(a\) and \(b\) are coprime.
Since our initial assumption leads to a contradiction, it must be false. Therefore, the square root of 6 cannot be expressed as a fraction of two integers, meaning that it is an irrational number.
Another way to demonstrate the irrationality of √6 is by examining its decimal expansion. The value of √6 is approximately 2.44948974278... and this decimal representation does not terminate or repeat, which is a defining characteristic of irrational numbers.
In summary, both the proof by contradiction and the non-repeating, non-terminating decimal expansion confirm that the square root of 6 is an irrational number.
Contradiction Method
The contradiction method is a powerful mathematical proof technique used to demonstrate the irrationality of certain numbers, such as the square root of 6. To prove that the square root of 6 is irrational using contradiction, we assume the opposite of what we want to prove and then show that this assumption leads to a logical inconsistency.
Assume that is a rational number. This means it can be expressed as a fraction where p and q are integers with no common factors (i.e., the fraction is in its simplest form), and q is not zero.
According to our assumption:
Squaring both sides gives:
Rearranging this equation, we get:
This implies that squared is a multiple of 6, and hence, itself must be a multiple of 6. Let for some integer k.
Substituting into the equation , we get:
Which simplifies to:
And further simplifies to:
This implies that squared is also a multiple of 6, and hence, itself must be a multiple of 6.
Thus, both and are multiples of 6, which contradicts our initial assumption that is in its simplest form.
Since our initial assumption leads to a contradiction, we conclude that cannot be expressed as a fraction of two integers. Therefore, the square root of 6 is irrational.
Numerical Approximation of Square Root of 6
The square root of 6, denoted as \( \sqrt{6} \), is an irrational number. This means it cannot be expressed as a simple fraction and its decimal representation is non-terminating and non-repeating. The approximate value of \( \sqrt{6} \) is 2.44948974278. Here are a few methods to approximate this value:
Estimation Method
This method involves making an initial guess and refining it through successive iterations:
- Start with an initial guess. Since 6 is between 4 (22) and 9 (32), the square root of 6 is between 2 and 3.
- Take a number between 2 and 3, say 2.5, and square it: \( 2.5 \times 2.5 = 6.25 \).
- Since 6.25 is higher than 6, try a smaller number, like 2.4, and square it: \( 2.4 \times 2.4 = 5.76 \).
- Continue adjusting your guess until you get close to the actual value. A more refined guess can be 2.45, and square it: \( 2.45 \times 2.45 = 6.0025 \).
- Further refine to get more accurate results.
Long Division Method
The long division method is a systematic way to find the square root of a number to several decimal places:
- Make a pair of digits from the number starting from the decimal point.
- Find a number such that when multiplied by itself, the product is less than or equal to 6. We start with 2 because \( 2 \times 2 = 4 \).
- Subtract 4 from 6 to get 2, then bring down a pair of zeros to make it 200.
- Double the current guess (2), giving us 4. Find a number X such that \( 4X \times X \) is less than 200. The closest number is 4. The new number is 2.4.
- Continue the process to find more decimal places. The next number is 44, and the process continues until the desired precision is reached.
Using a Calculator
Modern calculators use efficient algorithms to quickly find the square roots. Simply inputting 6 and pressing the square root button will yield an approximation of \( 2.44948974278 \).
Decimal Expansion
The decimal expansion of \( \sqrt{6} \) is non-terminating and non-repeating. Its value to several decimal places is:
\[ \sqrt{6} \approx 2.44948974278 \]
This non-repeating, non-terminating decimal confirms the irrationality of \( \sqrt{6} \), as it cannot be expressed as a fraction of two integers.
Decimal Expansion of Square Root of 6
The decimal expansion of the square root of 6, denoted as \( \sqrt{6} \), is a non-terminating and non-repeating decimal, which classifies it as an irrational number. The process of finding this decimal expansion involves several mathematical techniques such as the long division method, which provides an iterative way to approximate the square root to any desired precision.
Using the long division method, we start by approximating \( \sqrt{6} \). The steps are as follows:
Initial Guess: Since 6 lies between \( 2^2 \) (which is 4) and \( 3^2 \) (which is 9), we know that \( \sqrt{6} \) is between 2 and 3. A good initial guess is 2.5.
Square the Guess: \( 2.5^2 = 6.25 \). Since 6.25 is greater than 6, we adjust our guess downward.
Refine the Guess: We continue this process with more precise values. Next, try 2.45:
- \( 2.45^2 = 6.0025 \) (which is very close to 6)
Further Refinement: For more precision, we continue the long division method:
| Step | Dividend | Divisor | Quotient | Remainder |
| 1 | 600 | 4 | 2 | 2 |
| 2 | 200 | 40 | 4 | 16 |
| 3 | 2400 | 484 | 5 | 360 |
| 4 | 36000 | 4885 | 7 | 2305 |
Continuing this process, the decimal approximation of \( \sqrt{6} \) is found to be approximately 2.44948974278..., and it goes on indefinitely without repeating. This clearly indicates the irrational nature of \( \sqrt{6} \).
Therefore, the decimal expansion of \( \sqrt{6} \) demonstrates the properties of an irrational number, showcasing a non-terminating and non-repeating pattern.

Historical Context and Discovery
The concept of square roots has a rich history that dates back to ancient civilizations. The square root of 6, while not as historically significant as the discovery of the square root of 2, still shares a fascinating backdrop in the broader context of mathematical discovery and the understanding of irrational numbers.
One of the earliest recorded uses of square roots comes from ancient Babylon. The Babylonians, around 1800 BC to 1600 BC, created clay tablets such as YBC 7289, which included calculations for the square root of 2. These computations were remarkably accurate, demonstrating an advanced understanding of numerical methods and approximations.
The Egyptians also had knowledge of square roots, as evidenced by the Rhind Mathematical Papyrus from around 1650 BC. This document is a copy of an even older text and shows that the Egyptians used inverse proportion methods to find square roots.
In ancient India, the Sulba Sutras (circa 800–500 BC) provided methods for approximating the square roots of 2 and 3, showcasing an early grasp of irrational numbers. The mathematician Apastamba (circa 600 BC) offered a precise approximation for the square root of 2, accurate to several decimal places, indicating a deep understanding of these concepts.
Ancient Greek mathematicians also played a crucial role in the development of the theory of irrational numbers. The Greeks, particularly through the works of Pythagoras and later Euclid, understood that the square roots of non-perfect squares, like 6, were irrational. This realization was a significant milestone in mathematical history. Euclid’s Elements, dating back to around 300 BC, included proofs of the irrationality of square roots of numbers that are not perfect squares, laying the foundation for much of modern number theory.
The discovery of irrational numbers was a profound moment for Greek mathematics, as it challenged the then-prevailing notion that all numbers could be expressed as ratios of integers. This revelation is often attributed to the Pythagoreans, and it fundamentally changed the course of mathematical thought.
Today, the square root of 6 is recognized as an irrational number, a concept that has been deeply explored and understood through centuries of mathematical advancement. This historical journey highlights the progressive nature of mathematical discovery and the continuous quest for knowledge.
Importance in Mathematics
The square root of 6, being an irrational number, holds significant importance in various fields of mathematics and its applications. Understanding its properties and behavior enriches our comprehension of numerical systems and their complexities.
1. Fundamental Concepts
The square root of 6 exemplifies irrational numbers, which cannot be expressed as a ratio of two integers. This property challenges our understanding of numbers and helps develop advanced mathematical theories.
2. Mathematical Proofs
The irrationality of the square root of 6 can be proven using methods like the contradiction method, which are foundational techniques in higher mathematics. These proofs enhance logical reasoning and problem-solving skills.
3. Role in Algebra
Irrational numbers like the square root of 6 are crucial in solving algebraic equations, particularly quadratic equations. Their presence in the roots of polynomials influences the study and classification of polynomial equations.
4. Geometry and Trigonometry
The square root of 6 appears in various geometric contexts, such as calculating lengths and areas involving irrational distances. It also plays a role in trigonometric identities and equations, contributing to the study of angles and periodic functions.
5. Calculus and Analysis
In calculus, the behavior of irrational numbers like the square root of 6 is essential for understanding limits, continuity, and differentiability. These concepts are foundational in analyzing functions and their behavior.
6. Real-World Applications
Irrational numbers are not just theoretical constructs but also have practical applications in fields such as physics, engineering, and computer science. For example, they are used in calculations involving wave frequencies, electrical circuits, and algorithm design.
7. Philosophical Implications
The discovery and understanding of irrational numbers have profound philosophical implications, challenging the notion of numerical completeness and promoting the idea that there are always new, uncharted territories in mathematics.
Overall, the square root of 6, as an irrational number, plays a crucial role in advancing mathematical thought, providing a deeper insight into the nature of numbers, and fostering developments across various mathematical disciplines.
Applications of Irrational Numbers
Irrational numbers, such as the square root of 6, play a crucial role in various fields of mathematics and science. Their unique properties make them indispensable in many practical applications. Here are some key areas where irrational numbers are utilized:
- Geometry: Irrational numbers frequently appear in geometry, especially in relation to shapes and figures. For example, the diagonal of a square with side length 1 is the square root of 2, an irrational number. Similarly, the square root of 6 can be used to calculate distances and measurements in more complex geometric problems.
- Trigonometry: Many trigonometric functions yield irrational numbers. For instance, the sine and cosine of non-special angles often result in irrational values. These numbers are essential for precise calculations in fields such as engineering and physics.
- Calculus: Irrational numbers are prevalent in calculus. They appear in limits, integrals, and series expansions. For example, the value of certain integrals involving square roots can be irrational, which helps in finding exact solutions to complex problems.
- Physics: In physics, irrational numbers are used to describe quantities that cannot be expressed as simple fractions. For instance, the period of a simple pendulum with a given length involves the square root of the gravitational constant divided by the length, often resulting in an irrational number.
- Engineering: Engineers use irrational numbers in the design and analysis of systems. For example, when determining the natural frequencies of mechanical systems, the square roots of certain parameters often result in irrational numbers, which are crucial for accurate modeling and prediction.
- Computer Science: In computer graphics and simulations, irrational numbers are used to create realistic models and animations. Algorithms that involve rotation, scaling, and other transformations often rely on irrational numbers for precision and accuracy.
- Finance: In finance, irrational numbers can appear in various calculations, such as those involving compound interest and risk assessment. Accurate financial models often require the use of these numbers to ensure precise results.
The significance of irrational numbers extends beyond these specific examples. They are fundamental to our understanding of the mathematical world and its applications, providing the necessary precision and depth required for advanced scientific and engineering endeavors.
Common Misconceptions
There are several common misconceptions regarding the square root of 6 and irrational numbers in general. Understanding these misconceptions can help clarify the true nature of irrational numbers and their properties.
- Misconception 1: All Square Roots are Rational
Many people assume that all square roots are rational numbers, especially when they are familiar with simple cases like \(\sqrt{4} = 2\). However, this is not true for most numbers. The square root of 6, for example, is irrational because it cannot be expressed as a fraction of two integers.
- Misconception 2: Decimal Representation Indicates Rationality
Another common misconception is that if a number has a decimal representation, it must be rational. In reality, the square root of 6 has a non-terminating and non-repeating decimal expansion, which is a hallmark of irrational numbers. The decimal representation of \(\sqrt{6}\) is approximately 2.449489742783178, and it continues infinitely without repeating.
- Misconception 3: Irrational Numbers Can Be Approximated as Rational
While it is true that we can approximate irrational numbers with rational numbers for practical purposes, this does not change their irrational nature. For instance, approximating \(\sqrt{6}\) as 2.45 is useful in calculations but does not imply that \(\sqrt{6}\) is rational.
- Misconception 4: Multiplying an Irrational Number by a Rational Number Yields a Rational Number
Multiplying an irrational number by a rational number does not necessarily result in a rational number. For example, \(2 \times \sqrt{6} = 2\sqrt{6}\) is still irrational. This highlights the persistent nature of irrationality in certain mathematical operations.
- Misconception 5: All Non-Integer Square Roots are Irrational
While many non-integer square roots are irrational, this is not a universal rule. For example, \(\sqrt{1/4} = 1/2\), which is a rational number. Each case must be evaluated individually to determine if a square root is rational or irrational.
By understanding these misconceptions, we can better appreciate the unique characteristics of irrational numbers like the square root of 6 and their role in mathematics.

Video giải thích khái niệm căn bậc hai bằng tiếng Việt. Tìm hiểu cách tính căn bậc hai và ứng dụng của nó trong toán học với Mr. J.
Căn Bậc Hai Là Gì? | Toán Học với Mr. J
READ MORE:
Video giải thích lý do tại sao căn bậc hai của bất kỳ số nguyên nào cũng là số vô tỷ, trừ các số chính phương. Tìm hiểu chi tiết về chứng minh toán học trong video này.
Chứng Minh Rằng Căn Bậc Hai của Bất Kỳ Số Nguyên Nào Cũng Là Số Vô Tỷ (Trừ Các Số Chính Phương)