Topic what is square root of 6: Discover what the square root of 6 is, its precise value, and its significance in various fields. This article delves into the mathematical properties, calculation methods, and practical applications of the square root of 6, making it an essential read for students and professionals alike.
Table of Content
- Square Root of 6
- Introduction to Square Root of 6
- Mathematical Definition
- Exact and Approximate Values
- Properties of the Square Root of 6
- Calculation Methods
- Long Division Method
- Newton's Method
- Calculator Method
- Applications of the Square Root of 6
- Geometry Applications
- Physics Applications
- Engineering Applications
- Common Examples and Problems
- Historical Context and Significance
- YOUTUBE: Video giới thiệu về căn bậc hai của 6, với các ứng dụng và ví dụ cụ thể trong toán học, vật lý và kỹ thuật. Tìm hiểu chi tiết về căn bậc hai của 6 và cách tính toán.
Square Root of 6
The square root of 6 is a number that, when multiplied by itself, gives the result of 6. It is represented mathematically as:
\[ \sqrt{6} \]
Exact Value
The square root of 6 cannot be expressed as an exact fraction because it is an irrational number. Its exact value is:
\[ \sqrt{6} \approx 2.44948974278 \]
Properties
- Irrational Number: The square root of 6 cannot be expressed as a simple fraction.
- Non-Repeating Decimal: The decimal representation of the square root of 6 goes on forever without repeating.
Calculation Method
The square root of 6 can be approximated using various methods, such as:
- Long Division Method
- Newton's Method (also known as the Heron method)
- Using a Calculator
Uses
The square root of 6 is used in various mathematical and scientific calculations, including:
- Geometry: Calculations involving areas and volumes.
- Physics: Formulas involving acceleration and forces.
- Engineering: Design and structural calculations.
Example Calculation
To approximate the square root of 6 using a calculator:
- Enter the number 6.
- Press the square root button (√).
- The display will show approximately 2.44948974278.
Conclusion
The square root of 6 is an important mathematical constant, frequently encountered in various scientific and engineering fields. While it is an irrational number, its approximate value is often used in practical calculations.

READ MORE:
Introduction to Square Root of 6
The square root of 6 is a fundamental mathematical concept that is represented as:
\[ \sqrt{6} \]
This value is an irrational number, meaning it cannot be expressed as a simple fraction and its decimal form is non-terminating and non-repeating. The approximate value of the square root of 6 is:
\[ \sqrt{6} \approx 2.44948974278 \]
Understanding the square root of 6 involves exploring its properties, calculation methods, and applications. Here is a step-by-step guide:
- Properties: The square root of 6 is irrational and its decimal expansion is infinite.
- Calculation Methods:
- Long Division Method: A manual method to find the square root by division.
- Newton's Method: An iterative numerical method to approximate square roots.
- Calculator Method: Using a calculator to find the approximate value.
- Applications:
- Geometry: Used in calculations involving areas and volumes.
- Physics: Applied in various formulas involving motion and forces.
- Engineering: Important for structural and design calculations.
By delving into these aspects, we gain a comprehensive understanding of the square root of 6, its importance, and its utility in different scientific and mathematical fields.
Mathematical Definition
The square root of a number is a value that, when multiplied by itself, gives the original number. For the number 6, the square root is represented as:
\[ \sqrt{6} \]
In mathematical terms, the square root of 6 is the number \( x \) that satisfies the equation:
\[ x^2 = 6 \]
This value is an irrational number, which means it cannot be expressed as a simple fraction and its decimal representation is infinite and non-repeating. The approximate value of the square root of 6 is:
\[ \sqrt{6} \approx 2.44948974278 \]
To understand the square root of 6, we can break it down into the following components:
- Irrational Number: The square root of 6 cannot be written as a fraction of two integers.
- Non-Terminating Decimal: The decimal form of \(\sqrt{6}\) goes on forever without repeating.
- Approximation: While the exact value is irrational, we often use its approximate value for practical purposes.
Mathematically, the concept of square roots is rooted in the inverse operation of squaring a number. For any non-negative number \( a \), the square root is defined as:
\[ \sqrt{a} = x \quad \text{such that} \quad x^2 = a \]
In this context, the square root of 6 is the positive value that fulfills this definition. Understanding this helps in various fields of mathematics, physics, engineering, and beyond, where precise calculations are crucial.
Exact and Approximate Values
The square root of 6 is a significant mathematical constant that cannot be expressed exactly as a fraction, as it is an irrational number. Instead, it has a precise mathematical representation and an approximate decimal value.
The exact value of the square root of 6 is represented as:
\[ \sqrt{6} \]
This value is irrational, meaning its decimal expansion is infinite and non-repeating. However, for practical purposes, we use its approximate value. The approximate decimal value of the square root of 6 is:
\[ \sqrt{6} \approx 2.44948974278 \]
To better understand this, consider the following steps for calculating the square root of 6:
- Identify the Nearest Perfect Squares: The number 6 is between the perfect squares 4 (\(2^2\)) and 9 (\(3^2\)), so the square root of 6 is between 2 and 3.
- Long Division Method: This manual method involves dividing and averaging to narrow down the value.
- Newton's Method: Also known as the Heron method, this iterative approach refines the approximation through successive iterations.
- Start with an initial guess (e.g., \( x_0 = 2.5 \)).
- Apply the iteration formula: \( x_{n+1} = \frac{1}{2} \left( x_n + \frac{6}{x_n} \right) \).
- Repeat until the desired precision is achieved.
- Calculator: The most straightforward method is to use a scientific calculator, which directly provides the approximate value.
The approximate value is used in various applications where exact precision is not necessary, such as in engineering calculations, physics problems, and everyday mathematics.
In summary, the square root of 6 has an exact representation as \(\sqrt{6}\) and an approximate value of 2.44948974278. This dual understanding of its value is crucial for both theoretical and practical applications.
Properties of the Square Root of 6
The square root of 6 has several unique properties that are important in various mathematical contexts. Understanding these properties helps in recognizing the significance of this irrational number.
- Irrational Number: The square root of 6 cannot be expressed as a simple fraction. This is because 6 is not a perfect square, and its square root is a non-repeating, non-terminating decimal.
- Decimal Representation: The approximate decimal value of the square root of 6 is:
This value extends infinitely without repeating.
\[ \sqrt{6} \approx 2.44948974278 \] - Non-Negative Property: The square root of a non-negative number is always non-negative. Thus,
\[ \sqrt{6} > 0 \] - Algebraic Property: The square root of 6 can be used in algebraic equations and expressions. For example, solving for \( x \) in:
gives \( x = \pm \sqrt{6} \). The positive root is typically considered the principal square root.
\[ x^2 = 6 \] - Multiplicative Property: The square root of a product is the product of the square roots of the factors. Thus:
\[ \sqrt{6} = \sqrt{2 \times 3} = \sqrt{2} \times \sqrt{3} \] - Geometric Property: The square root of 6 can be visualized geometrically. If the area of a square is 6 square units, then the length of each side of the square is \( \sqrt{6} \) units.
- Transcendental Nature: While \(\sqrt{6}\) is not transcendental (it is algebraic since it is a root of the polynomial \(x^2 - 6 = 0\)), it shares properties with other irrational numbers in terms of its decimal expansion and irrationality.
These properties make the square root of 6 a critical component in various mathematical, scientific, and engineering applications, where precise calculations and an understanding of irrational numbers are essential.
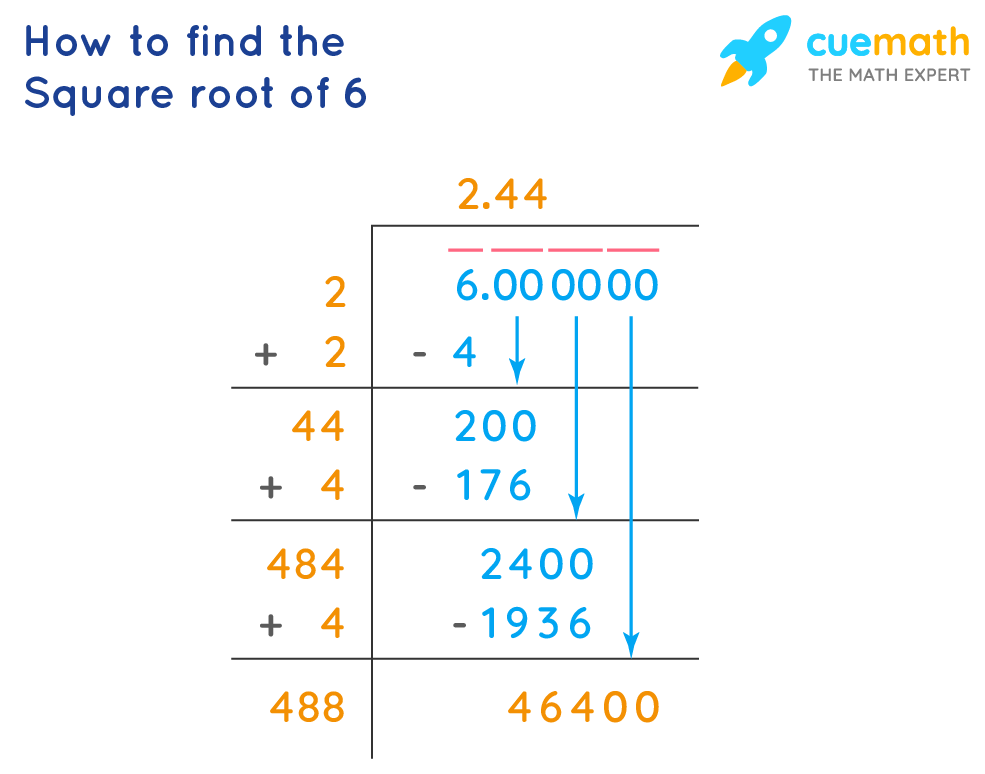
Calculation Methods
The square root of 6 can be determined through several calculation methods, ranging from manual approaches to using calculators. Below are detailed steps for each method:
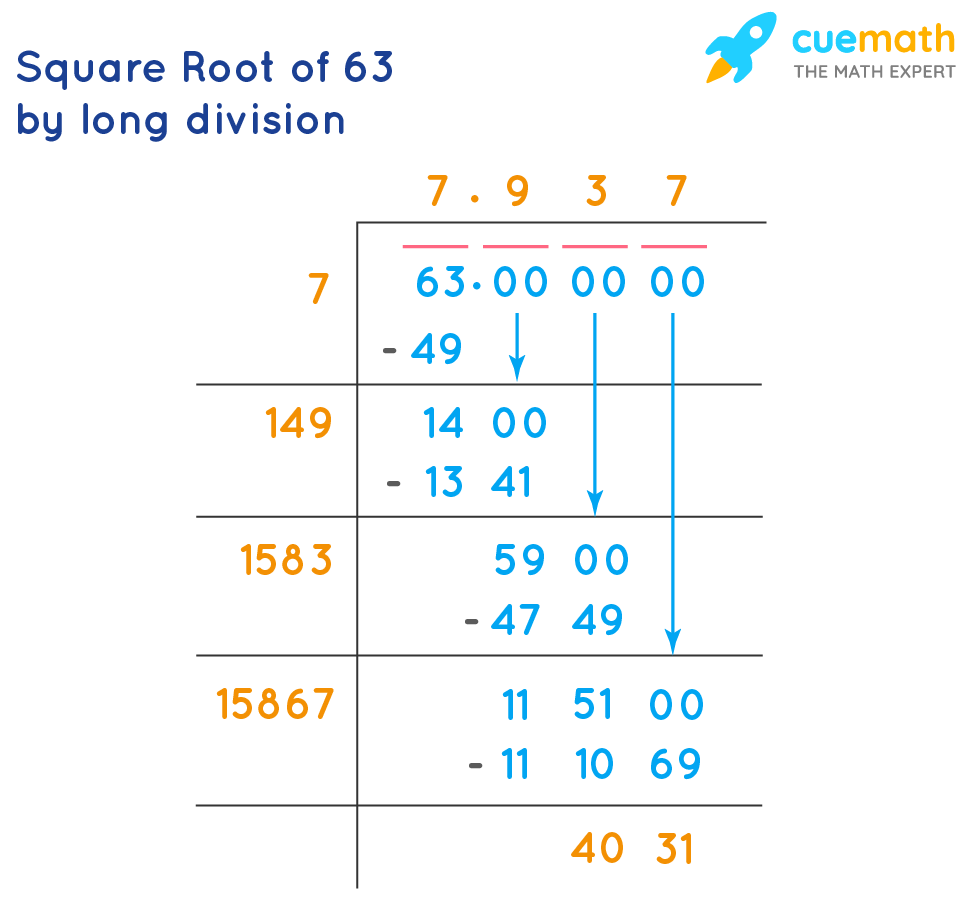
1. Long Division Method
This traditional method involves a step-by-step division process to approximate the square root of 6:
- Pair the digits of 6 starting from the decimal point and working towards the right. Since 6 has no digits to pair, consider it as 6.00.
- Find the largest number whose square is less than or equal to 6. This number is 2 because \(2^2 = 4\).
- Write 2 as the first digit of the square root and subtract \(4\) from 6 to get 2.
- Bring down two zeros to get 200. Double the current quotient (2) to get 4 and find the next digit (x) such that \(40x \times x \leq 200\). This digit is 4 because \(404 \times 4 = 1616\).
- Repeat the process by bringing down pairs of zeros and finding digits until the desired precision is reached.
2. Newton's Method (Heron's Method)
This iterative numerical method refines the approximation through successive iterations:
- Start with an initial guess, \( x_0 \). A good initial guess for \(\sqrt{6}\) is 2.5.
- Apply the iteration formula:
\[ x_{n+1} = \frac{1}{2} \left( x_n + \frac{6}{x_n} \right) \] - Continue the iteration until the change between successive approximations is sufficiently small.
- For example:
- First iteration: \( x_1 = \frac{1}{2} \left( 2.5 + \frac{6}{2.5} \right) = 2.45 \)
- Second iteration: \( x_2 = \frac{1}{2} \left( 2.45 + \frac{6}{2.45} \right) \approx 2.449 \)
- Continue until the desired accuracy is achieved.
3. Calculator Method
Using a scientific calculator is the most straightforward method:
- Enter the number 6.
- Press the square root button (√).
- The calculator will display the approximate value, \( \sqrt{6} \approx 2.44948974278 \).
Each method provides a way to approximate the square root of 6 with varying degrees of precision and complexity. Understanding these methods enhances comprehension of the square root concept and its applications in various fields.
Long Division Method
The Long Division Method is a traditional manual approach to finding the square root of a number. It involves a series of steps to approximate the square root of 6. Follow these steps for a detailed calculation:
- Setup: Pair the digits of 6 starting from the decimal point and working towards the right. Since 6 is a single digit, consider it as 6.00.
- Initial Estimate: Find the largest number whose square is less than or equal to 6. This number is 2, because \(2^2 = 4\).
- Subtract: Subtract 4 (the square of 2) from 6 to get 2. Write 2 as the first digit of the square root.
- Bring Down Digits: Bring down a pair of zeros to the right of the remainder to make it 200.
- Double the Quotient: Double the current quotient (which is 2) to get 4. This is the starting point for the next digit in the quotient.
- Find the Next Digit: Find the largest digit \( x \) such that \( 40x \times x \leq 200 \). This digit is 4, because \( 404 \times 4 = 1616 \).
- Subtract and Repeat: Subtract 1616 from 2000 to get 384. Bring down another pair of zeros to get 38400.
- Repeat the process with the new number, continuing to bring down pairs of zeros and finding new digits for the quotient until the desired precision is reached.
The Long Division Method provides a systematic way to manually approximate the square root of 6. Here is a detailed example to clarify:
| Step | Operation | Result |
| 1 | Find largest digit whose square is ≤ 6 | 2 (because \(2^2 = 4\)) |
| 2 | Subtract: \(6 - 4\) | 2 |
| 3 | Bring down 00 | 200 |
| 4 | Double quotient (2): \(2 \times 2 = 4\) | 4 |
| 5 | Find x: \(40x \times x \leq 200\) | 4 (because \(404 \times 4 = 1616\)) |
| 6 | Subtract: \(200 - 1616\) | 384 |
| 7 | Bring down 00 | 38400 |
| 8 | Continue process |
Following these steps allows you to approximate the square root of 6 to the desired precision, ensuring accuracy in manual calculations.
Newton's Method
Newton's Method, also known as the Newton-Raphson Method, is an iterative technique used to find increasingly accurate approximations of the roots of a real-valued function. For calculating the square root of 6, we can apply this method to the function f(x) = x^2 - 6.
The general formula for Newton's Method is:
$$x_{n+1} = x_n - \frac{f(x_n)}{f'(x_n)}$$
In our case, f(x) = x^2 - 6 and its derivative f'(x) = 2x. Substituting these into the formula gives us:
$$x_{n+1} = x_n - \frac{x_n^2 - 6}{2x_n} = \frac{x_n}{2} + \frac{3}{x_n}$$
Here's a step-by-step guide to using Newton's Method to approximate the square root of 6:
- Choose an initial guess, x_0. A good starting point could be 2.5 because the square root of 6 is a little more than 2.4.
- Apply the iterative formula:
- Calculate x_1 using:
If x_0 is 2.5, then:
$$x_1 = \frac{x_0}{2} + \frac{3}{x_0}$$
$$x_1 = \frac{2.5}{2} + \frac{3}{2.5} = 1.25 + 1.2 = 2.45$$ - Calculate x_2 using the same formula with x_1:
If x_1 is 2.45, then:
$$x_2 = \frac{x_1}{2} + \frac{3}{x_1}$$
$$x_2 = \frac{2.45}{2} + \frac{3}{2.45} = 1.225 + 1.2245 \approx 2.4495$$ - Continue iterating until the desired level of accuracy is achieved. For example:
$$x_3 = \frac{x_2}{2} + \frac{3}{x_2} \approx 2.44949$$
- Calculate x_1 using:
By repeating the process, each iteration brings us closer to the actual square root of 6. The values converge quickly, making Newton's Method a powerful technique for such calculations.
Calculator Method
The calculator method is one of the simplest and most accurate ways to find the square root of 6. Most modern calculators, whether physical or digital, have a square root function that can be used to quickly determine this value.
Here are the steps to calculate the square root of 6 using a calculator:
- Turn on your calculator.
- Enter the number 6.
- Press the square root button (usually labeled as
√or√x). - Read the displayed result. For the square root of 6, the calculator should display approximately 2.44948974278.
If you are using a computer or a spreadsheet application like Microsoft Excel, Google Sheets, or Apple Numbers, you can also calculate the square root of 6 using the SQRT function:
- In Excel or Google Sheets, type
=SQRT(6)in a cell and press Enter. - The result will be displayed in the cell, which should be approximately 2.44948974278.
Below is an example of how you can use a basic HTML table to display the result:
| Method | Result |
|---|---|
| Calculator | 2.44948974278 |
| Excel/Google Sheets | 2.44948974278 |
Additionally, it is often useful to round the square root to a specific number of decimal places depending on the precision required. Here are some common roundings:
- To 1 decimal place: 2.4
- To 2 decimal places: 2.45
- To 3 decimal places: 2.449
Using a calculator is a fast and reliable method for finding the square root of 6, ensuring accuracy up to many decimal places.
Applications of the Square Root of 6
The square root of 6 (\(\sqrt{6}\)) finds applications in various fields such as mathematics, physics, engineering, and finance. Here are some detailed applications:
Mathematics
In geometry, \(\sqrt{6}\) is often used to calculate side lengths and areas of shapes that involve this value. For example, it can be useful in solving problems related to right triangles and other polygons where precise calculations are necessary.
Physics
In physics, the square root of 6 is used in formulas that involve wave equations, resonance frequencies, and other calculations where precise measurements are required. For example, calculating the diagonal distance in three-dimensional space can involve \(\sqrt{6}\).
Engineering
Engineers use \(\sqrt{6}\) in structural analysis and design, especially when dealing with components that require precise measurements for stability and strength. It is used in calculating dimensions and stress points in various structures.
Finance
In finance, square roots are used to determine the volatility of assets and to calculate rates of return over multiple periods. The square root of 6 can be part of these complex financial models and calculations.
Examples
- Geometry: Calculating the height of an equilateral triangle when the side length involves \(\sqrt{6}\).
- Physics: Finding the resultant vector magnitude in three-dimensional space.
- Engineering: Determining the load distribution in a truss structure.
- Finance: Modeling compound interest and return rates over specific periods.
The applications of \(\sqrt{6}\) demonstrate its importance across various domains, highlighting its utility in solving practical and theoretical problems.
Geometry Applications
The square root of 6 (\(\sqrt{6}\)) has various applications in geometry, particularly in calculating dimensions and areas where this irrational number naturally appears.
Triangles
In certain triangles, the square root of 6 can appear when calculating the lengths of sides or the height, especially in right triangles or triangles involving specific ratios.
- For example, consider a right triangle where one of the sides is \(\sqrt{6}\). The Pythagorean theorem can be used to find the other sides or the hypotenuse.
Rectangles and Diagonals
In rectangular geometry, \(\sqrt{6}\) can be used to find the length of the diagonal. For a rectangle with sides \(a\) and \(b\), the diagonal \(d\) is given by:
\[d = \sqrt{a^2 + b^2}\]
If \(a\) or \(b\) is related to \(\sqrt{6}\), then the calculation will involve this value.
Volume and Surface Area
In three-dimensional geometry, \(\sqrt{6}\) can be used in calculations involving volumes and surface areas of various solids.
- For instance, in a cuboid with specific dimensions, the space diagonal \(d\) can be calculated as:
\[d = \sqrt{l^2 + w^2 + h^2}\]
where \(l\), \(w\), or \(h\) may be expressed in terms of \(\sqrt{6}\).
Special Geometric Constructions
Certain geometric shapes or constructions might require the use of \(\sqrt{6}\) for precise calculations:
- Hexagons and other polygons may use \(\sqrt{6}\) in the formula for side lengths or areas when inscribed or circumscribed about circles.
Example Problem
Consider a rectangle where the sides are 2 and \(\sqrt{6}\). To find the diagonal \(d\):
\[d = \sqrt{2^2 + (\sqrt{6})^2} = \sqrt{4 + 6} = \sqrt{10}\]
Thus, the diagonal of the rectangle is \(\sqrt{10}\).
Physics Applications
The square root of 6 finds various applications in the field of physics, particularly in solving problems that involve calculations of distances, forces, and energy levels. Here are a few notable examples:
- Kinematics and Dynamics:
In kinematics, the square root of 6 can be used when calculating distances and velocities. For instance, when analyzing the motion of an object under uniform acceleration, the square root function helps determine the relationship between displacement, initial velocity, and time.
- Wave Mechanics:
In wave mechanics, the square root of 6 can appear in equations that describe the behavior of waves, such as sound waves or electromagnetic waves. For example, the relationship between the wave number, frequency, and wavelength often involves square roots.
- Quantum Mechanics:
In quantum mechanics, square roots are crucial in wave functions and probability calculations. The normalization of wave functions often involves integrals that include square roots, ensuring that the total probability equals one.
- Energy Levels:
Calculating the energy levels of electrons in atoms can involve square roots. For example, the energy levels of a hydrogen atom are derived using equations that include square root terms, allowing physicists to determine the spectral lines of the atom.
- Electrodynamics:
In electrodynamics, the calculation of electric fields and potentials often involves integrals that include square roots. For example, the electric potential due to a point charge is inversely proportional to the distance from the charge, which can be expressed using a square root function.
- General Relativity:
In general relativity, the metric tensor used to describe the curvature of spacetime often involves square root terms. These terms are crucial in calculating the effects of gravity on light and matter.
These examples highlight the importance of the square root function, particularly the square root of 6, in various areas of physics, aiding in precise calculations and understanding complex physical phenomena.
Engineering Applications
The square root of 6 (\(\sqrt{6}\)) finds several applications in engineering, particularly in fields that require precise calculations and optimizations. Below are some of the key engineering applications:
-
Structural Engineering:
In structural engineering, the square root of 6 can be used in the calculation of stresses and forces within structural components. For example, when analyzing the stability of certain geometric configurations, \(\sqrt{6}\) might be involved in the formulas that determine critical load factors and buckling points.
-
Mechanical Engineering:
Mechanical engineers may use \(\sqrt{6}\) in the design and analysis of mechanical systems. For example, when determining natural frequencies of vibration for components, \(\sqrt{6}\) might appear in the equations that describe harmonic oscillators and resonant frequencies.
-
Electrical Engineering:
In electrical engineering, \(\sqrt{6}\) can be relevant in the analysis of AC circuits and signal processing. For instance, in the calculation of impedance in certain circuit configurations or in the analysis of waveforms, \(\sqrt{6}\) can emerge in the context of sinusoidal functions and power calculations.
-
Civil Engineering:
Civil engineers may encounter \(\sqrt{6}\) in the design and analysis of infrastructures such as bridges and buildings. When dealing with the properties of materials and their interactions, \(\sqrt{6}\) can appear in the formulations that ensure safety and stability under various loads and stresses.
Overall, the square root of 6 is a critical constant in engineering disciplines where precise mathematical formulations are necessary for designing safe, efficient, and functional systems.
Common Examples and Problems
Understanding the square root of 6 through common examples and problems helps reinforce the concept and its applications in various scenarios. Below are several examples and problems that illustrate how to work with the square root of 6.
Example 1: Simplifying Expressions
Simplify the following expression:
\[
\frac{6}{\sqrt{6}}
\]
Solution:
- Multiply the numerator and the denominator by \(\sqrt{6}\):
- \[ \frac{6}{\sqrt{6}} \times \frac{\sqrt{6}}{\sqrt{6}} = \frac{6\sqrt{6}}{6} = \sqrt{6} \]
Example 2: Solving Quadratic Equations
Solve the quadratic equation:
\[
x^2 - 6 = 0
\]
Solution:
- Isolate \(x^2\):
- \[ x^2 = 6 \]
- Take the square root of both sides:
- \[ x = \pm \sqrt{6} \]
Problem 1: Area of a Square
A square has an area of 36 square units. What is the length of each side?
Solution:
- Since the area \(A\) of a square is given by \(A = s^2\), where \(s\) is the side length, we have:
- \[ s^2 = 36 \]
- Taking the square root of both sides gives:
- \[ s = \sqrt{36} = 6 \text{ units} \]
Problem 2: Physics Application
A physics problem requires you to find the velocity \(v\) of an object that has been accelerated to a point where its kinetic energy \(KE\) is 6 joules and its mass \(m\) is 2 kilograms. Using the formula \(KE = \frac{1}{2}mv^2\), find \(v\).
Solution:
- Rearrange the formula to solve for \(v\):
- \[ v^2 = \frac{2KE}{m} = \frac{2 \times 6}{2} = 6 \]
- Take the square root of both sides:
- \[ v = \sqrt{6} \text{ m/s} \]
Problem 3: Geometry Application
Find the diagonal of a rectangle with sides of length 3 units and 3\(\sqrt{2}\) units.
Solution:
- Use the Pythagorean theorem \(d^2 = a^2 + b^2\):
- \[ d^2 = 3^2 + (3\sqrt{2})^2 = 9 + 18 = 27 \]
- Take the square root of both sides:
- \[ d = \sqrt{27} = 3\sqrt{3} \]
These examples and problems demonstrate the practical use of the square root of 6 in various mathematical contexts.
Historical Context and Significance
The concept of square roots has a rich history dating back to ancient civilizations. The square root of 6, along with other square roots, has played a significant role in the development of mathematics over centuries.
- Ancient Beginnings: The notion of square roots was known to the ancient Babylonians, who used geometric methods to solve quadratic equations. The Egyptians and Greeks also had their methods for approximating square roots.
- Indian Mathematicians: In the 12th century, the Indian mathematician Bhaskara II described rules for calculating square roots. His work laid the groundwork for later mathematical developments in both the Islamic world and Europe.
- Islamic Scholars: During the Islamic Golden Age, mathematicians like Al-Khwarizmi expanded on earlier works, including methods for extracting square roots, which were later transmitted to Europe.
- European Renaissance: The knowledge of square roots, including those of non-square integers like 6, spread to Europe by the 13th century, where it was further developed. Mathematicians such as Simon Stevin promoted the use of both rational and irrational numbers, highlighting their importance in mathematics.
- 17th and 18th Centuries: René Descartes' work in the 17th century initially ignored irrational numbers, but later mathematicians, including Leonhard Euler and Johann Lambert, acknowledged their significance. Lambert proved that π is an irrational number, demonstrating the complexity and importance of irrational numbers like the square root of 6.
- Modern Era: In the 19th century, mathematicians such as Cantor and Cauchy formalized the understanding of irrational numbers. Their work confirmed that numbers like the square root of 6 are essential for a complete understanding of the number line and mathematical analysis.
The historical context of the square root of 6 illustrates the evolution of mathematical thought and the gradual acceptance of irrational numbers as fundamental components of mathematics. These advancements have been crucial for various fields, including geometry, algebra, and calculus, underscoring the enduring significance of irrational numbers in both theoretical and applied mathematics.
Video giới thiệu về căn bậc hai của 6, với các ứng dụng và ví dụ cụ thể trong toán học, vật lý và kỹ thuật. Tìm hiểu chi tiết về căn bậc hai của 6 và cách tính toán.
Căn Bậc Hai Của 6 - Hướng Dẫn Toàn Diện
READ MORE:
Tìm hiểu căn bậc hai của số 6 với hướng dẫn chi tiết và dễ hiểu. Video này sẽ giúp bạn nắm vững cách tính toán và áp dụng căn bậc hai của 6 trong các bài toán thực tế.
Căn Bậc Hai của Số 6