Simplifying Square Root of 48: A Step-by-Step Guide

Simplifying the square root of a number involves finding the prime factors and simplifying the expression to its simplest form. Let's break down the process of simplifying the square root of 48 step by step.

Step-by-Step Guide

1. First, find the prime factors of 48. The prime factorization of 48 is:
   • 48 ÷ 2 = 24
   • 24 ÷ 2 = 12
   • 12 ÷ 2 = 6
   • 6 ÷ 2 = 3
   • 3 ÷ 3 = 1
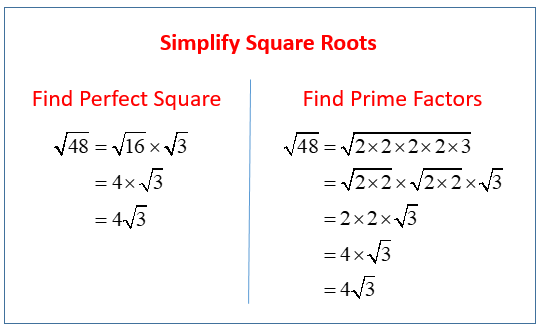
2. This gives us the prime factors: \(2 \times 2 \times 2 \times 2 \times 3\) or \(2^4 \times 3\).
3. Group the prime factors in pairs. For every pair of the same number, you can take one number out of the square root:
   • \(\sqrt{48} = \sqrt{2^4 \times 3} = \sqrt{(2^2)^2 \times 3} = 2^2 \times \sqrt{3}\).
   • This simplifies to \(4\sqrt{3}\).

Conclusion

The simplified form of \(\sqrt{48}\) is \(4\sqrt{3}\). By breaking down the number into its prime factors and pairing them, we can simplify the square root efficiently. Understanding this process can help simplify other square roots in a similar way.

Additional Tips

• Always look for perfect squares within the number.
• Practice prime factorization to make simplification easier.
• Use the properties of square roots to break down complex numbers.

Simplifying square roots is a fundamental skill in mathematics that makes complex calculations more manageable. It involves reducing a square root to its simplest form, making it easier to work with in equations and expressions. Let's explore how to simplify the square root of 48 step by step.

1. Understanding Square Roots: The square root of a number is a value that, when multiplied by itself, gives the original number. For example, \( \sqrt{48} \) represents the number that, when squared, equals 48.
2. Prime Factorization: Begin by finding the prime factors of 48. Prime factorization breaks down a number into its prime components:
   • 48 ÷ 2 = 24
   • 24 ÷ 2 = 12
   • 12 ÷ 2 = 6
   • 6 ÷ 2 = 3
   • 3 ÷ 3 = 1
   Thus, the prime factorization of 48 is \( 2^4 \times 3 \).
3. Grouping Factors: Group the prime factors into pairs. For each pair of the same number, you can take one number out of the square root:
   • \( \sqrt{48} = \sqrt{2^4 \times 3} = \sqrt{(2^2)^2 \times 3} \)
   • This simplifies to \( 2^2 \times \sqrt{3} \), or \( 4\sqrt{3} \).
4. Final Simplified Form: Therefore, the simplified form of \( \sqrt{48} \) is \( 4\sqrt{3} \).

By understanding and applying these steps, you can simplify square roots efficiently, making mathematical calculations more straightforward.

Square roots and radicals are essential concepts in mathematics that help us solve equations and simplify expressions. A square root of a number is a value that, when multiplied by itself, gives the original number. Radicals, on the other hand, refer to symbols that indicate the root of a number, such as the square root symbol (√).

1. Definition of Square Roots:

   The square root of a number \( x \) is written as \( \sqrt{x} \) and represents a value \( y \) such that \( y^2 = x \). For example, \( \sqrt{48} \) is the number that, when squared, equals 48.

2. Properties of Square Roots:
   • Non-negative: Square roots are always non-negative. For example, \( \sqrt{48} \) is positive.
   • Principal Square Root: The principal square root is the non-negative root of a number.
   • Product Property: \( \sqrt{a \times b} = \sqrt{a} \times \sqrt{b} \).
   • Quotient Property: \( \sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}} \).
3. Definition of Radicals:

   Radicals are expressions that include a root symbol. The most common radical is the square root, but there are other types, such as cube roots (∛) and fourth roots (∜).

4. Simplifying Radicals:

   Simplifying radicals involves reducing them to their simplest form by removing perfect squares from under the root symbol. For example, to simplify \( \sqrt{48} \), we find the prime factorization of 48 and group the factors to get \( 4\sqrt{3} \).

By understanding square roots and radicals, you can tackle a wide range of mathematical problems with confidence and ease.

The prime factorization method is a systematic way to simplify square roots by breaking down a number into its prime factors. This method helps identify pairs of factors that can be taken out of the square root, simplifying the expression.

1. Identify the Number:

   Start with the number you want to simplify. In this case, we are simplifying \( \sqrt{48} \).

2. Find the Prime Factors:

   Break down 48 into its prime factors. Divide the number by the smallest prime number (2) and continue dividing the quotient by prime numbers until you reach 1.

   • 48 ÷ 2 = 24
   • 24 ÷ 2 = 12
   • 12 ÷ 2 = 6
   • 6 ÷ 2 = 3
   • 3 ÷ 3 = 1

   The prime factorization of 48 is \( 2^4 \times 3 \).

3. Group the Prime Factors:

   Group the prime factors in pairs. Each pair of the same number can be taken out of the square root as a single number.

   • \( \sqrt{48} = \sqrt{2^4 \times 3} \)
   • \( \sqrt{2^4 \times 3} = \sqrt{(2^2)^2 \times 3} \)
   • \( \sqrt{(2^2)^2 \times 3} = 2^2 \times \sqrt{3} \)

   This simplifies to \( 4\sqrt{3} \).

4. Write the Simplified Form:

   The simplified form of \( \sqrt{48} \) is \( 4\sqrt{3} \). This process reduces the original square root to a more manageable expression.

The prime factorization method is an effective way to simplify square roots, making calculations easier and more efficient.

Simplifying the square root of 48 involves breaking it down into its prime factors and then simplifying the expression. Here is a detailed step-by-step process:

1. Prime Factorization:

   First, we find the prime factors of 48. The prime factorization of 48 is:

   \[ 48 = 2 \times 2 \times 2 \times 2 \times 3 \]

   In exponent form, this can be written as:

   \[ 48 = 2^4 \times 3^1 \]

2. Group the Factors:

   Next, we group the prime factors into pairs of the same number:

   \[ \sqrt{48} = \sqrt{2 \times 2 \times 2 \times 2 \times 3} \]

   This can be grouped as:

   \[ \sqrt{48} = \sqrt{(2 \times 2) \times (2 \times 2) \times 3} \]

3. Extract the Square Roots:

   We know that the square root of a product is the product of the square roots of the factors. Therefore, we take the square root of each pair:

   \[ \sqrt{48} = \sqrt{(2 \times 2)} \times \sqrt{(2 \times 2)} \times \sqrt{3} \]

   Since \(\sqrt{2 \times 2} = 2\), we get:

   \[ \sqrt{48} = 2 \times 2 \times \sqrt{3} \]

4. Simplify the Expression:

   Multiply the extracted square roots:

   \[ \sqrt{48} = 4 \times \sqrt{3} \]

5. Final Simplified Form:

   Thus, the simplified form of the square root of 48 is:

   \[ \sqrt{48} = 4\sqrt{3} \]

By following these steps, you can simplify the square root of any number by breaking it down into its prime factors and simplifying the radicals.

Simplifying the square root of 48 involves breaking it down into its prime factors and then applying the product property of square roots. Here's a step-by-step guide:

1. Start with the number 48:

   \( \sqrt{48} \)

2. Find the prime factorization of 48:

   48 = 2 × 2 × 2 × 2 × 3

3. Rewrite the square root of 48 using its prime factors:

   \( \sqrt{48} = \sqrt{2 \times 2 \times 2 \times 2 \times 3} \)

4. Group the prime factors into pairs of identical factors:

   \( \sqrt{2 \times 2 \times 2 \times 2 \times 3} = \sqrt{(2 \times 2) \times (2 \times 2) \times 3} \)

5. Take one factor out of each pair from under the square root sign:

   \( \sqrt{(2 \times 2) \times (2 \times 2) \times 3} = 2 \times 2 \times \sqrt{3} \)

6. Multiply the factors outside the square root:

   2 × 2 = 4

7. Combine the simplified factors:

   \( 2 \times 2 \times \sqrt{3} = 4\sqrt{3} \)

Therefore, the simplified form of \( \sqrt{48} \) is:

\( 4\sqrt{3} \)

Simplifying square roots can be tricky, and making mistakes is common. Here are some frequent errors to watch out for, along with tips on how to avoid them:

• Not identifying the largest perfect square factor: When simplifying √48, it's important to find the largest perfect square factor of 48, which is 16. A common mistake is to choose smaller factors like 4, which leads to incorrect simplification steps.
• Forgetting to simplify completely: After breaking down the square root into its factors, ensure you simplify all the way. For example, √48 should be simplified to 4√3, not just partially simplified.
• Ignoring the product rule: The product rule states that √(a * b) = √a * √b. Ensure you apply this rule correctly. For instance, √48 can be broken down as √(16 * 3) = √16 * √3 = 4√3.
• Misplacing the radical sign: Make sure the radical sign is placed correctly and consistently in your calculations. Misplacing it can lead to confusion and errors in the final result.
• Incorrect factorization: Properly factorizing the number inside the square root is crucial. For 48, the correct factorization is 2^4 * 3, leading to 4√3 when simplified.
• Overlooking prime factorization: Using prime factorization can help simplify complex square roots. For √48, breaking it down into prime factors (2^4 * 3) helps in identifying the largest perfect square factor.

By keeping these common mistakes in mind and applying the correct methods, you can ensure accurate and simplified results for square roots.

Simplifying square roots offers several key benefits that enhance mathematical comprehension and efficiency. Here are some of the main advantages:

• Ease of Calculation:

  Simplified square roots make calculations easier, especially in more complex mathematical operations. For example, working with \( \sqrt{48} \) is simpler when expressed as \( 4\sqrt{3} \).

• Improved Understanding:

  Simplifying square roots helps students understand the properties of numbers and radicals. This process involves breaking down numbers into their prime factors, which reinforces number theory concepts.

• Consistency in Solutions:

  Simplifying square roots ensures consistency when comparing results or solving equations. For instance, \( \sqrt{48} \) and \( 4\sqrt{3} \) are identical, but the latter form is often easier to recognize and use in further calculations.

• Preparation for Advanced Math:

  Mastering the simplification of square roots prepares students for more advanced topics in algebra and calculus, where simplifying expressions is crucial for solving complex problems.

• Practical Applications:

  Simplified square roots are often used in real-life applications, such as physics, engineering, and computer science, where precise and simplified calculations are necessary.

By understanding and practicing the simplification of square roots, students can enhance their mathematical skills and apply them more effectively in various academic and practical scenarios.

Practicing simplifying square roots helps solidify your understanding and enhances your problem-solving skills. Below are several practice problems, each designed to test different aspects of simplifying square roots.

1. Simplify \( \sqrt{75} \)

   Solution: \( 75 = 25 \times 3 \) and \( \sqrt{25} = 5 \), so \( \sqrt{75} = 5\sqrt{3} \)

2. Simplify \( \sqrt{200} \)

   Solution: \( 200 = 100 \times 2 \) and \( \sqrt{100} = 10 \), so \( \sqrt{200} = 10\sqrt{2} \)

3. Simplify \( \sqrt{98} \)

   Solution: \( 98 = 49 \times 2 \) and \( \sqrt{49} = 7 \), so \( \sqrt{98} = 7\sqrt{2} \)

4. Simplify \( \sqrt{32} \)

   Solution: \( 32 = 16 \times 2 \) and \( \sqrt{16} = 4 \), so \( \sqrt{32} = 4\sqrt{2} \)

5. Simplify \( \sqrt{180} \)

   Solution: \( 180 = 36 \times 5 \) and \( \sqrt{36} = 6 \), so \( \sqrt{180} = 6\sqrt{5} \)

6. Simplify \( \sqrt{128} \)

   Solution: \( 128 = 64 \times 2 \) and \( \sqrt{64} = 8 \), so \( \sqrt{128} = 8\sqrt{2} \)

7. Simplify \( \sqrt{72} \)

   Solution: \( 72 = 36 \times 2 \) and \( \sqrt{36} = 6 \), so \( \sqrt{72} = 6\sqrt{2} \)

These problems provide a range of difficulty to help you practice identifying and simplifying square roots efficiently. Make sure to verify your steps and confirm the prime factorization for accurate results.

Simplifying square roots can be taken to a more advanced level using various techniques. Here are some methods to further simplify square roots:

Using Rational Exponents

Instead of working with radicals, you can use rational exponents to simplify square roots. For example:

1. Express the square root as a fractional exponent: \( \sqrt{48} = 48^{1/2} \).
2. Use the properties of exponents to simplify: \( 48^{1/2} = (16 \cdot 3)^{1/2} = 16^{1/2} \cdot 3^{1/2} = 4\sqrt{3} \).

Combining Like Terms

When simplifying multiple square roots, combine like terms to simplify the expression:

1. Identify square roots with the same radicand: \( 2\sqrt{12} + 3\sqrt{12} \).
2. Add the coefficients while keeping the radicand unchanged: \( (2 + 3)\sqrt{12} = 5\sqrt{12} \).
3. Simplify further if possible: \( 5\sqrt{12} = 5\cdot2\sqrt{3} = 10\sqrt{3} \).

Prime Factorization Method

This method involves breaking down the number under the radical into its prime factors:

1. Factorize the radicand: \( 48 = 2^4 \cdot 3 \).
2. Group the factors into pairs: \( \sqrt{48} = \sqrt{(2^2 \cdot 2^2) \cdot 3} \).
3. Take the square root of each pair: \( \sqrt{48} = 2 \cdot 2 \cdot \sqrt{3} = 4\sqrt{3} \).

Using Absolute Values

Ensure that the result is non-negative by using absolute values when necessary:

1. For expressions like \( \sqrt{(x - 2)^2} \), simplify by considering the absolute value: \( \sqrt{(x - 2)^2} = |x - 2| \).

Working with Complex Numbers

For negative radicands, introduce imaginary units:

1. Express the negative radicand using \( i \): \( \sqrt{-48} = \sqrt{-1 \cdot 48} = \sqrt{-1} \cdot \sqrt{48} = i\sqrt{48} \).
2. Simplify the positive part: \( i\sqrt{48} = i \cdot 4\sqrt{3} = 4i\sqrt{3} \).

Combining Multiple Techniques

In some cases, you may need to combine several techniques for the best simplification:

1. For example, simplify \( \sqrt{75} + \sqrt{12} \):
2. Factorize each radicand: \( \sqrt{75} = \sqrt{25 \cdot 3} = 5\sqrt{3} \) and \( \sqrt{12} = \sqrt{4 \cdot 3} = 2\sqrt{3} \).
3. Combine like terms: \( 5\sqrt{3} + 2\sqrt{3} = 7\sqrt{3} \).

Simplified square roots are more than just a mathematical exercise; they have numerous practical applications in various fields. Here are some real-life scenarios where simplified square roots play a crucial role:

• Engineering: Simplified square roots are fundamental in engineering calculations, particularly in determining dimensions, stresses, and other critical measurements. For example, in structural engineering, the natural frequency of a building or a bridge can be determined using simplified square roots.
• Physics: In physics, many formulas involve square roots. For instance, the formula to calculate the time \( t \) it takes for an object to fall from a certain height \( h \) is \( t = \sqrt{\frac{2h}{g}} \), where \( g \) is the acceleration due to gravity. Simplifying the square root makes it easier to work with and understand these equations.
• Finance: In finance, simplified square roots are used in various calculations, such as determining the volatility of stock prices. The standard deviation, which is often used to measure risk, involves the square root of the variance.
• Architecture: Architects use simplified square roots to calculate dimensions and ensure the structural integrity of buildings. This includes determining the lengths of diagonal braces and other support structures.
• Astronomy: Astronomers use simplified square roots to calculate distances between celestial bodies. For instance, the distance between stars or planets can be found using the Pythagorean theorem, which often requires simplifying square roots.

Understanding and simplifying square roots not only makes mathematical problems easier to solve but also enhances accuracy and efficiency in various professional fields. These applications demonstrate the practical importance of mastering square root simplification.

Simplifying square roots is a fundamental skill in algebra that provides numerous benefits and applications. By mastering this skill, students and professionals can handle more complex mathematical problems with ease and confidence.

• Understanding Fundamentals:

  It is essential to understand the basics of square roots and radicals. Knowing how to identify and factor perfect squares helps in simplifying radical expressions efficiently.

• Application of Product and Quotient Rules:

  Applying the product rule \(\sqrt{a \cdot b} = \sqrt{a} \cdot \sqrt{b}\) and the quotient rule \(\sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}}\) allows for simplification of more complex expressions.

• Step-by-Step Simplification:

  Breaking down the simplification process into clear steps—factoring the radicand, separating the radical expressions, and simplifying—ensures accuracy and understanding.

• Real-Life Applications:

  Simplified square roots are useful in various fields such as physics, engineering, and computer science, where precise calculations are crucial.

• Avoiding Common Mistakes:

  Paying attention to common errors, such as incorrect factorization and improper application of rules, can prevent miscalculations and improve problem-solving skills.

In conclusion, the ability to simplify square roots enhances mathematical proficiency and prepares individuals for advanced mathematical concepts. Consistent practice and application of the techniques discussed will lead to greater confidence and success in mathematics.

• Q: What is the square root of 48 simplified?

  A: The simplified form of the square root of 48 is \(4\sqrt{3}\).

• Q: How do you simplify the square root of 48?

  A: To simplify \(\sqrt{48}\), you need to factor 48 into its prime factors: \(48 = 16 \times 3\). Since 16 is a perfect square, you can write \(\sqrt{48}\) as \(\sqrt{16 \times 3} = \sqrt{16} \times \sqrt{3} = 4\sqrt{3}\).

• Q: Why is simplifying square roots useful?

  A: Simplifying square roots makes it easier to perform arithmetic operations with radicals, helps in solving equations, and is useful in various applications like geometry, physics, and engineering.

• Q: Can all square roots be simplified?

  A: Not all square roots can be simplified to an integer, but many can be simplified to a product involving integers and square roots of smaller numbers. For example, \(\sqrt{50}\) simplifies to \(5\sqrt{2}\).

• Q: What are some common mistakes when simplifying square roots?

  A: Common mistakes include not correctly identifying perfect squares, forgetting to factor completely, and incorrectly simplifying the radical expression. Always ensure to break down the number into its prime factors and simplify step-by-step.

• Q: How do you multiply and divide square roots?

  A: To multiply square roots, you multiply the numbers inside the radicals and then simplify if possible: \(\sqrt{a} \times \sqrt{b} = \sqrt{ab}\). For division, you divide the numbers inside the radicals: \(\frac{\sqrt{a}}{\sqrt{b}} = \sqrt{\frac{a}{b}}\).