Topic how to simplify the square root of 20: Understanding how to simplify the square root of 20 can be straightforward and rewarding. This guide will take you through the step-by-step process, making it easy to follow and apply. Simplifying square roots not only enhances your math skills but also boosts your confidence in handling complex numbers. Let's dive in and simplify √20 together!
Table of Content
- How to Simplify the Square Root of 20
- Introduction to Simplifying Square Roots
- Understanding the Concept of Square Roots
- Prime Factorization Method
- Steps to Simplify √20 Using Prime Factorization
- Step-by-Step Guide
- Long Division Method for Simplifying Square Roots
- Examples of Simplifying Similar Square Roots
- Special Cases and Common Mistakes
- Practical Applications of Simplified Square Roots
- Conclusion and Additional Resources
- YOUTUBE: Video hướng dẫn cách đơn giản hóa căn bậc hai của 20 (√20) một cách chi tiết và dễ hiểu. Phương pháp phân tích số nguyên tố và phép chia dài được sử dụng.
How to Simplify the Square Root of 20
Simplifying the square root of a number involves expressing it in the simplest radical form. Here's a step-by-step guide on how to simplify the square root of 20:
Step 1: Find the Prime Factorization of 20
The first step is to express 20 as a product of prime factors.
20 can be factored into 2 and 10. Then, 10 can be further factored into 2 and 5. So, the prime factorization of 20 is:
\(20 = 2 \times 2 \times 5\)
Step 2: Pair the Prime Factors
Next, we look for pairs of the same prime number. In the prime factorization of 20, we have one pair of 2s.
\(20 = 2^2 \times 5\)
Step 3: Simplify the Radical
According to the property of square roots, \(\sqrt{a \times b} = \sqrt{a} \times \sqrt{b}\), we can separate the pairs from the remaining factors.
\(\sqrt{20} = \sqrt{2^2 \times 5} = \sqrt{2^2} \times \sqrt{5}\)
Since the square root of \(2^2\) is 2, we simplify the expression:
\(\sqrt{2^2} = 2\)
So, \(\sqrt{20} = 2 \times \sqrt{5}\)
Conclusion
Therefore, the simplest form of the square root of 20 is:
\(\sqrt{20} = 2\sqrt{5}\)
By following these steps, you can simplify the square root of 20 or any other number by finding its prime factors, pairing them, and then simplifying the radical expression.

READ MORE:
Introduction to Simplifying Square Roots
Square roots are an essential concept in mathematics, representing a value that, when multiplied by itself, gives the original number. Simplifying square roots means expressing them in their simplest radical form, which can make them easier to work with in various mathematical contexts.
To understand how to simplify square roots, let's start with the square root of 20. The goal is to find its simplest form, which involves breaking down the number inside the radical into its prime factors.
-
Identify the Prime Factors:
First, we factorize 20 into its prime factors:
20 = 2 × 2 × 5
-
Group the Factors:
We look for pairs of prime factors. Here, we have one pair of 2s:
20 = 22 × 5
-
Simplify the Radical:
Take the square root of each pair of factors and move them outside the radical sign:
√20 = √(22 × 5) = 2√5
Thus, the square root of 20 simplifies to 2√5. This form is more manageable and often required in further mathematical operations.
Understanding the process of simplifying square roots helps in solving equations, understanding geometric properties, and dealing with real-world applications such as calculating areas and solving physics problems.
Understanding the Concept of Square Roots
The concept of square roots is fundamental in mathematics and plays a crucial role in various mathematical operations. A square root of a number a is a number x such that x2 = a. In other words, it is a value that, when multiplied by itself, gives the original number.
The symbol for the square root is √, called the radical sign, and the number under the radical sign is called the radicand. For example, the square root of 20 is written as √20.
There are several key properties and rules associated with square roots:
- Non-Negative Property: The square root of a non-negative number is also non-negative. For instance, √20 is approximately 4.472, which is non-negative.
- Product Property: The square root of a product is equal to the product of the square roots of the factors. Mathematically, this is expressed as √(a * b) = √a * √b.
- Quotient Property: The square root of a quotient is equal to the quotient of the square roots. This is written as √(a / b) = √a / √b, where b ≠ 0.
To understand these properties better, consider the example of simplifying √20:
- First, factorize the radicand (20 in this case) into its prime factors: 20 = 2 × 2 × 5.
- Next, apply the product property: √20 = √(2 × 2 × 5).
- Group the prime factors into pairs: √(22 × 5).
- Take the square root of the pairs: √(22) × √5 = 2√5.
Thus, the simplest radical form of √20 is 2√5. This process of simplification helps in reducing the complexity of expressions involving square roots.
Understanding these concepts and properties of square roots is essential for solving various mathematical problems and for performing operations involving square roots efficiently.
Prime Factorization Method
Simplifying the square root of a number involves breaking it down into its prime factors. Here's a step-by-step guide to simplify the square root of 20 using the prime factorization method:
-
List the factors of 20:
- The factors of 20 are 1, 2, 4, 5, 10, and 20.
-
Identify the prime factors:
- 20 can be expressed as a product of prime numbers: 20 = 2 × 2 × 5.
-
Group the prime factors:
- Group the pairs of the same prime number: \(20 = 2^2 \times 5\).
-
Rewrite the expression using square roots:
- \(\sqrt{20} = \sqrt{2^2 \times 5} = \sqrt{2^2} \times \sqrt{5}\)
-
Simplify the square root:
- The square root of \(2^2\) is 2. Therefore, \(\sqrt{2^2} = 2\).
- So, \(\sqrt{20} = 2 \times \sqrt{5}\).
-
Final simplified form:
- Thus, \(\sqrt{20} = 2\sqrt{5}\).
Using the prime factorization method, we have simplified the square root of 20 to \(2\sqrt{5}\). This method can be applied to other numbers as well, making it a versatile technique for simplifying square roots.
Steps to Simplify √20 Using Prime Factorization
The prime factorization method is a straightforward way to simplify square roots by breaking down the radicand into its prime factors. Let's go through the steps to simplify √20 using this method:
-
Identify the prime factors of 20: Start by finding the prime numbers that multiply together to give 20. The prime factorization of 20 is:
\[ 20 = 2 \times 2 \times 5 = 2^2 \times 5 \]
-
Rewrite the square root using the prime factors: Replace 20 under the square root sign with its prime factors:
\[ \sqrt{20} = \sqrt{2^2 \times 5} \]
-
Separate the perfect square: Extract the square root of the perfect square (2²) and leave the remaining factor under the square root sign:
\[ \sqrt{2^2 \times 5} = \sqrt{2^2} \times \sqrt{5} \]
-
Simplify the square root: Calculate the square root of the perfect square:
\[ \sqrt{2^2} = 2 \]
-
Combine the results: Multiply the simplified square root of the perfect square by the remaining square root:
\[ \sqrt{20} = 2 \times \sqrt{5} \]
Therefore, the simplified form of \(\sqrt{20}\) is \(2\sqrt{5}\). This method can be applied to simplify other square roots as well, making the radicand easier to work with in calculations and problem-solving.

Step-by-Step Guide
To simplify the square root of 20 using the prime factorization method, follow these detailed steps:
-
Identify the Prime Factors:
Start by finding the prime factors of 20. The prime factorization of 20 is:
\(20 = 2 \times 2 \times 5 = 2^2 \times 5\)
-
Rewrite the Square Root:
Express the square root of 20 using its prime factors:
\(\sqrt{20} = \sqrt{2^2 \times 5}\)
-
Separate the Perfect Square:
Split the expression into the product of two square roots, one of which is a perfect square:
\(\sqrt{2^2 \times 5} = \sqrt{2^2} \times \sqrt{5}\)
-
Simplify the Perfect Square:
Take the square root of the perfect square (22):
\(\sqrt{2^2} = 2\)
So the expression simplifies to:
\(2 \times \sqrt{5}\)
-
Combine the Results:
Combine the simplified square root with the remaining factor:
\(\sqrt{20} = 2\sqrt{5}\)
Therefore, the simplified form of \(\sqrt{20}\) is \(2\sqrt{5}\).
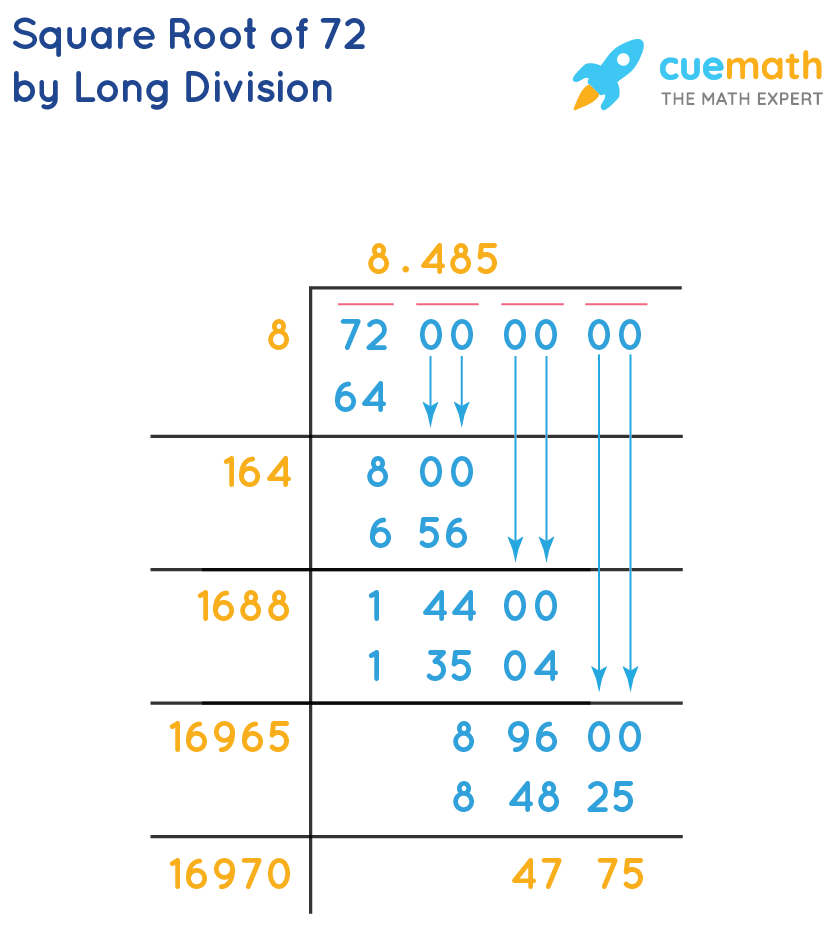
Long Division Method for Simplifying Square Roots
The long division method is a systematic way to find the square root of a number. Below are the steps to simplify the square root of 20 using this method:
- Preparation:
Write 20 as 20.0000, grouping digits in pairs from the decimal point. For 20, it looks like "20.00".
- Find the Largest Square:
Identify the largest square smaller than or equal to 20, which is 16 (4²). Place 4 above the line as the first digit of the root.
- Subtract and Bring Down:
Subtract 16 from 20 to get 4, then bring down the next pair of zeros to make it 400.
- Double the Current Result:
Double the current result (4) to get 8. Now, find a digit (X) such that 80X multiplied by X is less than or equal to 400. Here, X is 4 because 84 × 4 = 336.
- Repeat with Precision:
Subtract 336 from 400 to get 64, bring down the next pair of zeros to get 6400, then double the quotient (44) to get 88. Choose a digit (Y) so that 880Y multiplied by Y is just under 6400. Continue this process until reaching the desired level of accuracy.
Using the long division method, the square root of 20 is approximately 4.472.
Examples of Simplifying Similar Square Roots
Understanding how to simplify the square root of 20 can help in simplifying other square roots as well. Here are some examples to illustrate this process:
Example 1: Simplifying √50
Step-by-step process:
- Find the prime factors of 50: \(50 = 2 \times 5^2\).
- Group the prime factors into pairs: \(50 = (5^2) \times 2\).
- Take the square root of each group: \(\sqrt{50} = \sqrt{(5^2) \times 2} = 5\sqrt{2}\).
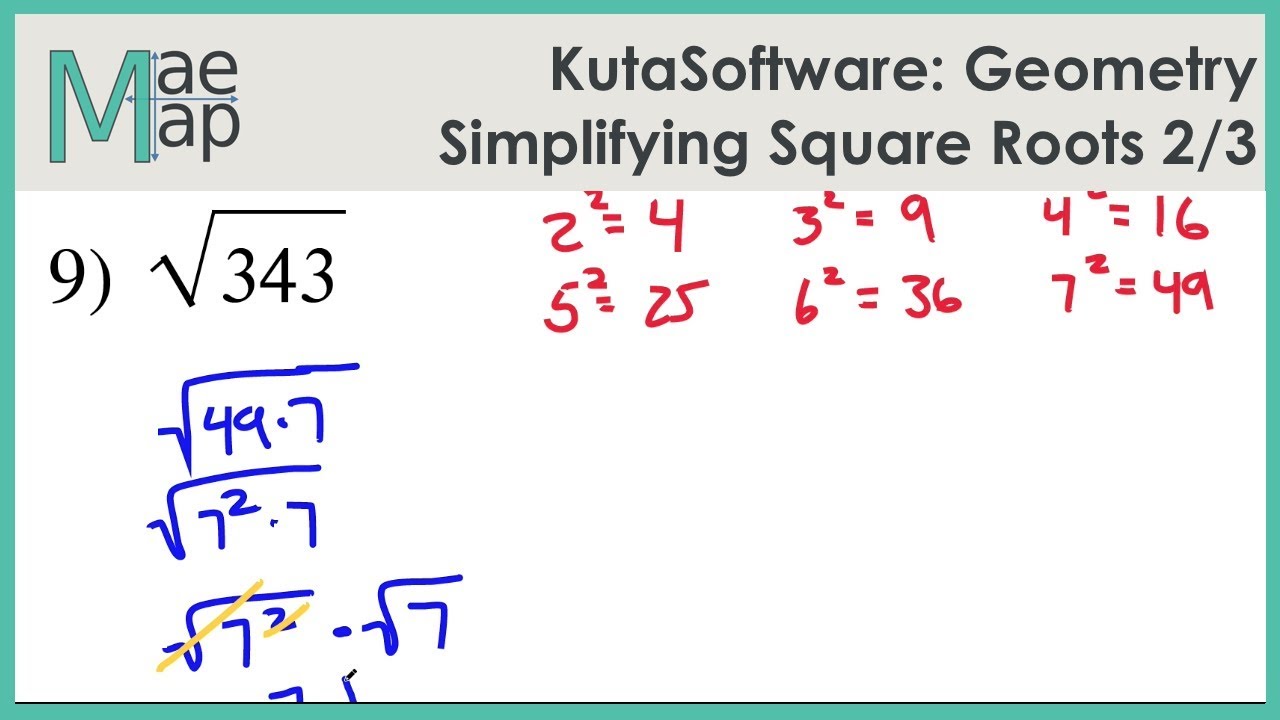
Example 2: Simplifying √72
Step-by-step process:
- Find the prime factors of 72: \(72 = 2^3 \times 3^2\).
- Group the prime factors into pairs: \(72 = (2^2) \times (3^2) \times 2\).
- Take the square root of each group: \(\sqrt{72} = \sqrt{(2^2) \times (3^2) \times 2} = 2 \times 3 \sqrt{2} = 6\sqrt{2}\).
Example 3: Simplifying √45
Step-by-step process:
- Find the prime factors of 45: \(45 = 3^2 \times 5\).
- Group the prime factors into pairs: \(45 = (3^2) \times 5\).
- Take the square root of each group: \(\sqrt{45} = \sqrt{(3^2) \times 5} = 3\sqrt{5}\).
Example 4: Simplifying √18
Step-by-step process:
- Find the prime factors of 18: \(18 = 2 \times 3^2\).
- Group the prime factors into pairs: \(18 = (3^2) \times 2\).
- Take the square root of each group: \(\sqrt{18} = \sqrt{(3^2) \times 2} = 3\sqrt{2}\).
Example 5: Simplifying √32
Step-by-step process:
- Find the prime factors of 32: \(32 = 2^5\).
- Group the prime factors into pairs: \(32 = (2^4) \times 2 = (2^2)^2 \times 2\).
- Take the square root of each group: \(\sqrt{32} = \sqrt{(2^4) \times 2} = 4\sqrt{2}\).
By following these steps, you can simplify various square roots using the prime factorization method.
Special Cases and Common Mistakes
Simplifying square roots can sometimes involve special cases or common mistakes that students often encounter. Understanding these can help avoid errors and ensure accurate simplification. Here are some key points to consider:
Special Cases
- Perfect Squares: When the number under the radical (radicand) is a perfect square, it simplifies to an integer. For example, √25 simplifies to 5 because 25 is a perfect square (5 x 5).
- Prime Numbers: If the radicand is a prime number, it cannot be simplified further using standard methods. For instance, √7 remains √7 because 7 is prime and has no perfect square factors.
- Negative Numbers: Square roots of negative numbers are not real numbers. Instead, they are expressed in terms of imaginary numbers. For example, √-16 is 4i, where i is the imaginary unit.
Common Mistakes
- Incorrect Factorization: Failing to factor the radicand correctly can lead to incorrect simplification. Ensure all factors are considered, especially the largest perfect square factor.
- Forgetting to Simplify Completely: Sometimes, students stop after partial simplification. Always check if further simplification is possible. For instance, √50 can be simplified to 5√2, but if you stop at 10√5, the simplification is incomplete.
- Misapplying Properties: Incorrectly applying the product or quotient properties of square roots can lead to errors. For example, √(a*b) should be simplified as √a * √b, not as (√a * b) or a * (√b).
- Confusing Radicals with Exponents: Remember that radicals and exponents follow different rules. For instance, (√a)^2 simplifies to a, but √(a^2) is a only when a is non-negative.
By keeping these special cases and common mistakes in mind, you can simplify square roots accurately and efficiently. Practice with various examples to strengthen your understanding and avoid errors.

Practical Applications of Simplified Square Roots
Understanding and simplifying square roots is not just a mathematical exercise; it has numerous practical applications in various fields. Here are some real-world examples where simplified square roots play a crucial role:
-
Finance
In finance, the volatility of stock prices, which indicates how much a stock's price can fluctuate, is calculated using square roots. This helps investors assess the risk associated with particular investments.
-
Architecture and Engineering
Square roots are used to determine the natural frequency of structures like bridges and buildings. This information is critical in predicting how these structures will respond to various forces, such as wind or traffic loads.
-
Science
Scientists use square roots in many calculations, such as determining the velocity of an object, the intensity of sound waves, or the amount of radiation absorbed by a material. These calculations help in understanding natural phenomena and developing new technologies.
-
Statistics
In statistics, square roots are used to calculate the standard deviation, which measures the amount of variation or dispersion in a set of values. This helps in analyzing data and making informed decisions based on statistical findings.
-
Geometry
Square roots are fundamental in geometry for calculating the area and perimeter of shapes and solving problems involving right triangles, such as using the Pythagorean theorem.
-
Computer Science
Square roots are used in various computer algorithms, including encryption, image processing, and game physics. For example, encryption algorithms use square roots to generate keys for secure data transmission.
-
Navigation
Pilots and navigators use square roots to calculate distances between points on maps and to estimate directions. This is essential for accurate navigation and route planning.
-
Electrical Engineering
In electrical engineering, square roots are used to calculate power, voltage, and current in circuits. These calculations are crucial for designing and analyzing electrical systems and devices.
These examples demonstrate the importance of mastering the simplification of square roots, as this skill is widely applicable across many fields and everyday situations.
Conclusion and Additional Resources
Simplifying the square root of a number like 20 is an essential skill in mathematics that aids in various calculations and problem-solving scenarios. Through the prime factorization method and the long division method, we have demonstrated how to simplify √20 to its simplest radical form, 2√5. This simplification process is not only useful for academic purposes but also has practical applications in fields such as engineering, physics, and architecture.
To further your understanding and practice, here are some additional resources and tools:
- - Step-by-step solutions and explanations.
- - Detailed guide on the prime factorization method.
- - Interactive tool to solve square roots and other algebra problems.
- - Comprehensive explanations and examples using both prime factorization and long division methods.
- - Simplification methods and practical applications in real-world scenarios.
By utilizing these resources, you can deepen your understanding of simplifying square roots and explore more complex mathematical concepts. Practice regularly to enhance your problem-solving skills and confidence in handling square roots.
Video hướng dẫn cách đơn giản hóa căn bậc hai của 20 (√20) một cách chi tiết và dễ hiểu. Phương pháp phân tích số nguyên tố và phép chia dài được sử dụng.
Làm Thế Nào Để Đơn Giản Hóa Căn Bậc Hai của 20: Sqrt(20)
READ MORE:
Video hướng dẫn cách đơn giản hóa căn bậc hai (√9, √20) để chuẩn bị cho số ảo trong môn Đại Số 2. Bao gồm các phương pháp phân tích số nguyên tố và phép chia dài.
Đại Số 2 - Đơn Giản Hóa Căn Bậc Hai để Chuẩn Bị Cho Số Ảo, căn(9), căn(20)