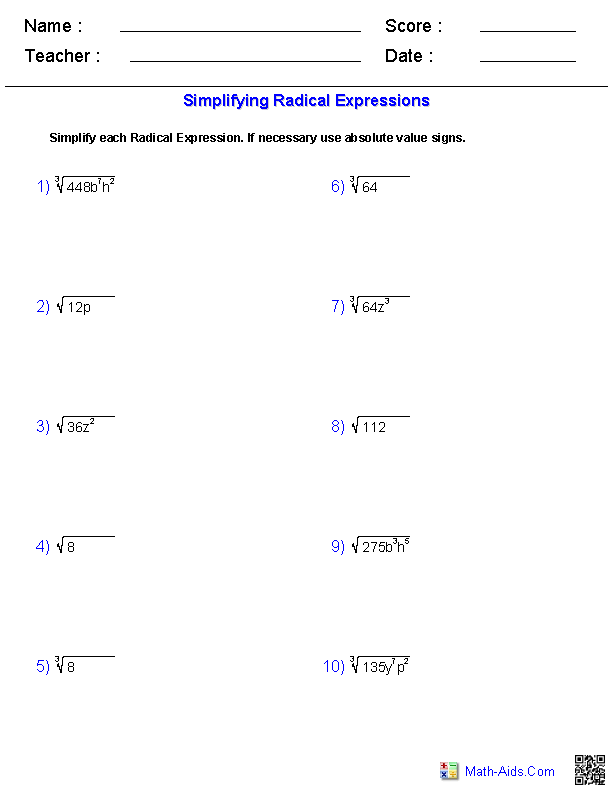Topic simplifying rational expressions examples: Simplifying rational expressions is a fundamental skill in algebra that helps in solving complex problems efficiently. In this article, we will explore various techniques with practical examples to simplify rational expressions, making the process easy and understandable for learners of all levels.
Table of Content
- Simplifying Rational Expressions
- Introduction to Rational Expressions
- Identifying and Simplifying Rational Expressions
- Common Techniques and Strategies
- Simplifying Rational Expressions with Opposite Binomial Factors
- Rational Functions and Their Simplification
- Multiplying and Dividing Rational Expressions
- Finding the Domain of Rational Expressions
- Practice Problems and Solutions
- Additional Resources and Tools
- YOUTUBE: Video hướng dẫn cách đơn giản hoá biểu thức hữu tỉ một cách dễ hiểu và chi tiết, giúp bạn nắm vững kiến thức.
Simplifying Rational Expressions
Simplifying rational expressions involves reducing the expression to its simplest form by canceling out common factors in the numerator and the denominator. This process is similar to simplifying numerical fractions.
Steps to Simplify Rational Expressions
- Factor both the numerator and the denominator completely.
- Identify and cancel out any common factors.
- Rewrite the simplified expression.
Examples of Simplifying Rational Expressions
Here are some examples demonstrating the simplification process:
Example 1: Basic Simplification
Simplify \(\frac{2x}{x^2}\):
- Factor the numerator and the denominator: \(\frac{2x}{x \cdot x}\)
- Cancel out the common factor \(x\): \(\frac{2}{x}\)
- The simplified form is \(\frac{2}{x}\).
Example 2: Factoring Polynomials
Simplify \(\frac{(x + 3)(x + 4)}{(x + 3)(x + 2)}\):
- Identify the common factor \((x + 3)\) in both the numerator and the denominator.
- Cancel out the common factor: \(\frac{x + 4}{x + 2}\)
- The simplified form is \(\frac{x + 4}{x + 2}\).
Example 3: More Complex Expressions
Simplify \(\frac{4x^3 + 8x^2}{2x}\):
- Factor the numerator: \(4x^3 + 8x^2 = 4x^2(x + 2)\)
- Factor the denominator: \(2x = 2x\)
- Cancel out the common factor \(2x\): \(\frac{4x^2(x + 2)}{2x} = 2x(x + 2)\)
- The simplified form is \(2x(x + 2)\).
Example 4: Rational Expressions with Multiple Variables
Simplify \(\frac{3x^2y^2}{4x^3y} \times \frac{4x^3y^4}{5x^2}\):
- Multiply the expressions: \(\frac{3x^2y^2 \cdot 4x^3y^4}{4x^3y \cdot 5x^2}\)
- Simplify the result: \(\frac{12x^5y^6}{20x^5y} = \frac{3y^5}{5}\)
- The simplified form is \(\frac{3y^5}{5}\).
Additional Tips
- Always factor completely before canceling any terms.
- Be careful not to cancel terms that are not common factors.
- Check your work by multiplying the factors back together to ensure they match the original expression.
By following these steps and practicing with various examples, you can master the simplification of rational expressions.

READ MORE:
Introduction to Rational Expressions
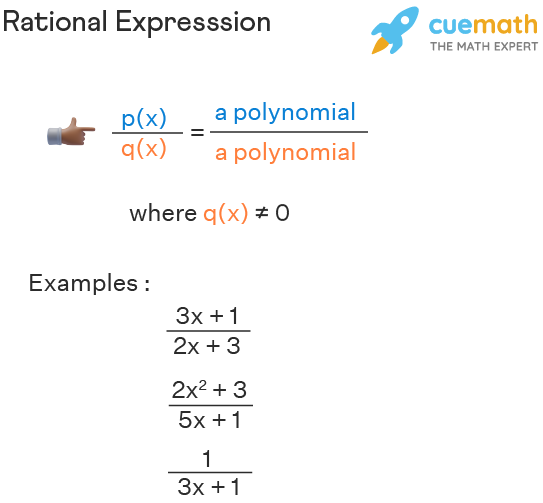
Rational expressions are a type of algebraic expression that represents the quotient of two polynomials. They are similar to fractions but involve variables in the numerator, the denominator, or both. Understanding and simplifying rational expressions is fundamental in algebra as it prepares students for more advanced topics in mathematics.
A rational expression is defined as the quotient of two polynomials \( \frac{P(x)}{Q(x)} \), where \( Q(x) \neq 0 \). For example, the expression \( \frac{x + 3}{x - 5} \) is a rational expression where both the numerator and the denominator are linear polynomials. However, the expression is undefined when \( x = 5 \) because the denominator becomes zero.
When working with rational expressions, it is essential to identify the domain, which includes all the possible values of the variable that do not make the denominator zero. For instance, for \( \frac{x + 3}{x - 5} \), the domain is all real numbers except \( x = 5 \).
Here are some steps to simplify rational expressions:
- Factor both the numerator and the denominator completely.
- Identify and cancel common factors in the numerator and the denominator.
- Rewrite the simplified expression.
For example, to simplify \( \frac{x^2 - 9}{x^2 - 3x} \):
- Factor the numerator and denominator: \( \frac{(x - 3)(x + 3)}{x(x - 3)} \).
- Cancel the common factor \( (x - 3) \): \( \frac{x + 3}{x} \).
- The simplified expression is \( \frac{x + 3}{x} \).
Rational expressions can also be combined using addition, subtraction, multiplication, and division, following similar rules as with numerical fractions but taking care to factor and simplify where possible. Mastery of these skills will greatly enhance your ability to handle more complex algebraic concepts.
Identifying and Simplifying Rational Expressions
Rational expressions are fractions that contain polynomials in the numerator and the denominator. Simplifying these expressions involves several steps to make them more manageable and to reveal their essential characteristics. The following guide will take you through the process of identifying and simplifying rational expressions step-by-step.
- Identify the Rational Expression:
Rational expressions are written as the ratio of two polynomials. For example, \( \frac{2x}{x^2 + 5x + 6} \) is a rational expression.
- Factorize the Polynomials:
Break down both the numerator and the denominator into their simplest factors. For instance, \( x^2 + 5x + 6 \) can be factored into \( (x + 2)(x + 3) \).
- Example: Simplify \( \frac{4x^3 + 8x^2}{2x} \)
- Factorize: \( \frac{4x^2(x + 2)}{2x} \)
- Cancel common factors: \( \frac{4x^2(x + 2)}{2x} = 2x(x + 2) \)
- Result: \( 2x(x + 2) \)
- Example: Simplify \( \frac{4x^3 + 8x^2}{2x} \)
- Cancel Common Factors:
Identify and cancel any common factors that appear in both the numerator and the denominator.
- Example: Simplify \( \frac{x^2 - 9}{x^2 + 6x + 9} \)
- Factorize: \( \frac{(x - 3)(x + 3)}{(x + 3)(x + 3)} \)
- Cancel common factors: \( \frac{(x - 3)(x + 3)}{(x + 3)(x + 3)} = \frac{x - 3}{x + 3} \)
- Result: \( \frac{x - 3}{x + 3} \)
- Example: Simplify \( \frac{x^2 - 9}{x^2 + 6x + 9} \)
- State the Restrictions:
Determine the values that make the denominator zero, as these are the restrictions for the rational expression. For example, in \( \frac{x^2 - 9}{x^2 + 6x + 9} \), the restrictions are \( x \neq -3 \).
By following these steps, you can effectively simplify rational expressions and work with them more easily in various mathematical contexts.
Common Techniques and Strategies
Simplifying rational expressions involves several common techniques and strategies. Understanding these methods is essential for working efficiently with rational expressions. Below, we outline the most effective approaches to simplify rational expressions step by step.
- Factorization: Factor both the numerator and the denominator completely. Look for common factors such as greatest common factors (GCF), differences of squares, trinomials, and other polynomial identities.
- Example: Simplify \( \frac{2x}{x^2} \). Factor both parts to get \( \frac{2x}{x \cdot x} \), then cancel the common \(x\) to get \( \frac{2}{x} \).
- Example: Simplify \( \frac{(x+3)(x+4)}{(x+3)(x+2)} \). Cancel the common factor \( (x+3) \) to get \( \frac{x+4}{x+2} \).
- Canceling Common Factors: After factoring, cancel any common factors that appear in both the numerator and the denominator. Only factors can be canceled, not terms.
- Example: Simplify \( \frac{(x-1)(x+2)}{(x-1)(x-3)} \). Cancel the common factor \( (x-1) \) to get \( \frac{x+2}{x-3} \).
- Multiplication and Division: When multiplying or dividing rational expressions, factor all numerators and denominators first. For division, multiply by the reciprocal of the divisor.
- Example: Multiply \( \frac{3x}{2y} \times \frac{4xy}{5x} \). This becomes \( \frac{3x \cdot 4xy}{2y \cdot 5x} = \frac{12x^2y}{10xy} = \frac{6x}{5} \).
- Example: Divide \( \frac{2x}{3y} \div \frac{5y}{x} \). This becomes \( \frac{2x}{3y} \times \frac{x}{5y} = \frac{2x^2}{15y^2} \).
- Addition and Subtraction: To add or subtract rational expressions, find a common denominator, rewrite each expression with this denominator, and then combine the numerators.
- Example: Add \( \frac{2}{x} + \frac{x}{x-2} \). Find the least common denominator (LCD), \( x(x-2) \), and rewrite each fraction: \( \frac{2(x-2)}{x(x-2)} + \frac{x^2}{x(x-2)} = \frac{2x-4 + x^2}{x(x-2)} = \frac{x^2+2x-4}{x(x-2)} \).
By mastering these techniques, you can simplify a wide range of rational expressions with confidence. Practice regularly to become proficient in recognizing and applying these strategies.
Simplifying Rational Expressions with Opposite Binomial Factors
Rational expressions often contain binomials that are opposite in sign, such as \( (x - a) \) and \( (a - x) \). These can be simplified by recognizing that they are equivalent up to a factor of \(-1\). Here is a step-by-step guide to simplifying such expressions:
Example 1
Simplify the expression \( \frac{(x - 3)(x + 2)}{(3 - x)(x + 4)} \).
- Identify the opposite binomials in the numerator and the denominator. In this case, \( (x - 3) \) and \( (3 - x) \).
- Rewrite the opposite binomial as a factor of \(-1\):
- Substitute this into the original expression:
- Cancel the common factors:
- Simplify the expression by distributing the negative sign:
\[
(3 - x) = -(x - 3)
\]
\[
\frac{(x - 3)(x + 2)}{-(x - 3)(x + 4)}
\]
\[
\frac{\cancel{(x - 3)}(x + 2)}{-\cancel{(x - 3)}(x + 4)} = \frac{x + 2}{-(x + 4)}
\]
\[
\frac{x + 2}{-(x + 4)} = -\frac{x + 2}{x + 4}
\]
Example 2
Simplify the expression \( \frac{x^2 - 4}{4 - x^2} \).
- Factor both the numerator and the denominator:
- Cancel the common factors:
\[
\frac{(x - 2)(x + 2)}{-(x - 2)(x + 2)}
\]
\[
\frac{\cancel{(x - 2)}\cancel{(x + 2)}}{-\cancel{(x - 2)}\cancel{(x + 2)}} = -1
\]
Practice Problems
- Simplify: \( \frac{2x - 8}{8 - 2x} \)
- Simplify: \( \frac{x^2 - 9}{9 - x^2} \)
- Simplify: \( \frac{4 - x}{x - 4} \)
Solution to Practice Problems
- \[ \frac{2(x - 4)}{-(2(x - 4))} = -1 \]
- \[ \frac{(x - 3)(x + 3)}{-(x - 3)(x + 3)} = -1 \]
- \[ \frac{-(x - 4)}{x - 4} = -1 \]
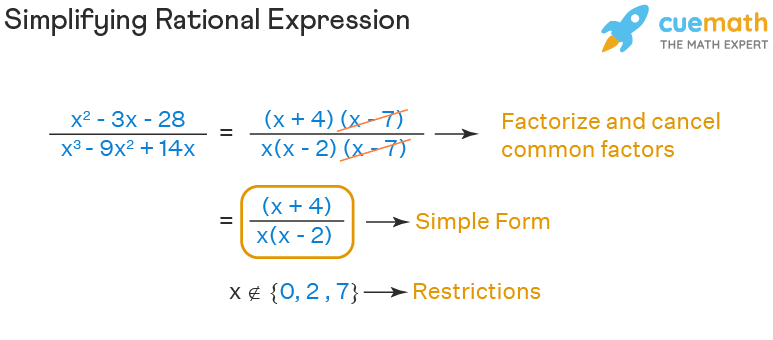
Rational Functions and Their Simplification
A rational function is a fraction where both the numerator and the denominator are polynomials. Simplifying rational functions involves factoring these polynomials and canceling common factors. This process helps to write the rational function in its lowest terms.
Here are the steps to simplify rational functions:
- Factor the Numerator and Denominator: Find the factors of both the numerator and the denominator.
- Identify Common Factors: Look for any common factors in the numerator and the denominator.
- Cancel Common Factors: Cancel out the common factors to simplify the expression.
- Simplify Further: If possible, further simplify the remaining expression.
Let's look at some examples:
| Example | Simplified Form |
|---|---|
|
\[
Factor both the numerator and the denominator: \[
|
Cancel the common factor \((x - 3)\): \[
|
|
\[
Factor both the numerator and the denominator: \[
|
Cancel the common factor \((x + 3)\): \[
|
Next, consider the domain of the simplified function. The domain includes all real numbers except those that make the denominator zero. For the example above, the simplified function is:
\[
\frac{x + 2}{x + 4}
\]
The original denominator \(x^2 + 7x + 12\) factors to \((x + 3)(x + 4)\), so the values \(x = -3\) and \(x = -4\) must be excluded from the domain.
By following these steps, you can simplify rational functions and determine their domains effectively.
Multiplying and Dividing Rational Expressions
Multiplying and dividing rational expressions involves simplifying each expression and then applying the rules for multiplication and division of fractions. Below are the detailed steps and examples to help you understand these processes.
Steps for Multiplying Rational Expressions
- Factor all numerators and denominators completely.
- Identify and cancel any common factors between the numerators and denominators.
- Multiply the remaining factors in the numerators together and do the same for the denominators.
- Simplify the resulting expression if possible.
Example of Multiplying Rational Expressions
Simplify \( \frac{3x}{2y} \times \frac{4xy}{5x} \).
- Factor numerators and denominators: \( \frac{3x}{2y} \times \frac{4xy}{5x} \).
- Cancel common factors: \( \frac{3 \cancel{x}}{2y} \times \frac{4 \cancel{xy}}{5 \cancel{x}} = \frac{3}{2y} \times \frac{4y}{5} \).
- Multiply remaining factors: \( \frac{3 \times 4y}{2y \times 5} = \frac{12y}{10y} \).
- Simplify: \( \frac{6}{5} \).
Steps for Dividing Rational Expressions
- Rewrite the division as multiplication by the reciprocal of the divisor.
- Factor all numerators and denominators completely.
- Identify and cancel any common factors between the numerators and denominators.
- Multiply the remaining factors in the numerators together and do the same for the denominators.
- Simplify the resulting expression if possible.
Example of Dividing Rational Expressions
Simplify \( \frac{2x}{3y} \div \frac{5y}{x} \).
- Rewrite as multiplication by the reciprocal: \( \frac{2x}{3y} \times \frac{x}{5y} \).
- Factor numerators and denominators: \( \frac{2x}{3y} \times \frac{x}{5y} \).
- Cancel common factors: \( \frac{2 \cancel{x}}{3y} \times \frac{\cancel{x}}{5y} = \frac{2}{3y} \times \frac{1}{5y} \).
- Multiply remaining factors: \( \frac{2 \times 1}{3y \times 5y} = \frac{2}{15y^2} \).
Practice Problems
- Simplify \( \frac{5x^2}{6y} \times \frac{2y^3}{x} \).
- Simplify \( \frac{3a^2 - 12}{4b} \div \frac{6a}{2b^2} \).
- Simplify \( \frac{x^2 - 9}{x^2 + 3x} \times \frac{x + 3}{x - 3} \).
- Simplify \( \frac{4x}{y^2 - 1} \div \frac{2x - 2}{y + 1} \).
Finding the Domain of Rational Expressions
To determine the domain of a rational expression, we need to identify all values of the variable that would cause the denominator to be zero. These values are excluded from the domain because division by zero is undefined.
Here are the steps to find the domain of a rational expression:
- Simplify the rational expression, if possible.
- Set the denominator equal to zero and solve for the variable.
- The solutions to the equation in step 2 are the values that are excluded from the domain.
Let's look at a detailed example:
Simplify and find the domain of the following expression:
\[\frac{x^2 - 4}{x^2 - x - 6}\]
- Factor the numerator and the denominator:
- Identify the values that make the denominator zero:
- \(x - 3 = 0 \Rightarrow x = 3\)
- \(x + 2 = 0 \Rightarrow x = -2\)
- The domain of the expression is all real numbers except \(x = 3\) and \(x = -2\).
\[\frac{(x-2)(x+2)}{(x-3)(x+2)}\]
\[(x-3)(x+2) = 0\]
Solve for \(x\):
Thus, the domain of \(\frac{x^2 - 4}{x^2 - x - 6}\) is:
\(\{x \in \mathbb{R} \mid x \neq 3, x \neq -2\}\)
Here's another example:
Simplify and find the domain of \(\frac{2x^2 - x - 1}{x^2 - 5x + 6}\):
- Factor the numerator and denominator:
- Set the denominator equal to zero and solve for \(x\):
- \(x - 2 = 0 \Rightarrow x = 2\)
- \(x - 3 = 0 \Rightarrow x = 3\)
- The domain of the expression is all real numbers except \(x = 2\) and \(x = 3\).
\[\frac{(2x+1)(x-1)}{(x-2)(x-3)}\]
\[(x-2)(x-3) = 0\]
Thus, the domain of \(\frac{2x^2 - x - 1}{x^2 - 5x + 6}\) is:
\(\{x \in \mathbb{R} \mid x \neq 2, x \neq 3\}\)
Remember, always check the simplified form and the original expression to identify all possible excluded values from the domain.
Practice Problems and Solutions
Practicing problems is a crucial step in mastering the simplification of rational expressions. Here, we provide a variety of problems ranging from basic to advanced levels, along with detailed solutions.
Basic Level Problems
-
Simplify the following rational expression:
\(\frac{4x^3 + 8x^2}{2x}\)
Solution:
- Factor the numerator: \(4x^3 + 8x^2 = 4x^2(x + 2)\).
- Simplify: \(\frac{4x^2(x + 2)}{2x} = 2x(x + 2)\).
Final answer: \(2x(x + 2)\)
-
Simplify the following rational expression:
\(\frac{x^2 - 9}{x^2 - 3x}\)
Solution:
- Factor both numerator and denominator: \(\frac{(x - 3)(x + 3)}{x(x - 3)}\).
- Cancel the common factor \((x - 3)\): \(\frac{x + 3}{x}\).
Final answer: \(\frac{x + 3}{x}\)
Intermediate Level Problems
-
Simplify the following rational expression:
\(\frac{x^2 + 2x - 15}{x^2 + x - 12}\)
Solution:
- Factor both numerator and denominator: \(\frac{(x + 5)(x - 3)}{(x + 4)(x - 3)}\).
- Cancel the common factor \((x - 3)\): \(\frac{x + 5}{x + 4}\).
Final answer: \(\frac{x + 5}{x + 4}\)
-
Simplify the following rational expression:
\(\frac{4x^2 + 4x + 1}{2x^3 + 11x^2 + 5x}\)
Solution:
- Factor both numerator and denominator (if possible).
- In this case, check for common factors or use polynomial long division if necessary.
Advanced Level Problems
-
Simplify the following rational expression and find the domain:
\(\frac{x^3 + 1}{x^2 + 7x + 6}\)
Solution:
- Factor both numerator and denominator: \(\frac{(x + 1)(x^2 - x + 1)}{(x + 6)(x + 1)}\).
- Cancel the common factor \((x + 1)\): \(\frac{x^2 - x + 1}{x + 6}\).
- Domain restriction: \(x \neq -6\).
Final answer: \(\frac{x^2 - x + 1}{x + 6}\)
-
Simplify the following rational expression and find the domain:
\(\frac{x^2 - 1}{x^3 - x}\)
Solution:
- Factor both numerator and denominator: \(\frac{(x - 1)(x + 1)}{x(x - 1)(x + 1)}\).
- Cancel the common factors: \(\frac{1}{x}\).
- Domain restriction: \(x \neq 0\).
Final answer: \(\frac{1}{x}\)
Summary Table of Practice Problems
| Problem | Simplified Form | Domain |
|---|---|---|
| \(\frac{4x^3 + 8x^2}{2x}\) | \(2x(x + 2)\) | All real numbers except \(x = 0\) |
| \(\frac{x^2 - 9}{x^2 - 3x}\) | \(\frac{x + 3}{x}\) | All real numbers except \(x = 0\) and \(x = 3\) |
| \(\frac{x^2 + 2x - 15}{x^2 + x - 12}\) | \(\frac{x + 5}{x + 4}\) | All real numbers except \(x = -4\) and \(x = 3\) |
| \(\frac{4x^2 + 4x + 1}{2x^3 + 11x^2 + 5x}\) | To be determined | Check for common factors or polynomial long division |
| \(\frac{x^3 + 1}{x^2 + 7x + 6}\) | \(\frac{x^2 - x + 1}{x + 6}\) | All real numbers except \(x = -6\) |
| \(\frac{x^2 - 1}{x^3 - x}\) | \(\frac{1}{x}\) | All real numbers except \(x = 0\) |
Additional Resources and Tools
Here are some valuable resources and tools to aid in simplifying rational expressions:
- Online Calculators: Use online calculators to simplify rational expressions step-by-step, providing instant solutions and explanations.
- Math Software: Tools like Wolfram Alpha and GeoGebra can help visualize and simplify complex rational expressions.
- Video Tutorials: Platforms like Khan Academy and YouTube offer comprehensive video lessons on rational expressions, covering various techniques and examples.
- Practice Worksheets: Printable worksheets from educational websites allow you to practice and reinforce your skills in simplifying rational expressions.
- Math Forums: Join forums such as Stack Exchange and Reddit's math communities to ask questions and learn from experienced mathematicians.
Remember, practice is key to mastering the simplification of rational expressions. Utilize these resources to enhance your understanding and problem-solving skills.
Video hướng dẫn cách đơn giản hoá biểu thức hữu tỉ một cách dễ hiểu và chi tiết, giúp bạn nắm vững kiến thức.
Đơn Giản Hoá Biểu Thức Hữu Tỉ
READ MORE:
Video giới thiệu về cách đơn giản hoá biểu thức hữu tỉ trong Đại số II, mang đến cái nhìn tổng quan và các ví dụ chi tiết.
Giới Thiệu Về Đơn Giản Hoá Biểu Thức Hữu Tỉ | Đại Số II | Khan Academy