Topic kuta software simplifying radical expressions: Explore how Kuta Software's resources for simplifying radical expressions can enhance your algebra skills. With comprehensive worksheets, step-by-step solutions, and engaging tutorials, you'll master radicals effortlessly. Delve into strategies that simplify complex expressions and bolster your mathematical confidence, paving the way for academic success.
Table of Content
- Simplifying Radical Expressions
- Introduction to Radical Expressions
- Understanding Radicals and Square Roots
- Methods for Simplifying Radicals
- Practice Problems and Worksheets
- Adding and Subtracting Radical Expressions
- Multiplying and Dividing Radical Expressions
- Operations with Radical Expressions
- Rationalizing Denominators
- Complex Numbers and Radicals
- Solving Radical Equations
- Graphing Radical Functions
- Resources and Further Learning
- YOUTUBE: Video về cách đơn giản hóa biểu thức căn của KutaSoftware, thích hợp cho những ai muốn học cách giải các biểu thức căn bằng phần mềm Kuta.
Simplifying Radical Expressions
Simplifying radical expressions involves rewriting them in their simplest form. This includes removing perfect square factors from under the radical, rationalizing denominators, and combining like terms.
Steps to Simplify Radicals
- Find the prime factorization of the number inside the radical.
- Pair the prime factors.
- Move pairs outside the radical.
- Simplify the expression.
Examples
Practice Problems
| Expression | Simplified Form |
|---|---|
Key Points
- Only similar radicals can be combined.
- Rationalizing the denominator eliminates radicals from the denominator.
- Use the distributive property when simplifying expressions with multiple terms.
Practicing these steps will help you master simplifying radical expressions. Make sure to work through multiple examples to become comfortable with the process.

READ MORE:
Introduction to Radical Expressions
Radical expressions are mathematical expressions that include a square root, cube root, or other root symbol. Understanding and simplifying these expressions is crucial in algebra. Simplifying radicals involves expressing them in their simplest form by following specific steps:
- Identify the radicand (the number under the root).
- Factor the radicand into its prime factors.
- Pair factors to move them outside the radical.
- Simplify any remaining radical expression.
For example, to simplify \( \sqrt{50} \):
- Factor 50 into \( 2 \times 5^2 \).
- Pair the 5s to get \( 5\sqrt{2} \).
- The simplified form is \( 5\sqrt{2} \).
Practicing these steps will enhance your ability to work with radicals in more complex algebraic expressions.
Understanding Radicals and Square Roots
Radicals and square roots are fundamental concepts in algebra that involve the use of roots to express numbers. A radical expression is an expression that includes a square root, cube root, or higher roots. The most common type of radical expression is the square root.
The square root of a number \(x\) is written as \(\sqrt{x}\) and is defined as the number that, when multiplied by itself, gives \(x\). For example:
- \(\sqrt{25} = 5\) because \(5 \times 5 = 25\).
- \(\sqrt{36} = 6\) because \(6 \times 6 = 36\).
To simplify square roots, we can use the following steps:
- Factor the number under the square root into its prime factors.
- Group the factors into pairs of the same number.
- For each pair, take one number out of the square root.
Let's simplify \(\sqrt{72}\) step by step:
- Factor 72 into prime factors: \(72 = 2 \times 2 \times 2 \times 3 \times 3\).
- Group the factors into pairs: \(72 = (2 \times 2) \times (3 \times 3) \times 2\).
- Take one number from each pair out of the square root: \(\sqrt{72} = 2 \times 3 \times \sqrt{2} = 6\sqrt{2}\).
When dealing with variables, the process is similar. For example, to simplify \(\sqrt{x^4 y^2}\):
- Factor \(x^4 y^2\) as \((x^2 \times x^2) \times y^2\).
- Take one number from each pair: \(\sqrt{x^4 y^2} = x^2 y\).
Here are some properties of square roots that are useful when simplifying expressions:
- \(\sqrt{a \times b} = \sqrt{a} \times \sqrt{b}\)
- \(\sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}}\)
- \(\sqrt{a^2} = |a|\)
Understanding and applying these properties can make working with radical expressions easier and more intuitive. Practice simplifying different radical expressions to become more comfortable with these techniques.
Methods for Simplifying Radicals
Simplifying radicals involves reducing the radical expression to its simplest form. Here are some methods to achieve this:
1. Prime Factorization
To simplify a radical using prime factorization:
- Find the prime factorization of the number under the radical.
- Group the prime factors into pairs.
- Move each pair of factors out from under the radical.
Example:
\(\sqrt{72}\)
Prime factorization of 72: \(72 = 2 \times 2 \times 2 \times 3 \times 3\)
Group into pairs: \(72 = (2 \times 2) \times (3 \times 3) \times 2\)
Simplify: \(\sqrt{72} = 2 \times 3 \times \sqrt{2} = 6\sqrt{2}\)
2. Simplifying Variables
When the radical contains variables, apply the same method:
- Separate the variables into groups of two.
- Move each group out from under the radical.
Example:
\(\sqrt{x^4 y^3}\)
Group into pairs: \(\sqrt{x^4 y^3} = \sqrt{(x^2 \times x^2) \times (y^2 \times y)}\)
Simplify: \(\sqrt{x^4 y^3} = x^2 y \sqrt{y}\)
3. Using the Quotient Property
The quotient property of radicals states that:
\(\sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}}\)
To simplify a radical expression using this property:
- Split the radical into the numerator and the denominator.
- Simplify each part separately.
Example:
\(\sqrt{\frac{50}{2}}\)
Simplify: \(\sqrt{25} = 5\)
\(\sqrt{\frac{50}{2}} = \sqrt{25} = 5\)
4. Rationalizing the Denominator
To rationalize the denominator:
- Multiply both the numerator and the denominator by the radical in the denominator.
- Simplify the resulting expression.
Example:
\(\frac{3}{\sqrt{5}}\)
Multiply by \(\sqrt{5}\): \(\frac{3}{\sqrt{5}} \times \frac{\sqrt{5}}{\sqrt{5}} = \frac{3\sqrt{5}}{5}\)
5. Combining Like Terms
To combine like terms involving radicals:
- Ensure the radicals have the same radicand.
- Add or subtract the coefficients of the like radicals.
Example:
\(2\sqrt{3} + 5\sqrt{3} = 7\sqrt{3}\)
\(6\sqrt{2} - 3\sqrt{2} = 3\sqrt{2}\)
By following these methods, you can simplify radical expressions efficiently.
Practice Problems and Worksheets
Practice problems and worksheets are essential for mastering the simplification of radical expressions. Below are some practice problems designed to help you become proficient in simplifying radicals.
Problem Set 1: Simplifying Basic Radicals
- Simplify \( \sqrt{50} \)
- Simplify \( \sqrt{72} \)
- Simplify \( \sqrt{98} \)
Solution Steps:
- Find the prime factorization of the number under the radical.
- Group the factors into pairs.
- Move each pair of factors outside the radical.
- Multiply the factors outside the radical.
Problem Set 2: Simplifying Radicals with Variables
- Simplify \( \sqrt{18x^4} \)
- Simplify \( \sqrt{50y^3} \)
- Simplify \( \sqrt{72z^5} \)
Solution Steps:
- Apply the same steps as above for the numerical part.
- For variables, use the rule \( \sqrt{x^2} = x \).
- Group the variable factors and simplify.
Practice Worksheets
Download and practice with the following worksheets to improve your skills:
Consistent practice with these problems and worksheets will help you gain confidence and accuracy in simplifying radical expressions.

Adding and Subtracting Radical Expressions
Adding and subtracting radical expressions involves combining like radicals, which are radicals with the same index and radicand. The process is similar to combining like terms in algebra. Below are the steps and examples to help you understand this process:
Steps for Adding and Subtracting Radical Expressions
- Identify like radicals: Ensure the radicals have the same index and radicand. For example, \(3\sqrt{2}\) and \(5\sqrt{2}\) are like radicals, but \(3\sqrt{2}\) and \(4\sqrt{3}\) are not.
- Combine the coefficients: Add or subtract the coefficients of the like radicals while keeping the radical part unchanged. For example, \(3\sqrt{2} + 5\sqrt{2} = (3 + 5)\sqrt{2} = 8\sqrt{2}\).
- Simplify the expression: Ensure all radicals are in their simplest form before combining. For instance, simplify \(\sqrt{20}\) to \(2\sqrt{5}\) before performing addition or subtraction.
Examples
- Example 1: Simplify \(2\sqrt{6} + 5\sqrt{6}\).
\[
2\sqrt{6} + 5\sqrt{6} = (2 + 5)\sqrt{6} = 7\sqrt{6}
\] - Example 2: Simplify \(7\sqrt[3]{5} + 3\sqrt[3]{5}\).
\[
7\sqrt[3]{5} + 3\sqrt[3]{5} = (7 + 3)\sqrt[3]{5} = 10\sqrt[3]{5}
\] - Example 3: Simplify \(4\sqrt{10} - 5\sqrt{10}\).
\[
4\sqrt{10} - 5\sqrt{10} = (4 - 5)\sqrt{10} = -\sqrt{10}
\] - Example 4: Simplify \(10\sqrt{5} + 6\sqrt{2} - 9\sqrt{5} - 7\sqrt{2}\).
\[
10\sqrt{5} + 6\sqrt{2} - 9\sqrt{5} - 7\sqrt{2} = (10 - 9)\sqrt{5} + (6 - 7)\sqrt{2} = \sqrt{5} - \sqrt{2}
\]
Practice Problems
- Simplify \(8\sqrt{3} - 2\sqrt{3}\).
- Simplify \(5\sqrt{7} + 3\sqrt{7} - \sqrt{7}\).
- Simplify \(4\sqrt{12} - 2\sqrt{27}\).
These problems help reinforce the concept of adding and subtracting like radicals. Remember, the key is to simplify radicals where possible and then combine the coefficients of like radicals.
Multiplying and Dividing Radical Expressions
When multiplying and dividing radical expressions, it's important to follow specific steps to simplify them properly. Here's a detailed guide:
Multiplying Radical Expressions
To multiply radical expressions, use the distributive property and then simplify each radical. The key steps are:
- Multiply the coefficients (numbers outside the radicals).
- Multiply the radicands (numbers inside the radicals).
- Simplify the resulting expression, if possible.
Example:
\(\sqrt{a} \cdot \sqrt{b} = \sqrt{a \cdot b}\)
Example:
\((\sqrt{x} - 5\sqrt{y})^2 = (\sqrt{x} - 5\sqrt{y})(\sqrt{x} - 5\sqrt{y})\)
First, apply the distributive property:
\(\sqrt{x} \cdot \sqrt{x} + \sqrt{x} \cdot (-5\sqrt{y}) + (-5\sqrt{y}) \cdot \sqrt{x} + (-5\sqrt{y}) \cdot (-5\sqrt{y})\)
Then simplify:
\(x - 10\sqrt{xy} + 25y\)
Dividing Radical Expressions
To divide radical expressions, you often need to rationalize the denominator, which means eliminating radicals from the denominator. Follow these steps:
- Multiply the numerator and the denominator by the conjugate of the denominator if it contains a binomial radical expression.
- Simplify the resulting expression.
Example:
\(\frac{\sqrt{a}}{\sqrt{b}} = \sqrt{\frac{a}{b}}\)
Example with rationalizing the denominator:
\(\frac{5}{\sqrt{2}} \cdot \frac{\sqrt{2}}{\sqrt{2}} = \frac{5\sqrt{2}}{2}\)
Practice Problems
- Simplify: \(\sqrt{3} \cdot \sqrt{12}\)
- Multiply: \((2\sqrt{5} + 3\sqrt{2})(\sqrt{5} - \sqrt{2})\)
- Divide: \(\frac{\sqrt{18}}{\sqrt{2}}\)
- Rationalize: \(\frac{7}{\sqrt{3}}\)
Practice these problems to master the multiplication and division of radical expressions!
Operations with Radical Expressions
Radical expressions often appear in various mathematical problems, and it's essential to understand how to perform basic operations with them, such as addition, subtraction, multiplication, and division. Below are step-by-step methods for performing these operations.
Adding and Subtracting Radical Expressions
To add or subtract radical expressions, follow these steps:
- Simplify each radical expression.
- Combine like terms (radicals with the same radicand and index).
For example:
- \(\sqrt{18} + 2\sqrt{2}\)
- Simplify \(\sqrt{18}\) to \(3\sqrt{2}\).
- Combine like terms: \(3\sqrt{2} + 2\sqrt{2} = 5\sqrt{2}\).
Multiplying Radical Expressions
To multiply radical expressions, use the following steps:
- Multiply the coefficients (numbers outside the radical).
- Multiply the radicands (numbers inside the radical).
- Simplify the resulting radical expression, if possible.
For example:
- \(\sqrt{3} \cdot \sqrt{12}\)
- Multiply the radicands: \(\sqrt{3 \cdot 12} = \sqrt{36}\).
- Simplify \(\sqrt{36}\) to \(6\).
Dividing Radical Expressions
To divide radical expressions, follow these steps:
- Divide the coefficients.
- Divide the radicands.
- Simplify the resulting radical expression, if possible.
For example:
- \(\frac{\sqrt{50}}{\sqrt{2}}\)
- Divide the radicands: \(\sqrt{\frac{50}{2}} = \sqrt{25}\).
- Simplify \(\sqrt{25}\) to \(5\).
Rationalizing Denominators
When a radical expression appears in the denominator, rationalize it by multiplying the numerator and denominator by a conjugate or suitable radical expression to eliminate the radical from the denominator.
For example:
- \(\frac{3}{\sqrt{2}}\)
- Multiply the numerator and denominator by \(\sqrt{2}\): \(\frac{3 \cdot \sqrt{2}}{\sqrt{2} \cdot \sqrt{2}} = \frac{3\sqrt{2}}{2}\).
Rationalizing Denominators
Rationalizing the denominator is a process used to eliminate radicals from the denominator of a fraction. This is done to make the expression easier to work with and to present it in its simplest form. Here is a step-by-step guide to rationalizing denominators:
Steps to Rationalize the Denominator
- Identify the radical in the denominator.
- Multiply the numerator and the denominator by a suitable radical that will eliminate the radical in the denominator.
- Simplify the resulting expression.
Examples
Consider the following examples:
- Example 1: Rationalize \(\frac{3}{\sqrt{2}}\)
- Multiply the numerator and the denominator by \(\sqrt{2}\): \(\frac{3 \cdot \sqrt{2}}{\sqrt{2} \cdot \sqrt{2}}\)
- This results in \(\frac{3\sqrt{2}}{2}\)
- Example 2: Rationalize \(\frac{5}{\sqrt[3]{4}}\)
- Multiply the numerator and the denominator by \(\sqrt[3]{16}\) (since \((\sqrt[3]{4})^2 \cdot \sqrt[3]{4} = \sqrt[3]{64} = 4\)): \(\frac{5 \cdot \sqrt[3]{16}}{\sqrt[3]{4} \cdot \sqrt[3]{16}}\)
- This results in \(\frac{5\sqrt[3]{16}}{4}\)
- Example 3: Rationalize \(\frac{\sqrt{5}}{\sqrt{3}}\)
- Multiply the numerator and the denominator by \(\sqrt{3}\): \(\frac{\sqrt{5} \cdot \sqrt{3}}{\sqrt{3} \cdot \sqrt{3}}\)
- This results in \(\frac{\sqrt{15}}{3}\)
Rationalizing with Conjugates
When the denominator is a binomial involving radicals, multiply by the conjugate to rationalize.
For example:
- Example 4: Rationalize \(\frac{1}{\sqrt{2} + 1}\)
- Multiply the numerator and the denominator by the conjugate \(\sqrt{2} - 1\): \(\frac{1 \cdot (\sqrt{2} - 1)}{(\sqrt{2} + 1) \cdot (\sqrt{2} - 1)}\)
- This results in \(\frac{\sqrt{2} - 1}{2 - 1} = \sqrt{2} - 1\)

Complex Numbers and Radicals
Complex numbers consist of a real part and an imaginary part, usually expressed in the form \(a + bi\), where \(i\) is the imaginary unit, defined as \(\sqrt{-1}\). Understanding how to simplify expressions involving radicals and complex numbers is crucial for solving advanced mathematical problems.
Basic Concepts
- Imaginary Unit (\(i\)): Defined as \(\sqrt{-1}\), it is used to simplify the square roots of negative numbers.
- Complex Number (\(a + bi\)): Combines a real number \(a\) and an imaginary number \(bi\).
Simplifying Radicals with Complex Numbers
- Identify the negative radicand in the expression.
- Express the negative radicand using \(i\). For example, \(\sqrt{-4}\) becomes \(2i\).
- Simplify further by performing any operations with the real parts and imaginary parts separately.
For instance, consider simplifying the expression \(\sqrt{-9} + \sqrt{16}\):
- Convert \(\sqrt{-9}\) to \(3i\).
- Calculate \(\sqrt{16}\) to get 4.
- The simplified expression is \(3i + 4\).
Operations with Complex Numbers and Radicals
When performing operations involving complex numbers and radicals:
- Addition/Subtraction: Combine like terms (real with real, imaginary with imaginary).
- Multiplication: Use the distributive property and remember that \(i^2 = -1\).
- Division: Multiply the numerator and denominator by the complex conjugate of the denominator to rationalize it.
Example:
To simplify \((2 + \sqrt{-4}) \cdot (1 - i)\):
- Simplify \(\sqrt{-4}\) to \(2i\), so the expression becomes \((2 + 2i) \cdot (1 - i)\).
- Distribute each term: \(2(1 - i) + 2i(1 - i)\).
- Calculate each part: \(2 - 2i + 2i - 2i^2\).
- Since \(i^2 = -1\), the expression becomes \(2 - 2(-1) = 2 + 2 = 4\).
Thus, the simplified expression is \(4\).
Solving Radical Equations
Radical equations are equations in which the variable is inside a radical, typically a square root. Solving these equations requires isolating the radical and eliminating it through appropriate mathematical operations.
Steps to Solve Radical Equations
- Isolate the Radical: Move the radical expression to one side of the equation if it isn't already.
- Eliminate the Radical: Square both sides of the equation to remove the square root. Be cautious as this can introduce extraneous solutions.
- Simplify and Solve: After squaring, simplify the equation and solve for the variable using standard algebraic methods.
- Check for Extraneous Solutions: Substitute your solutions back into the original equation to ensure they are valid, as squaring both sides can introduce false solutions.
Example:
Solve the equation \(\sqrt{x + 3} = x - 1\).
- Isolate the radical (already isolated).
- Square both sides: \((\sqrt{x + 3})^2 = (x - 1)^2\).
- Simplify: \(x + 3 = x^2 - 2x + 1\).
- Rearrange to form a quadratic equation: \(x^2 - 3x - 2 = 0\).
- Factor or use the quadratic formula to solve: \((x - 2)(x - 1) = 0\), so \(x = 2\) or \(x = 1\).
- Check solutions in the original equation:
- For \(x = 2\): \(\sqrt{2 + 3} = 2 - 1\) which is \( \sqrt{5} = 1\) (not true, so it's extraneous).
- For \(x = 1\): \(\sqrt{1 + 3} = 1 - 1\) which is \( \sqrt{4} = 0\) (also not true, so it's extraneous).
In this case, there are no valid solutions to the equation. Always verify solutions to avoid extraneous answers.
Graphing Radical Functions
Radical functions, particularly those involving square roots, have distinct graph shapes that reflect their mathematical properties. Understanding how to graph these functions is essential for visualizing solutions and analyzing their behavior.
Steps to Graph Radical Functions
- Identify the Function: Consider the general form of the radical function, such as \(y = \sqrt{x}\) or \(y = \sqrt{x - h} + k\), where \(h\) and \(k\) represent horizontal and vertical shifts.
- Determine the Domain: The domain consists of all \(x\) values for which the expression inside the radical is non-negative. For \(y = \sqrt{x - h}\), the domain is \(x \geq h\).
- Identify Key Points: Calculate and plot key points, including the vertex or starting point \((h, k)\) and additional points by choosing \(x\) values within the domain.
- Plot the Graph: Draw a smooth curve through the plotted points, ensuring the curve extends infinitely in the direction of increasing \(x\).
- Analyze the Range: The range of \(y = \sqrt{x - h} + k\) is \(y \geq k\), as the square root function cannot produce negative results.
Example:
Graph the function \(y = \sqrt{x - 2} + 1\).
- Identify the Domain: Since the expression inside the square root must be non-negative, the domain is \(x \geq 2\).
- Key Points:
- Starting point (vertex): \((2, 1)\).
- Additional point: For \(x = 3\), \(y = \sqrt{3 - 2} + 1 = 1 + 1 = 2\).
- Another point: For \(x = 6\), \(y = \sqrt{6 - 2} + 1 = 2 + 1 = 3\).
- Plot and Draw: Plot the points \((2, 1)\), \((3, 2)\), and \((6, 3)\), and draw a smooth curve through them.
- Range: The range is \(y \geq 1\).
The graph of \(y = \sqrt{x - 2} + 1\) starts at point \((2, 1)\) and rises gradually as \(x\) increases, reflecting the characteristics of a square root function.
Resources and Further Learning
Here are some valuable resources and further learning opportunities to help you master simplifying radical expressions:
-
Online Tutorials:
- - Access free printable worksheets to practice simplifying radical expressions.
- - Detailed video tutorials and exercises on simplifying radicals and other algebraic concepts.
- - Clear explanations and examples on working with radicals.
-
Books:
- Algebra and Trigonometry by Robert F. Blitzer - Comprehensive textbook covering radicals and other key algebra topics.
- Intermediate Algebra by K. Elayn Martin-Gay - In-depth explanations and practice problems on radicals and other intermediate algebra concepts.
-
Software Tools:
- - Software that generates customizable worksheets and quizzes for practicing radical expressions and other math topics.
- - Online graphing calculator to visualize and explore radical functions.
-
Practice Problems:
- - Extensive collection of worksheets to practice simplifying radicals.
- - Printable worksheets focusing on various aspects of radical expressions.
-
Additional Learning Platforms:
- - Online courses covering algebra and trigonometry, including radical expressions.
- - Comprehensive courses on algebra, including simplifying radicals.
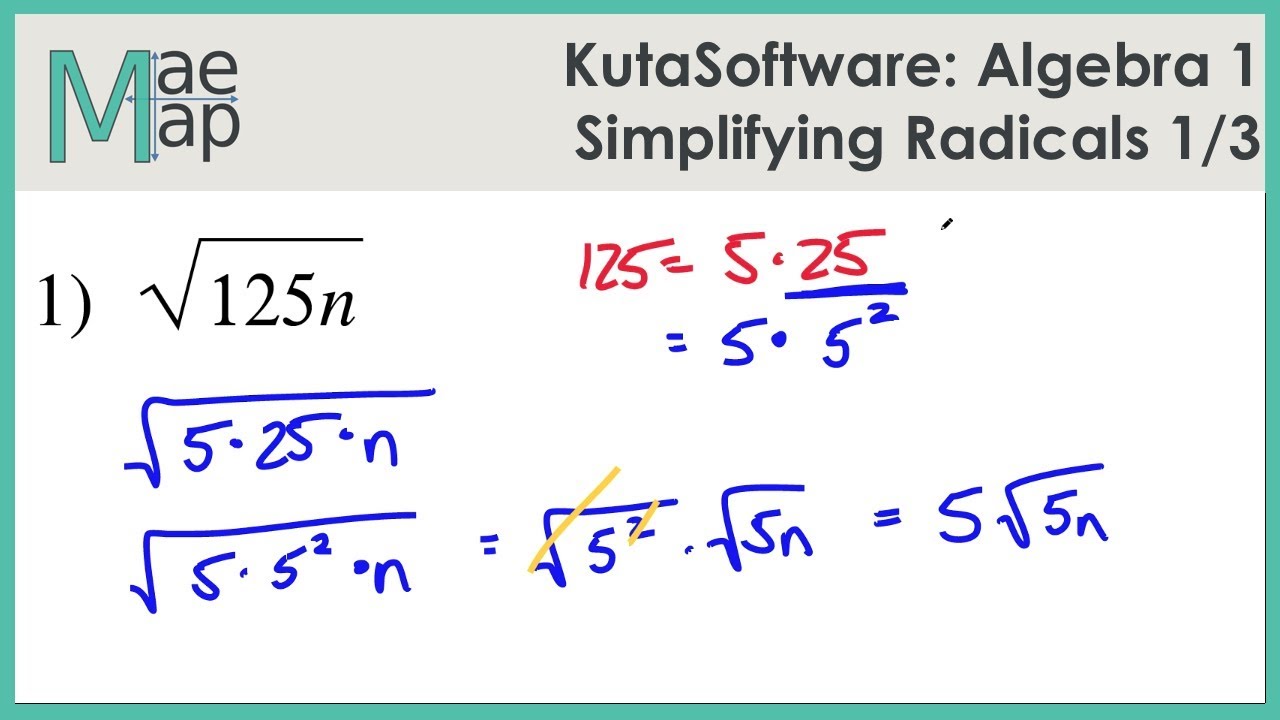
Video về cách đơn giản hóa biểu thức căn của KutaSoftware, thích hợp cho những ai muốn học cách giải các biểu thức căn bằng phần mềm Kuta.
KutaSoftware: Đại số 1 - Đơn giản hóa Biểu thức Căn phần 1
READ MORE:
Video về cách đơn giản hóa biểu thức căn phần 2 của KutaSoftware, giúp bạn tiếp tục học cách giải các biểu thức căn bằng phần mềm Kuta.
KutaSoftware: Đại số 1 - Đơn giản hóa Biểu thức Căn phần 2












