Topic simplifying radical expressions worksheet answers: Welcome to your ultimate guide for simplifying radical expressions worksheet answers! This comprehensive article provides clear and detailed solutions to help you master simplifying radicals. Whether you're a student seeking homework help or a teacher looking for resources, this guide will enhance your understanding and improve your skills in simplifying radical expressions.
Table of Content
- Answers to Simplifying Radical Expressions Worksheet
- Understanding Radical Expressions
- Prime Factorization Method
- Examples of Simplifying Square Roots
- Examples of Simplifying Cube Roots
- Combining Like Radicals
- Rationalizing the Denominator
- Simplifying Radicals with Variables
- Common Mistakes to Avoid
- Advanced Techniques in Radical Simplification
- Applications of Simplified Radical Expressions
- Summary and Review
- Additional Resources and Tools
- YOUTUBE: Tìm hiểu cách đơn giản hóa biểu thức căn bậc hai với video hướng dẫn chi tiết và đáp án bài tập.
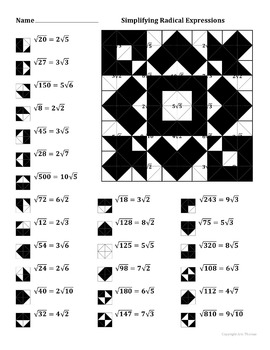
Answers to Simplifying Radical Expressions Worksheet
Introduction
Below are the detailed answers and explanations for the simplifying radical expressions worksheet. These solutions will help you understand the process of simplifying various types of radical expressions.
Basic Steps to Simplify Radical Expressions
- Identify and factor the radicand (the number inside the radical) into its prime factors.
- Group the prime factors into pairs (for square roots) or triples (for cube roots).
- Move the grouped factors outside the radical, leaving any ungrouped factors inside.
- Simplify the expression by multiplying the factors outside the radical and simplifying the radicand inside.
Examples and Solutions
- Example 1: Simplify \( \sqrt{50} \)
Solution: \( \sqrt{50} = \sqrt{2 \times 5 \times 5} = 5\sqrt{2} \)
- Example 2: Simplify \( \sqrt{72} \)
Solution: \( \sqrt{72} = \sqrt{2 \times 2 \times 2 \times 3 \times 3} = 6\sqrt{2} \)
- Example 3: Simplify \( \sqrt{18x^2} \)
Solution: \( \sqrt{18x^2} = \sqrt{2 \times 3 \times 3 \times x^2} = 3x\sqrt{2} \)
- Example 4: Simplify \( \sqrt{75y^3} \)
Solution: \( \sqrt{75y^3} = \sqrt{3 \times 5 \times 5 \times y^2 \times y} = 5y\sqrt{3y} \)
Practice Problems and Solutions
- Simplify \( \sqrt{45} \)
Solution: \( \sqrt{45} = \sqrt{3 \times 3 \times 5} = 3\sqrt{5} \)
- Simplify \( \sqrt{8x^4} \)
Solution: \( \sqrt{8x^4} = \sqrt{2 \times 2 \times 2 \times x^4} = 2x^2\sqrt{2} \)
- Simplify \( \sqrt{32y^6} \)
Solution: \( \sqrt{32y^6} = \sqrt{2 \times 2 \times 2 \times 2 \times 2 \times y^6} = 4y^3\sqrt{2} \)
- Simplify \( \sqrt{125z^5} \)
Solution: \( \sqrt{125z^5} = \sqrt{5 \times 5 \times 5 \times z^4 \times z} = 5z^2\sqrt{5z} \)
Advanced Techniques
- Combining Like Terms: When combining like terms with radicals, ensure that the radicand is the same. For example, \( 2\sqrt{3} + 4\sqrt{3} = 6\sqrt{3} \).
- Rationalizing the Denominator: If the radical is in the denominator, multiply both the numerator and denominator by the radical to eliminate it. For example, \( \frac{1}{\sqrt{2}} = \frac{\sqrt{2}}{2} \).
Conclusion
Understanding and practicing these steps will help you master simplifying radical expressions. Use the examples and practice problems provided to reinforce your learning and gain confidence in solving similar problems.
READ MORE:
Understanding Radical Expressions
Radical expressions are mathematical expressions that include a radical symbol (√) with a radicand (the number under the radical symbol). Understanding how to work with these expressions is essential for simplifying them accurately.
Here are the basic components of a radical expression:
- Radical Symbol (√): Indicates the root of the number.
- Radicand: The number inside the radical symbol. For example, in √16, 16 is the radicand.
- Index: The small number outside and to the upper left of the radical symbol that indicates the degree of the root. If not specified, it is assumed to be 2 (square root).
Simplifying radical expressions involves reducing the radicand to its simplest form. Here are the steps to simplify a radical expression:
- Identify Perfect Squares: Determine if the radicand contains a perfect square. A perfect square is a number that can be expressed as the product of an integer with itself. For example, 16 is a perfect square because \(4 \times 4 = 16\).
- Prime Factorization: If the radicand is not a perfect square, break it down into its prime factors. For example, 72 can be factored into \(2 \times 2 \times 2 \times 3 \times 3\).
- Group Factors: Group the prime factors into pairs. Each pair can be taken out of the radical as a single number. For example, \( \sqrt{72} = \sqrt{2 \times 2 \times 2 \times 3 \times 3} = 2 \times 3 \sqrt{2} = 6\sqrt{2} \).
- Simplify: Multiply the numbers outside the radical and leave the remaining factors inside. The simplified form of \( \sqrt{72} \) is \( 6\sqrt{2} \).
Understanding these steps allows you to simplify any radical expression efficiently. Mastering this skill is crucial for solving more complex algebraic problems involving radicals.
Prime Factorization Method
The Prime Factorization Method is a systematic approach to simplify radical expressions by breaking down the radicand (the number under the radical symbol) into its prime factors. This method is particularly useful when dealing with square roots, cube roots, and higher-order roots.
- Find the Prime Factors:
Start by finding the prime factorization of the radicand. Prime factors are numbers that are only divisible by 1 and themselves. For example, the prime factorization of 72 is:
\\[ 72 = 2 \times 2 \times 2 \times 3 \times 3 \\]
- Group the Prime Factors:
Next, group the prime factors based on the index of the radical. For square roots, group the factors in pairs; for cube roots, group them in triples, and so on. For the square root of 72, we group the factors in pairs:
\\[ 72 = (2 \times 2) \times (3 \times 3) \times 2 \\]
- Simplify the Radicand:
For each complete group of factors, bring one factor outside the radical. In our example, we have two pairs (\\(2 \times 2\\) and \\(3 \times 3\\)), so we take one 2 and one 3 out of the radical:
\\[ \sqrt{72} = \sqrt{(2 \times 2) \times (3 \times 3) \times 2} = 2 \times 3 \times \sqrt{2} \\]
Simplified, this is:
\\[ \sqrt{72} = 6 \sqrt{2} \\]
- Combine the Factors:
Finally, multiply the factors outside the radical to get the simplified form of the radical expression. In this example, the simplified form of \\( \sqrt{72} \\) is \\( 6 \sqrt{2} \\).
Let's consider another example with a cube root:
- Find the Prime Factors:
For the cube root of 216, the prime factorization is:
\\[ 216 = 2 \times 2 \times 2 \times 3 \times 3 \times 3 \\]
- Group the Prime Factors:
Group the factors in triples:
\\[ 216 = (2 \times 2 \times 2) \times (3 \times 3 \times 3) \\]
- Simplify the Radicand:
For each complete group, take one factor outside the radical:
\\[ \sqrt[3]{216} = \sqrt[3]{(2 \times 2 \times 2) \times (3 \times 3 \times 3)} = 2 \times 3 \\]
Simplified, this is:
\\[ \sqrt[3]{216} = 6 \\]
By following these steps, you can simplify any radical expression using the prime factorization method.
Examples of Simplifying Square Roots
Simplifying square roots involves breaking down the number inside the radical sign (the radicand) into its prime factors and then simplifying by extracting pairs of prime numbers. Here are some examples to illustrate the process:
-
Example 1: Simplifying \( \sqrt{50} \)
- Factor the radicand: \( 50 = 2 \times 5^2 \).
- Identify the pairs of prime factors. In this case, \( 5^2 \) forms a pair.
- Extract the pair from under the radical: \( \sqrt{50} = \sqrt{2 \times 5^2} = 5\sqrt{2} \).
So, \( \sqrt{50} \) simplifies to \( 5\sqrt{2} \).
-
Example 2: Simplifying \( \sqrt{72} \)
- Factor the radicand: \( 72 = 2^3 \times 3^2 \).
- Identify the pairs of prime factors. Here, \( 3^2 \) and \( 2^2 \) form pairs, leaving a single 2.
- Extract the pairs from under the radical: \( \sqrt{72} = \sqrt{(2^2 \times 3^2) \times 2} = 6\sqrt{2} \).
Thus, \( \sqrt{72} \) simplifies to \( 6\sqrt{2} \).
-
Example 3: Simplifying \( \sqrt{98} \)
- Factor the radicand: \( 98 = 2 \times 7^2 \).
- Identify the pairs of prime factors. Here, \( 7^2 \) forms a pair.
- Extract the pair from under the radical: \( \sqrt{98} = \sqrt{2 \times 7^2} = 7\sqrt{2} \).
Therefore, \( \sqrt{98} \) simplifies to \( 7\sqrt{2} \).
By following these steps, you can simplify any square root by factoring the radicand, identifying pairs of prime factors, and then extracting those pairs from under the radical sign.
Examples of Simplifying Cube Roots
Understanding how to simplify cube roots is essential for solving various algebraic problems. Here are a few examples to illustrate the process:
Example 1: Simplify \( \sqrt[3]{27} \).
- Identify the perfect cube factor of 27, which is \(3^3\).
- Rewrite the cube root: \( \sqrt[3]{27} = \sqrt[3]{3^3} \).
- Since the cube root of \(3^3\) is 3, we get: \( \sqrt[3]{27} = 3 \).
Example 2: Simplify \( \sqrt[3]{54} \).
- Find the prime factors of 54: \( 54 = 2 \times 3^3 \).
- Rewrite the cube root: \( \sqrt[3]{54} = \sqrt[3]{2 \times 3^3} \).
- Apply the cube root to each factor: \( \sqrt[3]{2} \times \sqrt[3]{3^3} \).
- Simplify the perfect cube: \( \sqrt[3]{2} \times 3 = 3\sqrt[3]{2} \).
Example 3: Simplify \( \sqrt[3]{250} \).
- Break down 250 into its prime factors: \( 250 = 2 \times 5^3 \).
- Rewrite the expression: \( \sqrt[3]{250} = \sqrt[3]{2 \times 5^3} \).
- Separate the cube root: \( \sqrt[3]{2} \times \sqrt[3]{5^3} \).
- Simplify the cube root: \( \sqrt[3]{2} \times 5 = 5\sqrt[3]{2} \).
Example 4: Simplify \( \sqrt[3]{128} \).
- Express 128 as a product of its prime factors: \( 128 = 2^7 \).
- Rewrite the expression: \( \sqrt[3]{128} = \sqrt[3]{2^7} \).
- Identify the largest cube factor: \( 2^6 = (2^2)^3 \).
- Simplify the cube root: \( \sqrt[3]{2^6} \times \sqrt[3]{2} = 2^2 \times \sqrt[3]{2} = 4\sqrt[3]{2} \).
By practicing these steps, you can master the process of simplifying cube roots for any given expression.

Combining Like Radicals
Combining like radicals involves simplifying expressions that contain radicals with the same radicand (the number or expression inside the radical). Similar to combining like terms in algebra, you can only combine radicals that have the same index and radicand.
Here are the steps to combine like radicals:
- Identify Like Radicals: Check if the radicals have the same index and radicand. For example, \( \sqrt{3} \) and \( 2\sqrt{3} \) are like radicals because they both have the same radicand (3) and the same index (2).
- Combine the Coefficients: Add or subtract the coefficients of the like radicals while keeping the radical part unchanged. For example:
- \( \sqrt{3} + 2\sqrt{3} = 3\sqrt{3} \)
- \( 5\sqrt{2} - 3\sqrt{2} = 2\sqrt{2} \)
- Simplify if Necessary: Ensure that the radicals are in their simplest form before combining. For example:
- \( 3\sqrt{12} + 2\sqrt{3} \) should first be simplified to \( 3\sqrt{4 \cdot 3} + 2\sqrt{3} = 3 \cdot 2\sqrt{3} + 2\sqrt{3} = 6\sqrt{3} + 2\sqrt{3} = 8\sqrt{3} \)
Here are some examples of combining like radicals:
- \( 4\sqrt{5} + 7\sqrt{5} = 11\sqrt{5} \)
- \( 3\sqrt{7} - 2\sqrt{7} = \sqrt{7} \)
- \( 2\sqrt{2} + 5\sqrt{3} - \sqrt{2} = (2 - 1)\sqrt{2} + 5\sqrt{3} = \sqrt{2} + 5\sqrt{3} \) (Note: \( \sqrt{2} \) and \( \sqrt{3} \) cannot be combined)
Combining like radicals is a fundamental skill in simplifying radical expressions, and understanding it helps in more complex operations involving radicals.
Rationalizing the Denominator
Rationalizing the denominator involves eliminating any radicals present in the denominator of a fraction. This process helps in simplifying the expression and making it easier to work with. Here are the steps to rationalize the denominator:
- Identify the Radical: Locate the radical in the denominator that needs to be rationalized. For example, in the fraction , the radical is .
- Multiply by the Conjugate: If the denominator is a binomial containing a radical, multiply both the numerator and the denominator by the conjugate of the denominator. The conjugate of is . For example, to rationalize , multiply by .
- Simplify the Expression: After multiplying, simplify the expression. Use the difference of squares formula to simplify the denominator when dealing with conjugates. For example,
- Simplify the Fraction: If possible, simplify the resulting fraction by reducing common factors. For instance,
3 4
Let's look at a detailed example:
- Consider the expression
5 7 - Multiply the numerator and the denominator by
7 5 7 7 - Simplify the fraction:
5 7 7
This completes the rationalization process, making the denominator a rational number.
Simplifying Radicals with Variables
When simplifying radicals involving variables, follow these steps:
- Identify the index and the radicand: Determine if the radical is a square root, cube root, etc., and identify the expression under the radical sign.
- Factor the radicand: Factor the expression under the radical into its prime factors.
- Extract perfect powers: For each factor inside the radical, identify pairs of factors that can be pulled out as perfect powers.
- Combine like radicals: Combine radicals that have the same index and radicand.
- Rationalize the denominator (if needed): Adjust fractions so that no radical appears in the denominator.
Let's illustrate with an example:
| Example: | \(\sqrt{18x^2}\) |
| Step 1: | Identify the index and the radicand: This is a square root and the radicand is \(18x^2\). |
| Step 2: | Factor the radicand: \(18x^2 = 2 \cdot 3^2 \cdot x^2\). |
| Step 3: | Extract perfect powers: \(\sqrt{18x^2} = \sqrt{2 \cdot 3^2 \cdot x^2} = 3x\sqrt{2}\). |
This process ensures that radicals with variables are simplified correctly, incorporating both numerical and variable factors under the radical sign.
Common Mistakes to Avoid
When simplifying radical expressions, watch out for these common mistakes:
- Incorrectly applying rules: Ensure you apply the rules of radical simplification correctly, especially when dealing with different indexes and radicands.
- Forgetting to simplify: Always simplify radicals completely by extracting perfect powers and combining like terms.
- Misidentifying radicands: Identify the correct expression under the radical sign to avoid errors in simplification.
- Overlooking variables: Pay attention to variables within radicands and ensure they are simplified correctly alongside numerical factors.
- Ignoring rationalizing the denominator: Rationalize the denominator when necessary to avoid leaving radicals in the denominator of fractions.
By being aware of these pitfalls, you can improve your accuracy in simplifying radical expressions and avoid common errors.
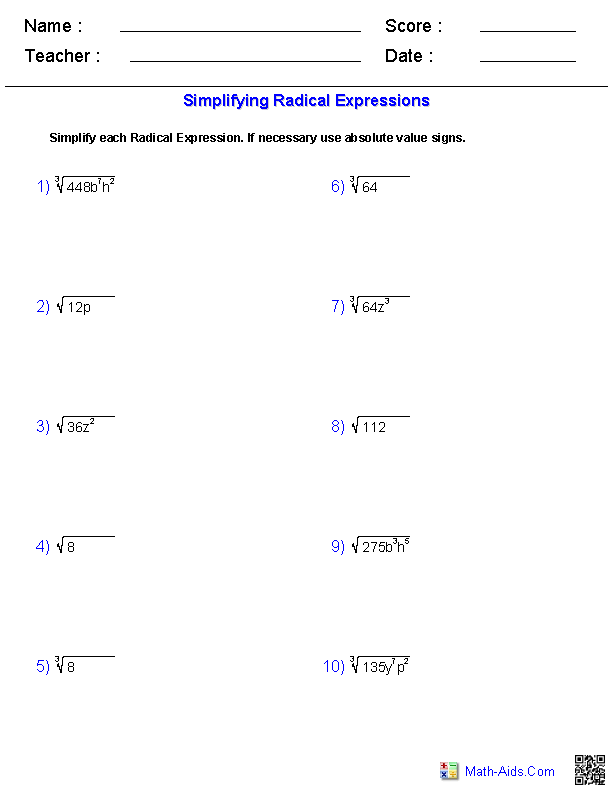
Advanced Techniques in Radical Simplification
Master advanced techniques for simplifying radical expressions with these methods:
- Using conjugates: Rationalize radicals by multiplying the numerator and denominator by the conjugate of the denominator.
- Handling higher indices: Apply rules for higher indices such as cube roots and higher, ensuring to simplify using appropriate factors.
- Simplifying complex expressions: Break down complex radicals into simpler components by factoring and identifying perfect powers.
- Addressing variables: Manage radicals containing variables by separating numerical factors and simplifying variable terms independently.
- Verifying solutions: Check your solutions by squaring the simplified radical expression to ensure correctness.
These techniques will enhance your ability to handle challenging radical simplification problems effectively and accurately.
Applications of Simplified Radical Expressions
Simplified radical expressions find practical applications in various fields:
- Engineering: Simplifying radicals helps in calculating dimensions and measurements involving square roots and cube roots.
- Physics: Radicals are used in formulas for calculating distances, velocities, and energies.
- Finance: Simplifying radicals aids in calculating interest rates, mortgage payments, and financial projections.
- Statistics: Radicals are utilized in statistical analysis, especially in calculating standard deviations and variance.
- Computer Science: Simplified radicals play a role in algorithm analysis and computational efficiency.
Understanding and applying simplified radical expressions are crucial in these fields to ensure accurate computations and analyses.
Summary and Review
In summary, mastering the simplification of radical expressions involves:
- Understanding basic principles: Recognize the rules for simplifying square roots, cube roots, and higher indices.
- Applying factorization: Factorize radicands into their prime factors to simplify expressions.
- Combining like terms: Combine radicals with similar indices and radicands to streamline expressions.
- Handling variables: Manage expressions containing variables by simplifying numerical factors and variable terms separately.
- Utilizing advanced techniques: Use conjugates and other advanced methods to simplify complex radical expressions effectively.
Regular practice and application of these techniques will enhance your ability to simplify radicals accurately and efficiently.
Additional Resources and Tools
Here are some valuable resources and tools to help you master simplifying radical expressions:
-
This worksheet includes 25 scaffolded questions ranging from basic to challenging problems. It also offers visual aids and video tutorials for better understanding.
-
This resource provides a series of worksheets and lessons to practice identifying and simplifying perfect and imperfect squares. It also includes review sheets and quizzes.
-
Math Monks offers a variety of printable worksheets for simplifying radicals, rationalizing denominators, and converting between radical and exponent forms. Suitable for different grade levels.
-
Khan Academy provides free video tutorials and practice problems on simplifying square roots, including step-by-step instructions and hints.
-
Purplemath offers comprehensive lessons on simplifying radical expressions with clear explanations, examples, and practice problems to reinforce learning.
-
Varsity Tutors provides detailed explanations and examples on simplifying radicals, including techniques for combining like radicals and rationalizing denominators.
These resources offer a range of tools from interactive tutorials to printable worksheets, helping students practice and master the skill of simplifying radical expressions effectively.

Tìm hiểu cách đơn giản hóa biểu thức căn bậc hai với video hướng dẫn chi tiết và đáp án bài tập.
Đáp Án Bài Tập Đơn Giản Hóa Biểu Thức Căn Bậc Hai
READ MORE:
Học cách đơn giản hóa căn thức với biến số, số mũ, phân số và căn bậc ba qua video hướng dẫn chi tiết về đại số.
Đơn Giản Hóa Căn Thức Với Biến Số, Số Mũ, Phân Số, Căn Bậc Ba - Đại Số











