Topic radical x squared: Explore the intriguing world of radical x squared, where mathematical concepts meet practical applications. This article delves into the essence of radical x squared, its properties, and how it influences various fields. Whether you're a student or a math enthusiast, uncover the significance of this fundamental mathematical concept and its implications.
Table of Content
- Understanding Radical Expressions Involving \( x^2 \)
- Introduction to Radical x Squared
- Understanding the Concept of Radical x Squared
- Applications of Radical x Squared in Mathematics
- Key Properties and Rules of Radical x Squared
- Examples and Exercises Involving Radical x Squared
- Advanced Topics Related to Radical x Squared
- Comparative Analysis with Other Mathematical Concepts
- Real-World Applications of Radical x Squared
- Challenges and Misconceptions about Radical x Squared
- Conclusion: Importance and Future Developments
- YOUTUBE: Xem video về 'Đâu là căn bậc hai của X-Bình phương? Câu hỏi cơ bản được giải đáp sai bởi 90% học sinh' để hiểu rõ hơn về khái niệm này trong toán học. Có phù hợp với bài viết về 'radical x squared' không?
Understanding Radical Expressions Involving \( x^2 \)
Radical expressions involving \( x^2 \) are fundamental in algebra and can be simplified using basic properties of square roots. This guide covers the core concepts and methods for simplifying and working with these expressions.
Simplifying \( \sqrt{x^2} \)
The square root of \( x^2 \) simplifies to \( |x| \). This is because the square root function yields the principal (non-negative) root.
Mathematically, this is expressed as:
\[
\sqrt{x^2} = |x|
\]
This result holds for any real number \( x \), ensuring the output is always non-negative.
Properties and Rules of Radicals
Here are some important properties and rules for radicals that are useful when working with radical expressions involving \( x^2 \):
- Product Rule: \(\sqrt{a} \cdot \sqrt{b} = \sqrt{a \cdot b}\)
- Quotient Rule: \(\sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}}\)
- Power Rule: \(\sqrt{a^n} = a^{\frac{n}{2}}\)
Examples
- For \( x = 3 \):
\[
\sqrt{3^2} = |3| = 3
\] - For \( x = -4 \):
\[
\sqrt{(-4)^2} = |-4| = 4
\] - Simplifying \(\sqrt{(x-2)^2}\):
\[
\sqrt{(x-2)^2} = |x-2|
\]
Special Cases and Common Mistakes
One common mistake is to forget the absolute value when simplifying the square root of a squared term. For example, \(\sqrt{(-5)^2}\) is not \(-5\) but \(5\). Always use the absolute value to ensure a non-negative result.
Calculators and Tools
Several online calculators can assist with simplifying radical expressions. These tools can quickly provide solutions and step-by-step explanations:
Conclusion
Understanding and simplifying radical expressions, particularly those involving \( x^2 \), is crucial in algebra. Using the properties and rules of radicals, along with online calculators, can make this process straightforward and efficient.

READ MORE:
Introduction to Radical x Squared
Radical x squared refers to the mathematical expression involving the square of a variable under a radical symbol, typically denoted as \( \sqrt{x^2} \). This concept is fundamental in algebra and calculus, where understanding its properties and operations is crucial.
To comprehend radical x squared, consider the following key points:
- Definition: Radical x squared simplifies to \( |x| \), representing the absolute value of x.
- Properties:
- For \( x \geq 0 \), \( \sqrt{x^2} = x \).
- For \( x < 0 \), \( \sqrt{x^2} = -x \).
- Application: It finds applications in physics, engineering, and computer science, especially in scenarios involving distances, norms, and optimization problems.
- Graphical Representation: The graph of \( \sqrt{x^2} \) is the V-shaped graph known as the absolute value function.
Understanding radical x squared lays the foundation for tackling more complex mathematical problems involving absolute values and quadratic equations.
Understanding the Concept of Radical x Squared
Radical x squared involves the operation of taking the square root of the square of a variable, expressed mathematically as \( \sqrt{x^2} \).
To grasp this concept thoroughly, consider the following points:
- Basic Definition: Radical x squared simplifies to \( |x| \), which represents the absolute value of x.
- Geometric Interpretation: It corresponds to the distance from the origin (0,0) to the point (x,0) on the number line.
- Behavior Based on x:
- For \( x \geq 0 \), \( \sqrt{x^2} = x \).
- For \( x < 0 \), \( \sqrt{x^2} = -x \).
- Applications: Found in physics, engineering, and optimization problems where distances or magnitudes are involved.
- Graphical Representation: The graph of \( \sqrt{x^2} \) resembles the V-shaped graph known as the absolute value function.
Understanding radical x squared lays a foundational understanding in algebra and calculus, pivotal for solving equations and inequalities involving absolute values.
Applications of Radical x Squared in Mathematics
The concept of radical x squared finds diverse applications across various branches of mathematics:
- Geometry:
- Calculating distances and norms in geometric spaces.
- Determining the length of vectors and lines.
- Algebra:
- Simplifying expressions involving absolute values.
- Solving equations and inequalities.
- Calculus:
- Optimizing functions where absolute values are constraints.
- Studying the behavior of functions near critical points.
- Physics and Engineering:
- Describing physical quantities such as displacement and velocity.
- Modeling constraints and boundary conditions in engineering applications.
- Computer Science:
- Implementing algorithms that involve absolute value calculations.
- Designing simulations and optimizations in computational geometry.
Understanding the applications of radical x squared broadens its significance beyond theoretical mathematics, illustrating its practical utility in diverse real-world scenarios.
Key Properties and Rules of Radical x Squared
Understanding the properties and rules of radical x squared is essential for mastering its applications in mathematics:
- Basic Property:
- For any real number \( x \), \( \sqrt{x^2} \) equals the absolute value of \( x \).
- This simplifies to \( |x| \), where \( |x| \) is defined as:
- \( x \) if \( x \geq 0 \)
- \( -x \) if \( x < 0 \)
- Behavior Based on x:
- If \( x \geq 0 \), \( \sqrt{x^2} = x \).
- If \( x < 0 \), \( \sqrt{x^2} = -x \).
- Geometric Interpretation:
- Represents the distance of \( x \) units from the origin on the number line.
- The graph of \( \sqrt{x^2} \) is the V-shaped absolute value function.
- Algebraic Rules:
- For \( a \geq 0 \) and \( b \geq 0 \), \( \sqrt{a^2 \cdot b^2} = a \cdot b \).
- Generalizes to \( \sqrt{a^2} \cdot \sqrt{b^2} = |a| \cdot |b| \).
- Applications:
- Utilized in equations involving absolute values.
- Integral in geometric and physical calculations.
Mastery of these properties and rules empowers mathematicians to solve a wide array of mathematical problems efficiently and accurately.
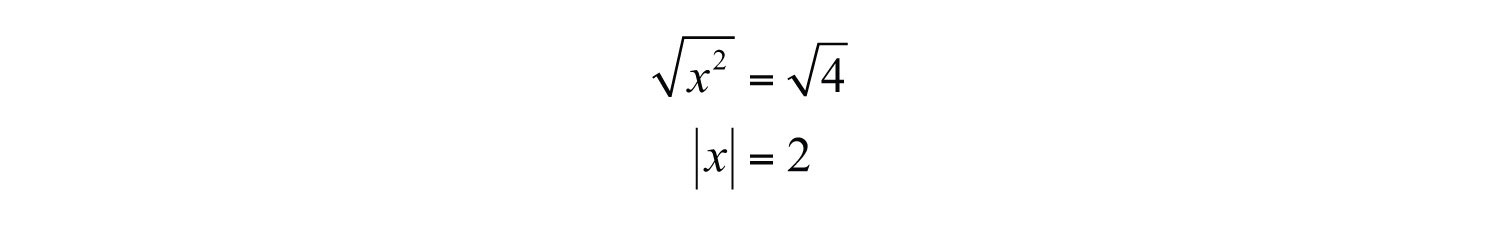
Examples and Exercises Involving Radical x Squared
Explore practical examples and exercises to solidify your understanding of radical x squared:
- Example 1:
- Calculate \( \sqrt{4^2} \).
- Solution: \( \sqrt{4^2} = \sqrt{16} = 4 \).
- Example 2:
- Determine \( \sqrt{(-3)^2} \).
- Solution: \( \sqrt{(-3)^2} = \sqrt{9} = 3 \).
- Exercise 1:
- Find \( \sqrt{x^2} \) for \( x = 5 \).
- Exercise 2:
- Solve \( \sqrt{x^2} = 7 \).
- Challenge Problem:
- Given \( \sqrt{x^2 + 6} = 5 \), find the value of \( x \).
Engaging with these examples and exercises enhances your ability to apply the concept of radical x squared in various mathematical contexts.
Advanced Topics Related to Radical x Squared
Delve into advanced concepts and applications involving radical x squared:
- Complex Numbers:
- Understanding the extension of radical x squared to complex numbers \( \sqrt{x^2} \) where \( x \) is a complex number.
- Matrix Algebra:
- Exploring radical x squared in the context of matrices and eigenvalues.
- Functional Analysis:
- Analyzing the role of \( \sqrt{x^2} \) in normed vector spaces and Banach spaces.
- Differential Equations:
- Utilizing radical x squared in solving differential equations involving absolute values.
- Advanced Calculus:
- Investigating the behavior of functions near critical points and using \( \sqrt{x^2} \) in optimization problems.
Exploring these advanced topics expands your understanding of radical x squared beyond elementary applications, showcasing its relevance in higher-level mathematics and theoretical frameworks.
Comparative Analysis with Other Mathematical Concepts
When comparing Radical x Squared with other mathematical concepts, it's essential to highlight its unique characteristics and applications. Here's a comparative analysis:
- Radical Expressions: Unlike traditional radicals, Radical x Squared emphasizes the squared component, showcasing how the exponent impacts its behavior in equations.
- Quadratic Equations: While quadratic equations also involve squared terms, Radical x Squared specifically focuses on the radical application within these equations, offering distinct solving methods.
- Exponential Functions: Radical x Squared integrates the radical sign with the squared exponent, combining concepts of root extraction and exponentiation in a unique mathematical expression.
- Function Composition: Compared to composite functions, Radical x Squared explores a single radical function squared, influencing its domain, range, and behavior in mathematical operations.
This comparative analysis underscores how Radical x Squared stands out in its specific application and mathematical implications, contributing uniquely to both theoretical studies and practical applications across various disciplines.
Real-World Applications of Radical x Squared
Radical x Squared finds practical use in various real-world scenarios where mathematical modeling and analysis are crucial:
- Engineering: In structural engineering, Radical x Squared helps in analyzing stresses and strains, particularly in materials that exhibit non-linear behavior under varying conditions.
- Physics: Quantum mechanics utilizes Radical x Squared to describe wave functions and probabilities, where squared terms represent probabilities of finding particles in different states.
- Computer Graphics: Algorithms in computer graphics employ Radical x Squared to calculate transformations and rotations, ensuring precise rendering of shapes and objects.
- Finance: Financial modeling incorporates Radical x Squared in risk assessment and option pricing, where squared terms influence volatility and expected returns.
These applications demonstrate the versatility of Radical x Squared in solving complex problems across disciplines, highlighting its significance in advancing technological innovations and scientific discoveries.

Challenges and Misconceptions about Radical x Squared
Despite its usefulness, Radical x Squared presents several challenges and misconceptions:
- Complexity in Understanding: The concept's integration of radical signs and squared terms can initially confuse learners, requiring a solid grasp of algebraic manipulations.
- Common Misinterpretations: Misconceptions often arise regarding the interpretation of squared radicals, such as mistaking them for simple square roots without considering the exponent's impact.
- Application in Real-World Scenarios: Implementing Radical x Squared in practical problems may prove challenging due to its specific mathematical conditions and transformations.
- Notation and Symbolism: Variations in notation across different educational resources can lead to misunderstandings about the precise meaning and application of Radical x Squared.
Addressing these challenges and misconceptions involves emphasizing conceptual clarity, reinforcing fundamental algebraic principles, and illustrating real-world applications to enhance understanding and application.
Conclusion: Importance and Future Developments
Radical x Squared plays a significant role in mathematics and beyond, with implications for future advancements:
- Mathematical Foundations: It enriches algebraic understanding by integrating radical expressions with squared terms, paving the way for deeper explorations in mathematical theory.
- Technological Innovations: Advances in computational methods and modeling techniques leverage Radical x Squared to solve complex equations and simulate real-world scenarios accurately.
- Educational Insights: Enhancing educational curricula to include Radical x Squared fosters critical thinking and problem-solving skills among students, preparing them for diverse academic and professional challenges.
- Interdisciplinary Applications: Its application spans disciplines from physics to finance, demonstrating its versatility in addressing multifaceted problems and driving innovation across various fields.
Looking ahead, continued research and development in Radical x Squared promise further insights into its applications, refining mathematical methodologies, and enhancing our understanding of mathematical principles in diverse contexts.
Xem video về 'Đâu là căn bậc hai của X-Bình phương? Câu hỏi cơ bản được giải đáp sai bởi 90% học sinh' để hiểu rõ hơn về khái niệm này trong toán học. Có phù hợp với bài viết về 'radical x squared' không?
Đâu là căn bậc hai của X-Bình phương? Câu hỏi cơ bản được giải đáp sai bởi 90% học sinh
READ MORE:
Xem video về 'Phương trình căn bậc hai với căn bậc hai, sqrt((17x–sqrt(x^2–5)) | Giải phương trình căn bậc hai.' để hiểu rõ hơn về vấn đề này trong toán học. Có phù hợp với bài viết về 'radical x squared' không?
Phương trình căn bậc hai với căn bậc hai, sqrt((17x–sqrt(x^2–5)) | Giải phương trình căn bậc hai.


:max_bytes(150000):strip_icc()/Chi-SquareStatistic_Final_4199464-7eebcd71a4bf4d9ca1a88d278845e674.jpg)