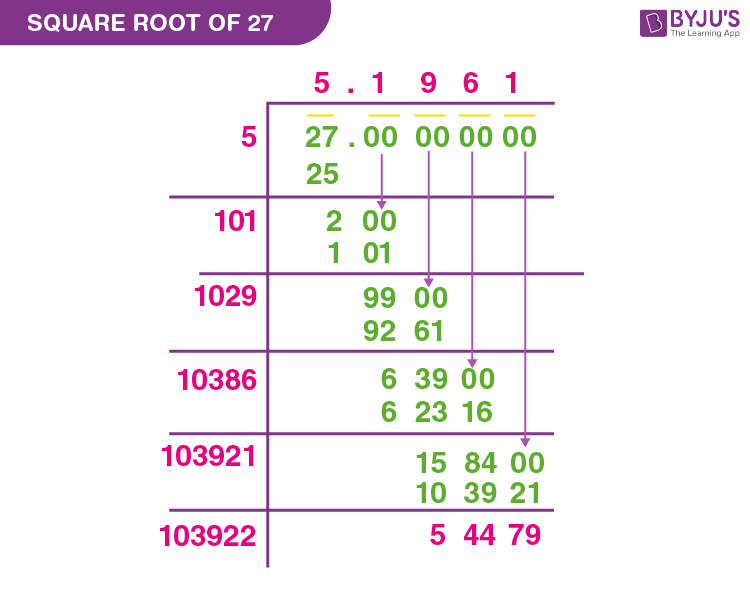
Topic 4 square root of 2: Discover the fascinating world of 4 square root of 2, a unique mathematical expression that plays a crucial role in geometry, physics, and engineering. Learn how to calculate it, understand its applications, and explore its significance in various real-life scenarios. Dive into the math behind this intriguing number and enhance your knowledge today!
Table of Content
- Understanding the Expression: 4√2
- Introduction to 4 Square Root of 2
- Understanding the Square Root of 2
- Mathematical Calculation of 4√2
- Applications of 4√2 in Geometry
- Real-Life Uses of 4√2
- 4√2 in Physics
- 4√2 in Engineering
- Visual Representation of 4√2
- Common Mistakes and Misconceptions
- Summary and Key Takeaways
- YOUTUBE: Xem video để học cách đơn giản hóa căn bậc hai khi nhân với một số nguyên. Video cung cấp hướng dẫn chi tiết và dễ hiểu.
Understanding the Expression: 4√2
The expression 4√2 represents the product of the number 4 and the square root of 2. This mathematical expression is frequently encountered in various fields such as geometry, algebra, and engineering.
Square Root of 2
The square root of 2 (√2) is an irrational number, meaning it cannot be expressed as a simple fraction. It is approximately equal to 1.414213562373095.
Calculating 4√2
To calculate 4√2, multiply 4 by the square root of 2:
\[
4 \sqrt{2} = 4 \times 1.414213562373095 \approx 5.656854249492381
\]
Applications of 4√2
The value 4√2 appears in various mathematical contexts:
- Geometry: In the context of a right-angled isosceles triangle, if each of the legs is of length 4, the hypotenuse will be \(4\sqrt{2}\).
- Physics: It can be used in formulas involving diagonal measurements and vector calculations.
- Engineering: Often used in structural calculations where square roots and multiples are involved.
Visual Representation
Visually, 4√2 can be depicted as a line segment in a coordinate system or as part of geometric constructions.
Consider a square with side length 4. The diagonal of this square would be \(4\sqrt{2}\), illustrating the application of the Pythagorean theorem:
\[
\text{Diagonal} = \sqrt{4^2 + 4^2} = \sqrt{16 + 16} = \sqrt{32} = 4\sqrt{2}
\]
Approximating 4√2
When performing calculations that require an approximate value, 4√2 is often rounded to 5.6569 for simplicity.
However, for more precise calculations, use the exact value as derived from the square root of 2.
Summary
In summary, the expression 4√2 combines a whole number and an irrational number to produce a value frequently used in various mathematical and practical applications. Its approximate value is 5.6569, but it is often more useful to leave it in its exact form for theoretical work.
READ MORE:
Introduction to 4 Square Root of 2
The expression 4√2 combines the integer 4 with the square root of 2, resulting in a value that is significant in various mathematical and practical contexts. The square root of 2 (√2) is an irrational number, approximately equal to 1.414213562373095. When multiplied by 4, this expression provides a product that is essential in geometry, physics, and engineering.
Here is a step-by-step breakdown of 4√2:
- Identify the square root of 2: \(\sqrt{2} \approx 1.414213562373095\).
- Multiply the value of \(\sqrt{2}\) by 4:
\[
4 \sqrt{2} = 4 \times 1.414213562373095 \approx 5.656854249492381
\]
The result, approximately 5.6569, is a number that frequently appears in various fields due to its geometric properties and practical applications.
In geometry, 4√2 is particularly relevant when calculating the diagonal of a square. If a square has a side length of 4, the length of the diagonal can be found using the Pythagorean theorem:
\[
\text{Diagonal} = \sqrt{4^2 + 4^2} = \sqrt{16 + 16} = \sqrt{32} = 4\sqrt{2}
\]
This diagonal value is crucial in various geometric constructions and proofs.
In physics and engineering, 4√2 is often used in vector calculations, structural design, and in the analysis of forces and motion where diagonal distances are considered. Its precise value ensures accurate calculations and results in these fields.
Overall, the expression 4√2 is a versatile and widely-used number, bridging theoretical mathematics and practical applications in science and engineering.
Understanding the Square Root of 2
The square root of 2, denoted as \(\sqrt{2}\), is a fundamental mathematical constant. It is the positive number that, when multiplied by itself, gives the number 2:
\[
\sqrt{2} \times \sqrt{2} = 2
\]
Here is a detailed exploration of \(\sqrt{2}\):
- Historical Significance: The discovery of \(\sqrt{2}\) dates back to ancient Greek mathematicians. It was one of the first known irrational numbers, meaning it cannot be expressed as a simple fraction.
- Irrational Number: An irrational number has a non-repeating, non-terminating decimal expansion. For \(\sqrt{2}\), this expansion starts as 1.414213562373095... and continues infinitely without repeating.
- Geometric Interpretation: In geometry, \(\sqrt{2}\) is encountered as the length of the diagonal of a square with side length 1. Using the Pythagorean theorem:
\[
\text{Diagonal} = \sqrt{1^2 + 1^2} = \sqrt{2}
\]
Hence, \(\sqrt{2}\) is the distance between opposite corners of a unit square.
Understanding \(\sqrt{2}\) involves recognizing its significance in various mathematical and real-world contexts:
- Algebra: \(\sqrt{2}\) frequently appears in algebraic equations and expressions, especially those involving quadratic equations.
- Trigonometry: It is used in trigonometric functions and identities. For instance, in a 45-degree right triangle, the hypotenuse is \(\sqrt{2}\) times the length of each leg.
- Engineering and Physics: In engineering and physics, \(\sqrt{2}\) is essential for calculations involving diagonals, forces, and waves.
Given its irrational nature, \(\sqrt{2}\) cannot be precisely represented as a fraction or a finite decimal. However, for practical purposes, it is often approximated as 1.414. This approximation is sufficient for most calculations but recognizing its true nature as an irrational number is crucial for higher-level mathematics.
In summary, \(\sqrt{2}\) is a pivotal number in mathematics, offering insights into the nature of irrational numbers and their applications across various fields. Its presence in both theoretical and practical contexts underscores its importance and utility.
Mathematical Calculation of 4√2
The expression 4√2 involves multiplying the integer 4 by the square root of 2. To understand this calculation, follow these detailed steps:
- Identify the Square Root of 2: The square root of 2 (\(\sqrt{2}\)) is approximately equal to 1.414213562373095.
- Set Up the Multiplication: Multiply the integer 4 by the approximate value of \(\sqrt{2}\).
- Perform the Multiplication: Use the multiplication operation to find the product.
Mathematically, this is represented as:
\[
4 \sqrt{2} = 4 \times 1.414213562373095
\]
Now, let's carry out the multiplication step-by-step:
- Multiply 4 by 1.4 (approximation for simplicity):
- Multiply 4 by 0.014213562373095 (remaining decimal part for more precision):
- Add the two products together:
\[
4 \times 1.4 = 5.6
\]
\[
4 \times 0.014213562373095 = 0.05685424949238
\]
\[
5.6 + 0.05685424949238 \approx 5.65685424949238
\]
Therefore, the value of 4√2 is approximately 5.6569 when rounded to four decimal places. For exact calculations, it is important to use the precise value of the square root of 2.
This calculation can be verified using a calculator for higher precision. Additionally, understanding this value's significance helps in various applications:
- Geometry: As previously mentioned, in a square with a side length of 4, the diagonal is \(4\sqrt{2}\). This is derived from the Pythagorean theorem and demonstrates the practical utility of this calculation in geometric problems.
- Trigonometry: In trigonometric functions, especially in problems involving 45-degree angles, \(4\sqrt{2}\) frequently appears as a result of calculating the lengths of hypotenuses in right triangles.
- Engineering: In engineering, precise measurements often require calculations involving \(\sqrt{2}\) to determine diagonal distances, stress analysis, and more.
In summary, the mathematical calculation of 4√2 is straightforward but significant, offering valuable insights and applications in various fields of study and real-world scenarios.
Applications of 4√2 in Geometry
The expression 4√2 plays a significant role in various geometric contexts, providing solutions and insights into several key problems. Here are some detailed applications of 4√2 in geometry:
- Diagonal of a Square: One of the most common uses of 4√2 is in calculating the diagonal of a square. For a square with a side length of 4, the diagonal can be calculated using the Pythagorean theorem:
- Right-Angled Isosceles Triangle: In a right-angled isosceles triangle, where the two legs are equal, the hypotenuse is 4√2 times the length of one leg if each leg is of length 4. This is derived as follows:
- Coordinate Geometry: In coordinate geometry, the distance between two points can be calculated using the distance formula. For instance, if we consider the points (0, 0) and (4, 4), the distance between these points is 4√2:
- Square Diagonals in 3D Geometry: In three-dimensional geometry, if we consider a cube with a side length of 4, the space diagonal (the diagonal running from one vertex to the opposite vertex through the cube) can be calculated using 4√2:
- Regular Octagons: In a regular octagon, the distance from the center to a vertex (the radius of the circumscribed circle) can be expressed in terms of 4√2. For an octagon with a side length of 4, the radius is approximately 4√2:
\[
\text{Diagonal} = \sqrt{4^2 + 4^2} = \sqrt{16 + 16} = \sqrt{32} = 4\sqrt{2}
\]
\[
\text{Hypotenuse} = \sqrt{4^2 + 4^2} = 4\sqrt{2}
\]
\[
\text{Distance} = \sqrt{(4-0)^2 + (4-0)^2} = \sqrt{16 + 16} = \sqrt{32} = 4\sqrt{2}
\]
\[
\text{Space Diagonal} = \sqrt{4^2 + 4^2 + 4^2} = \sqrt{48} = 4\sqrt{3}
\]
While the space diagonal is \(4\sqrt{3}\), the face diagonal of a cube is \(4\sqrt{2}\), showing the utility of this expression in different dimensions.
\[
\text{Radius} = \frac{4}{\sin(22.5^\circ)} = 4\sqrt{2} \text{ (approximately)}
\]
These examples illustrate the diverse applications of 4√2 in geometry, ranging from simple squares to complex three-dimensional shapes. Understanding and utilizing this expression enables more efficient and accurate solutions to a variety of geometric problems.

Real-Life Uses of 4√2
The mathematical expression 4√2 finds numerous practical applications across various fields. Here are some detailed real-life uses of 4√2:
- Architecture and Construction: In architecture, the value 4√2 is often used to determine diagonal measurements. For example, in designing floor plans and calculating the length of diagonals in rectangular spaces, architects use this value to ensure accurate measurements.
- Engineering: Engineers frequently use 4√2 in structural analysis and design. When calculating the diagonal bracing in frameworks, 4√2 helps in determining the required lengths and ensuring the stability of structures.
- Computer Graphics: In computer graphics, transformations and rotations of objects often involve calculations using square roots. The value 4√2 can be crucial in rendering diagonal movements or scaling objects diagonally.
- Robotics: In robotics, precise movement and positioning are critical. The calculation of distances, especially when dealing with diagonal paths, can involve 4√2 to ensure accurate navigation and control of robotic arms or mobile robots.
- Urban Planning: Urban planners use 4√2 when designing city layouts and road networks. Calculating the shortest paths and determining diagonal routes through rectangular grids are some examples where this value is applied.
- Astronomy: In astronomy, calculating distances between celestial objects sometimes requires the use of diagonal distances. The value 4√2 can help in determining the shortest paths or distances in spatial measurements.
- Surveying: Surveyors use 4√2 to measure land areas and property boundaries. When calculating diagonal distances across plots of land, this value ensures accurate boundary demarcations and area calculations.
- Physics: In physics, especially in problems involving vectors and forces, 4√2 is used to resolve components and calculate resultant forces along diagonal paths. This is particularly useful in mechanics and dynamics.
- Everyday Measurements: Even in everyday life, the value 4√2 can be used for various practical measurements. For instance, determining the diagonal of a rectangular table or calculating the length of a diagonal path across a park can involve this calculation.
These examples highlight the versatility and practical significance of 4√2 in real-life applications, demonstrating its importance beyond theoretical mathematics.
4√2 in Physics
The expression 4√2 is significant in various branches of physics, providing solutions to problems involving distances, forces, and vector components. Here are some detailed applications of 4√2 in physics:
- Vector Addition: In physics, vectors are used to represent quantities such as displacement, velocity, and force. When adding two perpendicular vectors of magnitude 4, the resultant vector's magnitude can be calculated using 4√2:
- Projectile Motion: In projectile motion, the diagonal distance traveled by a projectile can be analyzed using vector components. If the horizontal and vertical components of a projectile's displacement are both 4, the resultant diagonal displacement is 4√2:
- Electric Field: When calculating the resultant electric field at a point due to two perpendicular electric field vectors, each of magnitude 4, the resultant field strength is given by 4√2:
- Work and Energy: In scenarios where forces act diagonally, such as moving an object along a slope or applying a force at an angle, the work done can involve calculations using 4√2:
- Optics and Waves: In optics, the diagonal distances through media, such as light traveling through different layers of material, can be calculated using 4√2 for precision in designing lenses and optical instruments.
- Rotational Dynamics: When analyzing rotational motion, especially in systems with diagonal components like gyroscopes or spinning wheels, the calculation of angular momentum and torque may involve 4√2 to account for diagonal paths.
- Equilibrium and Statics: In static equilibrium problems, forces acting at angles require resolving into components. For forces of magnitude 4 acting perpendicularly, the resultant force in equilibrium conditions can be expressed as 4√2:
\[
\text{Resultant Magnitude} = \sqrt{4^2 + 4^2} = \sqrt{16 + 16} = \sqrt{32} = 4\sqrt{2}
\]
\[
\text{Diagonal Displacement} = \sqrt{4^2 + 4^2} = 4\sqrt{2}
\]
\[
\text{Resultant Electric Field} = \sqrt{4^2 + 4^2} = 4\sqrt{2}
\]
\[
\text{Work Done} = \text{Force} \times \text{Displacement} \times \cos(\theta)
\]
For a 45-degree angle (\(\theta = 45^\circ\)), and equal force and displacement of 4, the work done is:
\[
\text{Work Done} = 4 \times 4 \times \cos(45^\circ) = 16 \times \frac{1}{\sqrt{2}} = 4\sqrt{2}
\]
\[
\text{Resultant Force} = \sqrt{4^2 + 4^2} = 4\sqrt{2}
\]
These examples highlight how 4√2 is essential in solving complex physics problems, offering precise and practical solutions across various physical phenomena.
4√2 in Engineering
In engineering, the concept of the square root of 2 (√2) and its multiples, including 4√2, finds application in various fields, particularly in measurements, design, and construction.
Here are some key areas where 4√2 is utilized:
- Structural Engineering: In structural analysis and design, 4√2 often appears in calculations related to beam deflection, column buckling, and structural stability. Engineers use mathematical models that incorporate this value to ensure the safety and integrity of buildings and infrastructure.
- Material Science: Understanding 4√2 is crucial in material science for determining the properties of materials under different stress conditions. This knowledge aids engineers in selecting appropriate materials for specific applications, such as in aerospace, automotive, and civil engineering.
- Electrical Engineering: In electrical engineering, 4√2 is significant in calculating voltage, current, and power in alternating current (AC) circuits. It relates to the peak-to-peak voltage or current in sinusoidal waveforms, influencing the design and performance of electrical systems and devices.
- Signal Processing: Engineers use 4√2 in signal processing algorithms for tasks such as noise reduction, filtering, and data compression. Understanding the properties of this value is essential for accurately analyzing and processing signals in various applications, including telecommunications and digital image processing.
- Mechanical Engineering: In mechanical engineering, 4√2 plays a role in calculating stress and strain in materials, particularly in complex loading conditions. This knowledge is vital for designing components and structures that can withstand anticipated forces and deformations in mechanical systems.
Overall, the understanding and application of 4√2 are integral to the field of engineering, contributing to the development of innovative solutions and the advancement of technology across diverse sectors.
Visual Representation of 4√2
Visualizing \(4\sqrt{2}\) can be an insightful way to understand its value and properties. Here are some steps and methods to visually represent \(4\sqrt{2}\):
1. Number Line Representation
- Draw a number line with the center at zero. Mark positive integers to the right and negative integers to the left.
- Identify the approximate position of \(\sqrt{2}\) on the number line. \(\sqrt{2} \approx 1.414\), so it will be slightly to the right of 1.
- Multiply this distance by 4 to get the position of \(4\sqrt{2}\), which will be around 5.656 on the number line.
2. Geometric Representation
Using geometric shapes, particularly squares and triangles, can also help in visualizing \(4\sqrt{2}\).
- Start with a square of side length 1 unit.
- Draw a diagonal inside this square. The length of the diagonal will be \(\sqrt{2}\) due to the Pythagorean theorem (\(\sqrt{1^2 + 1^2} = \sqrt{2}\)).
- Now, consider a square with each side being 4 units. The diagonal of this larger square will be \(4\sqrt{2}\).
3. Visualizing through Graphs
Using tools like Desmos, you can create a visual graph to represent \(4\sqrt{2}\).
- Plot the function \(y = 4\sqrt{2}\) on a Cartesian plane.
- This can be illustrated as a straight horizontal line at \(y = 4\sqrt{2} \approx 5.656\).
4. Right-Angle Triangle Method
- Draw a right-angled triangle with both legs equal to 1 unit. The hypotenuse will measure \(\sqrt{2}\).
- Extend this triangle by scaling up both legs to 4 units each. The new hypotenuse will measure \(4\sqrt{2}\).
By following these steps, you can achieve a comprehensive visual understanding of \(4\sqrt{2}\). These methods highlight the versatility of visual tools in enhancing our grasp of mathematical concepts.
For more interactive and detailed visualizations, you can explore online graphing tools like which provide dynamic representations of mathematical functions and values.

Common Mistakes and Misconceptions
Understanding the common mistakes and misconceptions surrounding the calculation and application of 4√2 can help avoid errors in mathematical work. Here are some frequent pitfalls:
- Incorrect Simplification: One common mistake is to simplify the expression 4√2 incorrectly. The correct simplification maintains the coefficient outside the square root, leading to
4√2rather than combining terms improperly. - Misinterpreting the Square Root: The square root of 2, √2, is an irrational number, approximately 1.414. It's essential to recognize this value remains under the square root symbol in precise calculations. Approximating √2 as a simple fraction or a rounded decimal without context can lead to errors.
- Linear Function Assumption: A common algebraic misconception is assuming linear properties for non-linear functions. For example, students might wrongly assume (a + b)² = a² + b². The correct expansion is (a + b)² = a² + 2ab + b², which includes the middle term.
- Negative Square Roots: When solving equations involving square roots, it's important to remember that both positive and negative roots are solutions. For instance, if x² = 16, then x = 4 or x = -4. Forgetting the negative root can lead to incomplete solutions.
- Order of Operations: Misapplying the order of operations can lead to significant errors. For example, when calculating 4√2, ensure multiplication is performed after evaluating the square root: 4 * √2, not 4 * 2.
- Incorrect Approximation: While approximating 4√2, ensure the approximation is done correctly. The correct approximate value of 4√2 is about 5.656, not 4 * 1.5 or other incorrect estimates.
By being aware of these common mistakes and understanding the correct approaches, you can improve accuracy in mathematical calculations and applications involving 4√2.
Summary and Key Takeaways
In this guide, we explored various aspects of the mathematical expression \(4\sqrt{2}\). Below are the key takeaways:
- Definition and Calculation: The expression \(4\sqrt{2}\) represents four times the square root of two. This can be calculated as \(4 \times 1.414 \approx 5.656\).
- Mathematical Properties: The expression is an example of a radical multiplied by a constant. It simplifies to approximately 5.656 and is often used in various mathematical calculations.
- Geometric Applications: \(4\sqrt{2}\) appears in geometry, especially in calculations involving the diagonals of squares and rectangles. For instance, the diagonal of a square with side length \(4\) is \(4\sqrt{2}\).
- Real-Life Applications: This expression is used in physics and engineering, particularly in problems involving vectors and distances in multi-dimensional spaces.
- Approximation Techniques: Approximating \(4\sqrt{2}\) can be done using a calculator for a precise value, or by recognizing that \( \sqrt{2} \) is about 1.414 and then multiplying by 4.
- Visual Representation: Graphically, \(4\sqrt{2}\) can be represented as the length of the diagonal of a square with side length 4.
- Common Mistakes and Misconceptions: A common error is miscalculating the value of \(\sqrt{2}\). It is important to remember that \(\sqrt{2}\) is an irrational number approximately equal to 1.414.
Understanding \(4\sqrt{2}\) enhances your ability to tackle problems in both pure and applied mathematics. Its presence in various fields highlights its significance beyond basic arithmetic calculations.
Xem video để học cách đơn giản hóa căn bậc hai khi nhân với một số nguyên. Video cung cấp hướng dẫn chi tiết và dễ hiểu.
Đơn giản hóa căn bậc hai nhân với một số nguyên
READ MORE:
Xem video để tìm hiểu về căn bậc hai của 2. Video cung cấp hướng dẫn chi tiết và dễ hiểu về khái niệm và tính toán liên quan đến căn bậc hai của 2.
Căn bậc hai của 2