Topic proof by contradiction square root of 2 is irrational: Discover the elegant proof by contradiction that demonstrates the irrationality of the square root of 2. This article breaks down each step, making it easy to understand how mathematicians have proven that √2 cannot be expressed as a fraction, highlighting the beauty and logic of mathematical reasoning.
Table of Content
- Proof by Contradiction: The Square Root of 2 is Irrational
- Introduction
- What is an Irrational Number?
- Understanding Rational Numbers
- Historical Background of the Irrationality of √2
- Proof by Contradiction Method
- Step-by-Step Proof of the Irrationality of √2
- Assumption: √2 is Rational
- Squaring the Assumption
- Implications for Even and Odd Numbers
- Demonstrating Even Nature of a
- Substitution and Simplification
- Demonstrating Even Nature of b
- Contradiction of Coprimality
- Conclusion: √2 is Irrational
- Implications and Applications
- Further Reading and Resources
- References
- YOUTUBE:
Proof by Contradiction: The Square Root of 2 is Irrational
The proof by contradiction is a classical method to demonstrate that the square root of 2 is irrational. An irrational number is a number that cannot be expressed as a fraction of two integers. Here is the detailed proof:
Assumption
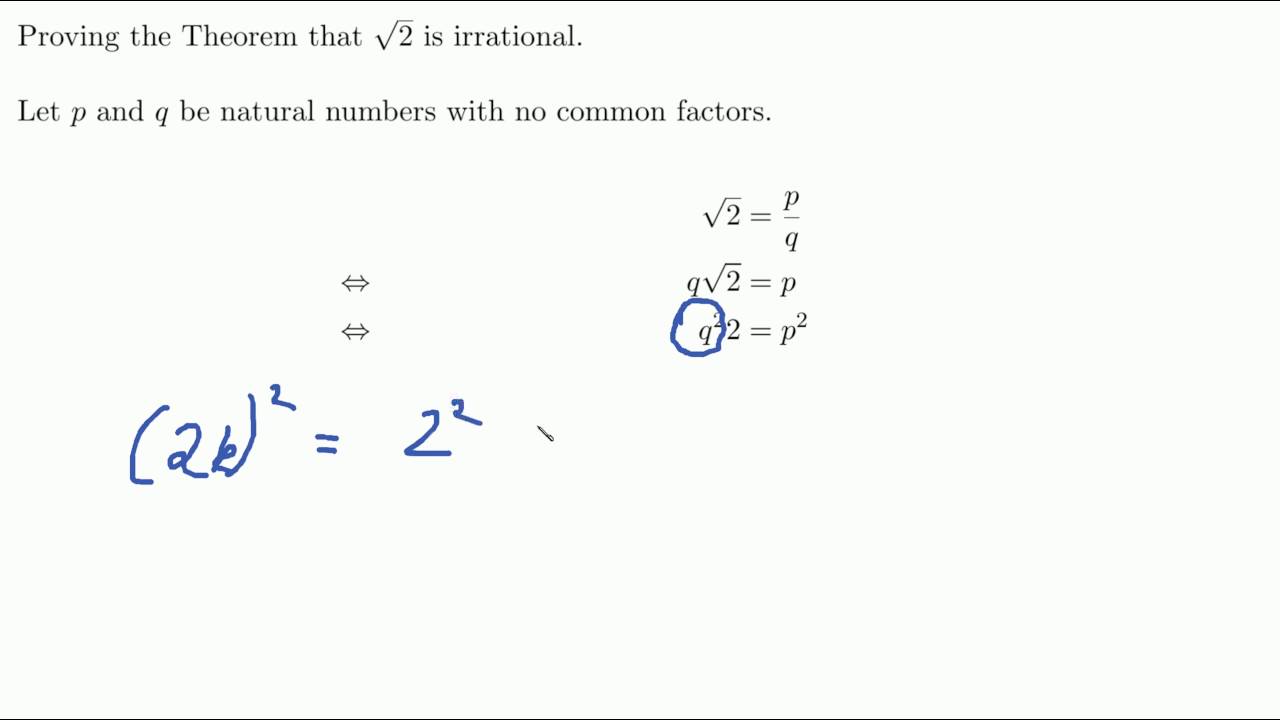
Assume, for the sake of contradiction, that \( \sqrt{2} \) is rational. This means that \( \sqrt{2} \) can be written as a fraction of two integers \( \frac{a}{b} \), where \( a \) and \( b \) are coprime integers (i.e., their greatest common divisor is 1).
So, we have:
\[ \sqrt{2} = \frac{a}{b} \]
Squaring both sides of the equation gives:
\[ 2 = \frac{a^2}{b^2} \]
Multiplying both sides by \( b^2 \) results in:
\[ 2b^2 = a^2 \]
Parity Argument
This equation shows that \( a^2 \) is even because it is equal to \( 2b^2 \) (an even number times 2 is even). Since \( a^2 \) is even, \( a \) must also be even (the square of an odd number is odd). Thus, we can write \( a \) as:
\[ a = 2k \]
for some integer \( k \). Substituting \( a = 2k \) into the equation \( 2b^2 = a^2 \) gives:
\[ 2b^2 = (2k)^2 \]
\[ 2b^2 = 4k^2 \]
\[ b^2 = 2k^2 \]
This implies that \( b^2 \) is also even, and therefore \( b \) must be even.
Contradiction
If both \( a \) and \( b \) are even, they share a common factor of 2. This contradicts our initial assumption that \( a \) and \( b \) are coprime (having no common factors other than 1). Therefore, our assumption that \( \sqrt{2} \) is rational must be false.
Conclusion
Since the assumption that \( \sqrt{2} \) is rational leads to a contradiction, we conclude that \( \sqrt{2} \) is irrational.

READ MORE:
Introduction
The concept of irrational numbers is fundamental in mathematics. Among the most famous irrational numbers is the square root of 2. The proof that \( \sqrt{2} \) is irrational is a classic example of a proof by contradiction, a powerful method used to establish the truth of a statement by demonstrating that assuming the opposite leads to a contradiction. This method not only confirms the irrationality of \( \sqrt{2} \) but also provides insight into the nature of numbers.
In this article, we will explore the proof by contradiction in detail. We will break down each step of the proof, starting with the assumption that \( \sqrt{2} \) is rational and leading to the inevitable contradiction that reveals its irrationality. By the end of this discussion, you will have a clear understanding of why \( \sqrt{2} \) cannot be expressed as a ratio of two integers.
- Assume \( \sqrt{2} \) is rational.
- Express \( \sqrt{2} \) as \( \frac{a}{b} \) where \( a \) and \( b \) are coprime integers.
- Square both sides to obtain \( 2 = \frac{a^2}{b^2} \).
- Multiply by \( b^2 \) to get \( 2b^2 = a^2 \).
- Show that \( a^2 \) is even, implying \( a \) is even.
- Express \( a \) as \( 2k \) and substitute back to get \( 2b^2 = 4k^2 \).
- Simplify to \( b^2 = 2k^2 \), showing \( b^2 \) is even, implying \( b \) is even.
- Conclude that both \( a \) and \( b \) are even, contradicting the coprime assumption.
- Thus, \( \sqrt{2} \) is irrational.
What is an Irrational Number?
An irrational number is a type of real number that cannot be expressed as a simple fraction or a ratio of two integers. This means that an irrational number cannot be written in the form \( \frac{a}{b} \), where \( a \) and \( b \) are integers, and \( b \) is not zero. Irrational numbers have non-repeating, non-terminating decimal expansions, which means their decimal representation goes on forever without repeating a pattern.
Some of the most well-known examples of irrational numbers include:
- \( \pi \) (Pi), the ratio of the circumference of a circle to its diameter.
- \( e \) (Euler's number), the base of the natural logarithm.
- \( \sqrt{2} \), the square root of 2, which we will prove to be irrational.
To better understand irrational numbers, let's compare them with rational numbers:
| Rational Numbers | Irrational Numbers |
|---|---|
| Can be written as a fraction \( \frac{a}{b} \) | Cannot be written as a fraction \( \frac{a}{b} \) |
| Have terminating or repeating decimal expansions | Have non-terminating, non-repeating decimal expansions |
| Examples: \( \frac{1}{2} \), \( 0.75 \), \( -3 \) | Examples: \( \pi \), \( e \), \( \sqrt{2} \) |
Understanding the distinction between rational and irrational numbers is crucial in many areas of mathematics, as it helps in grasping the structure of the number system and the properties of different types of numbers.
Understanding Rational Numbers
Rational numbers are a fundamental concept in mathematics. They are defined as numbers that can be expressed as the quotient or fraction \( \frac{a}{b} \) of two integers, where \( a \) (the numerator) and \( b \) (the denominator) are integers, and \( b \) is not zero. The set of rational numbers is denoted by \( \mathbb{Q} \).
Key properties of rational numbers include:
- Fraction Representation: Every rational number can be written as a fraction \( \frac{a}{b} \). For example, \( \frac{1}{2} \), \( -\frac{3}{4} \), and \( 5 \) (which can be written as \( \frac{5}{1} \)).
- Terminating or Repeating Decimals: When expressed in decimal form, rational numbers either terminate (end after a finite number of digits) or repeat a sequence of digits. For example, \( \frac{1}{4} = 0.25 \) (terminating) and \( \frac{1}{3} = 0.333\ldots \) (repeating).
- Closed Under Arithmetic Operations: The sum, difference, product, and quotient (except division by zero) of two rational numbers are also rational numbers. For example, \( \frac{1}{2} + \frac{1}{3} = \frac{3}{6} + \frac{2}{6} = \frac{5}{6} \), which is rational.
Let's explore some examples to solidify our understanding:
| Rational Number | Fraction Form | Decimal Form |
|---|---|---|
| One-half | \( \frac{1}{2} \) | 0.5 |
| One-third | \( \frac{1}{3} \) | 0.333... |
| Negative three-fourths | \( -\frac{3}{4} \) | -0.75 |
| Two | \( \frac{2}{1} \) | 2.0 |
Rational numbers are dense in the real numbers, meaning between any two rational numbers, there is another rational number. This density property is crucial in analysis and helps in approximating real numbers by rationals.
Understanding rational numbers is essential because it sets the stage for exploring more complex number systems, such as irrational numbers, which cannot be expressed in this fractional form. This distinction is vital in various fields of mathematics and its applications.
Historical Background of the Irrationality of √2
The discovery of irrational numbers, specifically the irrationality of \( \sqrt{2} \), is a significant milestone in the history of mathematics. The concept dates back to ancient Greece and is often attributed to the Pythagorean school of thought.
The Pythagoreans, followers of the mathematician Pythagoras, believed that all numbers could be expressed as the ratio of two integers (rational numbers). This belief was deeply rooted in their philosophy, which considered numbers to be the foundation of all reality. However, the discovery of the irrationality of \( \sqrt{2} \) challenged their worldview.
The story goes that a member of the Pythagorean school, Hippasus, was the first to prove that \( \sqrt{2} \) is irrational. According to legend, this discovery was so disturbing to the Pythagoreans that they drowned Hippasus to prevent the dissemination of this unsettling truth. Although this story's accuracy is debated, it highlights the profound impact of the discovery on the mathematical community of the time.
To understand why \( \sqrt{2} \) was such a groundbreaking discovery, consider the following historical context:
- Geometric Interpretation: The Pythagoreans initially encountered the irrationality of \( \sqrt{2} \) through geometry. Specifically, they studied the diagonal of a square with side length 1. According to the Pythagorean theorem, the length of the diagonal is \( \sqrt{2} \), which could not be expressed as a ratio of two integers.
- Mathematical Proof: The formal proof of the irrationality of \( \sqrt{2} \) involves the method of contradiction. This proof demonstrates that assuming \( \sqrt{2} \) is rational leads to a logical inconsistency, thus proving it is irrational. This method was a significant advancement in mathematical reasoning.
- Impact on Mathematics: The discovery of irrational numbers expanded the understanding of the number system. It showed that not all quantities could be expressed as ratios of integers, leading to the development of real numbers and further advancements in mathematical analysis.
The irrationality of \( \sqrt{2} \) is not just a mathematical curiosity; it represents a paradigm shift in how numbers and their properties are understood. This discovery laid the groundwork for the rigorous study of numbers and set the stage for future mathematical breakthroughs.

Proof by Contradiction Method
The proof by contradiction is a powerful and elegant method used in mathematics to establish the truth of a statement. It involves assuming the opposite of what you want to prove, and then showing that this assumption leads to a contradiction. This contradiction implies that the original assumption must be false, thereby proving the original statement true.
The proof by contradiction method follows these general steps:
- Assume the Negation: Begin by assuming the negation of the statement you want to prove. In the case of proving that \( \sqrt{2} \) is irrational, you start by assuming that \( \sqrt{2} \) is rational.
- Logical Deductions: Use logical deductions and known properties of numbers to explore the consequences of this assumption. For \( \sqrt{2} \), this means expressing it as a ratio of two integers and examining their properties.
- Reach a Contradiction: Show that the logical deductions lead to a contradiction with established facts or definitions. For the proof of the irrationality of \( \sqrt{2} \), this involves demonstrating that the integers in the assumed ratio cannot both be even and coprime at the same time.
- Conclude the Proof: Conclude that since the assumption leads to a contradiction, the original statement must be true. Therefore, \( \sqrt{2} \) is irrational.
Let's apply the proof by contradiction method to prove that \( \sqrt{2} \) is irrational:
- Assume \( \sqrt{2} \) is rational: Suppose \( \sqrt{2} = \frac{a}{b} \), where \( a \) and \( b \) are coprime integers (having no common factors other than 1).
- Square Both Sides: Square both sides of the equation to obtain: \[ 2 = \frac{a^2}{b^2} \]
- Clear the Fraction: Multiply both sides by \( b^2 \) to get: \[ 2b^2 = a^2 \]
- Even and Odd Properties: Since \( a^2 \) is even (because it equals \( 2b^2 \)), \( a \) must be even (the square of an odd number is odd). Let \( a = 2k \) for some integer \( k \).
- Substitute \( a = 2k \): Substitute \( a = 2k \) into the equation \( 2b^2 = a^2 \) to get: \[ 2b^2 = (2k)^2 \] \[ 2b^2 = 4k^2 \] \[ b^2 = 2k^2 \]
- Even \( b \): This implies \( b^2 \) is also even, so \( b \) must be even. Therefore, both \( a \) and \( b \) are even, which means they have a common factor of 2.
- Contradiction: This contradicts the assumption that \( a \) and \( b \) are coprime.
- Conclusion: Since the assumption leads to a contradiction, we conclude that \( \sqrt{2} \) is irrational.
The proof by contradiction method not only proves the irrationality of \( \sqrt{2} \) but also illustrates the power and elegance of logical reasoning in mathematics.
Step-by-Step Proof of the Irrationality of √2
To prove that \( \sqrt{2} \) is irrational, we will use a classic proof by contradiction. Follow these detailed steps:
- Assume the Opposite: Assume \( \sqrt{2} \) is rational. This means that it can be expressed as a ratio of two integers. Let's write: \[ \sqrt{2} = \frac{a}{b} \] where \( a \) and \( b \) are coprime integers (i.e., their greatest common divisor is 1).
- Square Both Sides: Square both sides of the equation to remove the square root: \[ 2 = \frac{a^2}{b^2} \]
- Clear the Fraction: Multiply both sides of the equation by \( b^2 \) to eliminate the denominator: \[ 2b^2 = a^2 \]
- Analyze the Equation: Notice that \( a^2 \) is an even number because it is equal to \( 2b^2 \) (two times a number is always even). Since \( a^2 \) is even, \( a \) must also be even (because the square of an odd number is odd).
- Express \( a \) in Terms of an Integer: Since \( a \) is even, we can write \( a \) as: \[ a = 2k \] for some integer \( k \).
- Substitute \( a = 2k \): Substitute \( a = 2k \) back into the equation \( 2b^2 = a^2 \): \[ 2b^2 = (2k)^2 \] \[ 2b^2 = 4k^2 \] \[ b^2 = 2k^2 \]
- Analyze the New Equation: This equation \( b^2 = 2k^2 \) shows that \( b^2 \) is also even, which means \( b \) must be even as well (for the same reason as \( a \)).
- Contradiction: Since both \( a \) and \( b \) are even, they have a common factor of 2. This contradicts our initial assumption that \( a \) and \( b \) are coprime (having no common factors other than 1).
- Conclusion: Because our assumption that \( \sqrt{2} \) is rational leads to a contradiction, we conclude that \( \sqrt{2} \) is irrational.
This step-by-step proof illustrates the power of the proof by contradiction method, showing that \( \sqrt{2} \) cannot be expressed as a ratio of two integers and is therefore irrational.
Assumption: √2 is Rational
To prove by contradiction that the square root of 2 is irrational, we start by assuming the opposite: that √2 is a rational number. This means we can express √2 as a fraction of two integers, p and q, where p and q are coprime (i.e., they have no common factors other than 1) and q ≠ 0.
So, we assume:
By squaring both sides of the equation, we get:
Rewriting this, we find:
This implies that is even, since it equals 2 times a square number. For to be even, p must also be even (since the square of an odd number is odd).
Let's express p as 2k for some integer k:
Substituting p = 2k back into the equation, we get:
Dividing both sides by 2, we find:
This implies that is also even, and thus q must be even. Now we have shown that both p and q are even, which contradicts our initial assumption that p and q are coprime (since they both share 2 as a common factor).
This contradiction implies that our initial assumption that √2 is rational is false. Therefore, √2 must be irrational.
Squaring the Assumption
Assume that \( \sqrt{2} \) is a rational number. By definition, this means it can be expressed as a fraction of two integers \( p \) and \( q \), where \( p \) and \( q \) have no common factors (i.e., the fraction is in its simplest form) and \( q \neq 0 \).
Therefore, we can write:
\[ \sqrt{2} = \frac{p}{q} \]
Next, we square both sides of the equation to remove the square root:
\[ (\sqrt{2})^2 = \left( \frac{p}{q} \right)^2 \]
Which simplifies to:
\[ 2 = \frac{p^2}{q^2} \]
To clear the fraction, we multiply both sides by \( q^2 \):
\[ 2q^2 = p^2 \]
This equation tells us that \( p^2 \) is an even number because it is equal to \( 2 \times q^2 \), which is clearly even (as it is two times some integer). Since \( p^2 \) is even, \( p \) itself must also be even (because the square of an odd number is odd).
Therefore, we can write \( p \) as:
\[ p = 2k \]
where \( k \) is an integer. Substituting \( 2k \) for \( p \) in the equation \( 2q^2 = p^2 \) gives us:
\[ 2q^2 = (2k)^2 \]
which simplifies to:
\[ 2q^2 = 4k^2 \]
Dividing both sides by 2, we get:
\[ q^2 = 2k^2 \]
This equation shows that \( q^2 \) is also even, which implies that \( q \) must be even as well (since the square of an odd number is odd).
Since both \( p \) and \( q \) are even, they have a common factor of 2. This contradicts our initial assumption that \( p \) and \( q \) have no common factors and that the fraction \( \frac{p}{q} \) is in its simplest form.
Therefore, our initial assumption that \( \sqrt{2} \) is rational must be false. This proves that \( \sqrt{2} \) is irrational.

Implications for Even and Odd Numbers
To further understand the proof by contradiction for the irrationality of √2, we need to explore the implications for even and odd numbers. These properties play a crucial role in deriving the contradiction.
Recall our assumption: we started with √2 being a rational number, which can be expressed as a fraction of two integers \( \frac{p}{q} \) in simplest form, where \( p \) and \( q \) are coprime (they have no common factors other than 1).
- Given \( \sqrt{2} = \frac{p}{q} \), squaring both sides yields:
\( 2 = \frac{p^2}{q^2} \)
This can be rearranged to:
\( 2q^2 = p^2 \) - From the equation \( 2q^2 = p^2 \), we observe that \( p^2 \) must be even because it is equal to \( 2 \) times \( q^2 \). Since \( p^2 \) is even, \( p \) must also be even (the square of an odd number is odd).
Let \( p = 2k \) for some integer \( k \). - Substituting \( p = 2k \) into the equation \( 2q^2 = p^2 \) gives:
\( 2q^2 = (2k)^2 \)
\( 2q^2 = 4k^2 \)
Dividing both sides by 2:
\( q^2 = 2k^2 \) - From \( q^2 = 2k^2 \), we see that \( q^2 \) must also be even, which implies that \( q \) is even.
Let \( q = 2m \) for some integer \( m \).
At this point, we have shown that both \( p \) and \( q \) are even, which means both can be divided by 2. This contradicts our original assumption that \( \frac{p}{q} \) is in its simplest form with \( p \) and \( q \) being coprime.
Thus, we arrive at a contradiction, proving that our initial assumption—that \( \sqrt{2} \) is rational—is false. Therefore, \( \sqrt{2} \) must be irrational.
Demonstrating Even Nature of a
To demonstrate that \(a\) is even, we start from our previous equation:
\[ a^2 = 2b^2 \]
This equation shows that \(a^2\) is twice some integer (\(b^2\)), which means \(a^2\) must be even. This is because any number that is twice another number is even.
Next, we need to understand that if \(a^2\) is even, \(a\) itself must also be even. This is due to the fact that the square of an odd number is always odd. Mathematically, we can express an even number as \(2k\) where \(k\) is an integer. Thus, we can write:
\[ a = 2k \]
Substituting \(a = 2k\) back into the original equation \(a^2 = 2b^2\), we get:
\[ (2k)^2 = 2b^2 \]
Simplifying this, we have:
\[ 4k^2 = 2b^2 \]
Dividing both sides by 2, we get:
\[ 2k^2 = b^2 \]
This equation indicates that \(b^2\) is also even, and consequently, \(b\) must be even because, as previously noted, the square of an odd number cannot be even.
Therefore, we have demonstrated that both \(a\) and \(b\) must be even. This will lead us to the next step in our proof where we will show that this leads to a contradiction of our initial assumption that \(a\) and \(b\) are coprime (having no common factors other than 1).
Substitution and Simplification
Having established that both a and b are even, we can express them as:
- a = 2m for some integer m
- b = 2n for some integer n
We substitute a = 2m into the equation 2b2 = a2:
\[ 2b^2 = (2m)^2 \]
This simplifies to:
\[ 2b^2 = 4m^2 \]
Next, we divide both sides of the equation by 2:
\[ b^2 = 2m^2 \]
From this, we can see that b2 is also even because it is equal to 2 times another integer (2m2), meaning b must be even.
We now have both a and b as even numbers. This contradicts our initial assumption that a and b are coprime (they have no common factors other than 1). If both are even, they share at least the factor 2.
Therefore, our assumption that \(\sqrt{2}\) is rational leads to a contradiction. Thus, we conclude that \(\sqrt{2}\) is irrational.
Demonstrating Even Nature of b
In the previous steps, we showed that if \( \sqrt{2} \) is rational, then we can write it as \( \frac{a}{b} \) where \( a \) and \( b \) are coprime integers (they have no common factors other than 1). We also derived the equation \( 2b^2 = a^2 \) and demonstrated that \( a \) must be even. Now, we will show that \( b \) must also be even, leading to a contradiction.
Since \( a \) is even, we can write \( a \) as \( 2k \) for some integer \( k \). Substituting this into our equation:
\[ 2b^2 = (2k)^2 \]
\[ 2b^2 = 4k^2 \]
\[ b^2 = 2k^2 \]
From the equation \( b^2 = 2k^2 \), we see that \( b^2 \) is also even because it is equal to \( 2 \) times \( k^2 \). Since \( b^2 \) is even, \( b \) must be even (the square of an odd number is always odd, and the square of an even number is always even).
Thus, we have shown that both \( a \) and \( b \) are even. This contradicts our initial assumption that \( a \) and \( b \) are coprime because if both are even, they share a common factor of 2. This contradiction implies that our original assumption that \( \sqrt{2} \) is rational is false.

Contradiction of Coprimality
To reach a contradiction in our proof, we need to consider the property of coprimality of \(a\) and \(b\). Recall that we assumed \( \sqrt{2} \) can be expressed as the simplified fraction \( \frac{a}{b} \), where \(a\) and \(b\) are coprime integers (i.e., their greatest common divisor is 1).
From our earlier steps, we have established the following:
- \(a\) must be even, so we can write \(a = 2k\) for some integer \(k\).
- Substituting \(a = 2k\) into the equation \(a^2 = 2b^2\), we get \( (2k)^2 = 2b^2 \), which simplifies to \( 4k^2 = 2b^2 \).
- Dividing both sides by 2, we obtain \( 2k^2 = b^2 \), indicating that \(b^2\) is also even, and therefore, \(b\) must be even.
At this point, we have shown that both \(a\) and \(b\) are even. This implies that both \(a\) and \(b\) share a common factor of 2.
However, this conclusion contradicts our initial assumption that \(a\) and \(b\) are coprime (having no common factors other than 1). If both \(a\) and \(b\) are even, they cannot be coprime.
Thus, our initial assumption that \( \sqrt{2} \) can be expressed as a fraction \( \frac{a}{b} \) where \(a\) and \(b\) are integers is flawed. Therefore, the only logical conclusion is that \( \sqrt{2} \) is irrational.
Conclusion: √2 is Irrational
Through the method of proof by contradiction, we have established that the square root of 2 is irrational. Here is a summary of the logical steps that lead to this conclusion:
-
We began by assuming the opposite of what we want to prove: that \(\sqrt{2}\) is rational. This means that we can write \(\sqrt{2}\) as a fraction of two integers \(a\) and \(b\) (where \(b \ne 0\)), in simplest form. Thus, \(\sqrt{2} = \frac{a}{b}\) with \(a\) and \(b\) being coprime integers.
-
We squared both sides of the equation to eliminate the square root: \(2 = \left(\frac{a}{b}\right)^2\), which simplifies to \(2b^2 = a^2\).
-
We analyzed the implications of the equation \(2b^2 = a^2\), showing that \(a^2\) is even because it is two times another integer (\(2b^2\)). Since \(a^2\) is even, \(a\) must also be even (because the square of an odd number is odd).
-
We expressed \(a\) as \(2k\) (for some integer \(k\)) since \(a\) is even. Substituting \(a = 2k\) into the equation \(2b^2 = a^2\), we get \(2b^2 = (2k)^2\), which simplifies to \(2b^2 = 4k^2\), and further simplifies to \(b^2 = 2k^2\).
-
This simplification shows that \(b^2\) is also even, and thus \(b\) must be even. However, this contradicts our initial assumption that \(a\) and \(b\) are coprime (i.e., they have no common factors other than 1), since both \(a\) and \(b\) being even means they share at least the common factor 2.
-
Since our assumption that \(\sqrt{2}\) is rational leads to a logical contradiction, we conclude that \(\sqrt{2}\) cannot be written as a fraction of two integers. Therefore, \(\sqrt{2}\) is irrational.
This elegant proof not only shows the irrationality of \(\sqrt{2}\) but also demonstrates the power of the proof by contradiction technique in mathematical reasoning.
Implications and Applications
The proof that \(\sqrt{2}\) is irrational has significant implications and applications across various fields of mathematics and science. Here, we explore some of the key impacts:
-
Number Theory: The irrationality of \(\sqrt{2}\) highlights the distinction between rational and irrational numbers. This distinction is fundamental in understanding the properties of the real number line and the distribution of numbers.
-
Mathematical Proofs: The proof by contradiction method used to show the irrationality of \(\sqrt{2}\) is a powerful technique in mathematics. It is widely applied in various proofs to demonstrate the impossibility or existence of certain mathematical statements.
-
Geometry: The discovery that \(\sqrt{2}\) is irrational has historical significance in geometry, particularly in the study of the diagonal of a square. This was one of the first known instances of an irrational number, leading to a deeper exploration of geometric properties and measurements.
-
Computational Mathematics: Understanding irrational numbers is crucial in computational mathematics, where approximations of irrational numbers are often used in algorithms and numerical methods. The irrationality of \(\sqrt{2}\) implies that its decimal expansion is non-repeating and non-terminating, requiring approximations for practical computations.
-
Cryptography: The properties of irrational numbers are utilized in cryptographic algorithms. The unpredictability and infinite nature of their decimal expansions can be leveraged to enhance the security of cryptographic systems.
-
Philosophical Implications: The proof of \(\sqrt{2}\)'s irrationality has philosophical implications regarding the nature of mathematical truth and the existence of numbers that cannot be expressed as ratios of integers. It challenges our understanding of numbers and their representations.
The irrationality of \(\sqrt{2}\) is a cornerstone result in mathematics, influencing numerous areas and inspiring further research into the nature of numbers and their applications.
Further Reading and Resources
To deepen your understanding of the proof by contradiction that the square root of 2 is irrational, and to explore further topics related to irrational numbers and mathematical proofs, consider the following resources:
-
Mathematics LibreTexts: This resource provides comprehensive coverage of mathematical principles, including detailed proofs and explanations. The section on proof by contradiction is particularly helpful for understanding the nuances of proving irrationality.
Visit:
-
Khan Academy: Known for its educational videos, Khan Academy offers clear and concise lessons on various mathematical concepts, including proofs and irrational numbers.
Visit:
-
ChiliMath: This site provides a step-by-step guide to understanding the proof that the square root of 2 is irrational, along with other mathematical topics.
Visit:
-
ProofWiki: An extensive resource for various mathematical proofs, including the irrationality of square roots and other numbers. It provides formalized and detailed proofs for those interested in rigorous mathematical reasoning.
Visit:
-
Coursera and edX: These platforms offer online courses from universities around the world, including courses on mathematical reasoning, proofs, and number theory.
Visit: |
These resources will provide you with a thorough understanding and additional insights into the proof of the irrationality of the square root of 2, as well as other related mathematical topics.
References
-
Stewart, Ian. The Story of Mathematics. London: Quercus, 2008. This book provides a comprehensive overview of the history of mathematics, including a discussion on the irrationality of the square root of 2.
-
Courant, Richard, and Herbert Robbins. What is Mathematics? An Elementary Approach to Ideas and Methods. Oxford: Oxford University Press, 1996. This text covers a range of mathematical topics and includes a proof by contradiction for the irrationality of √2.
-
Niven, Ivan. Numbers: Rational and Irrational. Washington, DC: Mathematical Association of America, 1961. This book offers a detailed examination of rational and irrational numbers, with a specific section on the proof that √2 is irrational.
-
Bryant, Victor. Yet Another Introduction to Analysis. Cambridge: Cambridge University Press, 1990. Provides a clear and detailed explanation of various mathematical proofs, including the irrationality of √2.
-
Simmons, George F. Calculus Gems: Brief Lives and Memorable Mathematics. New York: McGraw-Hill, 1992. This book combines biographical sketches of famous mathematicians with explanations of key mathematical concepts, including the proof of the irrationality of √2.
-
Jones, Alexander. "The Pythagorean Theorem and its Many Proofs." Mathematics Teacher, vol. 102, no. 6, 2008, pp. 431-437. An article that explores different proofs of the Pythagorean Theorem and its implications, including a discussion on the irrationality of √2.
-
Wikipedia Contributors. "Square root of 2." Wikipedia, The Free Encyclopedia, Wikimedia Foundation, . This entry provides an extensive overview of the square root of 2, its properties, and proofs of its irrationality.
Chứng Minh: Căn Bậc Hai Của 2 Là Số Vô Tỉ
READ MORE:
Chứng Minh Căn Bậc Hai Của 2 Là Số Vô Tỉ | Đại Số I | Khan Academy