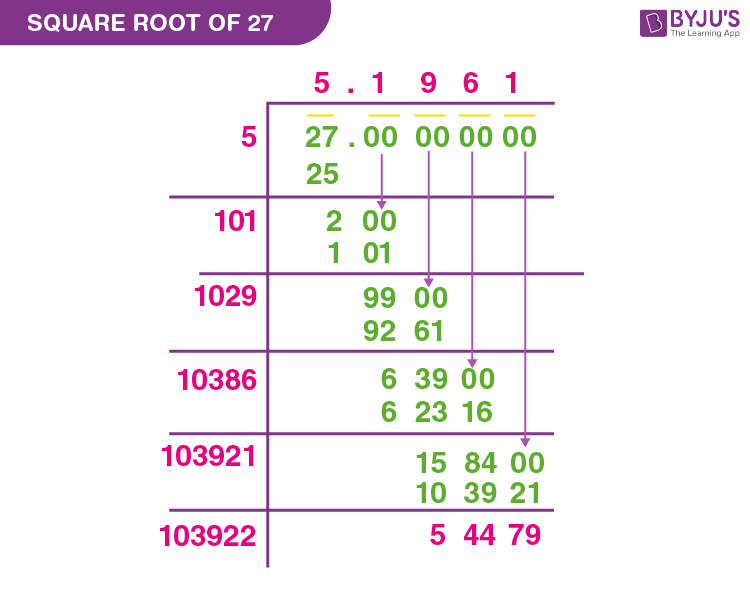
Topic proof of square root of 2 is irrational: Discover the fascinating proof that the square root of 2 is irrational. This article provides a clear and thorough explanation of the mathematical reasoning behind this classic proof, demonstrating why this fundamental concept is both intriguing and essential for understanding the nature of numbers. Dive in to explore the beauty of mathematics.
Table of Content
- Proof that the Square Root of 2 is Irrational
- Introduction
- Basic Definitions and Concepts
- Historical Context
- Rational and Irrational Numbers
- Proof by Contradiction: An Overview
- Detailed Steps of the Proof
- Assumption: Rationality of Square Root of 2
- Squaring the Assumption
- Analyzing the Even and Odd Properties
- Identifying the Contradiction
- Conclusion of the Proof
- Implications of the Proof
- Applications in Mathematics
- Common Misconceptions
- Related Proofs and Concepts
- Additional Resources and References
- YOUTUBE: Khám phá bằng chứng toán học rằng căn bậc hai của 2 là số vô tỉ, một kiến thức cơ bản và quan trọng trong toán học.
Proof that the Square Root of 2 is Irrational
The proof that the square root of 2 is irrational is a classic example of a proof by contradiction. Here, we will demonstrate this proof step by step.
Assumption
Assume, for the sake of contradiction, that the square root of 2 is rational. This means that we can express √2 as a fraction of two integers a and b (where a and b have no common factors other than 1).
So, we can write:
\[\sqrt{2} = \frac{a}{b}\]
Squaring Both Sides
Next, we square both sides of the equation to remove the square root:
\[\left(\sqrt{2}\right)^2 = \left(\frac{a}{b}\right)^2\]
This simplifies to:
\[2 = \frac{a^2}{b^2}\]
Rearranging the Equation
To get rid of the fraction, multiply both sides by \(b^2\):
\[2b^2 = a^2\]
This implies that \(a^2\) is even (since it is equal to \(2b^2\), which is clearly even).
Properties of Even and Odd Numbers
If \(a^2\) is even, then \(a\) must also be even (since the square of an odd number is odd). Therefore, we can write \(a\) as:
\[a = 2k\]
for some integer \(k\). Substituting \(a = 2k\) into the equation \(2b^2 = a^2\), we get:
\[2b^2 = (2k)^2\]
\[2b^2 = 4k^2\]
Dividing both sides by 2, we obtain:
\[b^2 = 2k^2\]
This implies that \(b^2\) is also even, and thus \(b\) must be even.
Contradiction
We have now shown that both \(a\) and \(b\) are even, which means that they have a common factor of 2. This contradicts our initial assumption that \(a\) and \(b\) have no common factors other than 1.
Conclusion
Since our assumption that \(\sqrt{2}\) is rational leads to a contradiction, we must conclude that the square root of 2 is irrational.

READ MORE:
Introduction
The proof that the square root of 2 is irrational is a cornerstone in the study of mathematics. This classic proof, often taught in introductory courses on number theory, showcases the power of logical reasoning and the concept of proof by contradiction. Understanding this proof not only deepens one's appreciation for mathematics but also enhances problem-solving skills and analytical thinking.
We will explore the proof in a detailed, step-by-step manner, ensuring clarity and comprehension. The proof relies on fundamental properties of rational and irrational numbers, and the technique of proof by contradiction, where we assume the opposite of what we want to prove and show that this leads to a contradiction.
The main steps of the proof are as follows:
- Assume that the square root of 2 is rational, meaning it can be expressed as a fraction a/b, where a and b are integers with no common factors other than 1.
- Square both sides of the equation to remove the square root, resulting in the equation \(2 = \frac{a^2}{b^2}\).
- Rearrange the equation to \(2b^2 = a^2\), demonstrating that \(a^2\) is an even number.
- Since \(a^2\) is even, \(a\) must also be even. Therefore, we can write \(a\) as \(2k\) for some integer \(k\).
- Substitute \(a = 2k\) into the equation \(2b^2 = a^2\), which simplifies to \(2b^2 = 4k^2\) or \(b^2 = 2k^2\).
- This implies that \(b^2\) is also even, meaning \(b\) is even as well.
- Since both \(a\) and \(b\) are even, they have a common factor of 2, which contradicts our initial assumption that \(a\) and \(b\) have no common factors other than 1.
By reaching this contradiction, we conclude that our initial assumption must be false, and therefore, the square root of 2 cannot be rational. This proof elegantly demonstrates the impossibility of expressing \(\sqrt{2}\) as a fraction, solidifying its place as a fundamental result in mathematics.
Basic Definitions and Concepts
Before delving into the proof that the square root of 2 is irrational, it is essential to understand some basic definitions and concepts in mathematics. These foundational ideas will provide the necessary background and context for the proof.
Rational Numbers
A rational number is any number that can be expressed as the quotient or fraction \(\frac{a}{b}\) of two integers, where \(a\) (the numerator) and \(b\) (the denominator) are integers and \(b \neq 0\). Rational numbers include integers, fractions, and terminating or repeating decimals.
- Examples of rational numbers: \( \frac{1}{2}, -\frac{3}{4}, 5, 0.75 \) (since 0.75 = \( \frac{3}{4} \)), \(0.333\ldots\) (since 0.333... = \( \frac{1}{3} \))
Irrational Numbers
An irrational number is a number that cannot be expressed as a simple fraction; in other words, it cannot be written as \(\frac{a}{b}\), where \(a\) and \(b\) are integers and \(b \neq 0\). Irrational numbers have non-terminating and non-repeating decimal expansions.
- Examples of irrational numbers: \(\pi, e, \sqrt{2}\)
Proof by Contradiction
Proof by contradiction is a logical method used to prove a statement by assuming the opposite of the statement and then showing that this assumption leads to a contradiction. Since the assumption leads to a contradiction, the original statement must be true.
- Assume the opposite of what you want to prove.
- Show that this assumption leads to a logical contradiction.
- Conclude that the original statement must be true because the opposite assumption is false.
Even and Odd Numbers
Understanding even and odd numbers is crucial for the proof:
- An even number is any integer that can be divided by 2 without a remainder. It can be written in the form \(2k\), where \(k\) is an integer.
- An odd number is any integer that cannot be divided by 2 without a remainder. It can be written in the form \(2k + 1\), where \(k\) is an integer.
These basic definitions and concepts are essential for understanding the proof that the square root of 2 is irrational. With this foundation, we can proceed to explore the proof in detail.
Historical Context
The discovery that the square root of 2 is irrational is a significant milestone in the history of mathematics, dating back to ancient Greece. This revelation challenged the Greeks' understanding of numbers and led to important developments in mathematical theory.
The Pythagoreans
The concept of irrational numbers was first encountered by the Pythagoreans, a group of ancient Greek mathematicians and philosophers led by Pythagoras. The Pythagoreans believed that all numbers could be expressed as the ratio of two integers, which fit their belief in the harmony and order of the universe.
Discovery of Irrationality
The discovery of irrational numbers is often attributed to Hippasus, a member of the Pythagorean school. While investigating the properties of geometric shapes, Hippasus found that the diagonal of a square with side length 1 could not be expressed as a ratio of two integers. This diagonal is the square root of 2.
The traditional account suggests that Hippasus' discovery was met with shock and disbelief by the Pythagoreans, as it contradicted their belief that all numbers are rational. According to legend, Hippasus was ostracized, and his findings were suppressed.
Geometric Interpretation
The irrationality of the square root of 2 can be understood geometrically. Consider a square with each side of length 1 unit. The length of the diagonal, according to the Pythagorean theorem, is given by:
\[
\text{Diagonal} = \sqrt{1^2 + 1^2} = \sqrt{2}
\]
Hippasus showed that this length could not be expressed as a fraction, indicating the existence of irrational numbers.
Impact on Mathematics
The discovery of irrational numbers had a profound impact on mathematics. It led to the development of more advanced mathematical concepts and a deeper understanding of number theory. The existence of irrational numbers demonstrated that the number system is more complex than previously thought and paved the way for further exploration of real numbers and their properties.
Legacy
Today, the proof of the irrationality of the square root of 2 is a fundamental part of mathematical education. It serves as an introduction to the concept of irrational numbers and the methods of proof by contradiction. The historical context of this discovery highlights the dynamic and evolving nature of mathematical knowledge, driven by curiosity and the pursuit of truth.
Rational and Irrational Numbers
Understanding the concepts of rational and irrational numbers is fundamental to grasping the proof that the square root of 2 is irrational. These concepts form the basis of number theory and are essential for distinguishing between different types of numbers.
Rational Numbers
A rational number is any number that can be expressed as the quotient or fraction \(\frac{a}{b}\) of two integers, where \(a\) (the numerator) and \(b\) (the denominator) are integers and \(b \neq 0\). Rational numbers can be positive, negative, or zero and include both integers and fractions.
Properties of rational numbers include:
- They can be written as a simple fraction \(\frac{a}{b}\).
- The decimal representation of a rational number either terminates or repeats periodically.
- Examples of rational numbers: \( \frac{1}{2}, -\frac{3}{4}, 5, 0.75 \) (since \(0.75 = \frac{3}{4}\)), \(0.333\ldots\) (since \(0.333\ldots = \frac{1}{3}\)).
Irrational Numbers
An irrational number is a number that cannot be expressed as a fraction \(\frac{a}{b}\), where \(a\) and \(b\) are integers and \(b \neq 0\). Irrational numbers have non-terminating and non-repeating decimal expansions, which means their decimal forms go on forever without repeating a pattern.
Properties of irrational numbers include:
- They cannot be written as a simple fraction.
- Their decimal representations are non-terminating and non-repeating.
- Examples of irrational numbers: \(\pi, e, \sqrt{2}, \sqrt{3}\).
Distinguishing Between Rational and Irrational Numbers
To determine whether a number is rational or irrational, consider the following steps:
- Check if the number can be written as a fraction \(\frac{a}{b}\), where \(a\) and \(b\) are integers and \(b \neq 0\). If it can, it is rational.
- If the decimal representation terminates (e.g., 0.5) or repeats (e.g., 0.333...), it is rational.
- If the decimal representation neither terminates nor repeats, the number is irrational.
Importance in Mathematics
The distinction between rational and irrational numbers is crucial in mathematics because it helps in understanding the structure of the number system. Rational numbers are well-behaved and can be easily manipulated using arithmetic operations. Irrational numbers, on the other hand, require more sophisticated techniques to handle and understand. Together, rational and irrational numbers make up the real numbers, providing a complete picture of continuous quantities.
The discovery that \(\sqrt{2}\) is irrational was a groundbreaking moment in mathematics, revealing the existence of numbers that could not be expressed as fractions and prompting deeper exploration into the nature of numbers and their properties.

Proof by Contradiction: An Overview
Proof by contradiction is a powerful and widely used technique in mathematics. This method involves assuming the opposite of what you want to prove and then demonstrating that this assumption leads to a contradiction. The contradiction implies that the original assumption must be false, thereby proving the desired statement. This approach is particularly useful when direct proof is challenging.
Steps in a Proof by Contradiction
The general structure of a proof by contradiction involves the following steps:
- Assume the Negation: Assume that the statement you want to prove is false. This assumption is known as the negation of the statement.
- Develop Logical Consequences: Using logical reasoning and known facts, develop the consequences of this assumption. This often involves algebraic manipulation, applying definitions, and leveraging other mathematical properties.
- Identify a Contradiction: Show that these consequences lead to a contradiction, either with the initial assumption, with known facts, or with established mathematical principles.
- Conclude the Proof: Since the assumption leads to a contradiction, conclude that the original statement must be true.
Example: Proving the Irrationality of \(\sqrt{2}\)
To illustrate proof by contradiction, let's outline the proof that \(\sqrt{2}\) is irrational:
- Assume the Opposite: Assume that \(\sqrt{2}\) is rational. This means there exist integers \(a\) and \(b\) (with no common factors other than 1) such that \(\sqrt{2} = \frac{a}{b}\).
- Square Both Sides: Squaring both sides of the equation gives \(2 = \frac{a^2}{b^2}\), which simplifies to \(2b^2 = a^2\).
- Analyze Parity: From \(2b^2 = a^2\), we see that \(a^2\) must be even (since it equals \(2b^2\), which is even). Hence, \(a\) must be even (the square of an odd number is odd). Let \(a = 2k\) for some integer \(k\).
- Substitute and Simplify: Substitute \(a = 2k\) into the equation \(2b^2 = a^2\) to get \(2b^2 = (2k)^2\), which simplifies to \(2b^2 = 4k^2\) or \(b^2 = 2k^2\).
- Analyze Parity Again: This shows that \(b^2\) is also even, so \(b\) must be even as well.
- Contradiction: Since both \(a\) and \(b\) are even, they share a common factor of 2, contradicting the assumption that \(a\) and \(b\) have no common factors other than 1.
By reaching a contradiction, we conclude that the assumption that \(\sqrt{2}\) is rational is false. Therefore, \(\sqrt{2}\) must be irrational. This elegant proof highlights the effectiveness of proof by contradiction in establishing mathematical truths.
Detailed Steps of the Proof
The proof that the square root of 2 is irrational is typically done by contradiction. This method assumes the opposite of what we want to prove and then shows that this assumption leads to a logical contradiction. Here are the detailed steps:
Assume that \(\sqrt{2}\) is a rational number. By definition, a rational number can be expressed as the ratio of two integers \(a\) and \(b\) (where \(a\) and \(b\) have no common factors other than 1), so we can write:
\[
\sqrt{2} = \frac{a}{b}
\]Square both sides of the equation to remove the square root:
\[
2 = \left(\frac{a}{b}\right)^2
\]which simplifies to:
\[
2 = \frac{a^2}{b^2}
\]Multiply both sides of the equation by \(b^2\) to get rid of the fraction:
\[
2b^2 = a^2
\]From this equation, we see that \(a^2\) is an even number (since it is equal to \(2b^2\), and \(2b^2\) is clearly even because it is 2 times some integer). Since \(a^2\) is even, \(a\) must also be even (because the square of an odd number is odd).
Since \(a\) is even, we can write \(a\) as:
\[
a = 2k
\]for some integer \(k\).
Substitute \(a = 2k\) back into the equation \(2b^2 = a^2\):
\[
2b^2 = (2k)^2
\]which simplifies to:
\[
2b^2 = 4k^2
\]Divide both sides by 2:
\[
b^2 = 2k^2
\]From this, we see that \(b^2\) is also even (since it is equal to \(2k^2\)). Since \(b^2\) is even, \(b\) must also be even.
Now we have shown that both \(a\) and \(b\) are even. This means that both \(a\) and \(b\) have at least 2 as a common factor. This contradicts our initial assumption that \(a\) and \(b\) have no common factors other than 1.
Therefore, the assumption that \(\sqrt{2}\) is rational must be false. Hence, \(\sqrt{2}\) is irrational.
Assumption: Rationality of Square Root of 2
To prove that the square root of 2 is irrational, we start by assuming the contrary, that √2 is a rational number. This means it can be expressed as a fraction of two integers \( \frac{p}{q} \), where \( p \) and \( q \) have no common factors other than 1, and \( q \neq 0 \).
- Assume \( \sqrt{2} = \frac{p}{q} \), where \( p \) and \( q \) are coprime integers.
- Square both sides of the equation:
\[ \sqrt{2} = \frac{p}{q} \implies 2 = \frac{p^2}{q^2} \]
- Multiply both sides by \( q^2 \) to clear the denominator:
\[ 2q^2 = p^2 \]
- Since \( p^2 = 2q^2 \), \( p^2 \) is even (because it is two times an integer). Hence, \( p \) must also be even.
- Let \( p = 2k \) for some integer \( k \), substituting into the equation gives:
\[ 2q^2 = (2k)^2 \implies 2q^2 = 4k^2 \implies q^2 = 2k^2 \]
- Therefore, \( q^2 \) is also even, which implies \( q \) is even.
- Since both \( p \) and \( q \) are even, they have a common factor of 2. This contradicts our initial assumption that \( \frac{p}{q} \) is in its simplest form.
This contradiction means our original assumption that \( \sqrt{2} \) is rational must be false. Therefore, \( \sqrt{2} \) is irrational.
Squaring the Assumption
To prove that the square root of 2 is irrational, we begin by assuming the opposite: that it is rational. This means we can express the square root of 2 as a ratio of two integers \( \frac{a}{b} \) in its simplest form, where \( a \) and \( b \) are coprime integers (i.e., their greatest common divisor is 1), and \( b \neq 0 \).
Let's denote the square root of 2 as \( \sqrt{2} \). By our assumption, we can write:
\[
\sqrt{2} = \frac{a}{b}
\]
Next, we square both sides of the equation to remove the square root:
\[
(\sqrt{2})^2 = \left(\frac{a}{b}\right)^2
\]
This simplifies to:
\[
2 = \frac{a^2}{b^2}
\]
By multiplying both sides of the equation by \( b^2 \) to clear the fraction, we get:
\[
2b^2 = a^2
\]
At this point, we have shown that \( a^2 \) is equal to \( 2b^2 \). This equation implies that \( a^2 \) is an even number because it is two times another integer (\( b^2 \)). Since \( a^2 \) is even, \( a \) must also be even (the square of an odd number is odd).
Let \( a \) be expressed as \( 2k \) for some integer \( k \). Substituting \( 2k \) for \( a \) in the equation \( 2b^2 = a^2 \) gives us:
\[
2b^2 = (2k)^2
\]
Simplifying this, we get:
\[
2b^2 = 4k^2
\]
Dividing both sides of the equation by 2, we obtain:
\[
b^2 = 2k^2
\]
This equation indicates that \( b^2 \) is also even, and consequently, \( b \) must be even as well.
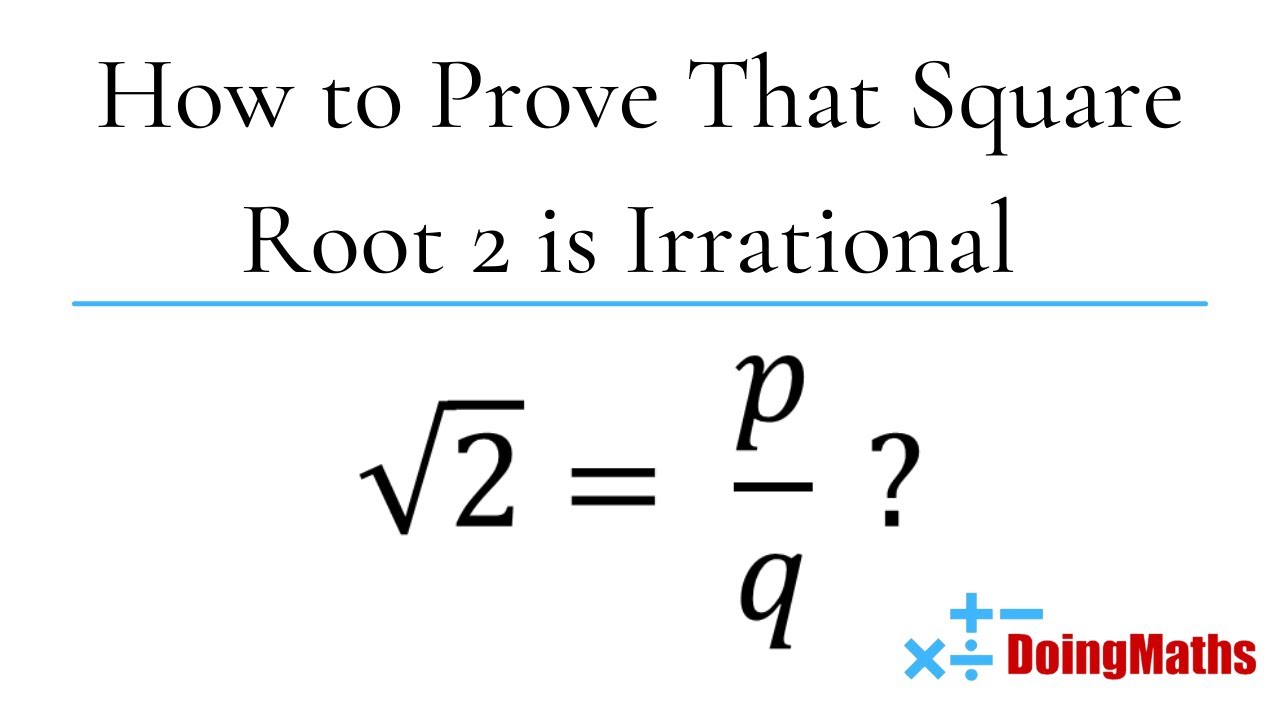
Analyzing the Even and Odd Properties
In this section, we will analyze the even and odd properties of the numbers involved in our proof to identify the contradiction that proves the square root of 2 is irrational.
Recall our equation from the previous section:
\( a^2 = 2b^2 \)
To proceed, consider the following steps:
- Assume \(a^2\) is an even number:
Since \( a^2 = 2b^2 \), \( a^2 \) is twice an integer (\( 2b^2 \)), which means \( a^2 \) must be an even number. This follows because any number multiplied by 2 is even.
- Determine the parity of \(a\):
Since \( a^2 \) is even, \( a \) itself must also be even. If \( a \) were odd, then \( a^2 \) would be odd (since the square of an odd number is odd), contradicting our earlier conclusion. Hence, we can write:
\( a = 2k \)
for some integer \( k \).
- Substitute \( a = 2k \) into the equation:
Replace \( a \) in the original equation \( a^2 = 2b^2 \):
\( (2k)^2 = 2b^2 \)
which simplifies to:
\( 4k^2 = 2b^2 \)
Dividing both sides by 2, we get:
\( 2k^2 = b^2 \)
- Determine the parity of \(b\):
This equation \( b^2 = 2k^2 \) implies that \( b^2 \) is also even (as it is twice some integer \( k^2 \)). Therefore, \( b \) must also be even.
- Identify the contradiction:
Since both \( a \) and \( b \) are even, they have a common factor of 2. This contradicts our initial assumption that \( a \) and \( b \) are coprime (i.e., their greatest common divisor is 1). Thus, our initial assumption that \( \sqrt{2} \) is rational must be false.
Therefore, we conclude that \( \sqrt{2} \) is irrational, as assuming it is rational leads to a contradiction.
Identifying the Contradiction
We have assumed that the square root of 2 (\(\sqrt{2}\)) is a rational number, expressed in its simplest form as \(\frac{p}{q}\) where \(p\) and \(q\) are coprime integers (i.e., they have no common factors other than 1).
- From our previous steps, we arrived at the equation \(2q^2 = p^2\).
- We determined that since \(p^2\) is even, \(p\) must also be even. Therefore, we can write \(p = 2k\) for some integer \(k\).
- Substituting \(p = 2k\) into the equation \(2q^2 = p^2\) gives:
- \(2q^2 = (2k)^2\)
- \(2q^2 = 4k^2\)
- Dividing both sides by 2, we get \(q^2 = 2k^2\).
- This shows that \(q^2\) is also even, which means that \(q\) must be even. Therefore, \(q\) can be written as \(q = 2m\) for some integer \(m\).
- Since both \(p\) and \(q\) are even, they have a common factor of 2. This contradicts our initial assumption that \(p\) and \(q\) have no common factors other than 1 (i.e., they are coprime).
This contradiction implies that our initial assumption that \(\sqrt{2}\) is rational is false. Therefore, \(\sqrt{2}\) must be irrational.
Conclusion of the Proof
We began by assuming that the square root of 2 (\(\sqrt{2}\)) is a rational number. This allowed us to write it in the form \(\frac{a}{b}\), where \(a\) and \(b\) are coprime integers (i.e., their greatest common divisor is 1).
Following this assumption, we squared both sides of the equation, leading to:
\[ \left(\frac{a}{b}\right)^2 = 2 \]
Which simplifies to:
\[ \frac{a^2}{b^2} = 2 \]
Multiplying both sides by \(b^2\) gives us:
\[ a^2 = 2b^2 \]
This equation shows that \(a^2\) is an even number because it is equal to 2 times \(b^2\), an integer. Since \(a^2\) is even, \(a\) must also be even (because the square of an odd number is odd).
Since \(a\) is even, we can write \(a\) as \(2k\) for some integer \(k\). Substituting \(2k\) for \(a\) in the equation \(a^2 = 2b^2\), we get:
\[ (2k)^2 = 2b^2 \]
Which simplifies to:
\[ 4k^2 = 2b^2 \]
Dividing both sides by 2 gives us:
\[ 2k^2 = b^2 \]
This equation shows that \(b^2\) is also even, which means \(b\) must be even as well.
We have now shown that both \(a\) and \(b\) are even, which contradicts our initial assumption that \(a\) and \(b\) are coprime (since they both share at least the factor 2).
Therefore, our initial assumption that \(\sqrt{2}\) is rational must be false. Thus, we conclude that \(\sqrt{2}\) is irrational.
Implications of the Proof
The proof that the square root of 2 is irrational has profound implications across various fields of mathematics and beyond. Here are some key areas impacted by this proof:
- Foundation for Algebra: This proof establishes the existence of irrational numbers, enriching our understanding of the number system. It shows that not all numbers can be expressed as fractions, which is a fundamental concept in algebra and analysis.
- Philosophical Implications: The discovery challenges our intuition about numbers and the nature of infinity. It demonstrates that some quantities cannot be neatly categorized, prompting deeper philosophical inquiries into the nature of mathematics and reality.
- Influence on Number Theory: The proof has led to significant advancements in number theory. It highlights the complexity of numbers and inspires further exploration of the properties of irrational numbers and their roles within mathematics.
- Application in Geometry: The irrationality of the square root of 2 has practical implications in geometry. For instance, it explains the incommensurability of the diagonal of a square with its side, a crucial concept in understanding geometric relationships.
- Impact on Mathematics Education: This proof is often used in educational settings to teach the principles of proof by contradiction. It serves as an excellent example of mathematical reasoning and helps students develop critical thinking skills.
Thus, the proof that the square root of 2 is irrational extends well beyond a mere mathematical curiosity. It influences various domains of mathematics, philosophy, and education, encouraging us to delve deeper into the mysteries of numbers and their properties.

Applications in Mathematics
The proof of the irrationality of the square root of 2 has significant implications and applications in various areas of mathematics:
-
Number Theory:
The proof serves as a foundational example of an irrational number, leading to a deeper understanding of the properties of numbers. It highlights the existence of numbers that cannot be expressed as fractions, broadening the scope of number theory beyond rational numbers.
-
Algebra:
In algebra, this proof is often used to introduce the concept of irrational numbers and their properties. It is a critical step in understanding the field of real numbers and the structure of algebraic numbers.
-
Analysis:
The irrationality of \(\sqrt{2}\) is essential in real analysis, particularly in the study of limits, continuity, and the uncountability of the real numbers. It provides an early example of how not all real numbers are rational, which is crucial for understanding the completeness of the real number line.
-
Geometry:
In geometry, the proof is linked to the Pythagorean theorem. The discovery of the irrationality of \(\sqrt{2}\) is attributed to the Pythagoreans, arising from the diagonal of a unit square, which cannot be measured exactly by any fraction.
-
Cryptography:
Irrational numbers play a role in modern cryptography. Algorithms often rely on properties of numbers, including their irrationality, to ensure security. Understanding irrational numbers helps in developing encryption methods that are difficult to break.
-
Computational Mathematics:
In computational mathematics, the concept of irrationality is crucial for numerical methods and algorithms. It affects how we approximate and compute values to a required degree of accuracy, impacting fields such as numerical analysis and computer science.
Common Misconceptions
When discussing the proof that the square root of 2 is irrational, several misconceptions frequently arise. This section aims to clarify these misunderstandings and provide accurate insights into this fundamental mathematical truth.
-
Misconception: Irrational Means Not Real
Some may confuse "irrational" with "imaginary" or "not real." However, irrational numbers are very much real; they simply cannot be expressed as a fraction of two integers. The square root of 2 is a real number that cannot be written as a ratio of two integers.
-
Misconception: The Proof is Merely Theoretical
While the proof is abstract, it has practical implications, influencing how we understand numbers and their properties in both theoretical and applied mathematics. It demonstrates a fundamental property of numbers that has real-world applications, particularly in geometry and algebra.
-
Misconception: All Square Roots are Irrational
Not all square roots are irrational. For instance, the square roots of perfect squares like 1, 4, 9, 16, etc., are rational. The irrationality is specific to the square roots of non-square numbers, like 2, which cannot be exactly expressed as fractions.
-
Misconception: Irrational Numbers are Uncommon
Many people think irrational numbers are rare, but they are actually abundant. Between any two rational numbers, there are infinitely many irrational numbers. They are an essential part of the real number line.
Understanding these common misconceptions helps in grasping the importance and implications of the proof that the square root of 2 is irrational. It highlights the depth and complexity of mathematical concepts and their significance in various fields.
Related Proofs and Concepts
The proof of the irrationality of the square root of 2 is a classic example of a proof by contradiction. This method of proof can be applied to other numbers and concepts. Here are some related proofs and mathematical concepts that build on or relate to the proof of the irrationality of the square root of 2:
1. Proof of the Irrationality of Other Square Roots
Similar to the proof for the square root of 2, we can prove the irrationality of other square roots.
- Square Root of 3: The proof for the square root of 3 follows a similar structure to the proof for the square root of 2. Assume that √3 is rational, express it as a fraction p/q in its lowest terms, and derive a contradiction.
- Square Root of 5: Using the same method of proof by contradiction, assume that √5 is rational, and show that this assumption leads to a logical inconsistency.
2. Proof of the Irrationality of Certain Cubic Roots
The method can also extend to certain cubic roots, such as the cubic root of non-perfect cubes.
- Cubic Root of 2: Assume that ∛2 is rational, express it as p/q where p and q are integers with no common factors, and show that this leads to a contradiction.
3. Rational and Irrational Numbers
Understanding the nature of rational and irrational numbers is crucial in these proofs.
- Rational Numbers: Numbers that can be expressed as a fraction a/b, where a and b are integers and b ≠ 0.
- Irrational Numbers: Numbers that cannot be expressed as a simple fraction. Their decimal expansions are non-repeating and non-terminating.
4. Proofs of Irrationality Using Other Methods
There are various methods to prove the irrationality of numbers apart from contradiction.
- Geometric Proofs: Some irrationality proofs use geometric arguments, such as the proof for the irrationality of the golden ratio.
- Continued Fractions: Proving irrationality using the properties of continued fractions.
5. Transcendental Numbers
Beyond irrational numbers, there are transcendental numbers which are not roots of any non-zero polynomial equation with rational coefficients.
- π (Pi): Proven to be transcendental by Ferdinand von Lindemann in 1882.
- e (Euler's Number): Proven to be transcendental by Charles Hermite in 1873.
6. Applications and Implications
The proof of irrationality has significant implications in various fields of mathematics.
- Number Theory: Understanding the properties of numbers and their distributions.
- Algebra: Studying the roots of polynomials and their properties.
- Real Analysis: The study of real numbers and functions, which often involves irrational numbers.
These related proofs and concepts illustrate the breadth and depth of mathematics stemming from the proof of the irrationality of the square root of 2. They highlight the importance of understanding rational and irrational numbers and the various methods used to explore their properties.
Additional Resources and References
To further understand the proof of the irrationality of the square root of 2 and explore related mathematical concepts, the following resources and references can be highly beneficial:
-
This video tutorial provides a step-by-step explanation of the classic proof by contradiction, demonstrating why the square root of 2 cannot be expressed as a rational number.
-
A detailed written guide that breaks down each step of the proof, complete with algebraic manipulations and logical deductions.
-
This page provides a comprehensive description of the historical context and the mathematical steps involved in the proof, along with references to classical sources.
-
An overview of irrational numbers, including the proof of the irrationality of √2 and other famous irrational numbers.
-
A student-friendly resource that explains the proof using clear diagrams and examples, ideal for learners at all levels.
These resources provide various approaches and perspectives on the proof, catering to different learning styles and levels of mathematical background. They are excellent for deepening your understanding and exploring the wider implications of irrational numbers in mathematics.

Khám phá bằng chứng toán học rằng căn bậc hai của 2 là số vô tỉ, một kiến thức cơ bản và quan trọng trong toán học.
Bằng Chứng: Căn Bậc Hai của 2 là Số Vô Tỉ
READ MORE:
Tìm hiểu cách chứng minh rằng căn bậc hai của 2 là số vô tỉ trong bài giảng Đại Số I của Khan Academy.
Bằng Chứng Rằng Căn Bậc Hai của 2 là Số Vô Tỉ | Đại Số I | Khan Academy