Proof of Square Root of 3 is Irrational: An In-Depth Exploration

We will use a proof by contradiction to show that the square root of 3 is irrational.

Assumption

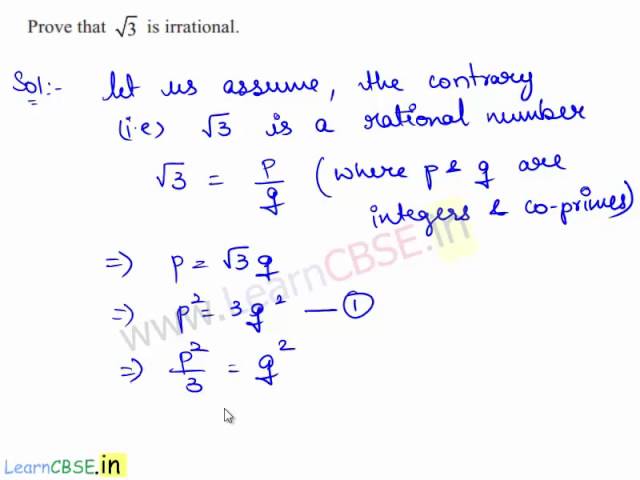
Assume, for the sake of contradiction, that $$

3

is a rational number. This means it can be expressed as a fraction of two integers in simplest form:

$$

3

=

a
b

where $$
a
and $$
b
are coprime integers (i.e., their greatest common divisor is 1).

Deriving a Contradiction

Squaring both sides of the equation, we get:

$$

3

=

a
b

→

a
b


)
2

=
3

This implies:

$$
a

)
2

=
3

b
2

Therefore, $$
a

)
2

is a multiple of 3. Thus, $$
a
must also be a multiple of 3 (since 3 is a prime number).

Let $$
a
=
3
k
for some integer $$
k
. Substituting this back into the equation, we get:

$$

3
k


)
2

=
3

b
2

which simplifies to:

$$
9

k
2

=
3

b
2

Dividing both sides by 3, we obtain:

$$
3

k
2

=

b
2

This implies that $$

b
2

is a multiple of 3, so $$
b
must also be a multiple of 3.

Therefore, both $$
a
and $$
b
are multiples of 3, which contradicts the assumption that $$
a
and $$
b
are coprime.

Conclusion

Since our initial assumption led to a contradiction, we conclude that $$

3

is irrational.

Irrational numbers are a fundamental concept in mathematics, representing numbers that cannot be expressed as a simple fraction of two integers. These numbers have non-repeating, non-terminating decimal expansions. Understanding irrational numbers is crucial for various mathematical theories and applications.

The discovery of irrational numbers dates back to ancient Greece, with the famous example of the square root of 2. Here, we focus on another notable irrational number: the square root of 3.

Characteristics of irrational numbers include:

• Non-repeating decimal expansions
• Non-terminating decimal expansions
• Cannot be expressed as a ratio of two integers

Let's delve into the mathematical proof demonstrating that the square root of 3 is irrational, highlighting the elegance and rigor of mathematical reasoning.

First, let's recall the definition of a rational number. A rational number can be written in the form $$

p
q

where $$
p
and $$
q
are integers and $$
q
is not zero. Irrational numbers, on the other hand, cannot be expressed in this form.

The proof by contradiction is a common method to show the irrationality of numbers like $$

3

. The key idea is to assume the opposite, that the number is rational, and then show that this assumption leads to a contradiction.

By understanding irrational numbers, we appreciate the richness of the number system and the intricate structures within mathematics. In the following sections, we will explore the detailed proof that demonstrates the irrationality of the square root of 3.

Rational and irrational numbers are two fundamental categories within the real number system. Grasping the differences between them is essential for a deeper understanding of mathematics.

Rational Numbers: Rational numbers are numbers that can be expressed as the quotient or fraction $$

p
q

where $$
p
and $$
q
are integers and $$
q
is not zero. Examples include:

• $1/2$
• $-3/4$
• $7$

Rational numbers have either terminating or repeating decimal expansions. For example:

• $\frac{1}{4}$ = 0.25 (terminating decimal)
• $\frac{1}{3}$ = 0.333... (repeating decimal)

Irrational Numbers: Irrational numbers cannot be expressed as a simple fraction. Their decimal expansions are non-terminating and non-repeating. Examples include:

• $\sqrt{2}$
• $\pi$
• $e$

To illustrate, the decimal expansion of $$

2

is approximately 1.4142135..., and it neither terminates nor repeats.

Why it Matters: Understanding the distinction between rational and irrational numbers helps in comprehending the structure of the number system. It also aids in various applications in science, engineering, and beyond.

In the context of this discussion, we are particularly interested in proving the irrationality of $$

3

. This proof not only demonstrates a specific property of the number but also exemplifies the elegance and rigor of mathematical reasoning.

In the next section, we will delve into the historical context of irrational numbers, setting the stage for our detailed exploration of the proof that $$

3

is indeed irrational.

The concept of irrational numbers has a rich history that dates back to ancient civilizations. Understanding this historical context provides valuable insights into the development of mathematical thought and the significance of proving the irrationality of numbers like the square root of 3.

Ancient Greece: The discovery of irrational numbers is often attributed to the ancient Greeks. The Pythagoreans, a group of mathematicians led by Pythagoras, initially believed that all numbers could be expressed as ratios of integers. This belief was shattered when they discovered the irrationality of the square root of 2 while studying the diagonal of a square.

Legend has it that Hippasus, a member of the Pythagorean school, demonstrated that $$

2

could not be expressed as a ratio of two integers. This discovery was so shocking and contrary to Pythagorean beliefs that, according to some accounts, Hippasus was ostracized or even drowned.

Advancements in Understanding: Despite the initial shock, the discovery of irrational numbers led to significant advancements in mathematics. Greek mathematicians began to explore other irrational quantities, laying the groundwork for future discoveries. Euclid’s "Elements," a foundational mathematical text, includes proofs about irrational numbers, showcasing their acceptance and study in classical mathematics.

Medieval and Renaissance Periods: During the medieval period, the study of irrational numbers continued in the Islamic world and later in Europe. Mathematicians such as Al-Khwarizmi and Fibonacci contributed to the understanding and notation of irrational numbers. The Renaissance period saw further advancements with mathematicians like Descartes and Newton expanding on the concepts of irrationality within their broader mathematical frameworks.

Modern Mathematics: In modern times, the formal definition and rigorous treatment of irrational numbers were established through the development of real analysis. Mathematicians such as Dedekind and Cantor provided a solid foundation for understanding the continuum of real numbers, distinguishing clearly between rational and irrational numbers.

Today, irrational numbers are a well-integrated part of mathematical theory, with proofs such as the irrationality of $$

3

serving as key examples of mathematical elegance and rigor.

Understanding the historical context of irrational numbers enhances our appreciation of the journey mathematical concepts undergo from discovery to acceptance. It also underscores the importance of proofs in establishing the properties and nature of numbers, contributing to the broader understanding of mathematics as a whole.

Before diving into the proof that the square root of 3 is irrational, it is essential to understand some key definitions and preliminary concepts that form the foundation of this proof.

Rational Numbers: A rational number is any number that can be expressed as the quotient or fraction $$

p
q

, where $$
p
and $$
q
are integers and $$
q
is not zero. Rational numbers have either terminating or repeating decimal expansions.

Irrational Numbers: An irrational number is a number that cannot be expressed as a simple fraction. Its decimal expansion is non-terminating and non-repeating. Examples include $$
π
, $$
e
, and $$

2

.

Fundamental Theorem of Arithmetic: This theorem states that every integer greater than 1 is either a prime number or can be uniquely factored into prime numbers. This property is crucial when discussing the rationality or irrationality of numbers.

Prime Numbers: A prime number is a natural number greater than 1 that has no positive divisors other than 1 and itself. Examples of prime numbers include 2, 3, 5, 7, and 11. Prime numbers play a significant role in proofs involving irrational numbers.

Let's outline the preliminary steps needed to prove that the square root of 3 is irrational:

1. Assume Rationality: Start by assuming, for the sake of contradiction, that $\sqrt{3}$ is rational. This means it can be written as $\frac{a}{b}$, where $a$ and $b$ are coprime integers (i.e., their greatest common divisor is 1).
2. Square Both Sides: By squaring both sides of the equation $\sqrt{3}=\frac{a}{b}$, we obtain $3=\frac{a^2}{b^2}$.
3. Analyze the Equation: This implies that $3b^2=a^2$, suggesting that $a^2$ is a multiple of 3.
4. Prime Factor Implication: If $a^2$ is a multiple of 3, then $a$ itself must be a multiple of 3. Let $a=3k$ for some integer $k$.
5. Substitute and Simplify: Substitute $a=3k$ back into the equation to get $3b^2=9k^2$, which simplifies to $b^2=3k^2$. This implies that $b^2$ is also a multiple of 3, meaning $b$ is a multiple of 3.
6. Contradiction: This contradicts the initial assumption that $a$ and $b$ are coprime.

These definitions and preliminaries set the stage for the rigorous proof that the square root of 3 is irrational. By understanding these foundational concepts, we can appreciate the elegance and precision of mathematical reasoning.

To prove that the square root of 3 (√3) is irrational, we use the method of assumption and contradiction. This method involves assuming the opposite of what we want to prove and then showing that this assumption leads to a contradiction.

Assume that √3 is rational. This means it can be expressed as the ratio of two integers a and b, where a and b have no common factors (i.e., they are coprime). Thus, we can write:

√3 = a / b

where a and b are integers with no common factors other than 1, and b ≠ 0.

Next, we square both sides of the equation to eliminate the square root:

3 = a² / b²

Multiplying both sides by b² gives:

3b² = a²

This implies that a² is divisible by 3. For this to be true, a must also be divisible by 3 (since 3 is a prime number and must appear squared in the prime factorization of a²). Therefore, we can write:

a = 3k

for some integer k.

Substituting a = 3k into the equation 3b² = a², we get:

3b² = (3k)²

3b² = 9k²

Dividing both sides by 3 gives:

b² = 3k²

This implies that b² is also divisible by 3, and therefore b must be divisible by 3.

Now, we have shown that both a and b are divisible by 3. However, this contradicts our original assumption that a and b have no common factors other than 1. Therefore, our initial assumption that √3 is rational must be false.

Hence, we conclude that the square root of 3 is irrational.

To prove that √3 is irrational, we use the method of contradiction. Assume, for the sake of contradiction, that √3 is a rational number. This means that it can be expressed as the ratio of two integers p and q (in lowest terms), such that:

\[ \sqrt{3} = \frac{p}{q} \]
where \( p \) and \( q \) are coprime integers (i.e., their greatest common divisor is 1).

Square both sides of the equation to remove the square root:

\[ 3 = \frac{p^2}{q^2} \]

By multiplying both sides by \( q^2 \), we get:

\[ p^2 = 3q^2 \]

This implies that \( p^2 \) is divisible by 3. Therefore, \( p \) must also be divisible by 3 (because if a prime divides the square of a number, it must divide the number itself).

Let's write \( p \) as \( 3k \) for some integer \( k \):

\[ p = 3k \]

Substitute \( p \) in the earlier equation:

\[ (3k)^2 = 3q^2 \]
\[ 9k^2 = 3q^2 \]
\[ 3k^2 = q^2 \]

This implies that \( q^2 \) is also divisible by 3, and hence \( q \) must be divisible by 3.

Now, we have shown that both \( p \) and \( q \) are divisible by 3, which contradicts our initial assumption that \( p \) and \( q \) are coprime (since their greatest common divisor would be at least 3, not 1).

Therefore, our assumption that √3 is a rational number must be false. Thus, we conclude that √3 is irrational.

The proof that the square root of 3 is irrational is done by contradiction. We assume the opposite of what we want to prove and show that this assumption leads to a contradiction.

1. Assume that \( \sqrt{3} \) is rational. This means that it can be expressed as a fraction of two integers \( \frac{a}{b} \) in simplest form, where \( a \) and \( b \) have no common factors other than 1, and \( b \neq 0 \). Thus, we can write:

   \[
   \sqrt{3} = \frac{a}{b}
   \]

2. Square both sides of the equation to remove the square root:

   \[
   3 = \frac{a^2}{b^2}
   \]

   Multiply both sides by \( b^2 \) to get rid of the denominator:

   \[
   3b^2 = a^2
   \]

3. This equation shows that \( a^2 \) is divisible by 3. Therefore, \( a \) must also be divisible by 3 (because the square of a number not divisible by 3 cannot be divisible by 3). Let \( a = 3k \) for some integer \( k \).

4. Substitute \( a = 3k \) into the equation \( 3b^2 = a^2 \):

   \[
   3b^2 = (3k)^2
   \]

   Simplify the equation:

   \[
   3b^2 = 9k^2
   \]

   Divide both sides by 3:

   \[
   b^2 = 3k^2
   \]

5. This equation shows that \( b^2 \) is also divisible by 3, and thus \( b \) must be divisible by 3.

6. Since both \( a \) and \( b \) are divisible by 3, this contradicts the assumption that \( \frac{a}{b} \) is in its simplest form (where \( a \) and \( b \) have no common factors other than 1).

7. Therefore, the assumption that \( \sqrt{3} \) is rational must be false. Hence, \( \sqrt{3} \) is irrational.

In the proof that the square root of 3 is irrational, several key theorems and lemmas play a crucial role. Below are the essential mathematical tools used in the proof:

• Fundamental Theorem of Arithmetic: Every integer greater than 1 is either a prime number or can be factorized as a unique product of prime numbers. This theorem ensures that the prime factorization of any integer is unique, which is vital for the contradiction in the proof.
• Properties of Rational Numbers: A rational number can be expressed as a fraction \( \frac{p}{q} \) where \( p \) and \( q \) are coprime integers (i.e., their greatest common divisor is 1).
• Prime Divisibility: If a prime number divides the square of an integer, it must also divide the integer itself. Formally, if \( p \) is a prime and \( p \mid a^2 \), then \( p \mid a \).

These theorems lead us to the following lemma which is used in the proof:

Lemma

If \( \sqrt{3} \) were rational, then it could be written as \( \frac{a}{b} \) in lowest terms, meaning \( a \) and \( b \) are coprime integers. This would imply \( a^2 = 3b^2 \). However, this leads to a contradiction because:

1. From \( a^2 = 3b^2 \), we see that \( a^2 \) is divisible by 3, implying \( a \) must also be divisible by 3 (from prime divisibility).
2. Let \( a = 3k \) for some integer \( k \). Substituting this back, we get \( (3k)^2 = 3b^2 \), which simplifies to \( 9k^2 = 3b^2 \) or \( 3k^2 = b^2 \).
3. This implies \( b^2 \) is also divisible by 3, and hence \( b \) must be divisible by 3.
4. Since both \( a \) and \( b \) are divisible by 3, this contradicts the assumption that \( a \) and \( b \) are coprime.

Thus, our initial assumption that \( \sqrt{3} \) is rational must be false. Therefore, \( \sqrt{3} \) is irrational.

The proof that \(\sqrt{3}\) is irrational is often demonstrated through a method called proof by contradiction. Here, we will explain the proof step by step with examples to make it clear and intuitive.

1. Assumption: Suppose \(\sqrt{3}\) is rational. This means it can be expressed as a fraction \(\frac{a}{b}\) where \(a\) and \(b\) are integers with no common factors other than 1 (i.e., they are coprime), and \(b \neq 0\).

2. Equation Setup: If \(\sqrt{3} = \frac{a}{b}\), then squaring both sides gives:

   \[ 3 = \frac{a^2}{b^2} \]

   which implies:

   \[ a^2 = 3b^2 \]

3. Divisibility Argument: This equation indicates that \(a^2\) is divisible by 3. Therefore, \(a\) must also be divisible by 3 (since 3 is a prime number). Let \(a = 3k\) for some integer \(k\).

4. Substitute and Simplify: Substituting \(a = 3k\) into the equation \(a^2 = 3b^2\) gives:

   \[ (3k)^2 = 3b^2 \]

   \[ 9k^2 = 3b^2 \]

   Dividing both sides by 3 yields:

   \[ 3k^2 = b^2 \]

   This implies that \(b^2\) is divisible by 3, and thus \(b\) must also be divisible by 3.

5. Contradiction: Since both \(a\) and \(b\) are divisible by 3, this contradicts our initial assumption that \(a\) and \(b\) have no common factors other than 1. Therefore, \(\sqrt{3}\) cannot be expressed as a fraction \(\frac{a}{b}\).

6. Conclusion: Since our initial assumption leads to a contradiction, we conclude that \(\sqrt{3}\) is irrational.

To further illustrate this, let's consider an example:

• Example 1: Verify the irrationality of \(\sqrt{3}\) using its decimal expansion.

  \(\sqrt{3} \approx 1.732050807568877...\)

  The decimal expansion of \(\sqrt{3}\) is non-terminating and non-repeating, which is characteristic of irrational numbers.

• Example 2: Constructing \(\sqrt{3}\) on a number line using geometric methods.

  Consider a right triangle with legs of 1 unit each. The hypotenuse, according to the Pythagorean theorem, is \(\sqrt{2}\). Construct another right triangle with one leg as \(\sqrt{2}\) and the other leg as 1. The hypotenuse of this new triangle will be \(\sqrt{3}\). This geometric construction helps visualize \(\sqrt{3}\) and reinforces its irrationality.

Understanding the proof that the square root of 3 (\(\sqrt{3}\)) is irrational can be challenging, and several common misconceptions often arise. Here are some of the most frequent misunderstandings:

• Misconception 1: Any square root can be rational if expressed in simplest form.

  Many people believe that any square root can be written as a fraction, especially if simplified correctly. However, this is not true for non-perfect squares. \(\sqrt{3}\) is an irrational number, meaning it cannot be expressed as a ratio of two integers. Unlike \(\sqrt{4} = 2\), which is a perfect square and thus rational, \(\sqrt{3}\) does not have such a property.

• Misconception 2: Rational and Irrational Numbers Can Be Confused.

  There is often confusion between rational and irrational numbers. Rational numbers are numbers that can be expressed as the quotient of two integers (like \(\frac{2}{3}\)). Irrational numbers, such as \(\sqrt{3}\), have non-repeating, non-terminating decimal expansions and cannot be expressed as a fraction of two integers.

• Misconception 3: Decimal Approximations Prove Rationality.

  Another common mistake is to assume that if a decimal approximation of \(\sqrt{3}\) is used (e.g., 1.732), it proves the number is rational. However, this is just an approximation. The true value of \(\sqrt{3}\) has an infinite, non-repeating decimal expansion, which is a key characteristic of irrational numbers.

• Misconception 4: Proofs by Contradiction Are Not Trustworthy.

  Some might doubt the validity of proofs by contradiction, thinking they are less rigorous. In reality, proof by contradiction is a robust mathematical method. For example, assuming \(\sqrt{3}\) is rational leads to a logical contradiction, thus proving it must be irrational.

• Misconception 5: Irrational Numbers Are Rare or Unimportant.

  There's a belief that irrational numbers are rare or of lesser importance. In fact, they are quite common and fundamental in various fields of mathematics and science. Numbers like \(\pi\) and \(e\) are also irrational and are crucial in geometry, calculus, and many real-world applications.

Understanding these misconceptions helps in grasping the true nature of irrational numbers and appreciating the rigorous proof techniques used to establish their properties.

Irrational numbers, such as the square root of 3, play a vital role in various fields of science, engineering, and everyday life. Here are some key applications:

• Geometry and Architecture

  The square root of 3 is crucial in constructing equilateral triangles and hexagons. For an equilateral triangle with side length 1, the height is √3, which is essential for achieving structural balance and aesthetic designs in architectural patterns and tiling.

• Electrical Engineering

  In three-phase power systems, the square root of 3 is used to calculate the line-to-line voltages from phase voltages, enhancing the efficiency and stability of electrical power distribution.

• Computer Science

  √3 is often encountered in algorithms and computer graphics, especially in calculations involving hexagonal grids and pixel geometry, where it helps in optimizing data representation and visual clarity.

• Trigonometry

  Trigonometric functions frequently utilize √3 in problems involving 30°, 60°, and 90° triangles. It appears in the sine, cosine, and tangent values of these angles, playing a critical role in solving geometric problems.

• Mathematical Analysis

  Irrational numbers like √3 are important in various proofs and theorems in higher mathematics. They illustrate the concept of numbers that cannot be expressed as fractions, expanding our understanding of numerical systems.

These applications underscore the significance of irrational numbers beyond pure mathematics, showcasing their integral role in various practical and theoretical constructs.

The proof of the irrationality of certain numbers, such as the square root of 3, holds significant importance in mathematics for several reasons:

• Foundation of Number Theory: Proving irrationality helps in understanding the fundamental properties of numbers. It distinguishes between rational and irrational numbers, offering a deeper insight into the structure of real numbers.
• Development of Mathematical Concepts: The proofs of irrationality often utilize methods such as proof by contradiction, which are essential techniques in higher mathematics. These methods enhance logical reasoning and problem-solving skills.
• Applications in Geometry: Irrational numbers frequently appear in geometric contexts, such as in the lengths of diagonals in polygons. For example, the length of the diagonal of a unit cube is the square root of 3, which is an irrational number.
• Implications for Algebra: Understanding irrational numbers is crucial for solving polynomial equations. The irrational roots theorem, for instance, states that irrational roots of polynomial equations with rational coefficients occur in conjugate pairs.
• Real-World Applications: Irrational numbers are used in various fields including physics, engineering, and computer science. For example, calculations involving wave frequencies and quantum mechanics often involve irrational numbers.

By proving the irrationality of numbers such as the square root of 3, mathematicians are able to classify numbers more precisely, leading to advancements in both theoretical and applied mathematics.

In addition to the traditional proof by contradiction, there are several alternative methods to demonstrate that the square root of 3 is irrational. Here, we explore a few of these methods in detail.

1. Proof by Infinite Descent

This method involves assuming that \( \sqrt{3} \) is rational and can be expressed as a fraction in its simplest form, and then deriving a smaller fraction that contradicts the assumption of simplicity.

1. Assume \( \sqrt{3} = \frac{a}{b} \) where \( a \) and \( b \) are coprime integers.
2. Then, \( 3b^2 = a^2 \).
3. This implies \( a^2 \) is divisible by 3, so \( a \) must also be divisible by 3. Let \( a = 3k \).
4. Substituting back, we get \( 3b^2 = (3k)^2 = 9k^2 \), which simplifies to \( b^2 = 3k^2 \).
5. This implies \( b^2 \) is also divisible by 3, so \( b \) must be divisible by 3, contradicting our assumption that \( a \) and \( b \) are coprime.
6. Thus, \( \sqrt{3} \) cannot be rational.

2. Proof Using the Well-Ordering Principle

This approach leverages the well-ordering principle, which states that every non-empty set of positive integers has a smallest element.

1. Assume \( \sqrt{3} = \frac{a}{b} \) where \( a \) and \( b \) are positive coprime integers, and \( a^2 = 3b^2 \).
2. We create a new pair \( (3b - a, a - b) \) which must also be integers.
3. If \( a \neq b \), then \( 3b - a < a \) and \( a - b < b \).
4. Since \( a \) and \( b \) are positive and coprime, and \( (3b - a, a - b) \) are smaller than \( a \) and \( b \), we contradict the well-ordering principle.
5. Thus, \( \sqrt{3} \) must be irrational.

3. Proof Using Continued Fractions

Continued fractions provide another elegant method to prove irrationality.

1. The continued fraction representation of \( \sqrt{3} \) is non-terminating and non-repeating.
2. If \( \sqrt{3} \) were rational, its continued fraction representation would be finite or eventually periodic.
3. The continued fraction for \( \sqrt{3} \) is \( [1; \overline{1, 2}] \), indicating it is non-terminating and non-repeating.
4. This non-periodicity confirms that \( \sqrt{3} \) is irrational.

4. Proof by Contradiction Using the Fundamental Theorem of Arithmetic

This method uses the uniqueness of prime factorization.

1. Assume \( \sqrt{3} = \frac{a}{b} \) where \( a \) and \( b \) are coprime integers.
2. Then \( a^2 = 3b^2 \).
3. The prime factorization of \( a^2 \) must include an even number of each prime factor.
4. However, the right side \( 3b^2 \) must include an odd number of the prime factor 3.
5. This contradiction implies that \( \sqrt{3} \) cannot be rational.

Each of these methods, while different in approach, arrives at the same conclusion: the square root of 3 is irrational.

Understanding irrational numbers can be challenging because they cannot be expressed as a simple fraction. Here, we will explore different ways to visualize irrational numbers to gain a better understanding of their nature and significance.

Number Line Representation

One of the simplest ways to visualize an irrational number like \(\sqrt{3}\) is by placing it on a number line. To do this:

1. Identify the approximate value of \(\sqrt{3}\), which is about 1.732.
2. Mark the position of 1.732 on the number line between 1 and 2.
3. This point shows where \(\sqrt{3}\) lies in relation to other numbers.

Despite being an approximation, this method helps us see that \(\sqrt{3}\) is between two rational numbers but cannot be exactly pinpointed as a fraction.

Geometric Representation

Another effective way to visualize \(\sqrt{3}\) is through geometry:

1. Draw an equilateral triangle with each side of length 2.
2. By splitting the triangle into two right triangles, each right triangle will have sides of lengths 1, \(\sqrt{3}\), and 2.
3. The hypotenuse (2) and one side (1) form a right angle, and the remaining side will be \(\sqrt{3}\).

This geometric representation shows that \(\sqrt{3}\) is the length of the side opposite the right angle in a right triangle with sides of lengths 1 and 2.

Continued Fractions

Continued fractions provide a unique way to represent irrational numbers. For \(\sqrt{3}\), the continued fraction representation is:

\[\sqrt{3} = 1 + \frac{1}{1 + \frac{1}{2 + \frac{1}{1 + \frac{1}{2 + \ldots}}}}\]

This representation illustrates the non-repeating and infinite nature of irrational numbers, highlighting that they cannot be expressed as a simple fraction.

Decimal Expansion

Irrational numbers have non-repeating, non-terminating decimal expansions. For example, the decimal expansion of \(\sqrt{3}\) is:

\[ \sqrt{3} \approx 1.732050807568877...\]

Observing the decimal expansion emphasizes the complexity and irregularity of irrational numbers, distinguishing them from rational numbers.

Applications and Practical Implications

Irrational numbers, including \(\sqrt{3}\), are crucial in various fields such as engineering, physics, and computer science. For example:

• In engineering, precise calculations involving irrational numbers are necessary for designing structures and systems.
• In physics, many natural phenomena and constants, like the golden ratio, involve irrational numbers.
• In computer science, algorithms often require precise irrational values for accurate computations and simulations.

Understanding and visualizing irrational numbers help us appreciate their importance and application in the real world.

The study of the irrationality of numbers, such as the square root of 3, opens the door to numerous advanced topics in mathematics. Here are some areas for further exploration:

1. Diophantine Approximation

Diophantine approximation deals with the approximation of real numbers by rational numbers. Theorems in this field, such as Dirichlet's approximation theorem, provide insights into how well irrational numbers can be approximated by rationals.

2. Continued Fractions

Continued fractions provide a way to represent irrational numbers with an infinite sequence of integer terms. The continued fraction representation of √3 can be explored to understand its properties and behavior.

3. Algebraic Number Theory

This branch of number theory studies algebraic structures related to algebraic integers. The proof of the irrationality of √3 can be extended to explore properties of algebraic numbers, which are roots of polynomial equations with integer coefficients.

4. Transcendental Number Theory

While √3 is an algebraic number, transcendental number theory studies numbers that are not roots of any non-zero polynomial with rational coefficients. Exploring the differences between algebraic and transcendental numbers deepens the understanding of irrationality.

5. Metric Number Theory

Metric number theory involves the study of the distribution of number sequences. Concepts such as measure theory and Borel sets are used to analyze the properties of irrational numbers in different mathematical spaces.

6. Rational Approximations and Liouville Numbers

Liouville numbers are a specific type of transcendental number that can be approximated "too well" by rational numbers. The study of these numbers helps in understanding the limits of rational approximation for irrational numbers.

7. p-adic Numbers

p-adic number systems extend the concept of integers and rationals. Exploring √3 in the context of p-adic numbers offers a unique perspective on number theory and the properties of irrational numbers in different number systems.

Further Reading

In conclusion, the proof that the square root of 3 is irrational relies on the method of contradiction, a powerful tool in mathematical reasoning. By assuming the contrary—that √3 is rational—we derive a contradiction, demonstrating that our initial assumption must be false.

To summarize the proof:

1. Assume √3 is rational, which means it can be expressed as a fraction of two integers in the form √3 = a/b, where a and b are coprime integers (having no common factors other than 1).
2. Squaring both sides of the equation yields 3 = a²/b², or equivalently, 3b² = a².
3. This implies that a² is divisible by 3. Consequently, a must also be divisible by 3 (since the square of a number divisible by a prime is also divisible by that prime).
4. Let a = 3k for some integer k. Substituting back into the equation, we get 3b² = (3k)², which simplifies to 3b² = 9k² or b² = 3k².
5. This implies that b² is also divisible by 3, and thus b must be divisible by 3.
6. However, this contradicts our initial statement that a and b are coprime (since both a and b would have a common factor of 3).

Since our initial assumption leads to a contradiction, we conclude that √3 cannot be expressed as a fraction of two integers, and hence, it is irrational.

The importance of this proof lies not only in demonstrating the irrationality of √3 but also in illustrating a general approach that can be applied to other non-square roots, reinforcing the depth and beauty of mathematical logic.

Understanding the irrationality of numbers like √3 has practical implications in various fields such as geometry, algebra, and number theory, and helps in the comprehension of more advanced mathematical concepts.