Topic what's the square root of 2: What's the square root of 2? Uncover the intriguing world of this unique irrational number. From its historical discovery to its vital role in mathematics, physics, and engineering, learn why the square root of 2 is more than just a number. Join us in exploring its endless applications and fascinating properties.
Table of Content
- Square Root of 2
- Introduction to Square Root of 2
- Historical Background and Discovery
- Decimal Representation and Approximation
- Mathematical Properties of Square Root of 2
- Geometric Significance
- Algebraic Significance
- Applications in Mathematics
- Applications in Physics
- Applications in Engineering
- Applications in Computer Science
- Role in Trigonometry
- Square Root of 2 in Popular Culture
- Interesting Facts and Trivia
- Conclusion
- YOUTUBE: Khám phá sự kỳ diệu của căn bậc hai của 2 trong video này. Tìm hiểu về lịch sử, tính chất toán học và ứng dụng của nó.
Square Root of 2
The square root of 2, often denoted as or √2, is a mathematical constant that is the positive algebraic solution to the equation . It is an irrational number, meaning that it cannot be expressed exactly as a fraction of two integers. Its decimal representation is non-repeating and non-terminating.
Decimal Representation
The approximate value of √2 to 10 decimal places is:
≈ 1.4142135623
Historical Significance
The discovery of the square root of 2 is often attributed to the ancient Greeks. It is said that the Pythagorean philosopher Hippasus discovered that √2 is irrational while attempting to express it as a fraction, which contradicted the Pythagorean belief that all numbers are rational.
Mathematical Properties
- √2 is irrational: It cannot be expressed as a simple fraction.
- √2 is algebraic: It is a root of the polynomial equation .
- Geometrical significance: It represents the length of the diagonal of a square with side length 1.
Proof of Irrationality
One common proof that √2 is irrational is by contradiction:
- Assume that √2 is rational, meaning it can be written as a fraction where p and q are coprime integers (having no common factors other than 1).
- Then, , or .
- This implies that is even, so p must be even. Let p = 2k for some integer k.
- Substitute p = 2k into the equation: , resulting in 4k² = 2q², or 2k² = q².
- This implies that q² is even, so q must also be even.
- However, if both p and q are even, they have a common factor of 2, contradicting the assumption that they are coprime.
- Therefore, √2 cannot be expressed as a fraction, proving it is irrational.
Applications
The square root of 2 is widely used in mathematics, physics, engineering, and computer science. Some notable applications include:
- Geometry: Calculating the diagonal of squares and rectangles.
- Trigonometry: In trigonometric functions and identities.
- Algebra: Solving quadratic equations.
- Computer Science: Algorithms and numerical methods.

READ MORE:
Introduction to Square Root of 2
The square root of 2, often denoted as √2, is a positive real number that, when multiplied by itself, equals 2. It is an irrational number, which means it cannot be expressed as a simple fraction and its decimal representation is non-terminating and non-repeating.
Mathematically, it can be represented as:
\[ \sqrt{2} \approx 1.4142135623730950488\ldots \]
The square root of 2 is significant in various fields of science and mathematics due to its unique properties and historical importance. It was one of the first numbers known to be irrational, a discovery attributed to the ancient Greeks, particularly the Pythagoreans.
The value of √2 is crucial in geometry, especially in the context of right-angled triangles. According to the Pythagorean theorem, the length of the hypotenuse of an isosceles right triangle with legs of length 1 is √2.
This value also appears in various mathematical constants and equations, making it a fundamental element in the study of mathematics and related disciplines.
Historical Background and Discovery
The square root of 2, often denoted as \( \sqrt{2} \), holds a significant place in the history of mathematics. Its discovery is attributed to ancient Greek mathematicians, particularly within the Pythagorean school around the 5th century BCE. The Greeks initially believed that all numbers could be expressed as the ratio of two integers, known as rational numbers.
However, the concept of irrational numbers emerged from the discovery of \( \sqrt{2} \). According to legend, the Greek mathematician Hippasus found that the diagonal of a square with side length 1 could not be expressed as a ratio of two integers. This discovery was so controversial that it allegedly led to his execution for revealing such an unsettling truth.
The mathematical proof of the irrationality of \( \sqrt{2} \) involves assuming it can be expressed as a fraction \( \frac{a}{b} \) in its simplest form, and showing that both \( a \) and \( b \) must be even, leading to a contradiction. This proof demonstrates that \( \sqrt{2} \) cannot be written as a ratio of integers, thus proving its irrationality.
The Greeks' approach to dealing with such irrational numbers was primarily geometric. In Euclid's "Elements," Book X is dedicated to the classification and properties of irrational magnitudes, where \( \sqrt{2} \) features prominently.
Today, \( \sqrt{2} \) is understood to be approximately 1.414213562, a non-repeating and non-terminating decimal. Its discovery was a pivotal moment in mathematics, highlighting the need for a more comprehensive number system beyond rational numbers.
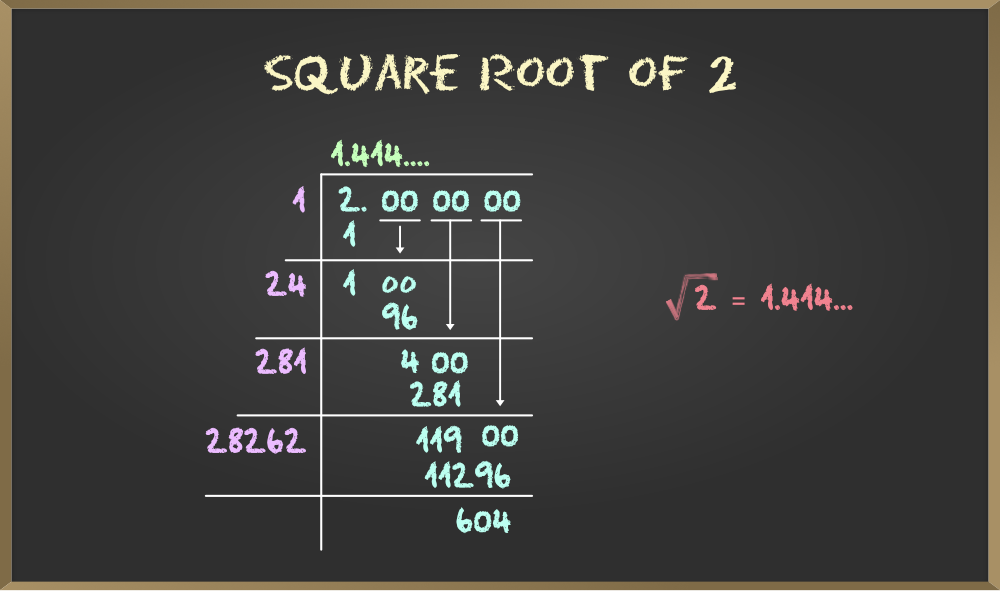
Decimal Representation and Approximation
The square root of 2, denoted as \(\sqrt{2}\), is an irrational number, meaning it cannot be expressed exactly as a simple fraction. Its decimal representation is non-repeating and non-terminating. The value of \(\sqrt{2}\) begins as follows:
\[
\sqrt{2} \approx 1.41421356237309504880168872420969807856967187537694807317667973799 \ldots
\]
Here are some methods to approximate \(\sqrt{2}\):
Newton's Method
Newton's method, also known as the Newton-Raphson method, is an iterative approach to approximate the roots of a real-valued function. For \(\sqrt{2}\), the iterative formula is:
\[
x_{n+1} = \frac{1}{2} \left( x_n + \frac{2}{x_n} \right)
\]
Starting with an initial guess \(x_0 = 1\), the first few iterations are:
- \(x_1 = \frac{1}{2} \left( 1 + \frac{2}{1} \right) = 1.5\)
- \(x_2 = \frac{1}{2} \left( 1.5 + \frac{2}{1.5} \right) \approx 1.41667\)
- \(x_3 = \frac{1}{2} \left( 1.41667 + \frac{2}{1.41667} \right) \approx 1.41422\)
Continued Fraction Representation
The continued fraction representation of \(\sqrt{2}\) is:
\[
\sqrt{2} = 1 + \frac{1}{2 + \frac{1}{2 + \frac{1}{2 + \ldots}}}
\]
This representation provides a sequence of increasingly accurate rational approximations. The first few are:
- \(\frac{1}{1} = 1\)
- \(\frac{3}{2} = 1.5\)
- \(\frac{7}{5} = 1.4\)
- \(\frac{17}{12} \approx 1.41667\)
- \(\frac{41}{29} \approx 1.41379\)
Estimation Formula
An estimation formula for square roots is given by:
\[
\sqrt{n} \approx \sqrt{p} + \frac{q}{2\sqrt{p} + 1}
\]
where \(n = p + q\), with \(p\) being the largest perfect square less than \(n\). For example, for \(n = 2\):
\[
\sqrt{2} \approx 1 + \frac{1}{2 \cdot 1 + 1} = 1 + \frac{1}{3} \approx 1.3333
\]
Using these methods, we can obtain highly accurate approximations of \(\sqrt{2}\) for practical purposes in various fields such as mathematics, physics, and engineering.
Mathematical Properties of Square Root of 2
The square root of 2, often denoted as \( \sqrt{2} \), is a fundamental mathematical constant with several interesting properties. Here, we explore some of its key mathematical properties:
- Irrationality: The square root of 2 is an irrational number, meaning it cannot be expressed as a ratio of two integers. This property was first proved by ancient Greek mathematicians.
- Decimal Representation: The decimal expansion of \( \sqrt{2} \) is non-repeating and infinite. Its approximate value is 1.41421356237, but it extends indefinitely without repeating.
- Algebraic Representation: Algebraically, \( \sqrt{2} \) is the solution to the equation \( x^2 = 2 \). This equation has two solutions, \( \sqrt{2} \) and \( -\sqrt{2} \), due to the property of square roots having both positive and negative solutions.
- Continued Fraction Representation: The square root of 2 can be represented as a continued fraction:
\[ \sqrt{2} = 1 + \frac{1}{2 + \frac{1}{2 + \frac{1}{2 + \ldots}}} \] - Geometric Significance: Geometrically, \( \sqrt{2} \) is the length of the diagonal of a square with side length 1. This follows from the Pythagorean theorem, where the diagonal forms the hypotenuse of a right-angled triangle with sides of length 1.
Decimal Approximation of \( \sqrt{2} \)
The value of \( \sqrt{2} \) to 50 decimal places is:
\[ \sqrt{2} \approx 1.41421356237309504880168872420969807856967187537694 \]
Mathematical Significance
In mathematics, the square root of 2 appears in various contexts, including:
- Trigonometry: It is related to trigonometric functions, particularly in the values of sine and cosine for 45° angles.
- Algebra: Appears in solutions to quadratic equations and in the simplification of expressions involving radicals.
Understanding the square root of 2 and its properties is essential in various branches of mathematics and science, highlighting its significance beyond basic arithmetic.

Geometric Significance
The square root of 2, often denoted as \( \sqrt{2} \), holds substantial geometric significance. One of the most classic geometric interpretations of \( \sqrt{2} \) arises from the properties of a square and its diagonal. Let's explore its significance step by step:
The Diagonal of a Square
Consider a square with a side length of 1 unit. According to the Pythagorean theorem, the length of the diagonal of this square is given by:
\[
\text{Diagonal} = \sqrt{1^2 + 1^2} = \sqrt{2}
\]
This shows that the diagonal of a unit square is \( \sqrt{2} \) units long, establishing the fundamental geometric presence of \( \sqrt{2} \) in the simplest of shapes.
Proportionality in Rectangles
The ratio \( \sqrt{2} \) is also significant in defining the proportions of certain rectangles, especially in paper sizes like the ISO 216 standard (A4, A3, etc.). When a \( \sqrt{2} \) rectangle is folded in half along its longer side, the resulting smaller rectangles maintain the same \( \sqrt{2} \) proportion.
Square Root of 2 in Isosceles Right Triangles
An isosceles right triangle, where the two legs are equal, also demonstrates the importance of \( \sqrt{2} \). If each leg of the triangle is of length 1, the hypotenuse will be \( \sqrt{2} \). This is derived as follows:
\[
\text{Hypotenuse} = \sqrt{1^2 + 1^2} = \sqrt{2}
\]
Geometric Proof of Irrationality
The geometric proof of the irrationality of \( \sqrt{2} \) further underscores its geometric importance. This proof often involves demonstrating that if \( \sqrt{2} \) were rational (i.e., expressible as a fraction of two integers), it would lead to a contradiction when analyzing the properties of isosceles right triangles formed by iteratively halving the triangles.
Applications in Architecture and Art
The properties of \( \sqrt{2} \) have been used in various fields, including architecture and art, where the proportions and symmetry of shapes and structures often adhere to the geometric properties defined by \( \sqrt{2} \). For example, the design of certain ancient structures and modern architectural designs frequently incorporate the √2 ratio to achieve aesthetically pleasing and structurally sound designs.
In conclusion, the geometric significance of \( \sqrt{2} \) spans from its fundamental role in defining the diagonal of a square to its applications in various fields, demonstrating its pervasive and essential presence in geometry and beyond.
Algebraic Significance
The square root of 2, often represented as \( \sqrt{2} \), holds significant algebraic properties and implications in mathematics. It is classified as an algebraic number, which means it is a root of a non-zero polynomial equation with rational coefficients. Specifically, \( \sqrt{2} \) is the solution to the polynomial equation:
\[ x^2 - 2 = 0 \]
This equation highlights its algebraic nature since the roots of this polynomial are \( \sqrt{2} \) and \( -\sqrt{2} \).
The algebraic significance of \( \sqrt{2} \) extends to various mathematical concepts and fields:
- Field Theory: In field theory, \( \sqrt{2} \) is a crucial example of an algebraic number that is not rational, demonstrating the existence of irrational algebraic numbers. The field \( \mathbb{Q}(\sqrt{2}) \) consists of all numbers of the form \( a + b\sqrt{2} \), where \( a \) and \( b \) are rational numbers, forming a simple field extension of \( \mathbb{Q} \).
- Polynomial Roots: The minimal polynomial of \( \sqrt{2} \) over the rationals is \( x^2 - 2 \). This polynomial is irreducible over \( \mathbb{Q} \), which means it cannot be factored into polynomials of lower degree with rational coefficients.
- Symmetry and Algebraic Structures: The properties of \( \sqrt{2} \) illustrate the symmetry in algebraic structures. For instance, the sum and product of \( \sqrt{2} \) with other algebraic numbers yield further algebraic numbers, maintaining the closure property of algebraic fields.
Moreover, the algebraic nature of \( \sqrt{2} \) contrasts with transcendental numbers, which are not roots of any non-zero polynomial equation with rational coefficients. This distinction emphasizes the different classes within the set of real numbers.
Understanding the algebraic significance of \( \sqrt{2} \) provides insights into the broader context of algebra and number theory, illustrating how specific numbers can have profound implications in mathematical structures and theories.
Applications in Mathematics
The square root of 2 (\(\sqrt{2}\)) holds significant importance in various mathematical contexts due to its unique properties and applications. Below are some key areas where \(\sqrt{2}\) is notably applied:
-
Pythagorean Theorem: The square root of 2 is famously linked to the Pythagorean theorem. For a right triangle with legs of length 1, the hypotenuse will have a length of \(\sqrt{2}\). This relationship is fundamental in Euclidean geometry.
-
Diophantine Equations: The study of solutions to polynomial equations with integer coefficients, \(\sqrt{2}\) appears in the context of irrational solutions. It exemplifies the need for real numbers beyond rationals in solving such equations.
-
Geometry: \(\sqrt{2}\) is crucial in various geometric constructions, such as the construction of a square's diagonal. Given a square with side length 1, the diagonal's length is \(\sqrt{2}\), demonstrating the concept of irrational lengths.
-
Trigonometry: In trigonometry, \(\sqrt{2}\) is encountered in the context of sine and cosine functions for 45-degree angles. Specifically, \(\sin(45^\circ) = \cos(45^\circ) = \frac{1}{\sqrt{2}}\).
-
Linear Algebra: The eigenvalues of certain matrices involve \(\sqrt{2}\), particularly in rotation matrices and transformations in two dimensions. This plays a significant role in understanding vector spaces and matrix operations.
The square root of 2 is thus integral in a variety of mathematical disciplines, demonstrating its fundamental nature and widespread applicability.
Applications in Physics
The square root of 2 has significant applications in various fields of physics. Below are some key areas where it is particularly important:
- Kinematics:
In kinematics, the equation
\(\upsilon = \sqrt{2gh}\) is used to describe the final velocity \(\upsilon\) of an object falling from a height \(h\) under the influence of gravity \(g\). This is derived from the conservation of energy principle where potential energy is converted into kinetic energy. - Wave Mechanics:
In wave mechanics, the ratio of the wavelengths of certain standing waves involves the square root of 2. For example, in the study of sound waves in pipes, the frequencies of the harmonic series include terms like
\(\sqrt{2}\) , indicating the relationship between different modes of vibration. - Electromagnetism:
In alternating current (AC) circuits, the root mean square (RMS) value of the current or voltage is often used, which involves the square root of 2. The RMS value of an AC waveform is its peak value divided by
\(\sqrt{2}\) , providing a measure of the equivalent DC value that would produce the same power dissipation. - Quantum Mechanics:
The Schrödinger equation, which describes how the quantum state of a physical system changes over time, sometimes involves terms that include the square root of 2 in solutions for the wave functions of particles in potential wells and other quantum systems.
- Relativity:
In special relativity, the Lorentz factor \(\gamma\) is used to describe time dilation and length contraction. For velocities close to the speed of light, the factor involves the term
\(\sqrt{1 - \frac{v^2}{c^2}}\) , and under certain conditions, the value approaches the square root of 2, affecting the relativistic equations.
These examples illustrate the broad impact of the square root of 2 across different domains of physics, highlighting its importance in both theoretical and applied contexts.
Applications in Engineering
The square root of 2 (\(\sqrt{2}\)) has numerous applications in various engineering fields. Here are some significant examples:
- Electrical Engineering: In electrical engineering, the root mean square (RMS) values are essential for calculating effective voltage and current in AC circuits. The RMS value is derived using the square root of 2:
- For a sinusoidal waveform, the relationship between the peak value (\(V_{\text{peak}}\)) and the RMS value (\(V_{\text{rms}}\)) is \( V_{\text{rms}} = \frac{V_{\text{peak}}}{\sqrt{2}} \). This calculation helps in designing circuits and ensuring their safe and efficient operation.
- Mechanical Engineering: In mechanical engineering, the concept of \(\sqrt{2}\) is used in stress and strain analysis, particularly in calculating the effective stress in components subjected to complex loading conditions. The von Mises stress criterion, which involves the square root of the sum of the squares of the principal stresses, helps predict yielding of materials under any loading condition.
- Structural Engineering: In structural engineering, the square root of 2 is used in the Pythagorean theorem to determine diagonal distances in rectangular frames and trusses, which are critical for calculating forces and designing stable structures.
- Civil Engineering: In civil engineering, \(\sqrt{2}\) is applied in the analysis of slopes and earth pressures. For example, in the calculation of the critical height of a slope using the slip circle method, \(\sqrt{2}\) appears in determining the factor of safety against sliding.
- Control Systems: In control systems engineering, \(\sqrt{2}\) is significant in the design of optimal controllers. For instance, in tuning proportional-integral-derivative (PID) controllers, the damping ratio of \(\frac{1}{\sqrt{2}}\) (approximately 0.707) is often used to achieve critical damping, providing a balance between speed and stability.
The applications of \(\sqrt{2}\) in engineering highlight its fundamental role in various calculations and design processes, ensuring accuracy, efficiency, and safety in engineering practices.
Applications in Computer Science
The square root of 2, often denoted as √2, plays a significant role in various fields of computer science. Its applications are broad and diverse, ranging from algorithms and computational geometry to data structures and computer graphics.
-
Algorithms and Computational Geometry:
In computational geometry, the square root of 2 frequently appears in algorithms involving distances and metric spaces. One classic example is the Euclidean distance formula in two dimensions, which is derived using the Pythagorean theorem. For instance, the distance between two points \((x_1, y_1)\) and \((x_2, y_2)\) is given by:
\[d = \sqrt{(x_2 - x_1)^2 + (y_2 - y_1)^2}\]
When the points form a right isosceles triangle, the distance is \(\sqrt{2}\), making it a fundamental constant in such computations.
-
Data Structures:
The square root of 2 is essential in optimizing certain data structures. For example, in quad-trees, which are used to partition a two-dimensional space by recursively subdividing it into four quadrants, the properties of √2 help in balancing and efficiently managing the spatial data. This is crucial in applications like image processing, geographical information systems (GIS), and computer graphics.
-
Computer Graphics:
In computer graphics, √2 is crucial in rendering and transformations. One common application is in scaling transformations, where maintaining the aspect ratio often involves factors of √2. Additionally, in the field of ray tracing and rasterization, the calculation of distances and angles frequently utilizes √2 to ensure accuracy and precision in visual representations.
-
Machine Learning and Data Analysis:
In machine learning, particularly in clustering algorithms like k-means, distance metrics such as the Euclidean distance use √2. When dealing with high-dimensional data, the distance between points often involves computations that include √2, affecting how clusters are formed and interpreted.
-
Cryptography:
In cryptography, the square root of 2 is sometimes involved in algorithms related to key generation and encryption processes. The mathematical properties of irrational numbers like √2 can be utilized to enhance the complexity and security of cryptographic methods.
Overall, the square root of 2 is a fundamental mathematical constant that significantly influences various aspects of computer science, from theoretical algorithms to practical applications in graphics and data analysis.
Role in Trigonometry
The square root of 2, denoted as √2, has a crucial role in trigonometry, particularly in the context of right triangles, unit circles, and trigonometric identities.
-
Right Triangles:
In a right isosceles triangle, the two legs are of equal length, and the hypotenuse is \(\sqrt{2}\) times the length of each leg. If each leg has length \(1\), the hypotenuse will be \(\sqrt{2}\). This relationship is derived from the Pythagorean theorem:
\[ \text{Hypotenuse}^2 = 1^2 + 1^2 = 2 \Rightarrow \text{Hypotenuse} = \sqrt{2} \]
-
Unit Circle:
In the unit circle, the coordinates of points at 45° (or \(\pi/4\) radians) involve \(\sqrt{2}/2\). These points are \((\cos(\pi/4), \sin(\pi/4)) = (\sqrt{2}/2, \sqrt{2}/2)\). This value is fundamental in understanding the symmetry and properties of trigonometric functions.
\[ \cos(\pi/4) = \sin(\pi/4) = \frac{\sqrt{2}}{2} \]
-
Trigonometric Identities:
The value of \(\sqrt{2}\) appears in several trigonometric identities and equations. For example, the sine and cosine of 45° are both \(\sqrt{2}/2\), leading to the identity:
\[ \sin^2(\theta) + \cos^2(\theta) = 1 \]
When \(\theta = \pi/4\):
\[ \left(\frac{\sqrt{2}}{2}\right)^2 + \left(\frac{\sqrt{2}}{2}\right)^2 = \frac{2}{4} + \frac{2}{4} = 1 \]
-
Rotation Matrices:
Rotation matrices in linear algebra and trigonometry use \(\sqrt{2}/2\) for rotations by 45°. The 2D rotation matrix for a 45° rotation is:
\[
R_{45} = \begin{pmatrix}
\cos(45^\circ) & -\sin(45^\circ) \\
\sin(45^\circ) & \cos(45^\circ)
\end{pmatrix} = \begin{pmatrix}
\frac{\sqrt{2}}{2} & -\frac{\sqrt{2}}{2} \\
\frac{\sqrt{2}}{2} & \frac{\sqrt{2}}{2}
\end{pmatrix}
\] -
Special Angles:
In trigonometric calculations involving special angles, such as 45° or \(\pi/4\), \(\sqrt{2}\) simplifies the process. These angles are pivotal in constructing trigonometric tables and simplifying complex trigonometric expressions.
Overall, \(\sqrt{2}\) is integral to understanding and applying trigonometric principles, from basic triangle properties to advanced identities and transformations.
Square Root of 2 in Popular Culture
The square root of 2, often represented as √2, has found its way into various aspects of popular culture, symbolizing mathematical intrigue and complexity. Its presence spans literature, films, music, and more, highlighting its influence beyond pure mathematics.
-
Literature:
√2 has been featured in numerous literary works, often symbolizing the beauty and mystery of mathematics. In particular, it is referenced in mathematical fiction where characters explore the concept of irrational numbers and their implications. The number's role in illustrating the infinite and the unknowable makes it a popular theme among authors who delve into philosophical and mathematical explorations.
-
Films and Television:
In films and TV shows, √2 often appears in scenes involving mathematics or engineering. It is sometimes used to represent a critical discovery or a key part of solving a complex problem. This number's unique properties make it a favorite tool for screenwriters aiming to inject a sense of intellectual achievement or to highlight a character's mathematical prowess.
-
Music:
Musicians and composers have occasionally used √2 in their works, especially in compositions that explore mathematical concepts. The use of irrational numbers in music can create unique and intriguing rhythms and harmonies, showcasing the connection between mathematics and art. This intersection highlights how mathematical constants like √2 can inspire creativity in unexpected ways.
-
Art and Design:
In the visual arts, √2 is used in design principles and aesthetics. The diagonal of a square, which measures √2 times the length of a side, is a common element in artistic compositions. This proportion is often employed to create visually appealing and balanced designs, demonstrating the pervasive influence of mathematical constants in art.
-
Games and Puzzles:
Mathematical puzzles and games frequently feature √2 to challenge players' understanding of irrational numbers. These games often require players to grasp the properties of √2 to solve complex problems, making it a popular choice for educational and recreational mathematics.
Overall, the square root of 2 transcends its mathematical origins, becoming a symbol of the enigmatic and the infinite in various cultural contexts. Its presence in popular culture underscores the deep connection between mathematics and human creativity.

Interesting Facts and Trivia
The square root of 2, denoted as √2, is a fascinating number with a rich history and many intriguing properties. Here are some interesting facts and trivia about √2:
-
First Known Irrational Number:
√2 is historically significant as the first known irrational number. The discovery is attributed to the ancient Greeks, particularly the Pythagoreans, who found that √2 could not be expressed as a ratio of two integers.
-
Geometric Significance:
In geometry, √2 is the length of the diagonal of a square with side length 1. This relationship is a direct consequence of the Pythagorean theorem:
\[ \text{Diagonal} = \sqrt{1^2 + 1^2} = \sqrt{2} \]
-
Decimal Representation:
√2 is approximately equal to 1.41421356237, but its decimal representation is non-repeating and non-terminating. This infinite nature reflects its irrationality.
-
Continued Fraction:
√2 can be expressed as a continued fraction:
\[ \sqrt{2} = 1 + \frac{1}{2 + \frac{1}{2 + \frac{1}{2 + \cdots}}} \]
This representation provides a way to approximate √2 with high precision.
-
Approximation in Ancient Civilizations:
Ancient civilizations, such as the Babylonians, had accurate approximations of √2. One Babylonian tablet, dated to around 1800 BCE, approximates √2 to four decimal places as 1.4142.
-
Unique Mathematical Property:
√2 is known to be the simplest example of a number that cannot be exactly calculated or expressed as a fraction. This simplicity makes it a cornerstone in the study of irrational numbers.
-
Use in Construction:
The value of √2 is used in construction and architecture, particularly in the design of A4 paper. The aspect ratio of A4 paper is 1:√2, which allows the paper to be folded or cut in half while maintaining the same aspect ratio.
-
Mathematical Symbolism:
In mathematics, √2 is often used to symbolize the concept of irrationality and the beauty of mathematical truths that go beyond simple fractions and integers.
The square root of 2 continues to captivate mathematicians and enthusiasts alike, symbolizing the elegance and mystery of mathematics.
Conclusion
The square root of 2, denoted as √2, is a remarkable and historically significant number that has captivated mathematicians for centuries. Its discovery as the first known irrational number marked a pivotal moment in the history of mathematics, challenging the ancient Greek belief that all numbers were rational.
√2 plays a vital role in various mathematical fields, including geometry, algebra, and trigonometry. Its presence in the diagonal of a unit square, the Euclidean distance formula, and trigonometric functions underscores its fundamental importance. Moreover, √2's irrational nature has profound implications, influencing the development of mathematical theory and the understanding of number systems.
Beyond mathematics, √2's influence extends to numerous applications in science, engineering, computer science, and even popular culture. Its appearance in algorithms, data structures, music, literature, and art demonstrates its universal appeal and versatility. The number continues to inspire creativity and innovation, bridging the gap between abstract theory and practical applications.
As an approximation, √2 is often represented as 1.41421356237, yet its exact value remains elusive, characterized by an infinite, non-repeating decimal expansion. This property highlights the depth and complexity inherent in mathematical concepts, inviting ongoing exploration and discovery.
In conclusion, the square root of 2 is much more than a mathematical constant. It symbolizes the beauty and intricacy of mathematics, serving as a testament to human curiosity and the quest for knowledge. Whether in theoretical studies or real-world applications, √2 continues to be a source of fascination and inspiration, embodying the enduring allure of mathematical inquiry.
Khám phá sự kỳ diệu của căn bậc hai của 2 trong video này. Tìm hiểu về lịch sử, tính chất toán học và ứng dụng của nó.
Căn Bậc Hai của 2
READ MORE:
Xem video của Numberphile để khám phá sự kỳ diệu của căn bậc hai của 2. Tìm hiểu về tính chất toán học và lịch sử của con số này.
Căn Bậc Hai của 2 - Numberphile