Topic 3 square root x: Discover the intricacies of the expression 3 square root x in this comprehensive guide. Learn about its mathematical properties, practical examples, and real-world applications. Perfect for students and enthusiasts, this article breaks down complex concepts into easy-to-understand language, ensuring you grasp the fundamentals and beyond of 3 square root x.
Table of Content
- Understanding the Expression \(3 \sqrt{x}\)
- Introduction to 3 √x
- Definition and Basic Concepts
- Mathematical Properties of 3 √x
- Examples and Calculations
- Graphical Representation of 3 √x
- Applications in Real-World Problems
- Common Mistakes and How to Avoid Them
- Advanced Topics Related to 3 √x
- Frequently Asked Questions (FAQs)
- Summary and Conclusion
- YOUTUBE:
Understanding the Expression \(3 \sqrt{x}\)
The expression \(3 \sqrt{x}\) represents three times the square root of a variable \(x\). In mathematical terms, this is written as:
\[
3 \sqrt{x}
\]
This means that you take the square root of \(x\) and then multiply the result by 3. The square root function is one of the most fundamental operations in mathematics, often appearing in various fields such as algebra, geometry, and calculus.
Key Points about \(3 \sqrt{x}\)
- \(x \geq 0\): The variable \(x\) must be non-negative because the square root of a negative number is not a real number.
- Multiplicative Property: The multiplication by 3 is straightforward and follows the distributive property.
- Simplification: Simplifying expressions involving \(3 \sqrt{x}\) often involves combining like terms and applying properties of square roots.
Examples
- If \(x = 4\), then:
\[
3 \sqrt{4} = 3 \times 2 = 6
\] - If \(x = 9\), then:
\[
3 \sqrt{9} = 3 \times 3 = 9
\] - If \(x = 25\), then:
\[
3 \sqrt{25} = 3 \times 5 = 15
\]
Graphical Representation
The function \(f(x) = 3 \sqrt{x}\) can be graphically represented. It is a transformation of the basic square root function \(y = \sqrt{x}\), stretched vertically by a factor of 3.

Applications
The expression \(3 \sqrt{x}\) appears in various real-world contexts, such as physics, engineering, and economics. For example, it can represent a proportional relationship where one quantity depends on the square root of another.
| Value of \(x\) | Value of \(3 \sqrt{x}\) |
|---|---|
| 1 | \(3 \sqrt{1} = 3\) |
| 4 | \(3 \sqrt{4} = 6\) |
| 9 | \(3 \sqrt{9} = 9\) |
| 16 | \(3 \sqrt{16} = 12\) |
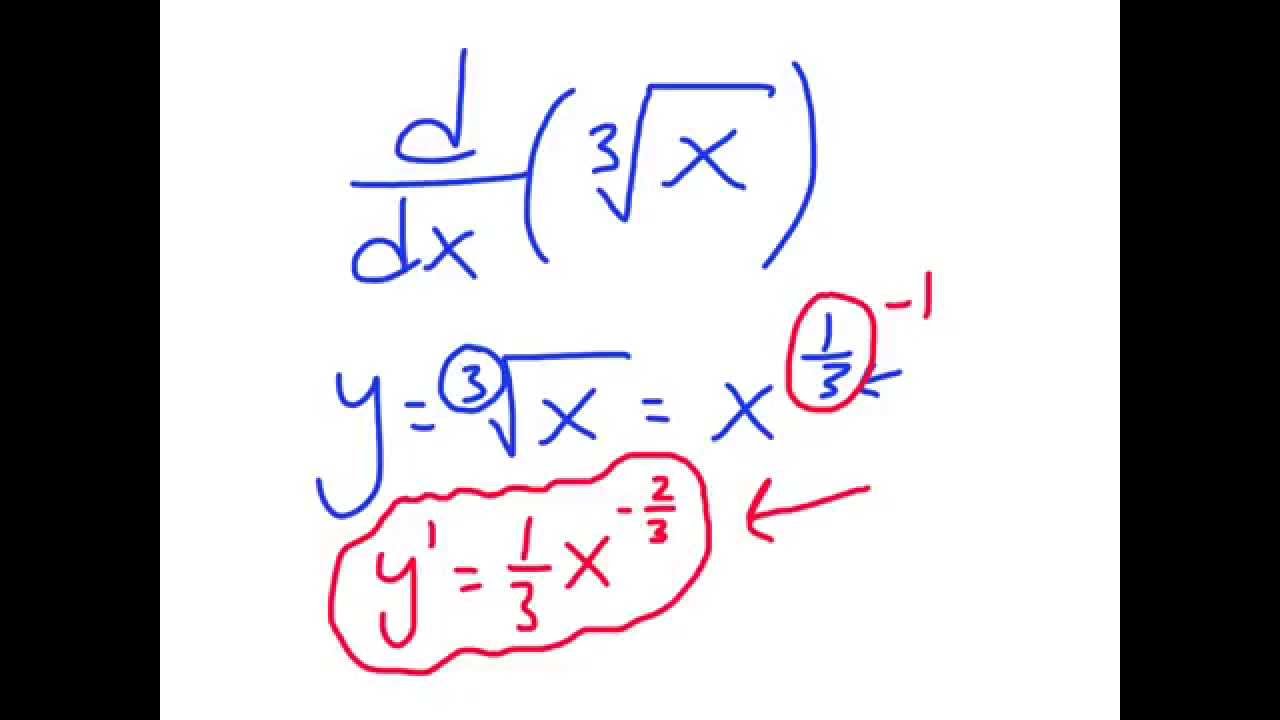
READ MORE:
Introduction to 3 √x
The expression \( 3 \sqrt{x} \), read as "3 times the square root of x," is a mathematical formula used extensively in various branches of mathematics and sciences. It represents a specific type of mathematical operation where the square root of x is taken and then multiplied by 3.
Understanding \( 3 \sqrt{x} \) is crucial for its applications in calculus, algebra, and real-world problem-solving. This expression often appears in contexts involving rates of change, optimization, and geometric transformations.
In this comprehensive guide, we will explore the definition, basic concepts, mathematical properties, examples, graphical representations, real-world applications, common mistakes to avoid, advanced topics, frequently asked questions, and conclude with a summary of \( 3 \sqrt{x} \)'s significance in mathematics and beyond.
Definition and Basic Concepts
The expression \(3 \sqrt{x}\) represents the cube root of \(x\), which is a number that, when multiplied by itself three times, gives the original number \(x\). In mathematical terms, it is written as:
\(\sqrt[3]{x} = y \quad \text{if and only if} \quad y^3 = x\)
The cube root function is denoted by \(\sqrt[3]{x}\) or \(x^{\frac{1}{3}}\). This function is defined for all real numbers, meaning that you can take the cube root of any real number, positive, negative, or zero.
Key Properties of the Cube Root Function
- Domain and Range: The domain and range of the cube root function are both all real numbers \((-\infty, \infty)\).
- Odd Function: The cube root function is an odd function, which means that \(\sqrt[3]{-x} = -\sqrt[3]{x}\).
- One-to-One: The cube root function is one-to-one, ensuring that each input corresponds to exactly one output, which makes it invertible.
- Continuity and Smoothness: The cube root function is continuous and smooth (differentiable) everywhere on its domain.
Basic Calculation Steps
- Identify the value of \(x\).
- Find the number \(y\) such that \(y^3 = x\).
- The value of \(y\) is \(\sqrt[3]{x}\).
Special Cases
- \(\sqrt[3]{0} = 0\): The cube root of zero is zero.
- \(\sqrt[3]{1} = 1\): The cube root of one is one.
- \(\sqrt[3]{-1} = -1\): The cube root of negative one is negative one.
Examples
| Expression | Calculation | Result |
|---|---|---|
| \(\sqrt[3]{8}\) | Find \(y\) such that \(y^3 = 8\) | \(y = 2\) because \(2^3 = 8\) |
| \(\sqrt[3]{-27}\) | Find \(y\) such that \(y^3 = -27\) | \(y = -3\) because \((-3)^3 = -27\) |
| \(\sqrt[3]{64}\) | Find \(y\) such that \(y^3 = 64\) | \(y = 4\) because \(4^3 = 64\) |
Mathematical Properties of 3 √x
The expression \( \sqrt[3]{x} \), also known as the cube root of \( x \), has several important mathematical properties. Understanding these properties is essential for working with cube roots in various mathematical contexts.
1. Definition
The cube root of a number \( x \) is a value that, when raised to the power of 3, gives \( x \). Mathematically, if \( y = \sqrt[3]{x} \), then \( y^3 = x \).
2. Domain and Range
- Domain: The domain of the cube root function is all real numbers (\( \mathbb{R} \)). This is because any real number has a real cube root.
- Range: The range of the cube root function is also all real numbers (\( \mathbb{R} \)).
3. Properties
- Odd Function: The cube root function is an odd function, which means \( \sqrt[3]{-x} = -\sqrt[3]{x} \).
- Monotonicity: The cube root function is monotonically increasing, meaning it is always increasing and never decreases.
- Simplification: The cube root of a product is the product of the cube roots: \( \sqrt[3]{ab} = \sqrt[3]{a} \cdot \sqrt[3]{b} \).
- Quotient Rule: The cube root of a quotient is the quotient of the cube roots: \( \sqrt[3]{\frac{a}{b}} = \frac{\sqrt[3]{a}}{\sqrt[3]{b}} \).
- Power Rule: The cube root of a number raised to a power can be simplified using \( \sqrt[3]{a^n} = a^{\frac{n}{3}} \).
4. Examples
Here are a few examples to illustrate the properties:
- \( \sqrt[3]{8} = 2 \) because \( 2^3 = 8 \).
- \( \sqrt[3]{-27} = -3 \) because \( (-3)^3 = -27 \).
- \( \sqrt[3]{1} = 1 \) because \( 1^3 = 1 \).
- \( \sqrt[3]{64} = 4 \) because \( 4^3 = 64 \).
5. Graphical Representation
The graph of the cube root function \( y = \sqrt[3]{x} \) is a curve that passes through the origin (0,0) and extends infinitely in both directions. It is symmetric with respect to the origin, reflecting its odd function property.
The curve gradually increases as \( x \) increases and gradually decreases as \( x \) decreases. It appears flatter near the origin and steeper as the value of \( x \) moves away from zero.
6. Real-World Applications
The cube root function is used in various real-world applications, such as determining the side length of a cube given its volume, solving cubic equations, and in fields such as physics and engineering where volume and density calculations are essential.
Examples and Calculations
To better understand the concept of the cube root of x, denoted as \( \sqrt[3]{x} \), let’s go through some detailed examples and calculations. These examples will help illustrate how to find the cube root of various numbers and apply this operation in different contexts.
Example 1: Calculating the Cube Root of a Perfect Cube
Consider the number 27. Since 27 is a perfect cube, its cube root can be found easily:
\[
\sqrt[3]{27} = 3 \quad \text{because} \quad 3^3 = 27
\]
Example 2: Calculating the Cube Root of a Non-Perfect Cube
For a number that is not a perfect cube, such as 20, we can approximate the cube root. One common method is to use a calculator or estimate manually.
Using a calculator, we find:
\[
\sqrt[3]{20} \approx 2.714
\]
Example 3: Applying the Cube Root in Real-World Problems
Cube roots are often used in volume calculations where one dimension is unknown. For instance, if we know the volume of a cube and need to find the length of one side:
Given a cube with volume 64 cubic units, the length of each side is:
\[
\sqrt[3]{64} = 4 \quad \text{because} \quad 4^3 = 64
\]
Example 4: Calculating with Cube Roots in Equations
Sometimes, cube roots are part of algebraic equations. Consider solving the equation for \( x \):
\[
x^3 = 125
\]
To find \( x \), we take the cube root of both sides:
\[
x = \sqrt[3]{125} = 5 \quad \text{because} \quad 5^3 = 125
\]
Table of Common Cube Roots
| Number | Cube Root |
|---|---|
| 1 | \(1\) |
| 8 | \(2\) |
| 27 | \(3\) |
| 64 | \(4\) |
| 125 | \(5\) |
Steps for Manual Estimation
To manually estimate the cube root of a number like 50, follow these steps:
- Identify two perfect cubes between which the number lies. For 50, it lies between \( \sqrt[3]{27} = 3 \) and \( \sqrt[3]{64} = 4 \).
- Make an initial guess, say 3.5, and cube it: \[ 3.5^3 = 42.875 \] Since 42.875 is less than 50, try a higher number, like 3.7: \[ 3.7^3 = 50.653 \]
- Since 50.653 is very close to 50, we refine our guess to about 3.68 for a more accurate cube root.
Conclusion
Calculating the cube root of a number involves either exact calculation for perfect cubes or approximation for non-perfect cubes. Understanding these methods is essential for solving various mathematical and real-world problems involving cube roots.

Graphical Representation of 3 √x
The function \( y = \sqrt[3]{x} \) represents the cube root of \( x \). This function is defined for all real numbers \( x \) and is an odd function, meaning that it is symmetric with respect to the origin. Below, we explore the key features and steps to graph \( y = \sqrt[3]{x} \).
Key Features:
- Domain: All real numbers (\( -\infty < x < \infty \))
- Range: All real numbers (\( -\infty < y < \infty \))
- Intercepts: The graph passes through the origin (0,0).
- Symmetry: The function is symmetric about the origin.
- End Behavior: As \( x \to \infty \), \( y \to \infty \); and as \( x \to -\infty \), \( y \to -\infty \).
Steps to Graph \( y = \sqrt[3]{x} \):
- Identify Key Points: Plot key points such as (-8, -2), (-1, -1), (0, 0), (1, 1), and (8, 2).
- Plot Symmetric Points: Because the function is symmetric about the origin, for every point (x, y), there is a point (-x, -y).
- Draw the Curve: Connect the points smoothly, noting that the graph will flatten near the origin and steepen as \( |x| \) increases.
Graph of \( y = \sqrt[3]{x} \):
Below is a graphical representation of the function \( y = \sqrt[3]{x} \).
Detailed Analysis:
| x | y = \( \sqrt[3]{x} \) |
|---|---|
| -8 | -2 |
| -1 | -1 |
| 0 | 0 |
| 1 | 1 |
| 8 | 2 |
Using these points, we can draw an accurate graph of \( y = \sqrt[3]{x} \). Notice that as \( x \) increases, \( y \) also increases, and similarly, as \( x \) decreases, \( y \) decreases. The curve passes through the origin and demonstrates a smooth, continuous increase.
Interactive Graph:
For a more interactive experience, you can use graphing tools like Desmos or GeoGebra to visualize and manipulate the graph of \( y = \sqrt[3]{x} \).
Applications in Real-World Problems
The cube root function \( y = \sqrt[3]{x} \) has various applications in real-world problems across different fields. Here, we explore some of these applications in detail.
1. Volume and Surface Area in Geometry:
The cube root function is useful in determining dimensions when given the volume of a cube. For instance, if the volume \( V \) of a cube is known, the length of each side \( s \) can be found using \( s = \sqrt[3]{V} \).
- Example: Given a cube with a volume of 27 cubic units, the length of each side is \( s = \sqrt[3]{27} = 3 \) units.
2. Physics and Engineering:
The cube root function is often used to describe scaling laws in physics and engineering. When scaling physical objects, the relationships between surface area, volume, and linear dimensions often involve cube roots.
- Example: In material science, the strength of a beam scales with its cross-sectional area. If the cross-sectional area is increased by a factor of \( k^2 \), the length must be scaled by \( k \) to maintain proportionality. The cube root helps in determining the new dimensions.
3. Chemistry:
In chemistry, the cube root function is used in the context of molar volumes and densities of substances. The molar volume of a substance can be related to the cube root of its molar mass.
- Example: The molar volume \( V_m \) of a substance can be found using \( V_m = \sqrt[3]{M} \), where \( M \) is the molar mass.
4. Economics:
The cube root function can be applied in economics to model diminishing returns and scale economies. It helps in understanding how different factors of production scale with output.
- Example: When analyzing production functions, if the output increases by a cube root of the input factor, it suggests diminishing marginal returns. For instance, doubling the input may result in a less than double increase in output, represented by \( y = \sqrt[3]{x} \).
5. Environmental Science:
In environmental science, the cube root function can describe phenomena such as the dispersion of pollutants. The concentration of a pollutant may decrease with the cube root of the distance from the source.
- Example: If a pollutant spreads in a manner that its concentration \( C \) decreases with distance \( d \) from the source, it can be modeled as \( C = k \sqrt[3]{d} \), where \( k \) is a constant.
The cube root function \( y = \sqrt[3]{x} \) thus finds utility in diverse real-world problems, illustrating its importance in various scientific and practical contexts.
Common Mistakes and How to Avoid Them
Understanding and working with the cube root function \( y = \sqrt[3]{x} \) can be challenging. Here, we outline common mistakes and provide tips on how to avoid them.
1. Misinterpreting the Domain:
Mistake: Assuming that the cube root function is only defined for non-negative numbers.
How to Avoid: Remember that the cube root function \( \sqrt[3]{x} \) is defined for all real numbers, including negative numbers. Unlike the square root function, the cube root of a negative number is also negative.
- Example: \( \sqrt[3]{-8} = -2 \).
2. Incorrectly Simplifying Expressions:
Mistake: Incorrectly simplifying expressions involving cube roots, such as assuming \( \sqrt[3]{a \cdot b} = \sqrt[3]{a} \cdot \sqrt[3]{b} \) is always valid.
How to Avoid: Use properties of cube roots correctly. The property \( \sqrt[3]{a \cdot b} = \sqrt[3]{a} \cdot \sqrt[3]{b} \) is valid, but be careful with negative numbers and ensure proper application.
3. Confusing Cube Roots with Square Roots:
Mistake: Confusing the properties and behavior of cube roots with those of square roots.
How to Avoid: Clearly distinguish between the two. For instance, while \( \sqrt{x^2} = |x| \), \( \sqrt[3]{x^3} = x \). Understand that the cube root function returns the original number, whether positive or negative.
4. Graphing Errors:
Mistake: Incorrectly graphing the function by not accounting for the symmetry and behavior at negative values of \( x \).
How to Avoid: Ensure to plot points for both positive and negative values of \( x \). Remember the graph of \( y = \sqrt[3]{x} \) is symmetric about the origin and passes through key points like (-8, -2), (0, 0), and (8, 2).
- Tip: Plot additional points and use a smooth curve to connect them, reflecting the correct behavior of the function.
5. Incorrect Application in Real-World Problems:
Mistake: Misapplying the cube root function in problems involving volume, area, and scaling laws.
How to Avoid: Understand the context and ensure the correct interpretation of the cube root in the given scenario. For example, in geometry, use \( s = \sqrt[3]{V} \) to find the side length of a cube from its volume.
- Example: For a volume of 125 cubic units, the side length \( s = \sqrt[3]{125} = 5 \) units.
6. Algebraic Mistakes:
Mistake: Making algebraic errors while solving equations involving cube roots, such as incorrectly isolating the cube root term.
How to Avoid: Follow systematic steps to isolate the cube root term. Raise both sides of the equation to the power of 3 to eliminate the cube root correctly.
- Example: To solve \( \sqrt[3]{x} = 4 \), cube both sides: \( (\sqrt[3]{x})^3 = 4^3 \), giving \( x = 64 \).
By recognizing these common mistakes and understanding how to avoid them, you can work more effectively with the cube root function \( y = \sqrt[3]{x} \) and apply it accurately in various mathematical and real-world contexts.
Advanced Topics Related to 3 √x
The cube root function \( y = \sqrt[3]{x} \) is not only fundamental in basic algebra but also plays a significant role in more advanced mathematical concepts and applications. This section explores some of the advanced topics related to \( \sqrt[3]{x} \).
1. Complex Numbers and Cube Roots:
While the cube root of a real number is straightforward, the cube root of a complex number involves understanding the principal root and the use of polar coordinates.
- Complex Cube Roots: For a complex number \( z = re^{i\theta} \), the cube roots are given by: \[ \sqrt[3]{z} = \sqrt[3]{r} \left( e^{i\frac{\theta}{3}} + e^{i\frac{\theta + 2\pi}{3}} + e^{i\frac{\theta + 4\pi}{3}} \right) \] This shows the three distinct cube roots of a complex number.
2. Solving Cubic Equations:
Cube roots are essential in solving cubic equations. The general cubic equation \( ax^3 + bx^2 + cx + d = 0 \) can be solved using methods such as Cardano's formula, which involves cube roots.
- Cardano's Formula: For the cubic equation \( x^3 + px + q = 0 \), the solution involves: \[ x = \sqrt[3]{-\frac{q}{2} + \sqrt{\left(\frac{q}{2}\right)^2 + \left(\frac{p}{3}\right)^3}} + \sqrt[3]{-\frac{q}{2} - \sqrt{\left(\frac{q}{2}\right)^2 + \left(\frac{p}{3}\right)^3}} \] This utilizes the concept of cube roots to find the roots of the cubic equation.
3. Transformations and Symmetry:
The function \( y = \sqrt[3]{x} \) exhibits interesting transformations and symmetry properties which are useful in advanced calculus and analysis.
- Symmetry: The cube root function is symmetric with respect to the origin, i.e., it is an odd function: \[ \sqrt[3]{-x} = -\sqrt[3]{x} \] This symmetry is important in integral calculus and solving differential equations.
- Transformation: The behavior of \( y = \sqrt[3]{ax + b} \) can be analyzed by translating and scaling the basic cube root graph, which has applications in various fields such as signal processing and data transformation.
4. Application in Calculus:
The cube root function appears frequently in calculus, particularly in integrals and derivatives.
- Derivatives: The derivative of \( y = \sqrt[3]{x} \) is given by: \[ \frac{d}{dx} \left( \sqrt[3]{x} \right) = \frac{1}{3} x^{-2/3} \] This derivative is used in solving problems related to rates of change and optimization.
- Integrals: The integral of the cube root function is: \[ \int \sqrt[3]{x} \, dx = \frac{3}{4} x^{4/3} + C \] This integral finds application in areas such as physics and engineering where accumulation functions are analyzed.
5. Fractals and Chaos Theory:
The cube root function and its transformations are used in the study of fractals and chaos theory, where self-similarity and complex patterns emerge from simple equations.
- Example: The Mandelbrot set and Julia sets, which are fundamental in fractal geometry, can involve transformations that include cube roots and other nonlinear functions.
By exploring these advanced topics, we can appreciate the depth and breadth of applications and implications of the cube root function \( y = \sqrt[3]{x} \) in higher mathematics and its numerous real-world applications.
Frequently Asked Questions (FAQs)
What does \( 3 \sqrt{x} \) mean?
The expression \( 3 \sqrt{x} \) represents the cube root of \( x \), multiplied by 3.
How do you simplify \( 3 \sqrt{x} \)?
To simplify \( 3 \sqrt{x} \), you can rewrite it as \( 3x^{1/3} \).
What are the properties of \( 3 \sqrt{x} \)?
- It is defined for non-negative values of \( x \).
- It is an odd function.
- As \( x \) increases, \( 3 \sqrt{x} \) also increases.
How can \( 3 \sqrt{x} \) be graphically represented?
The graph of \( 3 \sqrt{x} \) resembles a curve that starts from the origin (0,0) and increases as \( x \) increases.
What are some real-world applications of \( 3 \sqrt{x} \)?
It can be used in scenarios involving volume calculations, growth rates, and engineering designs where cubic relationships are involved.
What mistakes should be avoided when dealing with \( 3 \sqrt{x} \)?
- Confusing it with \( \sqrt[3]{x} \), which represents the cube root of \( x \).
- Forgetting that it is a cube root function, not a square root function.
Are there advanced topics related to \( 3 \sqrt{x} \)?
Yes, advanced topics include higher-dimensional extensions, applications in calculus and differential equations, and complex number interpretations.
Can you summarize \( 3 \sqrt{x} \)?
In summary, \( 3 \sqrt{x} \) is the cube root of \( x \), multiplied by 3. It has specific properties and applications in various fields.
Summary and Conclusion
In this comprehensive guide on the expression \( \sqrt[3]{x} \), we have explored various facets of this mathematical concept. Below is a detailed summary of the key points covered:
- Introduction to 3 √x: We began with an introduction to the cube root function, defining it as the value that, when cubed, returns the original number. This function is denoted as \( \sqrt[3]{x} \) or \( x^{1/3} \).
- Definition and Basic Concepts: The cube root of a number \( x \) is a number \( y \) such that \( y^3 = x \). It is important in solving equations where the variable is cubed.
- Mathematical Properties of 3 √x: Key properties include:
- The cube root function is the inverse of the cubic function.
- The function is defined for all real numbers.
- \( \sqrt[3]{x} \) is an odd function, meaning \( \sqrt[3]{-x} = -\sqrt[3]{x} \).
- Examples and Calculations: Practical examples demonstrated how to calculate the cube root of various numbers, both positive and negative. For instance, \( \sqrt[3]{27} = 3 \) and \( \sqrt[3]{-8} = -2 \).
- Graphical Representation of 3 √x: The graph of \( \sqrt[3]{x} \) was discussed, showing its continuous and smooth nature. It passes through the origin (0,0) and is symmetric with respect to the origin.
- Applications in Real-World Problems: We highlighted several applications, such as solving volume problems in geometry, physics, and engineering where cube roots naturally arise.
- Common Mistakes and How to Avoid Them: Some common errors include confusing cube roots with square roots and misunderstanding the properties of cube roots. Tips were provided to avoid these mistakes.
- Advanced Topics Related to 3 √x: This section covered more complex applications and extensions, such as the use of cube roots in polynomial equations and their role in higher mathematics.
- Frequently Asked Questions (FAQs): Common queries were addressed, providing clarifications and further insights into the cube root function.
In conclusion, the expression \( \sqrt[3]{x} \) is a fundamental concept with wide-ranging applications in various fields of mathematics and science. By understanding its properties, graphical representation, and practical uses, one can gain a deeper appreciation of its significance and utility.
Đơn Giản Hóa Biểu Thức Căn Bậc Hai: Căn Bậc Hai Của x^5
READ MORE:
Giới hạn khi x tiến tới 9: (9-x)/(3-căn bậc hai của x)