Simplify the Square Root of 8: A Step-by-Step Guide

The square root of 8 can be simplified by expressing it in terms of its prime factors and simplifying the expression.

Step-by-Step Simplification Process

1. Start with the expression: \(\sqrt{8}\)
2. Factorize 8 into prime factors: \(8 = 2 \times 2 \times 2 = 2^3\)
3. Rewrite the square root using the prime factors: \(\sqrt{8} = \sqrt{2^3}\)
4. Express the exponent in a form that can be simplified: \(\sqrt{2^3} = \sqrt{2^2 \times 2}\)
5. Use the property of square roots that \(\sqrt{a \times b} = \sqrt{a} \times \sqrt{b}\): \(\sqrt{2^2 \times 2} = \sqrt{2^2} \times \sqrt{2}\)
6. Simplify \(\sqrt{2^2}\) to 2: \(\sqrt{2^2} = 2\)
7. Multiply the simplified term by the remaining square root: \(2 \times \sqrt{2}\)

Simplified Form

Therefore, the simplified form of \(\sqrt{8}\) is:

\[\sqrt{8} = 2\sqrt{2}\]

Summary

By following these steps, we have shown that the square root of 8 can be simplified to \(2\sqrt{2}\).

Simplifying square roots is a fundamental concept in mathematics that makes complex expressions more manageable. It involves expressing a square root in its simplest form, often by factoring the number inside the root into its prime components. This process is particularly useful in algebra, geometry, and higher-level math courses.

To simplify the square root of a number, follow these general steps:

1. Factor the Number: Break down the number inside the square root into its prime factors. For example, for the square root of 8, factorize 8 into \(2 \times 2 \times 2\).
2. Group the Factors: Group the prime factors into pairs. For the square root of 8, this would be \(2^2 \times 2\).
3. Simplify the Root: Take one factor from each pair out of the square root. In this case, \(\sqrt{2^2 \times 2} = 2\sqrt{2}\).

Let’s illustrate this with an example:

• Example: Simplifying \(\sqrt{8}\)
• Step 1: Factor 8 into prime factors: \(8 = 2 \times 2 \times 2 = 2^3\)
• Step 2: Rewrite the expression: \(\sqrt{8} = \sqrt{2^2 \times 2}\)
• Step 3: Simplify using the property \(\sqrt{a \times b} = \sqrt{a} \times \sqrt{b}\): \(\sqrt{2^2 \times 2} = \sqrt{2^2} \times \sqrt{2} = 2\sqrt{2}\)

Thus, the simplified form of \(\sqrt{8}\) is \(2\sqrt{2}\). By mastering these steps, you can simplify any square root expression efficiently and accurately.

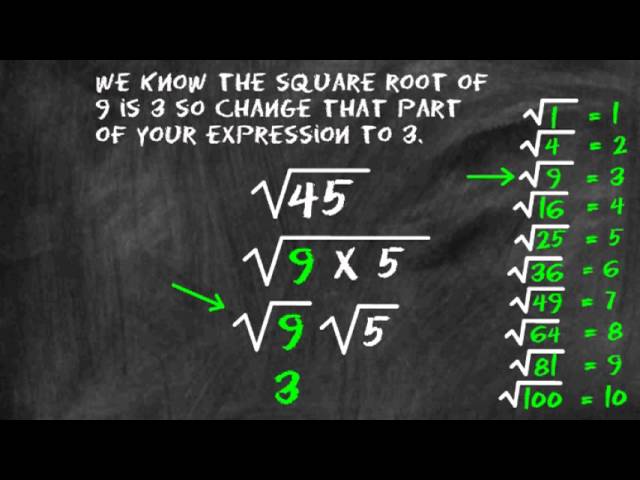
The square root of a number is a value that, when multiplied by itself, gives the original number. It is represented by the radical symbol \(\sqrt{}\). For example, the square root of 9 is 3, because \(3 \times 3 = 9\).

Square roots are fundamental in various branches of mathematics, including algebra and geometry. They help in solving quadratic equations, understanding geometric properties, and more.

Here are the key points to understand about square roots:

• Perfect Squares: These are numbers whose square roots are integers. For example, 1, 4, 9, 16, and 25 are perfect squares because their square roots are 1, 2, 3, 4, and 5, respectively.
• Non-Perfect Squares: These numbers do not have integer square roots. For example, 2, 3, 5, 8, and 10 are not perfect squares. Their square roots are irrational numbers, meaning they cannot be expressed as a simple fraction and have non-repeating, non-terminating decimal expansions.
• Simplifying Square Roots: To simplify a square root, you break down the number inside the radical into its prime factors and then simplify by pairing identical factors.

Let’s look at the example of simplifying \(\sqrt{8}\):

1. Factorize 8 into its prime factors: \(8 = 2 \times 2 \times 2 = 2^3\)
2. Rewrite the square root: \(\sqrt{8} = \sqrt{2^2 \times 2}\)
3. Use the property of square roots: \(\sqrt{a \times b} = \sqrt{a} \times \sqrt{b}\)
4. Simplify the expression: \(\sqrt{2^2 \times 2} = \sqrt{2^2} \times \sqrt{2} = 2\sqrt{2}\)

Understanding these principles makes it easier to work with square roots in various mathematical contexts, providing a foundation for more advanced topics.

The prime factorization method is a systematic way to simplify square roots by breaking down the number inside the square root into its prime factors. This method is particularly useful for simplifying complex square roots into more manageable forms.

Here are the detailed steps to simplify the square root of a number using the prime factorization method:

1. Find Prime Factors: Identify the prime factors of the number inside the square root. Prime factors are the prime numbers that multiply together to give the original number.
2. Group the Factors: Arrange the prime factors into pairs. Each pair of identical factors can be taken out of the square root.
3. Simplify the Expression: Take one number from each pair out of the square root and multiply them together. Any remaining factors stay inside the square root.

Let’s apply these steps to simplify \(\sqrt{8}\):

• Step 1: Find the prime factors of 8.
  \(8 = 2 \times 2 \times 2 = 2^3\)
• Step 2: Group the prime factors into pairs.
  \(2^3 = 2^2 \times 2\)
• Step 3: Simplify the expression.
  \(\sqrt{8} = \sqrt{2^2 \times 2} = \sqrt{2^2} \times \sqrt{2} = 2\sqrt{2}\)

Thus, by using the prime factorization method, we have simplified \(\sqrt{8}\) to \(2\sqrt{2}\). This method ensures that we can systematically break down and simplify any square root, making complex mathematical expressions easier to handle.

Simplifying the square root of 8 involves breaking down the number inside the square root into its prime factors and then simplifying the expression using properties of square roots. Here is a detailed, step-by-step process:

1. Factorize the Number: Start by finding the prime factors of 8.
   • 8 can be expressed as \(2 \times 2 \times 2\) or \(2^3\).
2. Rewrite the Square Root: Express the square root of 8 using its prime factors.
   • \(\sqrt{8} = \sqrt{2^3}\)
3. Group the Factors: Group the prime factors into pairs. In this case, we can pair two of the factors of 2.
   • \(2^3\) can be written as \(2^2 \times 2\).
4. Apply the Square Root Property: Use the property of square roots that \(\sqrt{a \times b} = \sqrt{a} \times \sqrt{b}\).
   • \(\sqrt{2^2 \times 2} = \sqrt{2^2} \times \sqrt{2}\)
5. Simplify the Expression: Simplify \(\sqrt{2^2}\) and multiply by \(\sqrt{2}\).
   • \(\sqrt{2^2} = 2\)
   • Thus, \(\sqrt{2^2} \times \sqrt{2} = 2\sqrt{2}\)

Therefore, the simplified form of \(\sqrt{8}\) is \(2\sqrt{2}\). This step-by-step method can be applied to simplify other square roots as well, providing a clear and systematic approach to handling square roots.

Understanding the properties of square roots is essential for simplifying and manipulating these mathematical expressions. Here are the key properties of square roots, explained in a detailed and positive manner:

1. Non-Negative Property:
   • The square root of a non-negative number is always non-negative. This is because a square root represents a number which, when squared, gives the original number.
   • For example, \(\sqrt{9} = 3\) because \(3^2 = 9\).
2. Product Property:
   • The square root of a product is the product of the square roots of the factors. Mathematically, this is expressed as \(\sqrt{ab} = \sqrt{a} \times \sqrt{b}\).
   • For example, \(\sqrt{36} = \sqrt{9 \times 4} = \sqrt{9} \times \sqrt{4} = 3 \times 2 = 6\).
3. Quotient Property:
   • The square root of a quotient is the quotient of the square roots of the numerator and the denominator. This property is expressed as \(\sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}}\).
   • For example, \(\sqrt{\frac{25}{4}} = \frac{\sqrt{25}}{\sqrt{4}} = \frac{5}{2} = 2.5\).
4. Power Property:
   • The square root of a power can be simplified by reducing the exponent by half. This property is expressed as \(\sqrt{a^2} = a\).
   • For example, \(\sqrt{16} = \sqrt{4^2} = 4\).
5. Sum and Difference Property:
   • Unlike products and quotients, the square root of a sum or difference is not the sum or difference of the square roots. This is important to remember when simplifying expressions.
   • For example, \(\sqrt{a + b} \neq \sqrt{a} + \sqrt{b}\). If \(a = 4\) and \(b = 5\), \(\sqrt{4 + 5} = \sqrt{9} = 3\), but \(\sqrt{4} + \sqrt{5} = 2 + \sqrt{5}\), which is not equal to 3.

These properties of square roots provide a foundation for simplifying and working with square root expressions effectively. By applying these properties, you can manipulate and simplify complex mathematical problems involving square roots.

Simplifying square roots involves breaking down the number inside the square root into its prime factors and then simplifying where possible. Here are some examples to illustrate the process:

Example 1: Simplifying the Square Root of 8

1. First, find the prime factorization of 8.
   • 8 can be expressed as 2 × 4.
   • 4 can be further factored into 2 × 2.
   • So, the prime factorization of 8 is 2 × 2 × 2, or \(2^3\).
2. Rewrite the square root of 8 using its prime factors:

   \[\sqrt{8} = \sqrt{2^3}\]

3. Since \(\sqrt{2^3} = \sqrt{2^2 \times 2}\), we can separate the perfect square (2²) from the remaining factor:

   \[\sqrt{2^3} = \sqrt{2^2 \times 2} = \sqrt{2^2} \times \sqrt{2}\]

4. Simplify the square root of the perfect square:

   \[\sqrt{2^2} = 2\]

5. Combine the simplified term with the remaining square root:

   \[\sqrt{8} = 2\sqrt{2}\]

Example 2: Simplifying the Square Root of 18

1. Find the prime factorization of 18.
   • 18 can be expressed as 2 × 9.
   • 9 can be factored into 3 × 3.
   • So, the prime factorization of 18 is 2 × 3 × 3, or \(2 \times 3^2\).
2. Rewrite the square root of 18 using its prime factors:

   \[\sqrt{18} = \sqrt{2 \times 3^2}\]

3. Separate the perfect square (3²) from the remaining factor:

   \[\sqrt{2 \times 3^2} = \sqrt{3^2} \times \sqrt{2}\]

4. Simplify the square root of the perfect square:

   \[\sqrt{3^2} = 3\]

5. Combine the simplified term with the remaining square root:

   \[\sqrt{18} = 3\sqrt{2}\]

Example 3: Simplifying the Square Root of 50

1. Find the prime factorization of 50.
   • 50 can be expressed as 2 × 25.
   • 25 can be factored into 5 × 5.
   • So, the prime factorization of 50 is 2 × 5 × 5, or \(2 \times 5^2\).
2. Rewrite the square root of 50 using its prime factors:

   \[\sqrt{50} = \sqrt{2 \times 5^2}\]

3. Separate the perfect square (5²) from the remaining factor:

   \[\sqrt{2 \times 5^2} = \sqrt{5^2} \times \sqrt{2}\]

4. Simplify the square root of the perfect square:

   \[\sqrt{5^2} = 5\]

5. Combine the simplified term with the remaining square root:

   \[\sqrt{50} = 5\sqrt{2}\]

Example 4: Simplifying the Square Root of 32

1. Find the prime factorization of 32.
   • 32 can be expressed as 2 × 16.
   • 16 can be factored into 2 × 8, and 8 can be factored into 2 × 4, and 4 can be factored into 2 × 2.
   • So, the prime factorization of 32 is 2 × 2 × 2 × 2 × 2, or \(2^5\).
2. Rewrite the square root of 32 using its prime factors:

   \[\sqrt{32} = \sqrt{2^5}\]

3. Since \(\sqrt{2^5} = \sqrt{2^4 \times 2}\), separate the perfect square (2⁴) from the remaining factor:

   \[\sqrt{2^5} = \sqrt{2^4 \times 2} = \sqrt{2^4} \times \sqrt{2}\]

4. Simplify the square root of the perfect square:

   \[\sqrt{2^4} = 2^2 = 4\]

5. Combine the simplified term with the remaining square root:

   \[\sqrt{32} = 4\sqrt{2}\]

When simplifying square roots, it's easy to make mistakes. Here are some common errors to watch out for:

• Not factoring completely: Ensure you factor the number inside the radical completely to identify all perfect squares. For instance, when simplifying \( \sqrt{8} \), recognize that \( 8 = 4 \times 2 \), where 4 is a perfect square. Hence, \( \sqrt{8} = \sqrt{4 \times 2} = 2\sqrt{2} \).
• Incorrect multiplication under the radical: Remember that \( \sqrt{a} \times \sqrt{b} = \sqrt{ab} \). For example, \( \sqrt{3} \times \sqrt{12} = \sqrt{36} = 6 \).
• Forgetting to simplify fractions: When simplifying a square root of a fraction, apply the square root to both the numerator and the denominator separately. For example, \( \sqrt{\frac{9}{16}} = \frac{\sqrt{9}}{\sqrt{16}} = \frac{3}{4} \).
• Not pulling out all perfect squares: Always extract all perfect square factors. For example, \( \sqrt{72} \) should be simplified as follows: \( \sqrt{72} = \sqrt{36 \times 2} = 6\sqrt{2} \).
• Ignoring the properties of radicals: Remember the property \( \sqrt{a^2} = a \). For example, \( \sqrt{25} = 5 \), not \( \sqrt{25} = \sqrt{5 \times 5} \).

By keeping these common mistakes in mind, you can avoid errors and ensure your square root simplifications are accurate.

Simplified square roots have a wide range of applications across various fields. Understanding how to simplify square roots can be particularly useful in the following areas:

• Geometry:

  Simplified square roots are frequently used in geometry to find the lengths of sides in right-angled triangles, areas of squares, and other shapes. For instance, the Pythagorean theorem often results in square roots that need to be simplified.

• Physics:

  In physics, simplified square roots are used in formulas involving acceleration, force, and energy. For example, the formula for kinetic energy \( E_k = \frac{1}{2}mv^2 \) may result in square root simplifications when solving for velocity or mass.

• Engineering:

  Engineers use simplified square roots in various calculations, such as determining the stress and strain on materials, electrical circuit analysis, and signal processing.

• Computer Science:

  Square roots are important in algorithms for computer graphics, cryptography, and numerical methods. Simplifying these roots can lead to more efficient and faster computations.

• Finance:

  In finance, square roots appear in calculations of standard deviation and variance, which are measures of market volatility. Simplifying these roots helps in making more precise financial predictions and analyses.

• Statistics:

  Square roots are used in statistical formulas, such as those for standard deviation and the normal distribution. Simplifying these roots is essential for accurate data interpretation and analysis.

To illustrate these applications, let's consider a few specific examples:

1. Finding the Hypotenuse of a Right Triangle:

   Given a right triangle with legs of length 6 and 8, the hypotenuse \( c \) can be found using the Pythagorean theorem:

   \[ c = \sqrt{6^2 + 8^2} = \sqrt{36 + 64} = \sqrt{100} = 10 \]

2. Calculating Standard Deviation in Statistics:

   In a dataset, if the variance \( \sigma^2 \) is 25, the standard deviation \( \sigma \) is:

   \[ \sigma = \sqrt{25} = 5 \]

3. Electrical Engineering - Resonant Frequency of an LC Circuit:

   The resonant frequency \( f \) of an LC circuit is given by:

   \[ f = \frac{1}{2\pi\sqrt{LC}} \]

   If \( L = 1 \) H and \( C = 1 \) F, then:

   \[ f = \frac{1}{2\pi\sqrt{1 \cdot 1}} = \frac{1}{2\pi} \]

By mastering the simplification of square roots, you can solve these types of problems more efficiently and accurately in your studies and professional work.

Practicing the simplification of square roots is essential for mastering this skill. Here are several problems to help you practice:

1. Simplify \( \sqrt{18} \)
   • Factor 18: \( 18 = 9 \times 2 \)
   • Apply the square root: \( \sqrt{18} = \sqrt{9 \times 2} = \sqrt{9} \times \sqrt{2} = 3\sqrt{2} \)
2. Simplify \( \sqrt{50} \)
   • Factor 50: \( 50 = 25 \times 2 \)
   • Apply the square root: \( \sqrt{50} = \sqrt{25 \times 2} = \sqrt{25} \times \sqrt{2} = 5\sqrt{2} \)
3. Simplify \( \sqrt{72} \)
   • Factor 72: \( 72 = 36 \times 2 \)
   • Apply the square root: \( \sqrt{72} = \sqrt{36 \times 2} = \sqrt{36} \times \sqrt{2} = 6\sqrt{2} \)
4. Simplify \( \sqrt{32} \)
   • Factor 32: \( 32 = 16 \times 2 \)
   • Apply the square root: \( \sqrt{32} = \sqrt{16 \times 2} = \sqrt{16} \times \sqrt{2} = 4\sqrt{2} \)
5. Simplify \( \sqrt{200} \)
   • Factor 200: \( 200 = 100 \times 2 \)
   • Apply the square root: \( \sqrt{200} = \sqrt{100 \times 2} = \sqrt{100} \times \sqrt{2} = 10\sqrt{2} \)
6. Simplify \( \sqrt{45} \)
   • Factor 45: \( 45 = 9 \times 5 \)
   • Apply the square root: \( \sqrt{45} = \sqrt{9 \times 5} = \sqrt{9} \times \sqrt{5} = 3\sqrt{5} \)

These practice problems will help you become more comfortable with the process of simplifying square roots. Remember to always look for the largest perfect square factor to minimize the number of steps needed for simplification.

When simplifying square roots, there are several advanced techniques that can be utilized to handle more complex expressions. These methods often involve the use of algebraic manipulation, properties of exponents, and creative approaches to factorization. Below are some advanced techniques:

1. Simplifying Products and Quotients of Square Roots

One useful property is that the square root of a product is the product of the square roots:

\(\sqrt{a \cdot b} = \sqrt{a} \cdot \sqrt{b}\)

Similarly, for quotients:

\(\sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}}\)

Example:

• Simplify \(\sqrt{50 \cdot 2}\):

\(\sqrt{50 \cdot 2} = \sqrt{100} = 10\)

• Simplify \(\sqrt{\frac{18}{2}}\):

\(\sqrt{\frac{18}{2}} = \sqrt{9} = 3\)

2. Rationalizing the Denominator

Rationalizing the denominator involves eliminating square roots from the denominator of a fraction. This is done by multiplying the numerator and the denominator by a suitable square root.

Example:

Simplify \(\frac{1}{\sqrt{2}}\):

• Multiply by \(\frac{\sqrt{2}}{\sqrt{2}}\):

\(\frac{1}{\sqrt{2}} \cdot \frac{\sqrt{2}}{\sqrt{2}} = \frac{\sqrt{2}}{2}\)

3. Combining Like Terms with Square Roots

Just like algebraic expressions, you can combine like terms involving square roots.

Example:

• Simplify \(2\sqrt{3} + 4\sqrt{3}\):

\(2\sqrt{3} + 4\sqrt{3} = 6\sqrt{3}\)

• Simplify \(\sqrt{18} + \sqrt{50}\):

\(\sqrt{18} = 3\sqrt{2}\) and \(\sqrt{50} = 5\sqrt{2}\)

Thus, \(\sqrt{18} + \sqrt{50} = 3\sqrt{2} + 5\sqrt{2} = 8\sqrt{2}\)

4. Using Algebraic Identities

Some algebraic identities can simplify the process of working with square roots.

Example:

• Using the identity \((a+b)^2 = a^2 + 2ab + b^2\) to simplify expressions:

Simplify \(\sqrt{4 + 4\sqrt{3} + 3}\):

\(\sqrt{4 + 4\sqrt{3} + 3} = \sqrt{(2 + \sqrt{3})^2} = 2 + \sqrt{3}\)

5. Handling Higher-Order Roots

Sometimes, you may encounter roots that are not square roots, such as cube roots. The methods are similar, but they involve different exponents.

Example:

• Simplify \(\sqrt[3]{27x^6}\):

\(\sqrt[3]{27x^6} = 3x^2\)

6. Simplifying Nested Radicals

Nested radicals are expressions where a square root contains another square root. These can often be simplified using algebraic methods or known formulas.

Example:

• Simplify \(\sqrt{6 + \sqrt{20}}\):

Notice that \(\sqrt{20} = 2\sqrt{5}\), so the expression becomes \(\sqrt{6 + 2\sqrt{5}}\).

Using algebraic techniques, this can often be simplified further depending on the context.

In this article, we explored various aspects of simplifying square roots, focusing specifically on the square root of 8. By understanding the process and applying different techniques, we can simplify complex expressions to their simplest forms. Let's summarize the key points discussed:

• The square root of 8 simplifies to \(2\sqrt{2}\), as we broke down 8 into its prime factors and applied the properties of square roots.
• We utilized the prime factorization method, identifying that \(8 = 2^3\), and simplified \( \sqrt{8} \) accordingly.
• Common mistakes to avoid include not fully breaking down the factors and incorrectly applying the properties of square roots.
• Applications of simplified square roots span across various fields including geometry, physics, and engineering, highlighting the practical importance of mastering this skill.

By practicing these methods and being aware of common pitfalls, you can confidently simplify any square root expression. The ability to simplify square roots is a fundamental mathematical skill that will aid in solving more complex problems efficiently.

We hope this guide has provided a clear and thorough understanding of simplifying square roots, empowering you to apply these techniques in your mathematical endeavors.