How to Simplify the Square Root of 48: A Step-by-Step Guide

Simplifying the square root of 48 involves expressing it in its simplest radical form. Here’s a step-by-step guide on how to do it:

Step-by-Step Simplification

1. Find the prime factorization of 48.

   The prime factors of 48 are:

   • 48 = 2 × 24
   • 24 = 2 × 12
   • 12 = 2 × 6
   • 6 = 2 × 3

   So, the prime factorization of 48 is:

   \(48 = 2 \times 2 \times 2 \times 2 \times 3\) or \(48 = 2^4 \times 3\)

2. Group the prime factors in pairs.

   We can pair the factors as follows:

   \(2 \times 2\) and \(2 \times 2\)

3. Take the square root of each pair of factors.

   The square root of \(2 \times 2\) is 2.

   Since we have two pairs of 2’s, the square root of \(2^4\) is:

   \(\sqrt{2^4} = 2^2 = 4\)

4. Multiply the results and include any remaining factors under the square root.

   The remaining factor is 3.

   So, the simplified form is:

   \(\sqrt{48} = 4 \times \sqrt{3}\)

Final Simplified Form

Therefore, the simplified form of the square root of 48 is:

\[\sqrt{48} = 4 \sqrt{3}\]

Square roots are fundamental concepts in mathematics that involve finding a number which, when multiplied by itself, gives the original number. For example, the square root of 16 is 4 because \(4 \times 4 = 16\). Understanding square roots helps in various areas of math, including algebra and geometry.

Here’s a detailed breakdown of the key concepts:

1. Definition of a Square Root

   The square root of a number \( n \), denoted as \( \sqrt{n} \), is a value that, when multiplied by itself, equals \( n \). Formally:

   \[ \sqrt{n} \times \sqrt{n} = n \]

2. Perfect Squares

   Perfect squares are numbers whose square roots are integers. Examples include:

   • 1 (\(1 \times 1\))
   • 4 (\(2 \times 2\))
   • 9 (\(3 \times 3\))
   • 16 (\(4 \times 4\))

3. Non-Perfect Squares

   Numbers that are not perfect squares have square roots that are irrational numbers. For example, the square root of 2 (\(\sqrt{2}\)) is approximately 1.414 and cannot be expressed as an exact fraction.

4. Simplifying Square Roots

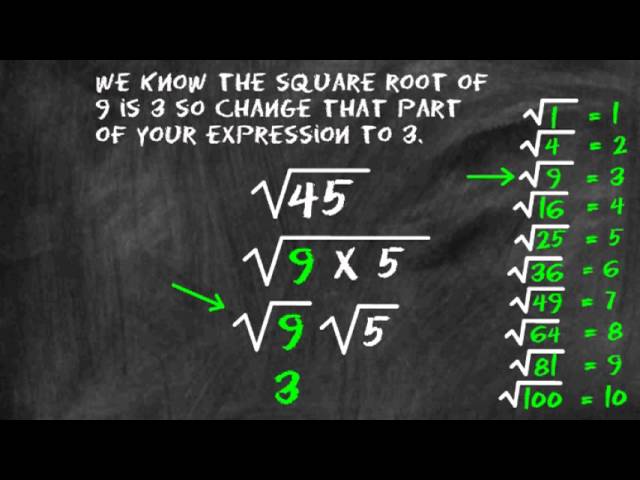
   To simplify a square root, look for perfect square factors of the number under the root. For instance, to simplify \(\sqrt{48}\), recognize that 48 can be broken down into 16 and 3, where 16 is a perfect square:

   \[ \sqrt{48} = \sqrt{16 \times 3} = \sqrt{16} \times \sqrt{3} = 4 \sqrt{3} \]

5. Square Roots and Real Numbers

   All non-negative real numbers have a non-negative square root. The square root of a negative number involves imaginary numbers (not covered here).

Understanding these basics is crucial for simplifying square roots and solving related mathematical problems efficiently.

The prime factorization of a number involves breaking it down into its prime number factors. For 48, this process helps in simplifying its square root. Here's a detailed step-by-step guide to finding the prime factors of 48:

1. Start with the Smallest Prime Number

   Begin by dividing 48 by the smallest prime number, which is 2:

   \[ 48 \div 2 = 24 \]

   Since 2 is a prime number, we record it and proceed with the quotient, 24.

2. Continue Dividing by 2

   Divide 24 by 2 again:

   \[ 24 \div 2 = 12 \]

   Record the 2 and continue with the quotient, 12.

3. Repeat the Division

   Divide 12 by 2:

   \[ 12 \div 2 = 6 \]

   Record another 2 and proceed with 6.

4. Divide Once More

   Divide 6 by 2:

   \[ 6 \div 2 = 3 \]

   Record one more 2. Now, we are left with 3, which is a prime number.

5. Finish with the Last Prime Number

   Since 3 is a prime number, we stop the division process here.

Thus, the prime factorization of 48 is:

\[ 48 = 2 \times 2 \times 2 \times 2 \times 3 \] or \[ 48 = 2^4 \times 3 \]

Using the prime factorization, we can now simplify the square root of 48 more easily. Recognizing the prime factors allows us to pair them, aiding in the simplification process.

After finding the prime factorization of 48, the next step in simplifying its square root is grouping and pairing these prime factors. This step is crucial because it allows us to simplify the square root by taking pairs of factors out of the radical. Here’s how you can do it:

1. List the Prime Factors

   From the prime factorization of 48, we have:

   \[ 48 = 2 \times 2 \times 2 \times 2 \times 3 \] or \[ 48 = 2^4 \times 3 \]

   These are the factors we will group.

2. Pair the Prime Factors

   Group the prime factors into pairs. Each pair of the same number under a square root can be simplified to a single factor outside the square root. For 48, we group the factors as follows:

   \[ 2 \times 2 \] and \[ 2 \times 2 \]

   This leaves us with one unpaired factor:

   \[ 3 \]

3. Apply the Pairing Rule

   According to the square root property, \(\sqrt{a \times b} = \sqrt{a} \times \sqrt{b}\). For each pair of the same number, you can take one of those numbers out of the square root. Thus, for the pairs of 2’s:

   \[ \sqrt{2 \times 2} = 2 \] and \[ \sqrt{2 \times 2} = 2 \]

   We now have two 2’s outside the square root.

4. Combine the Factors Outside the Square Root

   Multiply the numbers outside the square root together. For the two pairs of 2’s, this gives:

   \[ 2 \times 2 = 4 \]

   The remaining factor inside the square root is 3.

5. Write the Simplified Form

   Combining the factors, we get:

   \[ \sqrt{48} = 4 \sqrt{3} \]

   This is the simplified form of the square root of 48.

By grouping and pairing the prime factors, we can easily simplify the square root of any number, turning complex expressions into simpler forms.

To simplify the square root of 48, we need to use the prime factorization method. Once we have the prime factors, we can apply the square root to each pair of prime factors. Here is the step-by-step process:

1. Prime factorize 48: \( 48 = 2 \times 24 = 2 \times 2 \times 12 = 2 \times 2 \times 2 \times 6 = 2 \times 2 \times 2 \times 2 \times 3 \). Therefore, the prime factorization of 48 is \( 2^4 \times 3 \).
2. Group the prime factors into pairs: In the prime factorization \( 2^4 \times 3 \), we can group the four 2s into two pairs of 2s.
3. Apply the square root to each pair: According to the properties of square roots, the square root of a product is equal to the product of the square roots. Thus:
   • \( \sqrt{48} = \sqrt{2^4 \times 3} \)
   • \( = \sqrt{2^4} \times \sqrt{3} \)
   • \( = \sqrt{(2^2)^2} \times \sqrt{3} \)
   • \( = 2^2 \times \sqrt{3} \)
   • \( = 4 \times \sqrt{3} \)
4. Combine the simplified terms: After applying the square root to each pair, we get the simplified form \( 4 \sqrt{3} \).

Therefore, the simplified form of \( \sqrt{48} \) is \( 4 \sqrt{3} \).

To simplify the square root of 48, we first need to perform prime factorization. The number 48 can be expressed as the product of its prime factors:

\[
48 = 2 \times 2 \times 2 \times 2 \times 3 = 2^4 \times 3^1
\]

We can then group the prime factors into pairs:

• First pair: \(2 \times 2\)
• Second pair: \(2 \times 2\)
• Remaining factor: \(3\)

Applying the square root to each pair and to the remaining factor, we get:

\[
\sqrt{48} = \sqrt{2^4 \times 3} = \sqrt{(2^2)^2 \times 3} = \sqrt{(2^2)^2} \times \sqrt{3}
\]

Since \(\sqrt{(2^2)^2} = 2^2 = 4\), we can simplify the expression further:

\[
\sqrt{48} = 4 \sqrt{3}
\]

Thus, the simplified form of the square root of 48 is:

\[
\boxed{4 \sqrt{3}}
\]

Now that we have identified the prime factors and paired them, we can write the final simplified form of the square root of 48. Let's go through the steps:

1. Factorize the number:

   We previously determined that the prime factorization of 48 is:

   \(48 = 2^4 \times 3\)

2. Pair the factors:

   Group the prime factors into pairs of two:

   \(2^4\) can be paired as \((2^2) \times (2^2)\).

3. Apply the square root to each pair:

   Since the square root of a squared number is the base number:

   \(\sqrt{2^4} = \sqrt{(2^2) \times (2^2)} = 2 \times 2 = 4\)

4. Combine with remaining factors:

   The remaining factor, which does not have a pair, stays under the radical sign:

   \(\sqrt{48} = \sqrt{(2^4) \times 3} = 4 \sqrt{3}\)

Thus, the final simplified form of the square root of 48 is:

\(\sqrt{48} = 4 \sqrt{3}\)

This is the simplest radical form of the square root of 48.

To better understand how to simplify square roots, let's look at a few similar examples:

• Example 1: Simplifying √75
  1. Find the prime factors of 75: \(75 = 3 \times 5 \times 5\).
  2. Group the factors into pairs: \(\sqrt{75} = \sqrt{3 \times 5^2}\).
  3. Take the square root of each pair: \(\sqrt{3} \times \sqrt{5^2} = 5\sqrt{3}\).
  4. Simplified form: \(\sqrt{75} = 5\sqrt{3}\).
• Example 2: Simplifying √32
  1. Find the prime factors of 32: \(32 = 2 \times 2 \times 2 \times 2 \times 2\).
  2. Group the factors into pairs: \(\sqrt{32} = \sqrt{(2 \times 2) \times (2 \times 2) \times 2}\).
  3. Take the square root of each pair: \(\sqrt{(2 \times 2)} \times \sqrt{(2 \times 2)} \times \sqrt{2} = 2 \times 2 \times \sqrt{2} = 4\sqrt{2}\).
  4. Simplified form: \(\sqrt{32} = 4\sqrt{2}\).
• Example 3: Simplifying √50
  1. Find the prime factors of 50: \(50 = 2 \times 5 \times 5\).
  2. Group the factors into pairs: \(\sqrt{50} = \sqrt{2 \times 5^2}\).
  3. Take the square root of each pair: \(\sqrt{2} \times \sqrt{5^2} = 5\sqrt{2}\).
  4. Simplified form: \(\sqrt{50} = 5\sqrt{2}\).
• Example 4: Simplifying √72
  1. Find the prime factors of 72: \(72 = 2 \times 2 \times 2 \times 3 \times 3\).
  2. Group the factors into pairs: \(\sqrt{72} = \sqrt{(2 \times 2) \times 2 \times (3 \times 3)}\).
  3. Take the square root of each pair: \(\sqrt{(2 \times 2)} \times \sqrt{(3 \times 3)} \times \sqrt{2} = 2 \times 3 \times \sqrt{2} = 6\sqrt{2}\).
  4. Simplified form: \(\sqrt{72} = 6\sqrt{2}\).

By practicing these examples, you can develop a stronger understanding of simplifying square roots and apply the same steps to other numbers.

Here are some practice problems to help you master simplifying square roots. Follow the steps outlined to simplify each square root. Solutions are provided for you to check your work.

Practice Problems

1. Simplify \( \sqrt{75} \)
2. Simplify \( \sqrt{200} \)
3. Simplify \( \sqrt{98} \)
4. Simplify \( \sqrt{128} \)
5. Simplify \( \sqrt{180} \)

Solutions

1. Simplify \( \sqrt{75} \):

   • Find the prime factorization of 75: \( 75 = 3 \times 5 \times 5 \)
   • Group the pairs: \( 75 = 3 \times (5^2) \)
   • Apply the square root to each pair: \( \sqrt{75} = \sqrt{3 \times 5^2} = 5\sqrt{3} \)

   Final simplified form: \( \sqrt{75} = 5\sqrt{3} \)

2. Simplify \( \sqrt{200} \):

   • Find the prime factorization of 200: \( 200 = 2 \times 2 \times 2 \times 5 \times 5 \)
   • Group the pairs: \( 200 = (2^2) \times (5^2) \times 2 \)
   • Apply the square root to each pair: \( \sqrt{200} = \sqrt{(2^2) \times (5^2) \times 2} = 2 \times 5 \sqrt{2} = 10\sqrt{2} \)

   Final simplified form: \( \sqrt{200} = 10\sqrt{2} \)

3. Simplify \( \sqrt{98} \):

   • Find the prime factorization of 98: \( 98 = 2 \times 7 \times 7 \)
   • Group the pairs: \( 98 = 2 \times (7^2) \)
   • Apply the square root to each pair: \( \sqrt{98} = \sqrt{2 \times 7^2} = 7\sqrt{2} \)

   Final simplified form: \( \sqrt{98} = 7\sqrt{2} \)

4. Simplify \( \sqrt{128} \):

   • Find the prime factorization of 128: \( 128 = 2 \times 2 \times 2 \times 2 \times 2 \times 2 \times 2 \)
   • Group the pairs: \( 128 = (2^6) \times 2 \)
   • Apply the square root to each pair: \( \sqrt{128} = \sqrt{(2^6) \times 2} = 2^3\sqrt{2} = 8\sqrt{2} \)

   Final simplified form: \( \sqrt{128} = 8\sqrt{2} \)

5. Simplify \( \sqrt{180} \):

   • Find the prime factorization of 180: \( 180 = 2 \times 2 \times 3 \times 3 \times 5 \)
   • Group the pairs: \( 180 = (2^2) \times (3^2) \times 5 \)
   • Apply the square root to each pair: \( \sqrt{180} = \sqrt{(2^2) \times (3^2) \times 5} = 2 \times 3 \sqrt{5} = 6\sqrt{5} \)

   Final simplified form: \( \sqrt{180} = 6\sqrt{5} \)

When simplifying the square root of 48, students often make several common mistakes. Being aware of these errors can help ensure accurate results. Below are some key points to remember:

• Incorrect Prime Factorization: Ensure you correctly factorize 48 into its prime factors. The correct factorization is 48 = 2 × 2 × 2 × 2 × 3.
• Ignoring Perfect Squares: Identify and use perfect squares within the factors. In this case, 2 × 2 × 2 × 2 forms a perfect square (16), and 3 remains.
• Incorrect Grouping of Factors: Make sure to group the prime factors correctly. Group pairs of the same number to pull them out from under the radical. For √48, this is √(16 × 3) = √16 × √3.
• Misapplication of Square Roots: Apply the square root to each pair of factors correctly. The square root of 16 is 4, leading to 4√3, not 2√12 or any other incorrect form.
• Omitting Simplification Steps: Do not skip steps in simplification. Ensure each step is performed methodically to avoid mistakes.
• Incorrect Final Answer: The simplified form of √48 should be 4√3. Ensure this is the form you end with after all simplifications.

By paying attention to these common mistakes, you can simplify the square root of 48 accurately and confidently.

For those looking to further their understanding of simplifying square roots and related mathematical concepts, the following resources offer comprehensive explanations, examples, and practice problems:

• Khan Academy:

  An excellent resource for video tutorials and practice exercises on simplifying square roots and other algebraic concepts. Visit the for detailed lessons.

• Math Is Fun:

  This website provides clear explanations and step-by-step guides for simplifying square roots, including multiple examples. Check out their for more information.

• BYJU's:

  Offers detailed methods for simplifying square roots, including both the prime factorization and long division methods. Visit the to explore these techniques.

• Interactive Mathematics:

  This resource provides interactive lessons and exercises to help reinforce the concepts of simplifying square roots. Explore their site for more interactive learning.

These resources should provide a solid foundation for mastering the process of simplifying square roots and help avoid common mistakes. Happy learning!