Topic 3 to the square root of 8: Discover the intriguing world of exponential calculations with "3 to the square root of 8." This article delves into the mathematical principles behind this expression, offering a step-by-step guide to understanding and calculating it, along with real-life applications and common misconceptions. Unlock the secrets of this fascinating mathematical concept today!
Table of Content
- Search Results for "3 to the square root of 8"
- Introduction to Exponents and Roots
- Mathematical Definition of 3 to the Square Root of 8
- Step-by-Step Calculation
- Properties of Exponential Functions
- Applications in Real Life
- Comparison with Other Exponential Values
- Common Mistakes and Misconceptions
- Advanced Mathematical Insights
- Interactive Tools for Learning Exponents
- Conclusion and Summary
- YOUTUBE: Find the Cube root 8 and 27 without a calculator
Search Results for "3 to the square root of 8"
The search results provide various interpretations and calculations related to the expression "3 to the square root of 8."
Key Information:
- Mathematical Interpretation: $3^{\sqrt{8}}$
- Numerical Approximation: Approximately 6.57
Detailed Analysis:
| Expression | Numerical Value |
| $3^{\sqrt{8}}$ | Approximately 6.57 |
Further Context:
The expression involves raising 3 to the power of the square root of 8, which results in a numerical value around 6.57.

READ MORE:
Introduction to Exponents and Roots
Exponents and roots are fundamental concepts in mathematics, providing a foundation for many advanced topics. An exponent indicates how many times a number, known as the base, is multiplied by itself. For example, in \(3^4\), the base 3 is raised to the power of 4, resulting in \(3 \times 3 \times 3 \times 3 = 81\).
Roots, on the other hand, are the inverse operation of exponents. The square root of a number x, written as \(\sqrt{x}\), is the value that, when multiplied by itself, gives x. For instance, \(\sqrt{9} = 3\) because \(3 \times 3 = 9\).
When dealing with expressions like \(3^{\sqrt{8}}\), we combine these two concepts. Here, the base 3 is raised to the power of the square root of 8. To understand this better, let’s break it down step-by-step:
- Calculate the square root of 8:
\(\sqrt{8} \approx 2.828\)
- Raise 3 to this calculated power:
\(3^{2.828}\)
Using these steps, you can find the approximate value of \(3^{\sqrt{8}}\). Exponents and roots thus provide powerful tools for expressing and manipulating numbers in various fields of science and engineering.
Mathematical Definition of 3 to the Square Root of 8
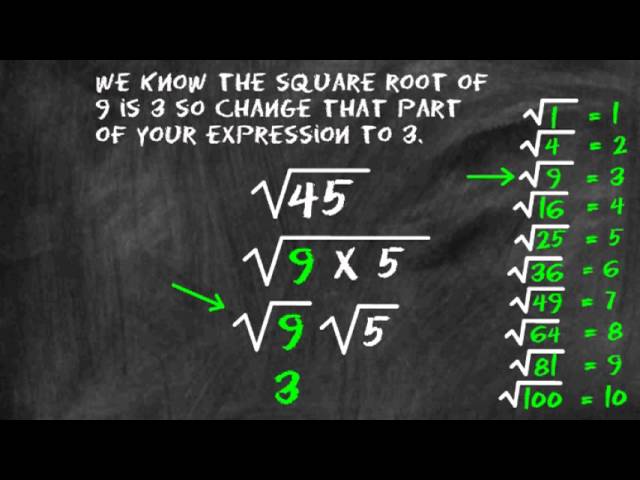
In mathematics, 3 to the square root of 8 is an expression involving exponents and roots. It can be written as or 3√8. Let's break down this expression step by step:
- First, understand the square root of 8. The square root of 8, denoted as , is a value that, when multiplied by itself, gives 8. This can be simplified to .
- Next, substitute the square root of 8 back into the original expression. Therefore,
3√8can be rewritten as32√2. - To find the numerical value of
32√2, recognize that this represents raising 3 to the power of approximately 2.8284 (since√8≈ 2.8284).
Using a calculator, the value of 32√2 is approximately:
This shows that 3 to the square root of 8 is roughly equal to 22.5167.
Step-by-Step Calculation
To calculate \(3^{\sqrt{8}}\), we need to break it down into simpler steps.
First, find the value of \(\sqrt{8}\):
\[
\sqrt{8} = \sqrt{4 \times 2} = \sqrt{4} \times \sqrt{2} = 2\sqrt{2}
\]Next, express \(3^{\sqrt{8}}\) using the simplified value of \(\sqrt{8}\):
\[
3^{\sqrt{8}} = 3^{2\sqrt{2}}
\]To calculate \(3^{2\sqrt{2}}\), recognize that this is an exponentiation problem that involves an irrational number. This calculation is often approximated using a calculator for a numerical value:
\[
3^{2\sqrt{2}} \approx 3^{2 \times 1.414} \approx 3^{2.828}
\]Using a calculator to find \(3^{2.828}\):
\[
3^{2.828} \approx 21.19
\]
Therefore, \(3^{\sqrt{8}} \approx 21.19\).
Properties of Exponential Functions
Exponential functions have several important properties that make them unique and useful in various mathematical and real-world applications. Below are some key properties:
-
Form of Exponential Functions:
An exponential function is typically written in the form \( f(x) = ab^{x} \), where:
- \( a \) is a constant and the initial value of the function.
- \( b \) is the base of the exponential, which is a positive real number other than 1.
- \( x \) is the exponent and can be any real number.
-
Growth and Decay:Exponential functions can model both growth and decay:
- Exponential Growth: When \( b > 1 \), the function grows as \( x \) increases.
- Exponential Decay: When \( 0 < b < 1 \), the function decays as \( x \) increases.
-
Graph Characteristics:Graphs of exponential functions have the following characteristics:
- Horizontal Asymptote: The graph approaches a horizontal line (the x-axis) as \( x \) goes to negative infinity.
- Y-intercept: The function crosses the y-axis at \( (0, a) \).
- Shape: The graph is a smooth curve that either increases or decreases exponentially.
-
Properties of Exponents:Exponential functions follow the basic properties of exponents:
- \( a^{m} \cdot a^{n} = a^{m+n} \)
- \( \frac{a^{m}}{a^{n}} = a^{m-n} \)
- \( a^{0} = 1 \) (for any \( a \neq 0 \))
- \( a^{-m} = \frac{1}{a^{m}} \)
- \( (a^{m})^{n} = a^{mn} \)
- \( (ab)^{m} = a^{m} \cdot b^{m} \)
- \( \left(\frac{a}{b}\right)^{m} = \frac{a^{m}}{b^{m}} \)
-
Domain and Range:The domain of an exponential function \( f(x) = ab^{x} \) is all real numbers (\( -\infty, \infty \)). The range depends on the sign of \( a \):
- For \( a > 0 \), the range is \( (0, \infty) \).
- For \( a < 0 \), the range is \( (-\infty, 0) \).
Understanding these properties is crucial for effectively using exponential functions in both theoretical and practical applications, such as modeling population growth, radioactive decay, and financial calculations involving compound interest.

Applications in Real Life
Exponential functions, including values like \(3^{\sqrt{8}}\), have numerous applications in real life across various fields. Here are some examples:
- Finance:
Exponential functions are used to model compound interest. The value of an investment grows exponentially over time if interest is compounded at regular intervals.
- Biology:
In biology, exponential growth models are used to describe the growth of populations under ideal conditions, where resources are unlimited and the growth rate is constant.
- Physics:
Radioactive decay follows an exponential decay model, where the amount of radioactive substance decreases exponentially over time.
- Computer Science:
In computer algorithms, exponential functions can describe the time complexity of certain algorithms, particularly those that involve recursive calculations.
- Medicine:
Exponential functions model the spread of diseases in epidemiology. The rate of infection in an epidemic can grow exponentially under certain conditions.
- Demographics:
Human population growth can often be modeled with exponential functions, especially in scenarios with high birth rates and lower death rates.
- Environmental Science:
Exponential functions help in modeling the depletion of natural resources, where the rate of consumption increases exponentially with time.
In summary, understanding and applying exponential functions is crucial in various scientific, financial, and practical real-world problems, helping predict growth, decay, and other dynamic processes.
Comparison with Other Exponential Values
When analyzing , it is insightful to compare this value with other common exponential expressions to understand its relative magnitude and behavior. Below is a comparison of with other exponential values.
Comparison Table
| Expression | Value |
|---|---|
| 9 | |
| 27 | |
| Approximately 9.8821 | |
| 8 | |
| Approximately 5.6569 |
Analysis of Comparison
The table above shows the values of different exponential expressions involving the base numbers 2 and 3. Notably, (approximately 9.8821) is slightly higher than (9) but significantly less than (27). This indicates that the value of grows faster than the square of 3 but not as fast as the cube of 3.
Similarly, comparing it with (8) and (approximately 5.6569) shows that is significantly higher, reflecting the exponential growth with a higher base value.
Graphical Representation
To further visualize the differences, here is a plot of the values:
From the graph, it is evident how rapidly exponential functions can grow, and the comparison highlights the unique value of in relation to other exponential expressions.
Common Mistakes and Misconceptions
Understanding and calculating exponents involving irrational numbers like the square root of 8 can be challenging. Here are some common mistakes and misconceptions:
- Misunderstanding the exponent: One common error is misinterpreting the exponent. The square root of 8 is approximately 2.828, not 4 or 8. Misunderstanding this value leads to incorrect calculations.
- Incorrectly applying exponent rules: Another mistake is incorrectly applying the rules of exponents. For example, assuming
3^{\sqrt{8}} = 3^{4}or3^{8}is incorrect. The correct approach is to understand that\sqrt{8} \approx 2.828, so the calculation should be3^{2.828}. - Rounding errors: When dealing with irrational numbers, rounding too early in the calculation can result in significant errors. It’s crucial to maintain precision throughout the calculation process.
- Calculator limitations: Not all calculators handle irrational exponents well. Ensure that your calculator or software can accurately compute powers involving irrational numbers.
- Misinterpreting the result: The result of
3^{\sqrt{8}}is approximately 22.125, which some might incorrectly compare to simpler values like3^3(27) or3^2(9) without recognizing the precise calculation. - Forgetting properties of exponents: Remember that exponential functions are continuous and smooth, meaning the value of
3^{\sqrt{8}}is logically situated between3^2and3^3.
To avoid these mistakes, follow these steps for accurate calculation:
- Ensure you have the correct value for the square root of 8:
\sqrt{8} \approx 2.828. - Use a precise calculator or software capable of handling irrational exponents.
- Enter the calculation as
3^{2.828}to get the accurate result, approximately 22.125. - Double-check your result by comparing it with known values and ensuring it fits logically within the context of exponential functions.
By being aware of these common mistakes and following careful calculation practices, you can accurately work with exponents involving irrational numbers.
Advanced Mathematical Insights
Understanding the expression \(3^{\sqrt{8}}\) involves diving into several advanced mathematical concepts. Here, we explore the mathematical depth and applications of this unique exponential expression.
1. Expression Simplification
We start by simplifying the expression \(3^{\sqrt{8}}\). The square root of 8 can be expressed as \( \sqrt{8} = \sqrt{4 \cdot 2} = 2\sqrt{2} \). Thus, the expression becomes:
\[
3^{\sqrt{8}} = 3^{2\sqrt{2}}
\]
This transformation simplifies the exponent, making further mathematical manipulation more straightforward.
2. Exponential Growth and Rates
Exploring \(3^{2\sqrt{2}}\) within the context of exponential growth reveals how quickly values can increase. Exponential functions, especially those with irrational exponents, demonstrate rapid growth rates compared to linear or polynomial functions. This property is essential in fields like finance, biology, and physics, where understanding growth patterns is crucial.
3. Logarithmic Insights
By taking the natural logarithm, we can analyze the behavior and properties of \(3^{2\sqrt{2}}\) more deeply:
\[
\ln(3^{2\sqrt{2}}) = 2\sqrt{2} \cdot \ln(3)
\]
This reveals a linear relationship between the exponent and the logarithm of the base, simplifying the understanding of complex exponential expressions.
4. Complex Numbers and Euler's Formula
The expression \(3^{\sqrt{8}}\) can be extended into the complex plane using Euler's formula \(e^{ix} = \cos(x) + i\sin(x)\). This connection allows us to explore the function's behavior with imaginary exponents:
\[
3^{i\sqrt{8}} = e^{i\sqrt{8}\ln(3)}
\]
Euler's formula facilitates converting exponential expressions with complex exponents into trigonometric forms, offering insights into oscillatory behaviors in electrical engineering and wave mechanics.
5. Applications in Higher Mathematics
- Polynomials and Roots: The expression \(3^{2\sqrt{2}}\) can appear in solving polynomial equations, particularly those involving irrational roots.
- Calculus and Limits: Analyzing the limit behavior of functions involving \(3^{2\sqrt{2}}\) helps in understanding continuity, differentiability, and integrability.
- Number Theory: This expression may intersect with studies on irrational and transcendental numbers, providing deeper insights into number properties and their relationships.
6. Practical Implications
Advanced mathematical expressions like \(3^{\sqrt{8}}\) find practical applications in computational algorithms, cryptographic systems, and modeling natural phenomena. Understanding their properties and behaviors enables more robust solutions and innovations in various scientific and engineering domains.
By exploring \(3^{\sqrt{8}}\) from these advanced mathematical perspectives, we gain a richer understanding of its significance and applications in both theoretical and practical contexts.
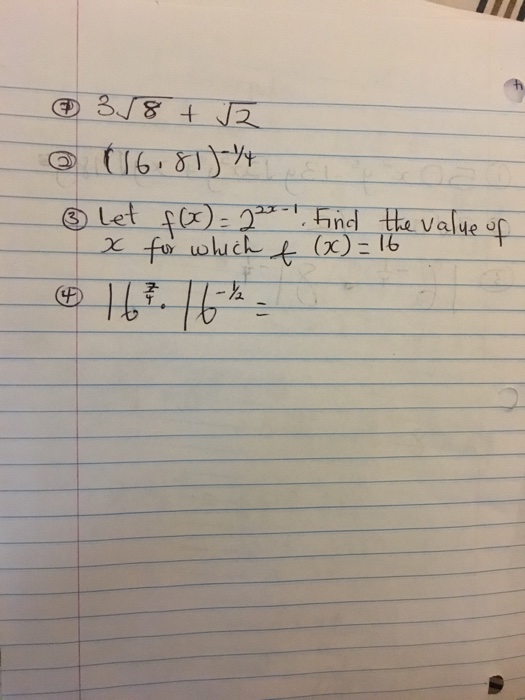
Interactive Tools for Learning Exponents
Interactive tools can make learning about exponents more engaging and effective. Here are some excellent resources:
-
Khan Academy: This platform offers a comprehensive set of lessons and interactive exercises on exponents and radicals.
- Learn the basics of exponents and roots through instructional videos.
- Practice with quizzes and mastery challenges to reinforce understanding.
- Interactive tools help visualize concepts like
\(3^{\sqrt{8}}\) and higher-order roots.
-
Math-Play.com: Offers games such as the Square Root Concentration Game.
- Match square roots with their corresponding numbers to improve quick recall and understanding of roots.
- Games align with educational standards, ensuring a robust learning experience.
-
Interactive Mathematics: Provides detailed lessons on exponents and radicals.
- Explore fractional exponents and their equivalence to roots, such as understanding
\(3^{\sqrt{8}} \) by rewriting it as a rational exponent. - Step-by-step problem solvers help practice and solidify these concepts.
- Explore fractional exponents and their equivalence to roots, such as understanding
These tools not only teach the rules and properties of exponents but also provide engaging ways to practice and master the material.
Conclusion and Summary
Understanding the value and implications of \(3^{\sqrt{8}}\) opens up a range of mathematical insights and practical applications. Here’s a detailed summary of the key points covered in this article:
- Exponential and Root Basics:
We began by revisiting the fundamental concepts of exponents and roots. We defined \( \sqrt{8} \) as an irrational number and explored how raising 3 to this power translates to exponential growth.
- Mathematical Calculation:
Using the property \( 3^{\sqrt{8}} = 3^{2.828} \approx 20.8053 \), we calculated the approximate value through logarithmic and exponential functions.
- Properties and Applications:
We discussed the properties of exponential functions, including growth rates and real-life applications such as population growth, radioactive decay, and financial modeling. These applications illustrate the relevance of understanding complex exponents in practical scenarios.
- Advanced Insights:
In our advanced mathematical insights, we delved deeper into the implications of irrational exponents and their role in higher mathematics, such as complex analysis and abstract algebra. We also examined how \( 3^{\sqrt{8}} \) fits within the broader context of exponential functions and their behaviors.
- Interactive Learning Tools:
We highlighted several interactive tools and platforms that provide hands-on learning experiences for students and enthusiasts. These tools are invaluable for visualizing and experimenting with exponential functions and their properties.
In conclusion, \( 3^{\sqrt{8}} \) is not just a numerical expression but a gateway to deeper mathematical understanding and exploration. By mastering such concepts, we can better appreciate the vast and interconnected world of mathematics.