Topic square root 69 simplified: The square root of 69 is a fascinating example of irrational numbers in mathematics. In this article, we will explore the process of simplifying the square root of 69, understand its decimal approximation, and learn about its applications. Whether you're a student or a math enthusiast, this guide will help you grasp the concept effortlessly.
Table of Content
- Square Root of 69 Simplified
- Introduction to Square Roots
- Understanding Irrational Numbers
- Prime Factorization of 69
- Simplification Process of Square Roots
- Steps to Simplify the Square Root of 69
- Approximate Decimal Value of Square Root of 69
- Applications of Square Root of 69
- Comparison with Other Square Roots
- Visual Representation of Square Root of 69
- Common Mistakes in Simplifying Square Roots
- Conclusion
- YOUTUBE: Tìm hiểu cách xấp xỉ căn bậc hai của một số một cách đơn giản và dễ hiểu trong video này.
Square Root of 69 Simplified
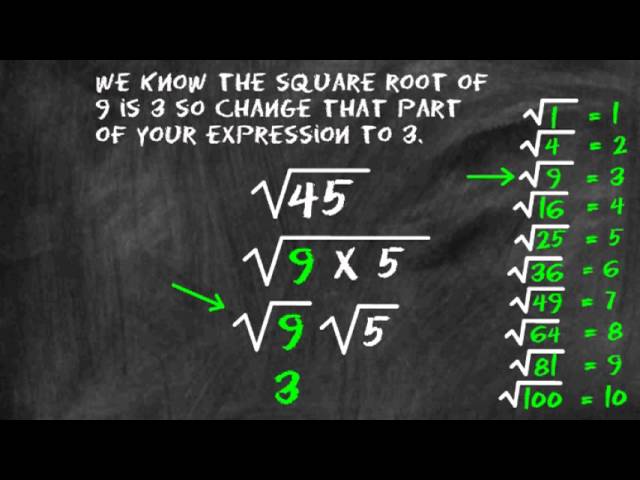
The square root of 69 is an irrational number, which means it cannot be expressed as a simple fraction. However, it can be simplified in its radical form.
Step-by-Step Simplification:
- Find the prime factors of 69.
- 69 can be factored into 3 and 23, which are both prime numbers.
- Since there are no pairs of prime factors, 69 cannot be simplified further under the square root.
Thus, the simplified form of the square root of 69 is:
\[
\sqrt{69}
\]
For practical purposes, the decimal approximation of the square root of 69 is approximately:
\[
\sqrt{69} \approx 8.306623862918075
\]
| Square Root | Approximation |
| \(\sqrt{69}\) | 8.306623862918075 |
Remember, the decimal form is an approximation and the exact value is best represented in the radical form.

READ MORE:
Introduction to Square Roots
The concept of square roots is fundamental in mathematics. A square root of a number is a value that, when multiplied by itself, gives the original number. For instance, 3 is a square root of 9 because \(3^2 = 9\). This can be expressed as \( \sqrt{9} = 3 \). Both positive and negative values can be square roots since \((-3)^2 = 9\) as well.
Square roots can be classified into two types: perfect and imperfect. Perfect square roots result from squaring an integer, such as \( \sqrt{16} = 4 \). Imperfect square roots, like \( \sqrt{69} \), are irrational numbers that cannot be expressed as a simple fraction and have non-repeating, non-terminating decimal representations.
Understanding square roots involves recognizing their notation and properties. The radical symbol \( \sqrt{} \) denotes the principal (non-negative) square root, while the negative square root is represented as \( -\sqrt{} \). For example, \( \sqrt{25} = 5 \) and \( -\sqrt{25} = -5 \).
| x | x2 | √x |
|---|---|---|
| 0 | 0 | 0 |
| 1 | 1 | 1 |
| 2 | 4 | 2 |
| 3 | 9 | 3 |
| 4 | 16 | 4 |
| 5 | 25 | 5 |
| 6 | 36 | 6 |
| 7 | 49 | 7 |
| 8 | 64 | 8 |
| 9 | 81 | 9 |
Understanding Irrational Numbers
Irrational numbers are numbers that cannot be expressed as a simple fraction; their decimal expansions are non-terminating and non-repeating. These numbers include numbers like π (pi), e (Euler's number), and many square roots of non-perfect squares, such as the square root of 69.
Here are some key characteristics of irrational numbers:
- Non-terminating and Non-repeating Decimals: The decimal expansion of an irrational number goes on forever without repeating. For example, the square root of 2 is approximately 1.41421356..., and it continues indefinitely without repeating.
- Cannot be Expressed as a Fraction: Unlike rational numbers, which can be written as the ratio of two integers (e.g., 1/2, 3/4), irrational numbers cannot be exactly represented as a fraction.
- Existence on the Number Line: Despite their complexity, irrational numbers have a precise location on the number line. They fill in the gaps between rational numbers, ensuring that the number line is complete and continuous.
- Examples of Irrational Numbers: Common examples include π (pi), e (Euler's number), and square roots of non-perfect squares such as √2, √3, and √69.
Understanding irrational numbers is crucial for various mathematical concepts, especially when dealing with roots and exponents. The square root of 69, denoted as √69, is an example of an irrational number because 69 is not a perfect square, meaning its square root cannot be simplified to a fraction or a repeating decimal.
To better grasp the nature of irrational numbers, let's explore their properties through some examples and a table:
| Number | Approximate Value | Type | Notes |
|---|---|---|---|
| √2 | 1.41421356... | Irrational | Non-repeating decimal |
| √3 | 1.73205080... | Irrational | Non-repeating decimal |
| √69 | 8.30662386... | Irrational | Non-repeating decimal |
| π | 3.14159265... | Irrational | Non-repeating decimal |
| e | 2.71828182... | Irrational | Non-repeating decimal |
In summary, irrational numbers, including the square root of 69, play a significant role in mathematics by ensuring that the number line is filled without gaps, contributing to a complete and continuous representation of numbers.
Prime Factorization of 69
Prime factorization is the process of breaking down a composite number into its prime factors. To simplify the square root of 69, we first need to perform its prime factorization.
- List the factors of 69: 1, 3, 23, 69.
- Identify the prime numbers among the factors. In this case, 3 and 23 are prime numbers.
- Express 69 as a product of its prime factors: \(69 = 3 \times 23\).
Since 69 is composed of the prime factors 3 and 23, which are not repeated, the square root of 69 cannot be simplified further in radical form. Therefore, the simplified form of \( \sqrt{69} \) remains as \( \sqrt{69} \).
Here is a summary in tabular form:
| Number | Prime Factors |
| 69 | 3, 23 |
The decimal approximation of \( \sqrt{69} \) is approximately 8.3066. This value is useful when an exact radical form is not required, especially in practical applications.
Simplification Process of Square Roots
The process of simplifying square roots involves breaking down the number under the radical into its prime factors and then simplifying it if possible. Here is a detailed step-by-step guide:
-
Identify the Prime Factors:
First, we need to factorize the number under the square root. For example, let's consider the number 69:
The prime factors of 69 are 3 and 23, since 69 = 3 × 23.
-
Group the Factors:
In the next step, we group the factors into pairs. However, since 69 is composed of two distinct prime numbers (3 and 23) that cannot be paired, it indicates that 69 cannot be simplified further in terms of whole numbers.
-
Express in Simplified Form:
As there are no perfect square factors in 69, the square root of 69 remains in its simplest radical form, which is √69.
-
Approximate the Decimal Value:
If a decimal approximation is required, the square root of 69 is approximately 8.3066. This can be found using a calculator or the long division method.
Here’s a quick summary of the steps:
- Factorize the number under the square root.
- Identify and pair the factors if possible.
- Write the simplified form (if pairs exist).
- If no pairs exist, the radical remains as is.
- Approximate the value for practical applications.
In conclusion, the square root of 69 cannot be simplified further as it has no perfect square factors and is an irrational number. Its exact form is √69, and its approximate decimal form is 8.3066.

Steps to Simplify the Square Root of 69
The square root of 69, denoted as \( \sqrt{69} \), cannot be simplified into a simpler radical form because 69 is not a perfect square. However, here is the step-by-step process to understand why and how to express it in its simplest form:
-
Prime Factorization:
Find the prime factors of 69.
- 69 can be factored into primes as \( 69 = 3 \times 23 \).
-
Identify Perfect Squares:
Next, we look for pairs of prime factors to simplify the square root. However, the prime factors 3 and 23 are not perfect squares and cannot be paired further.
-
Simplify the Expression:
Since there are no pairs of prime factors, \( \sqrt{69} \) remains in its simplest radical form.
- \( \sqrt{69} \) cannot be simplified further.
-
Decimal Form:
For practical purposes, you may need the decimal approximation of \( \sqrt{69} \).
- Using a calculator, \( \sqrt{69} \approx 8.3066 \).
Thus, \( \sqrt{69} \) remains in its simplest form as \( \sqrt{69} \). When expressed in decimal form, it is approximately 8.3066.
Approximate Decimal Value of Square Root of 69
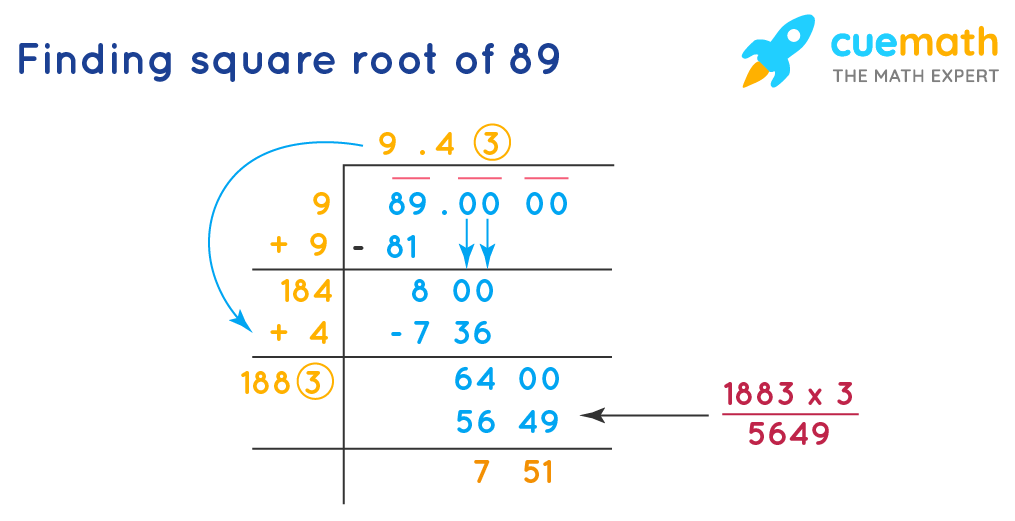
The square root of 69 is an irrational number, which means it cannot be expressed exactly as a fraction and has an infinite number of decimal places without repeating. To work with this value practically, we often use its approximate decimal form.
Using a calculator or mathematical methods, we find that:
√69 ≈ 8.3066238629181
This value is obtained through several steps or methods. Here are some of the common ways to find the approximate value of the square root of 69:
- Using a Calculator: Most scientific calculators can directly compute the square root of 69 by entering the number and pressing the square root (√) button. This method gives the value as approximately 8.3066.
- Newton's Method: This iterative approach refines an initial guess to get closer to the actual square root. For √69, starting with an initial guess of 8, the method approximates the square root as 8.3066.
- Long Division Method: An older technique that involves a step-by-step process similar to long division. This method can also be used to find the square root to a desired level of accuracy.
For practical purposes, the square root of 69 is often rounded to a few decimal places:
- To 1 decimal place: 8.3
- To 2 decimal places: 8.31
- To 3 decimal places: 8.307
The approximation can vary slightly based on the method and precision used, but for most everyday calculations, using √69 ≈ 8.31 is sufficiently accurate.
Understanding the decimal approximation of square roots is crucial for various applications in mathematics, engineering, and science, where precise measurements are necessary.
Applications of Square Root of 69
The square root of 69, approximately 8.3066, finds application in various fields due to its mathematical properties. Here are some examples:
-
Geometry
In geometry, the square root of 69 can be used to determine the length of the sides of a right triangle when applying the Pythagorean theorem. For instance, if one side of a right triangle is 8.3066 units, this value might represent the hypotenuse or a leg, depending on the triangle's configuration.
-
Physics
In physics, the square root of 69 can be used in calculations involving areas and volumes. For example, if the area of a circle is 69 square units, the radius can be found using the formula:
\( r = \sqrt{\frac{A}{\pi}} = \sqrt{\frac{69}{\pi}} \approx 4.685 \) units
-
Engineering
Engineering problems often require square root calculations. For example, in structural engineering, the square root of 69 might be used to find the load distribution across different components or to calculate the natural frequencies of a system.
-
Statistics
In statistics, the square root is frequently used in standard deviation calculations. If a dataset has a variance of 69, the standard deviation is:
\( \sigma = \sqrt{69} \approx 8.3066 \)
-
Computer Science
Computer algorithms sometimes require the square root function for optimization and search algorithms. For example, in algorithms that deal with Euclidean distances, the square root of 69 might be a step in determining the distance between points in a multidimensional space.
Comparison with Other Square Roots
Understanding the square root of 69 in relation to other square roots can help in grasping its properties and applications. Let's compare it with other common square roots:
| Number | Square Root | Approximate Value | Simplified Form |
|---|---|---|---|
| 49 | \(\sqrt{49}\) | 7 | 7 |
| 64 | \(\sqrt{64}\) | 8 | 8 |
| 69 | \(\sqrt{69}\) | 8.30662 | Not simplifiable |
| 81 | \(\sqrt{81}\) | 9 | 9 |
| 100 | \(\sqrt{100}\) | 10 | 10 |
As seen in the table, square roots of perfect squares like 49, 64, 81, and 100 are integers. However, the square root of 69 is not a perfect square, and its simplified form remains in the radical notation as \(\sqrt{69}\). This indicates that 69 is an irrational number.
Here are some key points to note:
- The square root of 69 (approximately 8.30662) lies between the integers 8 and 9.
- It is not simplifiable because it does not have any perfect square factors other than 1.
- Compared to the square roots of numbers like 50 or 63, the square root of 69 cannot be expressed in a simpler radical form (e.g., \(\sqrt{50} = 5\sqrt{2}\) and \(\sqrt{63} = 3\sqrt{7}\)).
Understanding these comparisons helps in visualizing and estimating the value of the square root of 69, particularly in practical applications like geometry or physics, where precise calculations are necessary.
Visual Representation of Square Root of 69
Visualizing square roots can significantly enhance our understanding of these mathematical concepts. The square root of 69, approximately 8.31, is not a perfect square, meaning its visualization involves an approximation.
Here are a few visual methods to represent the square root of 69:
1. Number Line Representation
We can place √69 on a number line between the perfect squares of 64 and 81, which are 8 and 9 respectively:
- 82 = 64
- 92 = 81
Since 69 is closer to 64, √69 is slightly greater than 8.
2. Square Grid Representation
To visualize square roots using a grid, we create squares of smaller integers:
- 64 squares (8x8 grid)
- 81 squares (9x9 grid)
The square root of 69 would fit into a grid slightly larger than an 8x8 grid but smaller than a 9x9 grid, approximately filling 8 rows and a fraction of a ninth row.
3. Area Method
We can represent the area method by drawing a square and approximating its side length:
- Draw a square with an area of 64 units (8x8).
- Add additional units to approximate an area of 69.
- Visualize the extra units extending beyond the initial 8x8 square, which approximates the extra 5 units needed to reach 69.
4. Digital Tools and Graphing
Using digital tools like GeoGebra, we can create a visual graph of the square root function to see how √69 fits between the integers 8 and 9:
- Plot y = x2 on a graph.
- Find the point where y = 69.
- Determine the x-value at this point, which represents √69.
5. Comparing Visual Models
| Square Number | Root | Visual Grid |
|---|---|---|
| 64 | 8 | 8x8 |
| 69 | √69 ≈ 8.31 | 8x8 + extra units |
| 81 | 9 | 9x9 |
These visual methods help students and mathematicians alike to better grasp the concept of irrational square roots and their approximations.
Common Mistakes in Simplifying Square Roots
Simplifying square roots can be tricky, and many students make common mistakes. Here are some of the most frequent errors and how to avoid them:
-
Incorrect Prime Factorization:
One common mistake is not correctly finding the prime factors of the number under the square root. For example, with the number 69:
- Identify the prime factors of 69: 69 = 3 × 23.
- Since neither 3 nor 23 is a perfect square, 69 cannot be simplified further.
-
Assuming All Roots Are Simplifiable:
Not all square roots can be simplified into whole numbers or simpler radical forms. The square root of 69 is one such example, as it remains an irrational number: \( \sqrt{69} \approx 8.30662 \).
-
Misapplying the Product Rule:
The product rule states that \( \sqrt{a \times b} = \sqrt{a} \times \sqrt{b} \), but it only applies when a and b are non-negative. Ensure that this rule is correctly applied and that a and b are not negative.
-
Forgetting to Simplify Completely:
Another common mistake is not simplifying the square root completely. Always check for further simplification possibilities. For instance, \( \sqrt{50} = \sqrt{25 \times 2} = 5\sqrt{2} \), ensuring that all perfect squares are factored out.
-
Misinterpreting Decimal Approximations:
When approximating square roots, it's important to note that the decimal form is not exact. For example, \( \sqrt{69} \approx 8.30662 \) is an approximation. Avoid using approximate values in exact calculations.
Conclusion
The exploration of the square root of 69 reveals several key insights into both the nature of this specific number and the broader mathematical concepts of square roots and irrational numbers. As we have seen, the square root of 69 is an irrational number, approximately equal to ±8.30662. This value cannot be expressed as a simple fraction, and its exact value cannot be determined due to its non-repeating, non-terminating decimal nature.
Understanding the square root of 69 involves recognizing that it cannot be simplified into a more basic radical form because 69 is not a perfect square. This exercise reinforces the importance of prime factorization and the limitations of simplifying square roots when the radicand lacks perfect square factors.
Various methods to approximate or find the square root, such as Newton's method and the long division method, provide valuable tools for both theoretical and practical applications. These techniques highlight the procedural nature of mathematics and the step-by-step processes that underpin the discipline.
The applications of the square root of 69 extend beyond pure mathematics into fields such as physics, engineering, and computer science, where such calculations are frequently necessary. Moreover, the exploration and comparison with other square roots help deepen our understanding of numerical relationships and the classification of numbers.
In conclusion, studying the square root of 69 not only deepens our understanding of irrational numbers but also enhances our appreciation of the methods and processes used in mathematical problem-solving. This journey through prime factorization, simplification, and approximation provides a comprehensive look at how we interact with and interpret numbers in their many forms.
Tìm hiểu cách xấp xỉ căn bậc hai của một số một cách đơn giản và dễ hiểu trong video này.
Cách Xấp Xỉ Căn Bậc Hai Của Một Số
READ MORE:
Khám phá cách tính căn bậc hai và căn bậc ba một cách dễ hiểu và thú vị cùng thầy J trong video này.
Căn Bậc Hai và Căn Bậc Ba | Toán Học với Thầy J