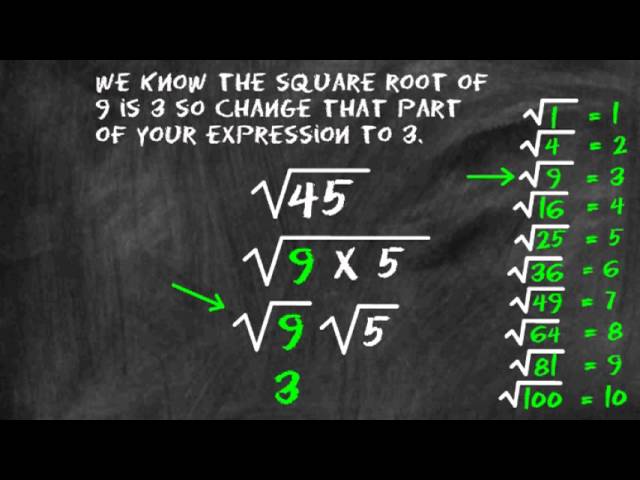
Topic 4 square root of 4: Welcome to our in-depth exploration of "4 Square Root of 4". In this article, we'll demystify this mathematical expression, clarify its different interpretations, and provide practical examples. Whether you're a student, a teacher, or just curious, you'll find valuable insights into the intriguing realm of square roots and their applications.
Table of Content
- Understanding the Expression: 4 √4
- Introduction to Square Roots
- Basics of Square Roots
- Different Interpretations of 4 √4
- Mathematical Calculation: 4 times the Square Root of 4
- Mathematical Calculation: Fourth Root of 4
- Real-world Applications of Square Roots
- Common Misunderstandings and Clarifications
- Practical Examples and Exercises
- Conclusion
- Additional Resources
- YOUTUBE: Video hướng dẫn cách đơn giản hóa căn bậc hai của số 4, giúp bạn hiểu rõ hơn về khái niệm này và áp dụng vào các bài toán thực tế.
Understanding the Expression: 4 √4
The expression "4 √4" can be interpreted in multiple ways depending on the context. Here, we break down the possible interpretations and their mathematical solutions.
Interpretation 1: 4 multiplied by the square root of 4
In this interpretation, the expression is treated as:
\[ 4 \times \sqrt{4} \]
Calculating this, we get:
\[ \sqrt{4} = 2 \]
Thus,
\[ 4 \times 2 = 8 \]
Therefore, the value is 8.
Interpretation 2: The fourth root of 4
In this interpretation, the expression is treated as:
\[ \sqrt[4]{4} \]
Calculating this, we use the property of exponents:
\[ 4^{1/4} \]
Approximating the value, we get:
\[ 4^{1/4} \approx 1.414 \]
Therefore, the value is approximately 1.414.
Summary
The expression "4 √4" can yield different results based on the interpretation:
- 4 multiplied by the square root of 4: 8
- The fourth root of 4: approximately 1.414
It's important to clarify the context to determine the correct interpretation and result.

READ MORE:
Introduction to Square Roots
Square roots are a fundamental concept in mathematics, representing a value that, when multiplied by itself, yields the original number. For example, the square root of 16 is 4, because \(4 \times 4 = 16\).
To understand square roots better, let's look at some key points:
- Notation: The square root of a number \( x \) is written as \( \sqrt{x} \).
- Positive and Negative Roots: While \( \sqrt{16} = 4 \), it is also true that \( -4 \times -4 = 16 \), hence \( \sqrt{16} \) can be \( 4 \) or \( -4 \).
- Perfect Squares: Numbers like 1, 4, 9, 16, and 25 are perfect squares because their square roots are integers.
- Non-Perfect Squares: Numbers like 2, 3, 5, and 10 are not perfect squares, and their square roots are irrational numbers.
Understanding square roots also involves interpreting more complex expressions, such as \( 4 \sqrt{4} \), where we combine square roots with multiplication.
Here's a breakdown of some square roots and their values:
| Number | Square Root |
|---|---|
| 1 | \( \sqrt{1} = 1 \) |
| 4 | \( \sqrt{4} = 2 \) |
| 9 | \( \sqrt{9} = 3 \) |
| 16 | \( \sqrt{16} = 4 \) |
| 25 | \( \sqrt{25} = 5 \) |
Square roots have various applications in geometry, physics, and everyday calculations, making them an essential part of mathematical learning.
Basics of Square Roots
The square root of a number is a value that, when multiplied by itself, gives the original number. It is denoted by the radical symbol √. For example, the square root of 16 is written as √16 and is equal to 4 because 4 multiplied by 4 equals 16.
Here are some key points about square roots:
- The square root of a positive number has two values: a positive and a negative value. For instance, √25 is both 5 and -5, since both 52 and (-5)2 equal 25.
- Every positive number has a real square root.
- The square root of zero is zero.
- Negative numbers do not have real square roots because no real number squared will result in a negative number. They have imaginary roots instead.
The concept of square roots can be extended beyond whole numbers to include fractions and irrational numbers. For example:
- The square root of ¼ is ½, since ½ multiplied by ½ equals ¼.
- The square root of 2 is an irrational number approximately equal to 1.414, which means it cannot be expressed as a simple fraction and its decimal representation is non-repeating and non-terminating.
Square roots are often encountered in various mathematical contexts, such as solving quadratic equations, geometry, and in real-world applications. Understanding the basics of square roots is essential for progressing in mathematics.
Different Interpretations of 4 √4
The expression 4 √4 can be interpreted in different ways depending on the mathematical context. Here are the primary interpretations:
1. 4 times the Square Root of 4
In this interpretation, the expression means 4 multiplied by the square root of 4. Mathematically, it can be represented as:
\[ 4 \times \sqrt{4} \]
Since the square root of 4 is 2, we have:
\[ 4 \times 2 = 8 \]
2. The Fourth Root of 4
Another interpretation is to consider the expression as the fourth root of 4. This can be written as:
\[ \sqrt[4]{4} \]
To find the fourth root of 4, we look for a number which, when raised to the power of 4, equals 4. This is a more complex calculation and involves recognizing that 4 can be expressed as:
\[ 4 = 2^2 \]
Therefore:
\[ \sqrt[4]{4} = \sqrt[4]{2^2} = (2^2)^{\frac{1}{4}} = 2^{\frac{2}{4}} = 2^{\frac{1}{2}} = \sqrt{2} \approx 1.414 \]
3. Misinterpretations and Common Errors
Sometimes, the expression 4 √4 can be misinterpreted due to ambiguity in notation. For clarity, always ensure the context is well-defined. Here are some common errors:
- Confusing multiplication with roots: Mistaking \( 4 \times \sqrt{4} \) for \( \sqrt[4]{4} \).
- Ignoring order of operations: Misinterpreting the sequence of operations, which can lead to incorrect results.
Understanding these different interpretations helps in correctly solving and applying the expression 4 √4 in various mathematical problems.
Mathematical Calculation: 4 times the Square Root of 4
The expression \( 4 \times \sqrt{4} \) involves two steps: finding the square root of 4 and then multiplying the result by 4. Let's go through these steps in detail:
Step 1: Finding the Square Root of 4
The square root of a number \( x \) is a value \( y \) such that \( y^2 = x \). For the number 4:
\[ \sqrt{4} = y \text{ where } y^2 = 4 \]
The number 2 satisfies this equation because:
\[ 2^2 = 4 \]
Therefore, \( \sqrt{4} = 2 \).
Step 2: Multiplying by 4
Now that we have found \( \sqrt{4} \) to be 2, we need to multiply this result by 4:
\[ 4 \times \sqrt{4} = 4 \times 2 \]
Performing the multiplication gives:
\[ 4 \times 2 = 8 \]
Summary
Combining these steps, we have:
\[ 4 \times \sqrt{4} = 8 \]
Therefore, the mathematical calculation of \( 4 \times \sqrt{4} \) results in 8.

Mathematical Calculation: Fourth Root of 4
The fourth root of a number \( x \) is a value \( y \) such that \( y^4 = x \). For the number 4, we can find the fourth root using the following steps:
Step 1: Express 4 in Terms of Powers
First, express the number 4 as a power of 2:
\[ 4 = 2^2 \]
Step 2: Apply the Fourth Root
To find the fourth root, we apply the fourth root operation to both sides of the equation:
\[ \sqrt[4]{4} = \sqrt[4]{2^2} \]
Step 3: Simplify the Expression
Using the property of exponents \( (a^m)^n = a^{mn} \), we can simplify the right-hand side:
\[ \sqrt[4]{2^2} = 2^{2/4} = 2^{1/2} \]
The expression \( 2^{1/2} \) is the same as the square root of 2:
\[ 2^{1/2} = \sqrt{2} \]
Step 4: Approximate the Value
The square root of 2 is an irrational number, which is approximately equal to:
\[ \sqrt{2} \approx 1.414 \]
Summary
Combining these steps, we have:
\[ \sqrt[4]{4} = \sqrt{2} \approx 1.414 \]
Therefore, the fourth root of 4 is approximately 1.414.
Real-world Applications of Square Roots
Square roots have a variety of applications in the real world, spanning different fields such as engineering, physics, finance, and everyday problem-solving. Here are some notable examples:
1. Engineering and Architecture
- Structural Design: Engineers use square roots in the calculation of load distributions and stress analysis to ensure the safety and stability of structures.
- Geometric Calculations: Square roots are essential in calculating distances and dimensions, especially when working with right-angled triangles using the Pythagorean theorem.
2. Physics
- Kinematics: In physics, square roots are used to determine the magnitude of vectors, such as velocity and acceleration, especially when working with components in different directions.
- Wave Propagation: The square root function is used in equations describing wave speed, such as the speed of sound or light in various mediums.
3. Finance
- Risk Assessment: Financial analysts use square roots to calculate standard deviation, a measure of the amount of variation or dispersion in a set of values, which is crucial for risk management and investment decisions.
- Compound Interest: Square roots are involved in the formulas for determining compound interest, helping to predict future investment values.
4. Medicine
- Dosage Calculations: Medical professionals use square roots to calculate correct dosages of medication based on body surface area, which is derived from height and weight measurements.
5. Everyday Problem-Solving
- Area and Perimeter: Square roots are commonly used in calculating the area and perimeter of squares and other geometric shapes.
- Estimations: People use square roots for quick estimations and comparisons in various practical situations, such as determining distances and measurements.
Examples
Here are a few practical examples demonstrating the use of square roots:
- Calculating the diagonal length of a square plot of land: If each side of the square is 50 meters, the diagonal length \(d\) can be found using the formula \(d = \sqrt{2 \times \text{side}^2} = \sqrt{2 \times 50^2} = \sqrt{5000} \approx 70.71\) meters.
- Determining the standard deviation in a set of data: If the variance of a data set is 16, the standard deviation is the square root of the variance, which is \( \sqrt{16} = 4 \).
Understanding and applying square roots is crucial in these and many other real-world scenarios, making it an essential mathematical concept.
Common Misunderstandings and Clarifications
When dealing with square roots, there are several common misunderstandings that can lead to errors. Here, we address these misconceptions and provide clear explanations to help avoid confusion.
1. Misinterpretation of Square Root Symbol
- Misunderstanding: Some people interpret the square root symbol (√) as always producing a single value.
- Clarification: The square root of a number \( x \) is actually two values: \( +\sqrt{x} \) and \( -\sqrt{x} \). For example, \(\sqrt{16}\) is both 4 and -4, because \(4^2 = 16\) and \((-4)^2 = 16\).
2. Square Root of Negative Numbers
- Misunderstanding: Some think the square root of a negative number is real.
- Clarification: The square root of a negative number is not a real number. Instead, it is an imaginary number. For example, \(\sqrt{-4} = 2i\), where \(i\) is the imaginary unit such that \(i^2 = -1\).
3. Simplifying Expressions Incorrectly
- Misunderstanding: Incorrectly simplifying square root expressions, such as assuming \(\sqrt{a + b} = \sqrt{a} + \sqrt{b}\).
- Clarification: The correct property is \(\sqrt{a \times b} = \sqrt{a} \times \sqrt{b}\). For example, \(\sqrt{9 \times 4} = \sqrt{36} = 6\), but \(\sqrt{9 + 4} = \sqrt{13} \approx 3.61\), not \(3 + 2\).
4. Misunderstanding Multiplication and Roots
- Misunderstanding: Confusing \(4 \times \sqrt{4}\) with \(\sqrt[4]{4}\).
- Clarification: The expression \(4 \times \sqrt{4}\) means 4 multiplied by the square root of 4. Since \(\sqrt{4} = 2\), this equals \(4 \times 2 = 8\). On the other hand, \(\sqrt[4]{4}\) is the fourth root of 4, which is \(\sqrt[4]{2^2} = 2^{1/2} = \sqrt{2} \approx 1.414\).
5. Overlooking Principal Square Root
- Misunderstanding: Forgetting that \(\sqrt{x}\) typically refers to the principal (non-negative) square root.
- Clarification: While \(\sqrt{x}\) can be both positive and negative, by convention, \(\sqrt{x}\) refers to the principal square root, which is the non-negative value. For example, \(\sqrt{9} = 3\), not -3.
6. Confusing Roots with Powers
- Misunderstanding: Assuming the root and the power operations are interchangeable without proper rules.
- Clarification: The square root of \( x \) is equivalent to raising \( x \) to the power of 1/2, not any other power. For example, \(\sqrt{x} = x^{1/2}\), and similarly, \(\sqrt[3]{x} = x^{1/3}\).
By understanding these common misunderstandings and their clarifications, you can avoid errors and correctly interpret and calculate square roots in various mathematical contexts.
Practical Examples and Exercises
To solidify your understanding of square roots and their applications, here are some practical examples and exercises. These problems will help you practice calculating square roots and applying them in various contexts.
Example 1: Simple Square Root Calculation
Find the square root of 25.
Solution:
\[ \sqrt{25} = 5 \] because \( 5^2 = 25 \).
Example 2: Multiplying by the Square Root
Calculate \( 3 \times \sqrt{9} \).
Solution:
\[ \sqrt{9} = 3 \]
Therefore, \( 3 \times 3 = 9 \).
Example 3: Using Square Roots in Geometry
A right triangle has legs of lengths 3 and 4. Find the length of the hypotenuse.
Solution:
Using the Pythagorean theorem:
\[ c = \sqrt{a^2 + b^2} = \sqrt{3^2 + 4^2} = \sqrt{9 + 16} = \sqrt{25} = 5 \]
Exercise 1: Square Root of a Product
Calculate the square root of 36 and then multiply by 5.
\[ 5 \times \sqrt{36} = ? \]
Exercise 2: Solving a Quadratic Equation
Solve for \( x \) in the equation \( x^2 = 49 \).
\[ x = \sqrt{49} = ? \]
Exercise 3: Real-world Application
A square garden has an area of 64 square meters. Find the length of one side of the garden.
\[ \text{Side length} = \sqrt{64} = ? \]
Exercise 4: Fourth Root Calculation
Calculate the fourth root of 16.
\[ \sqrt[4]{16} = ? \]
Exercise 5: Combining Operations
Find the value of \( 2 \times \sqrt{49} + \sqrt[3]{27} \).
\[ 2 \times \sqrt{49} + \sqrt[3]{27} = ? \]
Solutions to Exercises
- \( 5 \times \sqrt{36} = 5 \times 6 = 30 \)
- \( x = \pm 7 \) because \( 7^2 = 49 \) and \( (-7)^2 = 49 \)
- \( \text{Side length} = 8 \) meters because \( \sqrt{64} = 8 \)
- \( \sqrt[4]{16} = 2 \) because \( 2^4 = 16 \)
- \( 2 \times \sqrt{49} + \sqrt[3]{27} = 2 \times 7 + 3 = 14 + 3 = 17 \)
These examples and exercises should help you become more comfortable with calculating and applying square roots in various scenarios.

Conclusion
Understanding the concept of square roots and their various interpretations is fundamental in mathematics. Through this article, we explored different aspects of square roots, focusing on the expression \(4 \sqrt{4}\) and its potential meanings.
We began with a thorough introduction to square roots, outlining their basic properties and calculations. From there, we delved into the specific interpretations of \(4 \sqrt{4}\), distinguishing between \(4 \times \sqrt{4}\) and \(\sqrt[4]{4}\). Each interpretation was broken down step by step, ensuring a clear understanding of the processes involved.
Real-world applications highlighted the importance and practicality of square roots in various fields, such as engineering, physics, finance, and everyday problem-solving. Common misunderstandings were addressed to clarify any ambiguities and prevent typical errors associated with square roots.
The practical examples and exercises provided hands-on experience, reinforcing the concepts discussed and offering a chance to apply knowledge in different scenarios. These exercises aimed to enhance problem-solving skills and deepen comprehension.
In summary, mastering square roots and their applications is crucial for both academic and real-life contexts. The expression \(4 \sqrt{4}\) serves as an excellent example of how mathematical notation can have multiple interpretations, each with its own significance. By thoroughly understanding these concepts, one can confidently navigate through various mathematical challenges and apply these principles effectively in numerous fields.
Additional Resources
For those interested in further exploring the concept of square roots, including the specific case of 4√4, the following resources can provide valuable insights and tools:
- : A versatile tool that allows you to calculate the square root of any number, including step-by-step solutions.
- : Another powerful calculator that not only computes square roots but also provides detailed explanations for each step of the calculation.
- : This tool helps you find both the principal and negative square roots of real numbers, along with explanations and examples.
- : A video tutorial that provides a comprehensive introduction to the concept of square roots, including practical examples and common pitfalls.
- : An educational website that explains square roots in a fun and engaging way, suitable for learners of all ages.
These resources are excellent for gaining a deeper understanding of square roots and their applications, providing both theoretical explanations and practical tools.
Video hướng dẫn cách đơn giản hóa căn bậc hai của số 4, giúp bạn hiểu rõ hơn về khái niệm này và áp dụng vào các bài toán thực tế.
Cách Đơn Giản Hóa Căn Bậc Hai của 4: sqrt(4)
READ MORE:
Video này hướng dẫn cách tìm căn bậc hai của số 4 bằng phương pháp sử dụng cây phân tích. Thích hợp cho người học toán muốn hiểu rõ hơn về khái niệm này.
Căn bậc hai của 4 bằng cách sử dụng cây phân tích