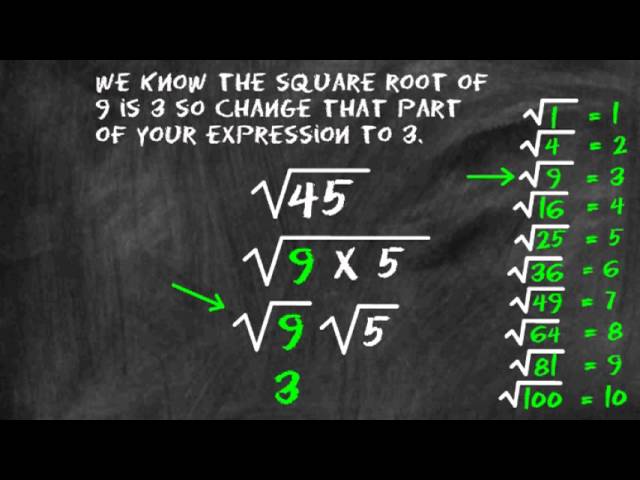
Topic simplify the square root of 81: Understanding how to simplify the square root of 81 is an essential math skill. This guide will walk you through simple, clear steps to demystify the process, helping you to confidently tackle similar problems in the future. Whether you're a student or just brushing up on your math, this article has you covered.
Table of Content
- Simplifying the Square Root of 81
- Introduction to Square Roots
- Understanding the Concept of Simplifying Square Roots
- Step-by-Step Guide to Simplify the Square Root of 81
- Breaking Down 81 into Prime Factors
- Finding the Square Root of Perfect Squares
- Using the Property of Square Roots
- Applying Simplification Rules to 81
- Common Mistakes to Avoid
- Practical Examples and Practice Problems
- FAQs about Simplifying Square Roots
- Conclusion and Summary
- YOUTUBE: Học cách đơn giản hóa căn bậc hai của một số bằng phân tích thừa số nguyên tố, Sqrt(81). Video này giúp bạn hiểu và áp dụng những phương pháp căn bậc hai để giảm đơn giản dạng Sqrt(81) một cách hiệu quả.
Simplifying the Square Root of 81
The process of simplifying the square root of 81 is straightforward. The goal is to express the square root in its simplest form. Here, we break down the steps to achieve this simplification:
Step-by-Step Simplification
- Identify the square root you want to simplify: \( \sqrt{81} \).
- Find the prime factors of the number under the square root. For 81, the prime factorization is:
\[
81 = 3 \times 3 \times 3 \times 3 = 3^4
\] - Rewrite the square root using the prime factors:
\[
\sqrt{81} = \sqrt{3^4}
\] - Apply the property of square roots that \(\sqrt{a^2} = a\):
\[
\sqrt{3^4} = 3^2 = 9
\]
Final Simplified Form
Thus, the square root of 81 simplifies to:
\[
\sqrt{81} = 9
\]
Conclusion
The square root of 81 is simplified to 9, which is its simplest form. This demonstrates how recognizing and utilizing prime factorization can make simplifying square roots a straightforward process.

READ MORE:
Introduction to Square Roots
Square roots are a fundamental concept in mathematics, representing a value that, when multiplied by itself, gives the original number. For example, the square root of 81 is the number that, when squared, equals 81.
In mathematical notation, the square root of a number \( x \) is written as \( \sqrt{x} \). For instance, \( \sqrt{81} = 9 \) because \( 9 \times 9 = 81 \).
To understand square roots better, let’s explore the key concepts:
- Perfect Squares: These are numbers like 1, 4, 9, 16, and 81, which have integer square roots.
- Non-Perfect Squares: Numbers that do not have an integer as their square root, resulting in an irrational number.
- Radical Symbol: The symbol \( \sqrt{} \) used to denote the square root.
- Simplifying Square Roots: The process of finding the simplest form of a square root.
Let’s break down the process of simplifying square roots:
- Identify Perfect Squares: Recognize numbers that are perfect squares, like 81, which simplifies directly to 9.
- Factorization: For non-perfect squares, factorize the number into its prime factors to simplify.
- Pairing Factors: Group the prime factors into pairs to simplify the square root.
In the next sections, we will apply these steps specifically to simplify the square root of 81.
Understanding the Concept of Simplifying Square Roots
Simplifying square roots involves breaking down the number inside the square root to its simplest form. The process typically involves prime factorization and recognizing perfect squares. Here's a step-by-step guide to understanding this concept:
-
Identify the Prime Factors:
To simplify a square root, start by finding the prime factors of the number inside the square root. For example, for the number 81, the prime factorization is:
\[
81 = 3 \times 3 \times 3 \times 3
\] -
Group the Factors into Pairs:
Next, group the prime factors into pairs of identical numbers. Each pair of identical numbers can be taken out of the square root as a single number:
\[
\sqrt{81} = \sqrt{(3 \times 3) \times (3 \times 3)}
\] -
Simplify the Expression:
For every pair of numbers, take one number out of the square root:
\[
\sqrt{81} = \sqrt{(3^2) \times (3^2)} = 3 \times 3 = 9
\]
Therefore, the simplified form of \(\sqrt{81}\) is \(9\). This method works for any perfect square, allowing us to simplify the square root to its most basic form.
Understanding this concept is crucial for solving more complex mathematical problems involving square roots. Simplifying square roots makes calculations easier and helps in recognizing patterns in numbers.
Step-by-Step Guide to Simplify the Square Root of 81
In this section, we will simplify the square root of 81 step by step. Understanding the process will help in simplifying other square roots as well.
-
Prime Factorization Method
First, we find the prime factors of 81:
- 81 can be written as 3 × 3 × 3 × 3 or \( 3^4 \).
Next, we group the prime factors in pairs of two:
- \( 81 = 3^2 \times 3^2 \)
Take the square root of each pair:
- \( \sqrt{81} = \sqrt{3^2 \times 3^2} \)
- \( \sqrt{81} = \sqrt{3^2} \times \sqrt{3^2} \)
- \( \sqrt{81} = 3 \times 3 = 9 \)
-
Repeated Subtraction Method
This method involves subtracting consecutive odd numbers from 81 until you reach zero. The number of subtractions gives the square root.
- 81 - 1 = 80
- 80 - 3 = 77
- 77 - 5 = 72
- 72 - 7 = 65
- 65 - 9 = 56
- 56 - 11 = 45
- 45 - 13 = 32
- 32 - 15 = 17
- 17 - 17 = 0
The total number of subtractions is 9, so the square root of 81 is 9.
-
Long Division Method
This method is more systematic and involves the following steps:
- Pair the digits of 81 from right to left.
- Find the largest number whose square is less than or equal to 81. This number is 9.
- Divide 81 by 9, which gives a quotient of 9 and a remainder of 0.
Thus, the square root of 81 is 9.
All these methods confirm that \( \sqrt{81} = 9 \).
Breaking Down 81 into Prime Factors
To simplify the square root of 81, we first need to break down the number 81 into its prime factors. Prime factorization is a method of expressing a number as a product of its prime factors. Here are the steps to break down 81 into prime factors:
- Start with the number 81.
- Identify the smallest prime number that divides 81 exactly. In this case, it is 3.
- Divide 81 by 3 to get 27:
\( \frac{81}{3} = 27 \) - Next, divide 27 by 3 to get 9:
\( \frac{27}{3} = 9 \) - Divide 9 by 3 to get 3:
\( \frac{9}{3} = 3 \) - Finally, divide 3 by 3 to get 1:
\( \frac{3}{3} = 1 \)
The prime factorization of 81 is:
\( 81 = 3 \times 3 \times 3 \times 3 \) or \( 81 = 3^4 \)
Using the prime factorization, we can express the square root of 81 as:
\( \sqrt{81} = \sqrt{3 \times 3 \times 3 \times 3} = \sqrt{(3^2) \times (3^2)} \)
Since the square root of a product is the product of the square roots, we get:
\( \sqrt{81} = \sqrt{(3^2)} \times \sqrt{(3^2)} = 3 \times 3 = 9 \)
Therefore, the square root of 81, simplified using prime factorization, is 9.

Finding the Square Root of Perfect Squares
The process of finding the square root of a perfect square is straightforward. A perfect square is a number that is the square of an integer. This means that it can be expressed as the product of an integer with itself.
For instance, consider the number 81. To find its square root, follow these steps:
-
Express 81 as the product of an integer with itself:
\[
81 = 9 \times 9
\] -
Since 81 can be written as \(9^2\), the square root of 81 is simply 9:
\[
\sqrt{81} = \sqrt{9^2} = 9
\]
This method works for any perfect square. Another example is 16, which can be written as:
\[
16 = 4 \times 4 \implies \sqrt{16} = \sqrt{4^2} = 4
\]
Understanding this concept is fundamental in simplifying square roots. When dealing with larger perfect squares, the process remains the same. Identify the integer that, when squared, gives you the perfect square, and that integer is the square root.
Here are a few more examples:
-
\[
\sqrt{25} = \sqrt{5^2} = 5
\] -
\[
\sqrt{36} = \sqrt{6^2} = 6
\] -
\[
\sqrt{49} = \sqrt{7^2} = 7
\]
Perfect squares are easy to recognize, and their square roots are integers. This property makes them simple to work with in various mathematical applications.
Using the Property of Square Roots
The properties of square roots are essential in simplifying expressions involving radicals. Here are the key properties and how they apply to the square root of 81:
- Product Property: This property states that the square root of a product is the product of the square roots of the factors. Mathematically, it can be expressed as:
\[\sqrt{a \cdot b} = \sqrt{a} \cdot \sqrt{b}\]
For example, if we have \(\sqrt{36 \cdot 81}\), we can simplify it as follows:
\[\sqrt{36 \cdot 81} = \sqrt{36} \cdot \sqrt{81} = 6 \cdot 9 = 54\] - Quotient Property: This property states that the square root of a quotient is the quotient of the square roots. Mathematically, it can be expressed as:
\[\sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}}\]
For example, if we have \(\sqrt{\frac{81}{9}}\), we can simplify it as follows:
\[\sqrt{\frac{81}{9}} = \frac{\sqrt{81}}{\sqrt{9}} = \frac{9}{3} = 3\]
Now, let’s apply these properties to the square root of 81:
- Recognizing Perfect Squares: 81 is a perfect square because it can be expressed as \(9^2\). Therefore, we can write: \[\sqrt{81} = 9\]
- Prime Factorization: Another way to simplify square roots is by prime factorization. For 81, we can break it down into its prime factors: \[81 = 3 \times 3 \times 3 \times 3 = 3^4\] Using the product property, we have: \[\sqrt{81} = \sqrt{3^4} = 3^2 = 9\]
Understanding and using these properties of square roots not only helps in simplifying \(\sqrt{81}\) but also in dealing with more complex radical expressions.
Applying Simplification Rules to 81
To simplify the square root of 81, we can use a step-by-step approach involving prime factorization. Here is the detailed process:
-
Find the Prime Factors of 81
First, break down 81 into its prime factors. Since 81 is a perfect square, it can be expressed as a product of its prime factors:
\[ 81 = 3 \times 3 \times 3 \times 3 = 3^4 \]
-
Group the Factors in Pairs
Next, group the prime factors in pairs of two identical numbers:
\[ 81 = (3 \times 3) \times (3 \times 3) = 9 \times 9 \]
-
Take One Factor from Each Pair
From each pair, take one factor:
\[ \sqrt{81} = \sqrt{(3^2 \times 3^2)} = 3 \times 3 = 9 \]
-
Result
The simplified form of the square root of 81 is:
\[ \sqrt{81} = 9 \]
This step-by-step method shows that the square root of 81 is 9. This simplification process is straightforward due to 81 being a perfect square.
Common Mistakes to Avoid
When simplifying square roots, it's essential to avoid common mistakes that can lead to incorrect results. Here are some key points to remember:
- Overlooking Perfect Squares: Always check for perfect squares within the radicand. For instance, in √81, recognize that 81 is a perfect square (9×9).
- Misapplying Properties: Remember that √a * √b = √(a*b), but √a + √b ≠ √(a + b). Use properties correctly for multiplication and division, not addition or subtraction.
- Ignoring Variables: Simplify variables as you would numbers. For example, √(x²) = x, assuming x is non-negative.
- Simplification Errors: Double-check arithmetic steps to ensure correct factorization and calculations.
- Forgetting to Rationalize the Denominator: Ensure to rationalize denominators when square roots appear in the denominator to present the expression in its simplest form.
By being mindful of these common pitfalls, you can improve your accuracy and efficiency in simplifying square roots.

Practical Examples and Practice Problems
Understanding how to simplify square roots can be enhanced by practicing with a variety of examples and problems. Here are some practical examples to help reinforce the concepts and provide practice opportunities:
Examples
-
Simplify \( \sqrt{25} \)
Solution: We need to find a number that, when multiplied by itself, gives 25. That number is 5, so:
\[
\sqrt{25} = 5
\] -
Simplify \( \sqrt{32} \)
Solution: 32 can be factored into 16 and 2. Since 16 is a perfect square:
\[
\sqrt{32} = \sqrt{16 \times 2} = \sqrt{16} \times \sqrt{2} = 4\sqrt{2}
\] -
Simplify \( \sqrt{50} \)
Solution: 50 can be factored into 25 and 2. Since 25 is a perfect square:
\[
\sqrt{50} = \sqrt{25 \times 2} = \sqrt{25} \times \sqrt{2} = 5\sqrt{2}
\] -
Simplify \( \sqrt{180} \)
Solution: 180 can be factored into 36 and 5. Since 36 is a perfect square:
\[
\sqrt{180} = \sqrt{36 \times 5} = \sqrt{36} \times \sqrt{5} = 6\sqrt{5}
\]
Practice Problems
Try simplifying the following square roots:
- Simplify \( \sqrt{64} \)
- Simplify \( \sqrt{98} \)
- Simplify \( \sqrt{200} \)
- Simplify \( \sqrt{72} \)
Solutions
-
Simplify \( \sqrt{64} \)
Solution: 64 is a perfect square:
\[
\sqrt{64} = 8
\] -
Simplify \( \sqrt{98} \)
Solution: 98 can be factored into 49 and 2. Since 49 is a perfect square:
\[
\sqrt{98} = \sqrt{49 \times 2} = \sqrt{49} \times \sqrt{2} = 7\sqrt{2}
\] -
Simplify \( \sqrt{200} \)
Solution: 200 can be factored into 100 and 2. Since 100 is a perfect square:
\[
\sqrt{200} = \sqrt{100 \times 2} = \sqrt{100} \times \sqrt{2} = 10\sqrt{2}
\] -
Simplify \( \sqrt{72} \)
Solution: 72 can be factored into 36 and 2. Since 36 is a perfect square:
\[
\sqrt{72} = \sqrt{36 \times 2} = \sqrt{36} \times \sqrt{2} = 6\sqrt{2}
\]
FAQs about Simplifying Square Roots
Here are some frequently asked questions about simplifying square roots along with detailed answers to help you understand the concepts better:
1. What does it mean to simplify a square root?
Simplifying a square root means rewriting it in its simplest form where the number inside the square root has no perfect square factors other than 1. For example, the square root of 81 simplifies to 9 because 81 is a perfect square.
2. How do you simplify a square root step-by-step?
To simplify a square root, follow these steps:
- Find the prime factorization of the number inside the square root.
- Pair the prime factors.
- Move one number from each pair outside the square root.
- Multiply the numbers outside the square root together.
For example, to simplify
- Prime factorization of 81: 3 × 3 × 3 × 3
- Pair the factors: (3 × 3) × (3 × 3)
- Move one number from each pair outside: 3 × 3
- Simplified form: 9
3. What is the difference between simplifying a square root and finding an approximate value?
Simplifying a square root results in an exact value or simplest radical form, while finding an approximate value involves using a calculator to get a decimal approximation. For example,
4. Can you simplify square roots of negative numbers?
No, you cannot simplify square roots of negative numbers within the set of real numbers. Square roots of negative numbers involve imaginary numbers. For instance,
5. What are common mistakes to avoid when simplifying square roots?
- Not fully factorizing the number inside the square root.
- Forgetting to pair factors correctly.
- Incorrectly assuming the square root of a non-perfect square is a simple fraction.
- Misplacing the negative sign when dealing with negative square roots.
6. Are there special rules for simplifying square roots involving fractions?
Yes, to simplify square roots of fractions, use the property:
7. How can I practice simplifying square roots?
You can practice by working through problems that require you to factorize and simplify square roots. Here are a few practice problems:
- Simplify
\(\sqrt{72}\) . - Simplify
\(\sqrt{50}\) and express it in simplest radical form. - Find the value of k in the equation
\(2\sqrt{81} + k = 28\) .
Conclusion and Summary
Simplifying the square root of 81 involves recognizing it as a perfect square. By breaking down 81 into its prime factors (3 × 3 × 3 × 3), we identify that the square root simplifies to 9. This process underscores the importance of understanding basic properties of square roots and applying them systematically.
Key takeaways include:
- 81 is a perfect square, making its square root an integer.
- Breaking down numbers into prime factors helps in simplifying square roots.
- The property √(ab) = √a * √b is crucial for simplifying square roots.
By avoiding common mistakes such as misidentifying perfect squares or skipping prime factorization, one can confidently simplify square roots like √81 in various mathematical contexts.
Học cách đơn giản hóa căn bậc hai của một số bằng phân tích thừa số nguyên tố, Sqrt(81). Video này giúp bạn hiểu và áp dụng những phương pháp căn bậc hai để giảm đơn giản dạng Sqrt(81) một cách hiệu quả.
Học cách đơn giản hóa căn bậc hai của một số bằng phân tích thừa số nguyên tố, Sqrt(81)
READ MORE:
Học cách đơn giản hóa căn bậc hai của số 81 với phương pháp sqrt(81). Video này cung cấp hướng dẫn chi tiết và thực hành để giảm đơn giản căn bậc hai của 81.
Cách đơn giản hóa căn bậc hai của 81: sqrt(81)