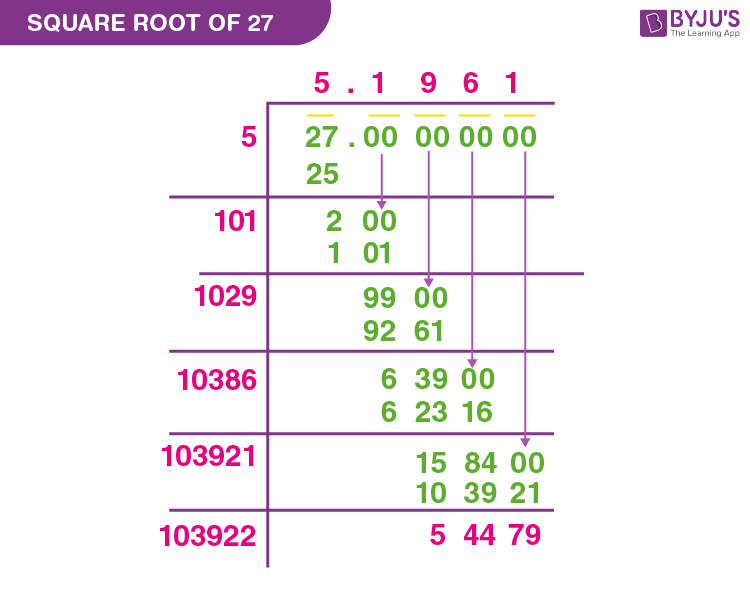
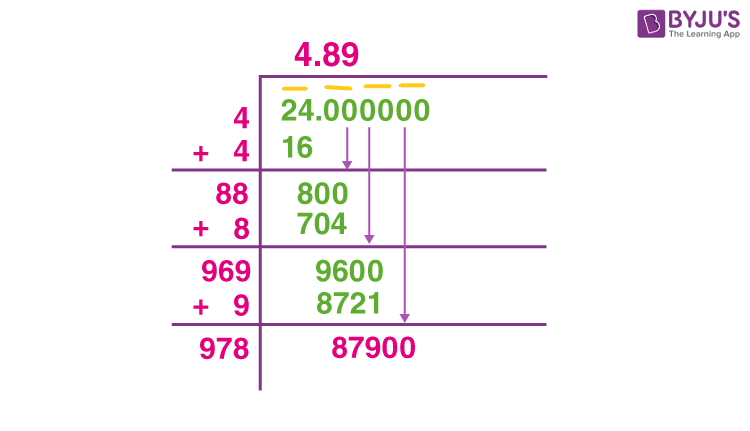
Topic what is the square root of 360: Curious about what the square root of 360 is? In this article, we break down the exact and decimal forms of the square root of 360, explore its mathematical properties, and provide easy-to-understand explanations to enhance your knowledge. Dive in to learn all about this intriguing number!
Table of Content
- Square Root of 360
- Introduction
- Understanding Square Roots
- What is the Square Root of 360?
- Exact Form of Square Root of 360
- Decimal Form of Square Root of 360
- Steps to Simplify the Square Root of 360
- Applications of the Square Root of 360
- Mathematical Properties of Square Root of 360
- Visual Representation of Square Root of 360
- Comparison with Other Square Roots
- Frequently Asked Questions
- Conclusion
- YOUTUBE: Video giới thiệu về căn bậc hai của số 360, bao gồm các ứng dụng và tính chất toán học. Tìm hiểu thêm về cách tính và tầm quan trọng của căn bậc hai trong toán học.
Square Root of 360
The square root of 360 can be expressed in both exact and decimal forms. Below are the details:
Exact Form
The square root of 360 can be simplified as follows:
So, the exact form is \( 6 \sqrt{10} \).
Decimal Form
The approximate decimal form of the square root of 360 is:
Table of Values
| Value | Expression |
|---|---|
| Exact Form | |
| Decimal Form | 18.97366596 |

READ MORE:
Introduction
The square root of 360 is a mathematical expression that can be represented in both exact and approximate decimal forms. Understanding square roots is essential for various mathematical calculations and applications. In this section, we will explore the concept of square roots, explain how to simplify the square root of 360, and provide its exact and decimal forms. Let's dive into the fascinating world of square roots and uncover the properties of the square root of 360.
- Definition of Square Roots
- Exact Form of Square Root of 360
- Decimal Form of Square Root of 360
- Steps to Simplify Square Root of 360
- Applications and Uses
The square root of a number is a value that, when multiplied by itself, gives the original number. For example, the square root of 360 can be simplified to a more manageable form using factorization techniques. The exact form involves simplifying the number into its prime factors, while the decimal form provides an approximate value for practical use.
Understanding Square Roots
Square roots are fundamental mathematical concepts used to find a number that, when multiplied by itself, yields the original number. For instance, the square root of 360 is a value which, when squared, equals 360. To comprehend square roots better, let's break down the concept into several key points:
- Definition: A square root of a number \( x \) is a number \( y \) such that \( y^2 = x \). For example, \( \sqrt{360} \) represents a number \( y \) where \( y^2 = 360 \).
- Notation: The square root is denoted by the radical symbol \( \sqrt{} \). Thus, the square root of 360 is written as \( \sqrt{360} \).
- Positive and Negative Roots: Every positive number has two square roots: one positive and one negative. For 360, these are \( \sqrt{360} \) and \( -\sqrt{360} \).
- Perfect Squares: Numbers like 1, 4, 9, 16, 25, etc., whose square roots are whole numbers, are called perfect squares. 360 is not a perfect square, which is why its square root is an irrational number.
- Simplification: Simplifying square roots involves factorizing the number into its prime factors. For example, 360 can be factored as \( 360 = 2^3 \times 3^2 \times 5 \). This helps in expressing the square root in its simplest form.
By understanding these basics, we can simplify and compute the square root of 360 efficiently. In the next sections, we'll delve deeper into the exact and decimal forms of the square root of 360 and demonstrate the steps to simplify it.
What is the Square Root of 360?
The square root of 360 is a number which, when multiplied by itself, equals 360. This value can be represented in both exact and decimal forms. Here, we will explore both representations and understand the process of simplifying the square root of 360.
Exact Form
The exact form of the square root of 360 can be derived through prime factorization:
- First, factorize 360 into its prime factors:
- 360 = 2 × 180
- 180 = 2 × 90
- 90 = 2 × 45
- 45 = 3 × 15
- 15 = 3 × 5
- Combining all prime factors, we get:
- 360 = 23 × 32 × 5
- To simplify the square root, pair the prime factors:
- \(\sqrt{360} = \sqrt{2^3 \times 3^2 \times 5} = \sqrt{(2^2 \times 2) \times (3^2) \times 5}\)
- \(\sqrt{360} = \sqrt{2^2} \times \sqrt{3^2} \times \sqrt{2 \times 5}\)
- \(\sqrt{360} = 2 \times 3 \times \sqrt{10}\)
- \(\sqrt{360} = 6\sqrt{10}\)
Decimal Form
The approximate decimal value of the square root of 360 can be calculated as follows:
This value can be rounded to 18.97 for simplicity in most practical applications.
Understanding both the exact and decimal forms of the square root of 360 helps in various mathematical computations and applications. By breaking down the process step by step, we can see how prime factorization aids in simplifying square roots, while decimal approximations provide practical values for everyday use.
Exact Form of Square Root of 360
The exact form of the square root of 360 can be determined by simplifying it through prime factorization. This process breaks down 360 into its prime components, allowing us to simplify the square root in an exact form. Here's a detailed step-by-step explanation:
- Prime Factorization:
- 360 can be factorized into prime numbers as follows:
- 360 = 2 × 180
- 180 = 2 × 90
- 90 = 2 × 45
- 45 = 3 × 15
- 15 = 3 × 5
- Thus, 360 = 23 × 32 × 5.
- Pairing the Prime Factors:
- To simplify the square root, we pair the prime factors:
- \(\sqrt{360} = \sqrt{2^3 \times 3^2 \times 5}\)
- Rewrite as: \(\sqrt{360} = \sqrt{(2^2 \times 2) \times (3^2) \times 5}\)
- Simplify further: \(\sqrt{360} = \sqrt{2^2} \times \sqrt{3^2} \times \sqrt{2 \times 5}\)
- Simplifying the Expression:
- \(\sqrt{2^2} = 2\)
- \(\sqrt{3^2} = 3\)
- Therefore, \(\sqrt{360} = 2 \times 3 \times \sqrt{10}\)
- \(\sqrt{360} = 6\sqrt{10}\)
Hence, the exact form of the square root of 360 is \(6\sqrt{10}\). This form is particularly useful in mathematical expressions where precision is crucial, and it helps in further simplifying related calculations.

Decimal Form of Square Root of 360
The decimal form of the square root of 360 provides an approximate value that is useful for practical applications where an exact form is not necessary. This can be calculated using a calculator or numerical methods. Here’s how we determine the decimal form step-by-step:
- Using a Calculator:
- Input the number 360 into the calculator.
- Press the square root (√) function.
- The display shows the square root of 360 as approximately 18.97366596.
- Manual Calculation (Approximation):
- To manually approximate the square root, you can use methods like the Newton-Raphson method, though it’s more complex than using a calculator.
- For simplicity, use the approximation: \(\sqrt{360} \approx 18.97\).
The decimal value of the square root of 360 is approximately:
In many practical situations, rounding this value to two decimal places is sufficient, resulting in 18.97. This approximation is useful for quick calculations and provides a sufficient level of precision for most everyday uses.
Steps to Simplify the Square Root of 360
Simplifying the square root of 360 involves breaking it down into its prime factors and finding perfect squares. Follow these steps to simplify:
- First, find the prime factorization of 360:
- 360 ÷ 2 = 180
- 180 ÷ 2 = 90
- 90 ÷ 2 = 45
- 45 ÷ 3 = 15
- 15 ÷ 3 = 5
- 5 ÷ 5 = 1
So, the prime factorization of 360 is \(2^3 \times 3^2 \times 5\).
- Next, pair the prime factors to identify the perfect squares:
- \(2^3\) can be written as \(2^2 \times 2\), where \(2^2\) is a perfect square.
- \(3^2\) is already a perfect square.
- \(5\) remains as it is.
- Group the perfect squares:
- \(\sqrt{2^2 \times 3^2 \times 2 \times 5} = \sqrt{2^2} \times \sqrt{3^2} \times \sqrt{2 \times 5}\)
- Simplify the square roots of the perfect squares:
- \(\sqrt{2^2} = 2\)
- \(\sqrt{3^2} = 3\)
So, \(\sqrt{2^2 \times 3^2 \times 2 \times 5} = 2 \times 3 \times \sqrt{10}\).
- Finally, multiply the simplified terms:
- \(2 \times 3 = 6\)
- \(6 \times \sqrt{10}\)
Therefore, the simplified form of \(\sqrt{360}\) is \(6\sqrt{10}\).
Applications of the Square Root of 360
The square root of 360, approximately 18.97, has several interesting applications in various fields. Here are some examples of how it can be used:
- Geometry and Area Calculations:
In geometry, square roots are often used to determine the dimensions of a square given its area. If a square has an area of 360 square units, the length of each side is the square root of 360, which is approximately 18.97 units.
- Physics and Gravity:
Square roots are used in physics, particularly in equations involving gravity. For instance, the time \( t \) (in seconds) it takes for an object to fall from a height \( h \) (in feet) can be determined using the formula \( t = \frac{\sqrt{h}}{4} \). If an object is dropped from a height of 360 feet, the time it takes to reach the ground is \( \frac{\sqrt{360}}{4} \), which is approximately 1.19 seconds.
- Accident Investigations:
In accident investigations, the length of skid marks can help determine the speed of a vehicle before braking. The speed \( v \) (in miles per hour) can be found using the formula \( v = \sqrt{24d} \), where \( d \) is the length of the skid marks in feet. If the skid marks are 360 feet long, the speed of the vehicle was approximately \( \sqrt{24 \times 360} \approx 93 \) miles per hour.
- Engineering and Construction:
Engineers and architects use square roots in various calculations involving measurements and material properties. For example, the diagonal length of a square with side length \( s \) can be found using the Pythagorean theorem, where the diagonal is \( s\sqrt{2} \). For a square with sides of length \( \sqrt{360} \approx 18.97 \), the diagonal is approximately \( 18.97 \sqrt{2} \approx 26.82 \) units.
Mathematical Properties of Square Root of 360
The square root of 360 has several interesting mathematical properties. Here are some key properties explained in detail:
- Principal Square Root: The square root of 360 is approximately \( \sqrt{360} \approx 18.973665961 \). This is the principal (positive) square root.
- Radical Form: The simplified form of the square root of 360 is expressed as \( \sqrt{360} = 6\sqrt{10} \). This is derived by recognizing that 36 is the largest perfect square factor of 360.
- Exponential Form: The square root can also be represented in exponential form: \( 360^{1/2} \) or \( 360^{0.5} \).
- Irrational Number: The square root of 360 is an irrational number, meaning it cannot be expressed as a simple fraction. It has a non-repeating, non-terminating decimal expansion.
- Properties of Square Roots:
- If \( x \) is the square root of a number \( X \), then both \( \sqrt{X} = x \) and \( \sqrt{X} = -x \). Therefore, \( \sqrt{360} \) also includes \( -18.973665961 \).
- The product of the square roots of two numbers is equal to the square root of their product: \( \sqrt{a} \times \sqrt{b} = \sqrt{a \times b} \). For example, \( \sqrt{36 \times 10} = \sqrt{360} \).
- Even and Odd Properties:
- The square root of an even number is always even if the number is a perfect square. Since 360 is not a perfect square, this property does not directly apply, but \( \sqrt{360} \) is still an irrational number.
- If a number ends in an even number of zeros, its square root will be an integer. 360 does not end in zeros, so its square root is not an integer.
These properties highlight the unique characteristics of the square root of 360 and how it fits into broader mathematical concepts.
Visual Representation of Square Root of 360
Visualizing the square root of 360 can help in understanding its properties and relationships with other numbers. Here are some ways to represent it visually:
1. Number Line Representation
On a number line, the square root of 360 lies between 18 and 19. To be precise, it is approximately 18.97. This can be shown by marking the positions of 18 and 19 on the number line and identifying the point where √360 falls.

2. Geometric Square Representation
One of the most straightforward ways to visualize square roots is through geometry. If we consider a square with an area of 360 square units, the side length of that square would be the square root of 360.

3. Graphical Plot
Using a graph, we can plot the function y = √x. The point (360, √360) will fall on this curve, allowing us to see where the square root of 360 lies relative to other square roots.

4. Square Root Spiral
A more artistic and intricate way to visualize square roots is by constructing a square root spiral. This spiral is created by plotting successive square roots on graph paper and connecting the points to form a continuous spiral. The distance between points increases as the values grow, showing the nature of square roots.

5. Using Desmos or Similar Tools
Interactive tools like Desmos can be used to dynamically illustrate the square root of 360. By inputting the function and zooming in, you can see a precise representation of √360.
These visual methods help in comprehending the value and significance of the square root of 360, making it easier to grasp its mathematical properties and applications.
Comparison with Other Square Roots
Understanding how the square root of 360 compares with other square roots can provide valuable insights into its properties and applications. Below, we will explore comparisons with both smaller and larger square roots.
Comparison with Smaller Square Roots
- Square Root of 100: The square root of 100 is 10. This is a perfect square, and the value is a whole number. In contrast, the square root of 360 is approximately 18.97, which is not a perfect square and is an irrational number.
- Square Root of 144: The square root of 144 is 12. Like 100, it is a perfect square and results in a whole number. The square root of 360, however, does not yield a whole number and is larger than the square root of 144.
- Square Root of 225: The square root of 225 is 15, another perfect square. It is closer to the square root of 360 but still smaller.
Comparison with Larger Square Roots
- Square Root of 400: The square root of 400 is 20. This value is slightly larger than the square root of 360, which is approximately 18.97.
- Square Root of 625: The square root of 625 is 25. This value is significantly larger than the square root of 360.
- Square Root of 900: The square root of 900 is 30. This value is much larger than the square root of 360, highlighting the difference between perfect squares and non-perfect squares.
Visual Representation of Comparisons
To visualize these comparisons, consider the following table:
| Number | Square Root | Type |
|---|---|---|
| 100 | 10 | Perfect Square |
| 144 | 12 | Perfect Square |
| 225 | 15 | Perfect Square |
| 360 | 18.97 | Non-Perfect Square |
| 400 | 20 | Perfect Square |
| 625 | 25 | Perfect Square |
| 900 | 30 | Perfect Square |
As shown in the table, the square root of 360 is unique among these examples because it is not a perfect square and results in an irrational number. This sets it apart from the others, providing a clear understanding of its position among other square roots.
Frequently Asked Questions
Here are some common questions and answers related to the square root of 360:
-
What is the square root of 360?
The square root of 360 is approximately 18.97366596.
-
Is the square root of 360 a rational or irrational number?
The square root of 360 is an irrational number because it cannot be expressed as a simple fraction and its decimal form is non-terminating and non-repeating.
-
Can the square root of 360 be simplified?
Yes, the square root of 360 can be simplified to \(6\sqrt{10}\) using prime factorization. Here's how it's done:
- Prime factorize 360: \(360 = 2^3 \times 3^2 \times 5\)
- Group the factors in pairs: \(\sqrt{360} = \sqrt{2^3 \times 3^2 \times 5} = \sqrt{2^2 \times 3^2 \times 2 \times 5} = 6\sqrt{10}\)
How do you calculate the square root of 360 using a calculator?
To find the square root of 360 using a calculator, enter 360 and use the square root function. The result will be approximately 18.97366596.
-
How is the square root of 360 used in real-life applications?
The square root of 360 can be used in various fields such as engineering, physics, and statistics where precise calculations are required, particularly in determining dimensions, distances, and in the Pythagorean theorem.
-
What is the principal square root of 360?
The principal square root of 360 is the positive value, which is approximately 18.97366596.
Conclusion
The square root of 360 is an interesting number with several unique properties and applications. Its approximate value is \(\sqrt{360} \approx 18.97\), and it can be expressed in its simplified radical form as \(6\sqrt{10}\). This number is irrational, meaning it cannot be expressed as a simple fraction, and its decimal representation is non-repeating and non-terminating.
Understanding the square root of 360 involves exploring various mathematical concepts, such as prime factorization, irrational numbers, and the simplification of radicals. The steps to simplify the square root of 360 reveal its structure, breaking it down into its prime factors and demonstrating the process of simplifying the expression.
In practical applications, the square root of 360 can be used in geometry, physics, engineering, and various fields where precise calculations are necessary. For example, it can be used to determine distances, calculate areas, and solve equations involving quadratic terms.
By comparing the square root of 360 with other square roots, we can appreciate the diversity of irrational numbers and their significance in mathematics. Additionally, visual representations help us to grasp the concept better, providing a concrete way to see how the square root fits into a number line or geometric context.
In summary, the square root of 360 is not just a number but a gateway to deeper mathematical understanding and exploration. Its properties and applications demonstrate the beauty and complexity of mathematics, encouraging further study and appreciation of this essential field.

Video giới thiệu về căn bậc hai của số 360, bao gồm các ứng dụng và tính chất toán học. Tìm hiểu thêm về cách tính và tầm quan trọng của căn bậc hai trong toán học.
Chuyên Đề Căn Bậc Hai của 360
READ MORE:
Video hướng dẫn mẹo nhanh để tính căn bậc hai của số 360, phù hợp cho những ai yêu thích toán học và các mẹo tính toán nhanh.
Mẹo nhanh tính căn bậc hai cho 360 #căn_bậc_hai #toán_học #mẹo_toán_học