What is the Negative Square Root of 1?

In mathematics, the concept of the square root of a negative number introduces us to imaginary numbers, denoted by the symbol \( i \).

What is the Square Root of Minus One?

The square root of minus one is an imaginary number represented as \( i \). By definition:

\[ i^2 = -1 \]

Thus, the principal square root of \(-1\) is \( i \), and its other square root is \(-i\).

How to Find the Square Root of Minus One?

To determine the square root of \(-1\), we use the property of imaginary numbers:

\[ \sqrt{-1} = i \]

This result stems from the definition of \( i \) as a number whose square is \(-1\).

Generalizing to Other Negative Numbers

The process of finding the square root of any negative number involves the imaginary unit \( i \). For any negative number \(-x\):

\[ \sqrt{-x} = i \sqrt{x} \]

Here, \( \sqrt{x} \) is the square root of the positive counterpart \( x \).

Examples

• Example 1: Finding the square root of \(-25\)

\[ \sqrt{-25} = \sqrt{-1 \cdot 25} = \sqrt{-1} \cdot \sqrt{25} = 5i \]

• Example 2: Finding the square root of \(-9\)

\[ \sqrt{-9} = \sqrt{9 \cdot -1} = \sqrt{9} \cdot \sqrt{-1} = 3i \]

Applications of Imaginary Numbers

Imaginary numbers, and more broadly complex numbers (which combine real and imaginary parts), have significant applications in various fields:

• Electrical engineering: Alternating current (AC) circuit analysis
• Signal processing: Fourier transforms for audio and image processing
• Mathematics: Solving equations that do not have real solutions
• Physics: Quantum mechanics and relativity theories

Conclusion

The concept of the square root of \(-1\) leads to the creation of imaginary numbers, opening a vast field of mathematics that extends into complex numbers, providing essential tools for science, engineering, and technology.

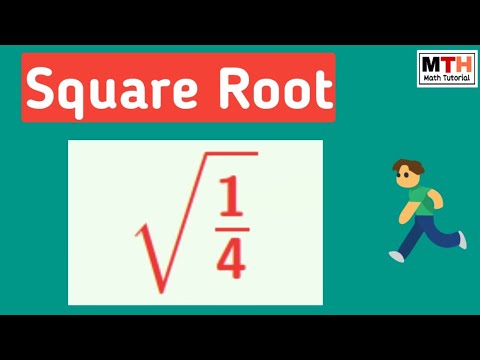
Square roots are fundamental mathematical operations used to find a number that, when multiplied by itself, yields the original number. The square root of a number \(x\) is denoted as \(\sqrt{x}\). For positive numbers, this is straightforward, but when dealing with negative numbers, the concept extends into the realm of complex numbers.

For instance, the square root of 1 is simply 1 because \(1 \times 1 = 1\). However, when we consider the square root of a negative number, such as -1, we need to introduce the concept of imaginary numbers. The imaginary unit is denoted by \(i\), where \(i^2 = -1\). Therefore, the square root of -1 is defined as \(i\), and the negative square root of -1 is \(-i\).

Imaginary numbers are a crucial part of complex numbers, which are numbers that have both a real part and an imaginary part. For example, a complex number can be written in the form \(a + bi\), where \(a\) and \(b\) are real numbers.

Square roots have various properties and rules that apply to them. For example, the square root of a product of two numbers is the product of the square roots of those numbers, but this property does not hold for negative numbers within the realm of real numbers.

In summary, understanding square roots, especially in the context of negative numbers, expands our mathematical toolkit and allows us to solve a wider range of equations and problems.

Imaginary numbers are a fundamental concept in mathematics, extending the number line to a complex plane. The unit imaginary number is denoted as \(i\), which is defined by the property:

\(i^2 = -1\)

This means that \(i\) is the principal square root of \(-1\). Imaginary numbers allow for the extension of the real number system to solve equations that have no real solutions.

Properties of Imaginary Numbers

• The imaginary unit \(i\) satisfies \(i = \sqrt{-1}\).
• Negative \(i\), or \(-i\), is also a square root of \(-1\).

Examples and Applications

Consider the equation \(x^2 + 1 = 0\). In the realm of real numbers, this equation has no solution because no real number squared results in a negative number. However, using imaginary numbers, we can solve it:

• First, rewrite the equation: \(x^2 = -1\).
• Taking the square root of both sides, we get \(x = \pm i\).

Thus, the solutions are \(x = i\) and \(x = -i\).

Combining Real and Imaginary Numbers

Imaginary numbers can be combined with real numbers to form complex numbers. A complex number is expressed in the form:

\(a + bi\)

where \(a\) and \(b\) are real numbers. For example, \(3 + 4i\) is a complex number where \(3\) is the real part and \(4i\) is the imaginary part.

Applications in Various Fields

Imaginary and complex numbers are used extensively in various fields such as engineering, physics, and signal processing. They are crucial in understanding phenomena in AC circuits, control theory, and in the representation of waves and oscillations.

Conclusion

Understanding imaginary numbers and their properties opens up a broader perspective in mathematics and its applications. They are not merely theoretical constructs but have practical uses that make complex problems solvable.

The imaginary unit, denoted as \(i\), is a mathematical concept that allows us to extend the real number system to include solutions to equations that have no real solutions. The fundamental property of the imaginary unit is that \(i\) is defined as the square root of \(-1\). This means that \(i^2 = -1\).

The introduction of the imaginary unit enables us to solve equations like \(x^2 + 1 = 0\). In the realm of real numbers, this equation has no solution because the square of a real number is always non-negative. However, using the imaginary unit, we can express the solutions as \(x = i\) and \(x = -i\).

Here is a step-by-step breakdown of how the imaginary unit is used in solving such equations:

1. Consider the equation \(x^2 + 1 = 0\).

2. Subtract 1 from both sides to get \(x^2 = -1\).

3. Take the square root of both sides: \(x = \pm \sqrt{-1}\).

4. Since \(\sqrt{-1} = i\), the solutions are \(x = \pm i\).

Thus, the equation \(x^2 + 1 = 0\) has two solutions in the complex number system: \(x = i\) and \(x = -i\).

In summary, the imaginary unit \(i\) is crucial in extending the number system to include complex numbers, which are numbers of the form \(a + bi\), where \(a\) and \(b\) are real numbers.

Understanding the concept of the negative square root of 1 involves delving into the realm of imaginary numbers. In mathematics, the square root of -1 is denoted by the imaginary unit \(i\), where \(i^2 = -1\). This concept is fundamental in complex number theory and has various applications in different fields of science and engineering.

To calculate the negative square root of 1, we follow these steps:

1. Recognize that the square roots of 1 are both 1 and -1, as \(1^2 = 1\) and \((-1)^2 = 1\).

2. When considering the negative square root, we introduce the imaginary unit \(i\). For negative numbers, the square root involves \(i\). Therefore, the square root of -1 is \(i\), and the negative square root is \(-i\).

In summary, the negative square root of 1, in the context of imaginary numbers, is \(-i\). This is crucial in complex number calculations and helps solve equations that are unsolvable within the realm of real numbers alone.

Understanding and working with the negative square root of 1 involves dealing with imaginary numbers. Here are some examples and exercises to help reinforce this concept:

Example 1: Simplifying Negative Square Roots

To find the negative square root of 1, we use the imaginary unit \( i \), where \( i = \sqrt{-1} \). Thus, the negative square root of 1 is \( -i \).

• Find the negative square root of -1: \( -\sqrt{1} = -i \)
• Simplify \( -\sqrt{4} \): Since \( \sqrt{4} = 2 \), \( -\sqrt{4} = -2i \)

Example 2: Solving Equations with Imaginary Numbers

Solve the equation \( x^2 + 1 = 0 \):

1. Subtract 1 from both sides: \( x^2 = -1 \)
2. Take the square root of both sides: \( x = \pm i \)

Exercise 1

Simplify the following:

1. \( -\sqrt{9} \)
2. \( -\sqrt{16} \)

Answers:

1. \( -\sqrt{9} = -3i \)
2. \( -\sqrt{16} = -4i \)

Exercise 2

Solve for \( x \) in the following equations:

1. \( x^2 + 4 = 0 \)
2. \( 2x^2 + 8 = 0 \)

Answers:

1. \( x^2 = -4 \Rightarrow x = \pm 2i \)
2. \( 2x^2 = -8 \Rightarrow x^2 = -4 \Rightarrow x = \pm 2i \)

There are several common misconceptions about the negative square root of 1 and imaginary numbers in general. Understanding these misconceptions is crucial for a clear grasp of the concept.

• Misconception 1: The square root of -1 is -1.

  Some might incorrectly assume that the square root of -1 is -1. In reality, the square root of -1 is an imaginary number represented as i, where i is defined as the imaginary unit.

• Misconception 2: Imaginary numbers are not real or useful.

  Despite their name, imaginary numbers are very real in the context of mathematics and engineering. They are used in complex number calculations, electrical engineering, and signal processing, among other fields.

• Misconception 3: Imaginary numbers are only theoretical.

  Imaginary numbers have practical applications in many areas such as control theory, quantum physics, and fluid dynamics. They are essential for solving certain types of equations that have no real solutions.

• Misconception 4: i is the only imaginary number.

  While i is the fundamental imaginary unit, any multiple of i is also an imaginary number. For example, 2i, 3i, and -i are all imaginary numbers.

• Misconception 5: Imaginary numbers cannot be graphed.

  Imaginary numbers can be graphed on the complex plane, where the x-axis represents the real part of the number and the y-axis represents the imaginary part. This is known as the Argand diagram.