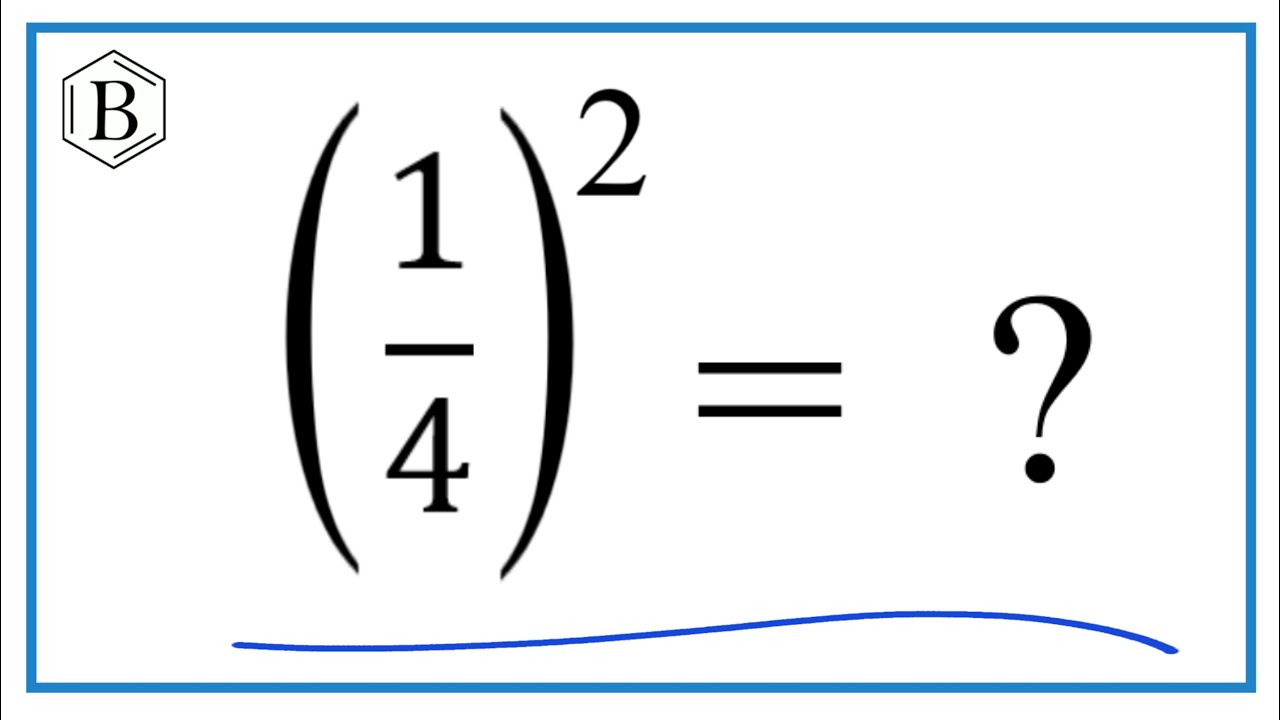Topic square root of 1 4th: The square root of 1/4 can be easily understood and calculated with simple mathematical principles. This guide will walk you through the steps to find the square root of this fraction, explain the underlying concepts, and provide practical examples to solidify your understanding. Dive in to master this fundamental math skill!
Table of Content
- Understanding the Square Root of 1/4
- Introduction to Square Roots
- Definition and Basic Properties of Square Roots
- Square Root Calculation Methods
- Simplifying Square Roots
- Perfect Squares and Non-Perfect Squares
- Fractional and Decimal Square Roots
- Step-by-Step Calculation of Square Root of 1/4
- Square Roots of Fractions
- Principal and Negative Square Roots
- Applications of Square Roots in Real Life
- Tools and Calculators for Finding Square Roots
- Frequently Asked Questions
- YOUTUBE: Video giải thích chi tiết về cách tính căn bậc hai của 1/4. Học cách đơn giản hóa và hiểu rõ hơn về các phép tính toán học liên quan đến căn bậc hai.
Understanding the Square Root of 1/4
The square root of a number is a value that, when multiplied by itself, gives the original number. For the fraction , we can find its square root using basic algebraic principles.
Step-by-Step Calculation
- Rewrite the fraction:
- Apply the square root to both the numerator and the denominator:
- Simplify the square roots:
Therefore, the square root of is .
Decimal Form
In decimal form, this is represented as:
Key Points to Remember
- The square root function essentially reverses the squaring process.
- For fractions, apply the square root to both the numerator and the denominator.
- The result should be simplified to its simplest form.
Applications
Understanding square roots is fundamental in various mathematical contexts, such as solving quadratic equations, understanding geometric properties, and in advanced mathematical fields such as calculus and complex numbers.
Further Reading and Tools

READ MORE:
Introduction to Square Roots
Square roots are fundamental elements in mathematics, involving the process of finding a number that, when multiplied by itself, yields the original number. The square root of a number \( x \) is denoted as \( \sqrt{x} \). For example, the square root of 9 is 3, because \( 3 \times 3 = 9 \). This principle applies to various mathematical fields, including algebra, geometry, and physics.
Square roots are represented using the radical symbol \( \sqrt{} \). For instance, \( \sqrt{16} = 4 \) because \( 4^2 = 16 \). In algebra, the concept of square roots extends to fractions and irrational numbers. The square root of 1/4 is \( \sqrt{1/4} = 1/2 \) because \( (1/2) \times (1/2) = 1/4 \).
Understanding square roots involves recognizing perfect squares, simplifying non-perfect squares, and applying the properties of square roots in various calculations. The square root of a product, for instance, can be simplified as \( \sqrt{a \times b} = \sqrt{a} \times \sqrt{b} \), which is particularly useful in algebraic manipulations.
Definition and Basic Properties of Square Roots
The square root of a number is a value that, when multiplied by itself, gives the original number. The square root is denoted by the radical symbol (√). For example, the square root of 16 is 4 because 4 × 4 = 16.
Here are some basic properties of square roots:
- Non-negative Property: Every non-negative number has a non-negative square root. For instance, √9 = 3.
- Principal Square Root: The principal square root of a number is the non-negative root. For example, although both 3 and -3 are square roots of 9, the principal square root is 3.
- Multiplication Property: The square root of a product is the product of the square roots. Mathematically, √(a * b) = √a * √b, where a and b are non-negative numbers.
- Division Property: The square root of a quotient is the quotient of the square roots. This is expressed as √(a / b) = √a / √b, provided b ≠ 0.
- Perfect Squares: A perfect square is an integer that is the square of another integer. Examples include 1, 4, 9, 16, 25, etc.
Let's take the example of finding the square root of 1/4:
- Write 1/4 as (1^2)/(2^2).
- Apply the square root to both the numerator and the denominator: √(1/4) = √(1^2/2^2) = √(1^2) / √(2^2).
- This simplifies to 1/2.
Therefore, the square root of 1/4 is 1/2. This example demonstrates the division property of square roots, making complex fractions easier to handle.
Square roots also have specific rules when dealing with negative numbers. The square root of a negative number involves imaginary numbers since no real number squared will result in a negative value. The imaginary unit is denoted by 'i', where i = √(-1).
Square Root Calculation Methods
Calculating square roots can be approached through various methods, each with its level of complexity and precision. Here are some common techniques used to find square roots:
- Prime Factorization
- Estimation and Averaging
- Using Calculators
Prime Factorization Method
Prime factorization involves breaking down the number into its prime factors and then pairing them to find the square root. For example, to find the square root of 36:
- Prime factors of 36: \(2 \times 2 \times 3 \times 3\)
- Group into pairs: \((2 \times 3) \times (2 \times 3)\)
- Square root: \(2 \times 3 = 6\)
Estimation and Averaging Method
This method uses an iterative approach to approximate the square root of a number:
- Estimate a number \(b\).
- Divide the original number by \(b\) to get \(c\).
- Average \(b\) and \(c\) to get a new estimate.
- Repeat steps 2 and 3 until the desired precision is achieved.
Example: Find \(\sqrt{27}\) to three decimal places:
- Guess: \(5.125\)
- \(27 \div 5.125 = 5.268\)
- Averaging: \((5.125 + 5.268) / 2 = 5.197\)
- Repeat: \(27 \div 5.197 = 5.195\)
- Averaging: \((5.195 + 5.197) / 2 = 5.196\)
- \(\sqrt{27} \approx 5.196\)
Using Calculators
Calculators provide a quick and accurate way to find square roots. The steps are simple:
- Enter the number into the calculator.
- Press the square root function (√).
- The calculator displays the square root.
For example, using an online calculator to find \(\sqrt{1/4}\) yields \(1/2\).
Simplifying Square Roots
Simplifying square roots involves breaking down the number inside the radical into its prime factors and finding pairs of these factors. This method helps to simplify the square root to its most basic form.
- Prime Factorization: Start by finding the prime factors of the number under the square root.
- Identify Pairs: Group the prime factors into pairs. Each pair can be taken out of the square root as a single number.
- Multiply: Multiply the numbers taken out of the square root. The remaining factors, if any, stay under the square root.
| Example | Simplification |
| \(\sqrt{50}\) | \(\sqrt{2 \times 5^2} = 5\sqrt{2}\) |
| \(\sqrt{72}\) | \(\sqrt{2^3 \times 3^2} = 6\sqrt{2}\) |
For fractional radicands, the same principles apply:
- Find the prime factorization of the numerator and the denominator separately.
- Apply the square root to both the numerator and the denominator.
- Simplify the resulting fraction.
| Example | Simplification |
| \(\sqrt{\frac{1}{4}}\) | \(\frac{\sqrt{1}}{\sqrt{4}} = \frac{1}{2}\) |
| \(\sqrt{\frac{9}{16}}\) | \(\frac{\sqrt{9}}{\sqrt{16}} = \frac{3}{4}\) |
By using these steps, you can simplify square roots to make calculations easier and expressions more manageable.
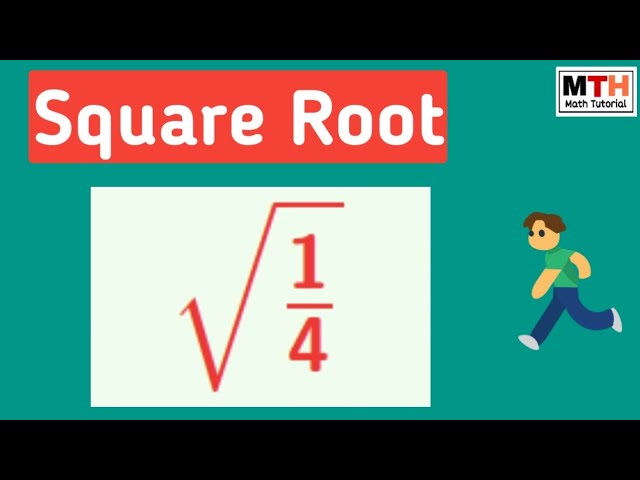
Perfect Squares and Non-Perfect Squares
Understanding the difference between perfect squares and non-perfect squares is crucial in simplifying square roots. A perfect square is a number that can be expressed as the product of an integer with itself. For example, \(1, 4, 9,\) and \(16\) are perfect squares because they can be written as \(1^2, 2^2, 3^2,\) and \(4^2\) respectively.
In contrast, non-perfect squares are numbers that cannot be expressed as the product of an integer with itself. Examples include \(2, 3, 5,\) and \(7\). The square roots of non-perfect squares are often irrational numbers, which cannot be expressed as a simple fraction and have non-repeating, non-terminating decimal expansions.
To simplify square roots of non-perfect squares, you often need to factor the number into its prime components and look for any perfect square factors. For example:
- \(\sqrt{18} = \sqrt{9 \times 2} = \sqrt{9} \times \sqrt{2} = 3\sqrt{2}\)
- \(\sqrt{50} = \sqrt{25 \times 2} = \sqrt{25} \times \sqrt{2} = 5\sqrt{2}\)
In these examples, the numbers \(9\) and \(25\) are perfect squares, making it possible to simplify the square roots by separating them from the non-perfect square factors.
On the other hand, square roots of perfect squares result in whole numbers:
- \(\sqrt{16} = 4\)
- \(\sqrt{81} = 9\)
Recognizing and simplifying square roots is a valuable skill in algebra, making calculations easier and expressions simpler.
Fractional and Decimal Square Roots
The square root of a fraction or a decimal follows the same principles as the square root of a whole number. It involves finding a number that, when multiplied by itself, gives the original fraction or decimal. Here is a step-by-step guide to understanding and calculating fractional and decimal square roots.
-
Calculating the Square Root of a Fraction
To find the square root of a fraction, such as , follow these steps:
- Rewrite the fraction under the square root sign:
- Apply the square root to the numerator and the denominator separately:
- Simplify the results:
Therefore, the square root of is .
-
Calculating the Square Root of a Decimal
To find the square root of a decimal, such as 0.25, follow these steps:
- Convert the decimal to a fraction: 0.25 =
- Simplify the fraction:
- Apply the square root to the simplified fraction:
1 4 1 2
Therefore, the square root of 0.25 is 0.5.
Step-by-Step Calculation of Square Root of 1/4
To find the square root of $\frac{1}{4}$, we will break down the steps using mathematical principles.
- Express the Fraction Under the Square Root:
First, we write the expression as a fraction under the square root symbol:
$\sqrt{\frac{1}{4}}$
- Apply the Square Root to Both Numerator and Denominator:
The square root of a fraction can be separated into the square root of the numerator and the square root of the denominator:
$\sqrt{\frac{1}{4}} = \frac{\sqrt{1}}{\sqrt{4}}$
- Calculate the Square Roots:
- The square root of 1 is 1, since $1 \times 1 = 1$.
- The square root of 4 is 2, since $2 \times 2 = 4$.
Substituting these values gives:
$\frac{\sqrt{1}}{\sqrt{4}} = \frac{1}{2}$
- Simplify the Fraction:
Since the square roots have been simplified to integers, the fraction is already in its simplest form:
$\sqrt{\frac{1}{4}} = \frac{1}{2}$
- Verify the Result:
To ensure our calculation is correct, we can square the result and check if we get the original fraction:
$\left(\frac{1}{2}\right)^2 = \frac{1}{4}$
This confirms that our solution is accurate.
Thus, the square root of $\frac{1}{4}$ is $\frac{1}{2}$.
Square Roots of Fractions
Finding the square root of fractions follows a similar process as finding the square root of whole numbers. We can simplify the square root of a fraction by applying the square root operation separately to the numerator and the denominator. Let’s explore this step-by-step.
- Understanding the Concept:
For any fraction $\frac{a}{b}$, where $a$ and $b$ are positive numbers, the square root of the fraction is given by:
$\sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}}$
This means we can find the square root of the numerator and the square root of the denominator separately, and then divide the results.
- Examples of Simplifying Square Roots of Fractions:
Let’s look at a few examples to illustrate this concept:
- Example 1:
Find the square root of $\frac{9}{16}$.
Calculate the square roots of the numerator and the denominator:
$\sqrt{9} = 3$ and $\sqrt{16} = 4$
Therefore, $\sqrt{\frac{9}{16}} = \frac{\sqrt{9}}{\sqrt{16}} = \frac{3}{4}$.
- Example 2:
Find the square root of $\frac{25}{49}$.
Calculate the square roots of the numerator and the denominator:
$\sqrt{25} = 5$ and $\sqrt{49} = 7$
Therefore, $\sqrt{\frac{25}{49}} = \frac{\sqrt{25}}{\sqrt{49}} = \frac{5}{7}$.
- Example 3:
Find the square root of $\frac{1}{64}$.
Calculate the square roots of the numerator and the denominator:
$\sqrt{1} = 1$ and $\sqrt{64} = 8$
Therefore, $\sqrt{\frac{1}{64}} = \frac{\sqrt{1}}{\sqrt{64}} = \frac{1}{8}$.
- Example 1:
- Handling Non-Perfect Squares:
If the numerator or the denominator is not a perfect square, we can still simplify the expression, but the result may not be a simple fraction.
- Example:
Find the square root of $\frac{2}{5}$.
Calculate the square roots separately:
$\sqrt{2} \approx 1.414$ and $\sqrt{5} \approx 2.236$
Therefore, $\sqrt{\frac{2}{5}} = \frac{\sqrt{2}}{\sqrt{5}} \approx \frac{1.414}{2.236} \approx 0.632$.
Alternatively, we can write it in radical form as $\frac{\sqrt{2}}{\sqrt{5}}$ or simplify it as $\sqrt{\frac{2}{5}}$.
- Example:
- General Formula for Square Roots of Fractions:
The general approach to finding the square root of a fraction $\frac{a}{b}$ is summarized as follows:
$\sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}}$
Where you can either calculate the decimal equivalents or leave the result in radical form for exact solutions.
- Special Case - Fraction with Unity:
If the numerator or denominator is 1, the calculation simplifies significantly:
- If the numerator is 1, $\sqrt{\frac{1}{b}} = \frac{1}{\sqrt{b}}$.
- If the denominator is 1, $\sqrt{\frac{a}{1}} = \sqrt{a}$.
Example: $\sqrt{\frac{1}{9}} = \frac{1}{3}$, since $\sqrt{9} = 3$.
Understanding how to find the square roots of fractions can simplify many mathematical problems, especially when dealing with ratios and proportions.

Principal and Negative Square Roots
The concept of square roots involves both the principal (positive) and the negative roots. When we take the square root of a number, we are looking for values that, when multiplied by themselves, give the original number. Let’s explore both types of square roots in detail.
- Understanding Principal (Positive) Square Roots:
The principal square root of a number is the non-negative root. It is the most commonly used and is represented using the square root symbol (√).
- Definition: The principal square root of a number $x$ is denoted as $\sqrt{x}$ and is always non-negative.
- Example: For $x = 4$, the principal square root is $\sqrt{4} = 2$ because $2 \times 2 = 4$.
- Fractions: For a fraction, the principal square root of $\frac{1}{4}$ is $\sqrt{\frac{1}{4}} = \frac{1}{2}$, since $\left(\frac{1}{2}\right)^2 = \frac{1}{4}$.
- Understanding Negative Square Roots:
The negative square root is simply the negative counterpart of the principal square root. It is not commonly written with the square root symbol alone but can be represented as $-\sqrt{x}$.
- Definition: The negative square root of a number $x$ is $-\sqrt{x}$.
- Example: For $x = 4$, the negative square root is $-\sqrt{4} = -2$ because $(-2) \times (-2) = 4$.
- Fractions: For the fraction $\frac{1}{4}$, the negative square root is $-\sqrt{\frac{1}{4}} = -\frac{1}{2}$, since $\left(-\frac{1}{2}\right)^2 = \frac{1}{4}$.
- Combining Both Roots:
When solving equations or working with square roots, it's important to consider both the principal and negative roots.
- Example: To solve $x^2 = 9$, we find both $x = 3$ and $x = -3$ because both $3 \times 3 = 9$ and $(-3) \times (-3) = 9$.
- Fractions: For the fraction $\frac{1}{4}$, the solutions are $x = \frac{1}{2}$ and $x = -\frac{1}{2}$.
- Representation in Equations:
In equations, both the principal and negative roots are often represented together using the $\pm$ symbol (plus-minus). For example:
$x = \pm\sqrt{4} \rightarrow x = \pm 2$
For the fraction $\frac{1}{4}$, this is written as:
$x = \pm\sqrt{\frac{1}{4}} \rightarrow x = \pm \frac{1}{2}$
Understanding both the principal and negative square roots allows for a complete solution set in many mathematical problems, especially when dealing with quadratic equations and complex number systems.
Applications of Square Roots in Real Life
Square roots are not just abstract mathematical concepts; they have practical applications in various aspects of real life. From engineering to finance, understanding square roots helps solve real-world problems. Here are some key areas where square roots are applied:
- Engineering and Construction:
Square roots are fundamental in engineering and construction. They are used in calculating dimensions and areas, especially when dealing with right triangles and the Pythagorean theorem.
- Example: To determine the length of a diagonal in a rectangular room, we can use the Pythagorean theorem, where the diagonal length $d$ is found by:
$d = \sqrt{l^2 + w^2}$
where $l$ is the length and $w$ is the width of the room.
- Physics and Motion:
In physics, square roots are essential for calculations involving speed, acceleration, and other dynamic properties. They help in deriving equations of motion and understanding wave behaviors.
- Example: The root mean square (RMS) value is used to calculate the effective value of alternating current (AC) voltage or current:
$V_{\text{RMS}} = \frac{V_{\text{peak}}}{\sqrt{2}}$
where $V_{\text{peak}}$ is the peak voltage.
- Finance and Economics:
Square roots are used in financial models and risk assessments. They help in calculating the standard deviation, which measures the volatility or risk of investment returns.
- Example: The standard deviation of a set of returns $R$ is calculated as:
$\sigma = \sqrt{\frac{\sum (R_i - \bar{R})^2}{N}}$
where $\bar{R}$ is the mean return, and $N$ is the number of returns.
- Geometry and Area Calculations:
Square roots are crucial in finding the side lengths of squares and determining the dimensions of geometric shapes when the area is known.
- Example: To find the side length of a square when its area $A$ is given, we use:
$s = \sqrt{A}$
If the area of a square is 16 square units, the side length is $\sqrt{16} = 4$ units.
- Astronomy and Space Science:
Square roots help in calculating distances and velocities in space. They are used in formulas for gravitational force and escape velocity.
- Example: The escape velocity $v_e$ from a planet's surface is given by:
$v_e = \sqrt{\frac{2GM}{R}}$
where $G$ is the gravitational constant, $M$ is the mass of the planet, and $R$ is the radius of the planet.
- Computer Graphics and Animation:
Square roots are used in graphics for calculating distances and scaling. They help in rendering images, animations, and 3D modeling.
- Example: In 3D graphics, to calculate the distance between two points $(x_1, y_1, z_1)$ and $(x_2, y_2, z_2)$, the distance $d$ is:
$d = \sqrt{(x_2 - x_1)^2 + (y_2 - y_1)^2 + (z_2 - z_1)^2}$
- Medicine and Pharmacology:
In medical fields, square roots are used in dosage calculations and in analyzing physiological data. They assist in standardizing doses and evaluating growth patterns.
- Example: Body surface area (BSA) for dosage calculations can be estimated using the square root formula:
$BSA = \sqrt{\frac{Height \times Weight}{3600}}$
where height is in centimeters and weight is in kilograms.
Square roots are integral to numerous disciplines, making them a valuable tool in solving practical problems and advancing scientific understanding.
Tools and Calculators for Finding Square Roots
Calculating square roots manually can be time-consuming and challenging, especially for non-perfect squares. Fortunately, there are numerous tools and calculators available to simplify this process. Here, we will explore various options, from online calculators to built-in tools in software applications, and explain how they can be used effectively.
- Online Square Root Calculators:
There are many free online calculators that can quickly compute the square root of any number, including fractions like $\frac{1}{4}$. These calculators are easy to use and accessible from any device with an internet connection.
- Example: To find the square root of $\frac{1}{4}$ using an online calculator:
- Open the calculator website or app.
- Enter the fraction or decimal value. For $\frac{1}{4}$, you can enter 0.25.
- Press the "Calculate" or "√" button to get the result.
- The calculator will display $\sqrt{\frac{1}{4}} = 0.5$.
- Calculator.net
- RapidTables
- Symbolab
Some popular online calculators include:
- Built-in Calculator Apps:
Most operating systems and smartphones come with a built-in calculator app that can perform square root calculations.
- Example: Using the built-in calculator on a smartphone:
- Open the calculator app.
- Switch to scientific mode if necessary.
- Enter the number 0.25 for $\frac{1}{4}$.
- Tap the square root (√) button.
- The app will display 0.5 as the square root of $\frac{1}{4}$.
- iPhone Calculator
- Android Calculator
- Windows Calculator
Popular devices with built-in calculator apps include:
- Spreadsheet Software:
Programs like Microsoft Excel, Google Sheets, and Apple Numbers include functions to calculate square roots, making them handy for more complex or repetitive calculations.
- Example: Finding the square root in Microsoft Excel:
- Open a new or existing Excel spreadsheet.
- Enter the number 0.25 in a cell (e.g., A1).
- In another cell, enter the formula:
=SQRT(A1). - Press Enter. The cell will display 0.5, which is the square root of $\frac{1}{4}$.
Similar steps can be followed in Google Sheets or Apple Numbers using their square root functions.
- Scientific Calculators:
Physical scientific calculators are designed to handle a wide range of mathematical functions, including square roots. They are ideal for students and professionals who frequently need to perform such calculations.
- Example: Using a scientific calculator to find $\sqrt{\frac{1}{4}}$:
- Turn on the calculator.
- Enter 0.25 for $\frac{1}{4}$.
- Press the square root (√) button.
- The display will show 0.5.
- Casio FX series
- Texas Instruments TI series
- HP Scientific Calculators
Common models include:
- Programming Languages:
For those familiar with programming, many languages provide built-in functions to calculate square roots. This is particularly useful in complex or iterative calculations.
- Example: Calculating the square root in Python:
- Open your Python IDE or a Jupyter notebook.
- Type the following code:
- Run the code. It will return 0.5 as the square root of $\frac{1}{4}$.
import math math.sqrt(0.25)Other languages like JavaScript, R, and MATLAB also have similar square root functions.
With these tools and calculators, finding the square roots of numbers, including fractions like $\frac{1}{4}$, becomes quick and easy. Whether you prefer online tools, built-in apps, or programming solutions, there is a method to suit every need.
Frequently Asked Questions
Understanding square roots, particularly of fractions like $\frac{1}{4}$, can raise several questions. Here, we address some common inquiries to clarify these concepts:
- What is the square root of $\frac{1}{4}$?
The square root of $\frac{1}{4}$ is $\frac{1}{2}$. This is because $(\frac{1}{2}) \times (\frac{1}{2}) = \frac{1}{4}$. In decimal form, $\frac{1}{4}$ is 0.25, and its square root is 0.5.
- How do you find the square root of a fraction?
To find the square root of a fraction $\frac{a}{b}$, take the square root of the numerator and the denominator separately:
$\sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}}$
For example, to find $\sqrt{\frac{1}{4}}$:
$\sqrt{\frac{1}{4}} = \frac{\sqrt{1}}{\sqrt{4}} = \frac{1}{2}$
- Can square roots be negative?
Yes, every positive number has both a positive and a negative square root. For instance, $\sqrt{4}$ can be $2$ or $-2$, since both $2 \times 2$ and $(-2) \times (-2)$ equal $4$. In the case of fractions like $\frac{1}{4}$, the square roots are $\frac{1}{2}$ and $-\frac{1}{2}$.
- Why is the principal square root always non-negative?
The principal square root of a number is defined as the non-negative root to provide a consistent and single-valued function. For example, the principal square root of 9 is 3, denoted as $\sqrt{9} = 3$. This avoids ambiguity, especially in mathematical and computational contexts.
- How do calculators compute square roots?
Calculators use various numerical methods to approximate square roots. Some common methods include the Newton-Raphson method and iterative algorithms. These methods quickly converge to the accurate square root value by performing a series of calculations.
- How is the square root symbol (√) used?
The square root symbol (√) is used to denote the principal square root of a number. For example, $\sqrt{25} = 5$. When dealing with fractions, the symbol can be applied to the entire fraction or separately to the numerator and denominator, such as $\sqrt{\frac{1}{4}} = \frac{\sqrt{1}}{\sqrt{4}} = \frac{1}{2}$.
- What are perfect squares?
A perfect square is a number that is the square of an integer. For example, $16$ is a perfect square because it equals $4 \times 4$. Similarly, $\frac{1}{4}$ is a perfect square because it equals $(\frac{1}{2}) \times (\frac{1}{2})$.
- Can square roots be simplified?
Yes, square roots can often be simplified. For example, $\sqrt{50}$ can be simplified to $5\sqrt{2}$. For fractions, simplifying might involve reducing the fraction first and then taking the square root. For instance, $\sqrt{\frac{4}{16}} = \sqrt{\frac{1}{4}} = \frac{1}{2}$.
- How are square roots used in real-life applications?
Square roots are used in various fields such as engineering, physics, finance, and everyday calculations. For example, they are essential in determining the dimensions of a square area, analyzing financial risks, calculating signal strengths, and solving problems involving rates and areas.
- Are there tools for calculating square roots?
Yes, many tools are available to calculate square roots, including online calculators, built-in apps on smartphones, spreadsheet software, scientific calculators, and programming languages. These tools provide quick and accurate square root calculations for any number, including fractions.
If you have more questions about square roots or need further clarification, don't hesitate to explore additional resources or ask a math expert.
Video giải thích chi tiết về cách tính căn bậc hai của 1/4. Học cách đơn giản hóa và hiểu rõ hơn về các phép tính toán học liên quan đến căn bậc hai.
Căn bậc hai của 1/4 | Root(1/4)
READ MORE:
Video hướng dẫn cách đơn giản hóa phân số dưới dấu căn bậc hai. Khám phá các mẹo toán học hữu ích để giải các bài toán căn bậc hai nhanh chóng và dễ dàng.
Đơn giản hóa phân số dưới căn bậc hai - Mẹo hợp pháp trong toán học