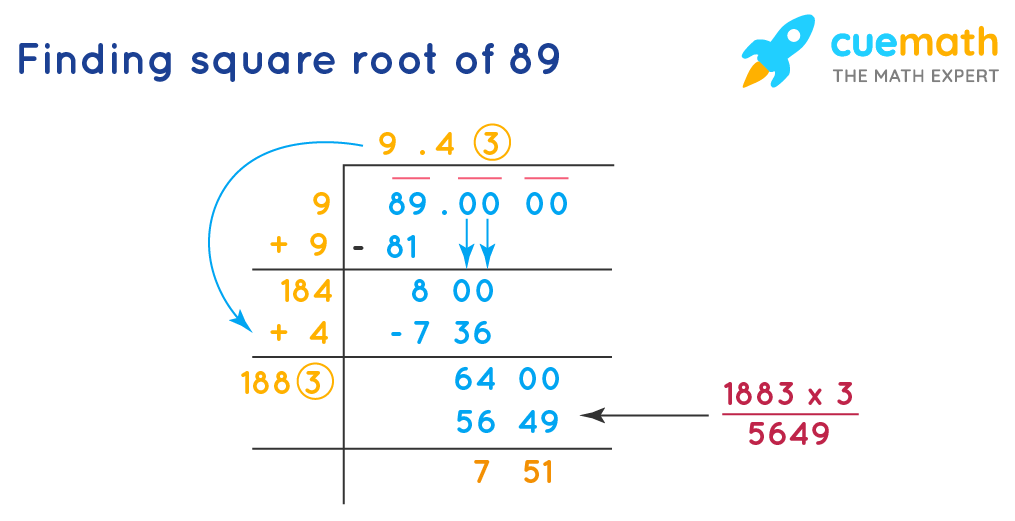
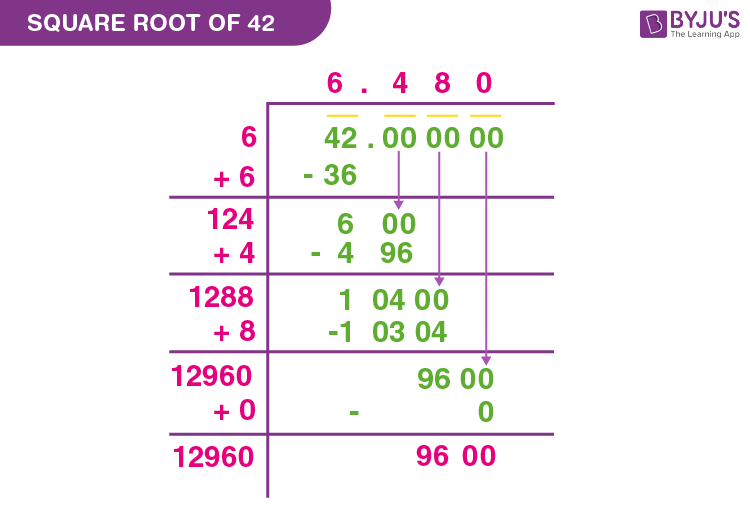
Topic square root simplest form: Unlock the secrets of simplifying square roots with our comprehensive guide. Learn the step-by-step process to find the simplest form, making complex calculations a breeze. Perfect for students and math enthusiasts, our easy-to-follow instructions and examples will help you master this essential math skill quickly and confidently.
Table of Content
- Square Root Simplest Form
- Introduction to Square Roots
- Understanding Simplest Form
- Steps to Simplify Square Roots
- Prime Factorization Method
- Perfect Squares and Simplification
- Examples of Simplifying Square Roots
- Special Cases in Square Root Simplification
- Simplifying Non-Perfect Squares
- Simplifying Fractions with Square Roots
- Common Mistakes to Avoid
- Practice Problems
- Applications in Real Life
- Additional Resources and References
- YOUTUBE:
Square Root Simplest Form
Finding the square root of a number and expressing it in its simplest form is an essential skill in mathematics. This involves simplifying the square root to its most reduced form, making it easier to understand and work with.
Steps to Simplify a Square Root
- Find the Prime Factors: Break down the number under the square root into its prime factors.
- Pair the Prime Factors: Group the prime factors into pairs.
- Simplify the Square Root: For each pair of prime factors, take one factor out of the square root.
- Multiply the Factors: Multiply the factors taken out of the square root to get the simplified form.
Example
Simplify the square root of 72:
- Step 1: Find the prime factors of 72: \(72 = 2 \times 2 \times 2 \times 3 \times 3\)
- Step 2: Group the prime factors into pairs: \((2 \times 2) \times (3 \times 3) \times 2\)
- Step 3: Simplify the square root: \(\sqrt{72} = \sqrt{(2^2) \times (3^2) \times 2}\)
- Step 4: Multiply the factors: \(\sqrt{72} = 2 \times 3 \times \sqrt{2} = 6\sqrt{2}\)
Thus, the simplest form of \(\sqrt{72}\) is \(6\sqrt{2}\).
Additional Examples
| Original Square Root | Simplest Form |
|---|---|
| \(\sqrt{50}\) | \(5\sqrt{2}\) |
| \(\sqrt{18}\) | \(3\sqrt{2}\) |
| \(\sqrt{98}\) | \(7\sqrt{2}\) |
Special Cases
When the number is a perfect square, the square root simplifies to an integer:
- \(\sqrt{16} = 4\)
- \(\sqrt{81} = 9\)
If the number is a prime number under the square root, it cannot be simplified further:
- \(\sqrt{7}\) remains \(\sqrt{7}\)
- \(\sqrt{11}\) remains \(\sqrt{11}\)
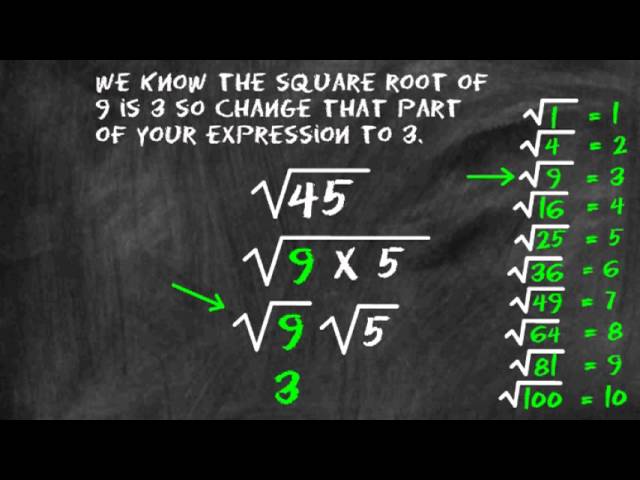
READ MORE:
Introduction to Square Roots
The square root is a fundamental concept in mathematics that involves finding a number which, when multiplied by itself, gives the original number. This concept is crucial in various fields such as algebra, geometry, and calculus.
Symbolically, the square root of a number \( x \) is denoted as \( \sqrt{x} \). For example, \( \sqrt{16} = 4 \) because \( 4 \times 4 = 16 \).
Understanding square roots involves several key points:
- Definition: The square root of a number \( x \) is a value that, when squared, equals \( x \).
- Notation: The radical symbol \( \sqrt{\phantom{x}} \) is used to denote the square root.
- Positive and Negative Roots: While every positive number has two square roots (one positive and one negative), the principal square root is the positive one.
- Perfect Squares: Numbers like 1, 4, 9, 16, 25, etc., are perfect squares because their square roots are whole numbers.
Here are some examples to illustrate:
| Number | Square Root |
|---|---|
| 1 | \(\sqrt{1} = 1\) |
| 4 | \(\sqrt{4} = 2\) |
| 9 | \(\sqrt{9} = 3\) |
| 16 | \(\sqrt{16} = 4\) |
| 25 | \(\sqrt{25} = 5\) |
For non-perfect squares, the square root is an irrational number, meaning it cannot be expressed as a simple fraction. For instance:
- \(\sqrt{2} \approx 1.414\)
- \(\sqrt{3} \approx 1.732\)
- \(\sqrt{5} \approx 2.236\)
Understanding square roots lays the foundation for learning more advanced mathematical concepts and solving complex problems effectively.
Understanding Simplest Form
The simplest form of a square root is a way of expressing the square root in its most reduced and basic form. This involves removing any perfect square factors from under the radical sign to make the expression as straightforward as possible.
Here is a step-by-step guide to simplify a square root:
-
Find the Prime Factors:
Decompose the number under the square root into its prime factors. For example, for \( \sqrt{72} \), the prime factors of 72 are \( 2 \times 2 \times 2 \times 3 \times 3 \).
-
Group the Prime Factors:
Pair the prime factors in groups of two. Using the previous example: \( (2 \times 2) \times (3 \times 3) \times 2 \).
-
Simplify the Square Root:
Take one factor out of each pair and place it outside the square root. Thus, \( \sqrt{72} = \sqrt{(2^2) \times (3^2) \times 2} = 2 \times 3 \times \sqrt{2} \).
-
Multiply the Factors:
Multiply the factors outside the square root to get the simplest form. In this case, \( 2 \times 3 = 6 \), so \( \sqrt{72} = 6\sqrt{2} \).
Below is a table illustrating the simplification of various square roots:
| Original Square Root | Simplified Form |
|---|---|
| \(\sqrt{50}\) | \(5\sqrt{2}\) |
| \(\sqrt{18}\) | \(3\sqrt{2}\) |
| \(\sqrt{98}\) | \(7\sqrt{2}\) |
| \(\sqrt{45}\) | \(3\sqrt{5}\) |
| \(\sqrt{200}\) | \(10\sqrt{2}\) |
By following these steps, you can simplify any square root to its simplest form, making it easier to work with in mathematical problems and applications.
Steps to Simplify Square Roots
Simplifying square roots involves breaking down the number inside the radical sign into its prime factors and then reducing it to its simplest form. Here is a detailed, step-by-step guide to help you simplify square roots effectively:
-
Find the Prime Factors:
Break down the number under the square root into its prime factors. For example, for \( \sqrt{72} \), the prime factors of 72 are \( 2 \times 2 \times 2 \times 3 \times 3 \).
-
Group the Prime Factors:
Organize the prime factors into pairs. Using the example above: \( (2 \times 2) \times (3 \times 3) \times 2 \).
-
Simplify the Square Root:
Take one factor out of each pair and place it outside the square root. For instance, \( \sqrt{72} = \sqrt{(2^2) \times (3^2) \times 2} \).
-
Multiply the Factors Outside the Radical:
Multiply the factors that were taken out of the square root to get the simplest form. In this example, \( 2 \times 3 = 6 \), so \( \sqrt{72} = 6\sqrt{2} \).
Let's look at a few more examples to illustrate the process:
| Original Square Root | Prime Factorization | Grouping | Simplified Form |
|---|---|---|---|
| \(\sqrt{50}\) | \(2 \times 5 \times 5\) | \((5^2) \times 2\) | \(5\sqrt{2}\) |
| \(\sqrt{18}\) | \(2 \times 3 \times 3\) | \((3^2) \times 2\) | \(3\sqrt{2}\) |
| \(\sqrt{98}\) | \(2 \times 7 \times 7\) | \((7^2) \times 2\) | \(7\sqrt{2}\) |
| \(\sqrt{45}\) | \(3 \times 3 \times 5\) | \((3^2) \times 5\) | \(3\sqrt{5}\) |
| \(\sqrt{200}\) | \(2 \times 2 \times 2 \times 5 \times 5\) | \((2^2) \times (5^2) \times 2\) | \(10\sqrt{2}\) |
By following these steps, you can simplify any square root to its simplest form, making complex calculations more manageable and easier to understand.
Prime Factorization Method
The prime factorization method is a powerful technique for simplifying square roots. It involves breaking down the number under the square root into its prime factors and using these factors to simplify the expression. Here is a detailed step-by-step guide:
-
Find the Prime Factors:
Start by determining the prime factors of the number under the square root. Prime factors are the prime numbers that multiply together to give the original number. For example, for \( \sqrt{72} \), the prime factors of 72 are \( 2 \times 2 \times 2 \times 3 \times 3 \).
-
Group the Prime Factors:
Organize the prime factors into pairs. Each pair of identical factors can be simplified out of the square root. Using the example above: \( (2 \times 2) \times (3 \times 3) \times 2 \).
-
Simplify the Square Root:
Take one factor out of each pair and place it outside the square root. For instance, \( \sqrt{72} = \sqrt{(2^2) \times (3^2) \times 2} \). Taking one 2 and one 3 out of the square root, we get \( 2 \times 3 \times \sqrt{2} \).
-
Multiply the Factors Outside the Radical:
Multiply the factors that were taken out of the square root to get the simplest form. In this example, \( 2 \times 3 = 6 \), so \( \sqrt{72} = 6\sqrt{2} \).
Below is a table illustrating the prime factorization method for various square roots:
| Original Square Root | Prime Factorization | Grouping | Simplified Form |
|---|---|---|---|
| \(\sqrt{50}\) | \(2 \times 5 \times 5\) | \((5^2) \times 2\) | \(5\sqrt{2}\) |
| \(\sqrt{18}\) | \(2 \times 3 \times 3\) | \((3^2) \times 2\) | \(3\sqrt{2}\) |
| \(\sqrt{98}\) | \(2 \times 7 \times 7\) | \((7^2) \times 2\) | \(7\sqrt{2}\) |
| \(\sqrt{45}\) | \(3 \times 3 \times 5\) | \((3^2) \times 5\) | \(3\sqrt{5}\) |
| \(\sqrt{200}\) | \(2 \times 2 \times 2 \times 5 \times 5\) | \((2^2) \times (5^2) \times 2\) | \(10\sqrt{2}\) |
By following these steps and using the prime factorization method, you can simplify any square root to its simplest form, making it easier to work with in mathematical calculations.

Perfect Squares and Simplification
A perfect square is a number that can be expressed as the product of an integer with itself. Recognizing perfect squares is crucial in simplifying square roots, as they allow for straightforward simplification. Here's a detailed guide on perfect squares and how to simplify them:
-
Identify Perfect Squares:
Perfect squares are numbers like 1, 4, 9, 16, 25, 36, 49, 64, 81, 100, etc. Each of these numbers can be written as the square of an integer:
- \(1 = 1 \times 1\)
- \(4 = 2 \times 2\)
- \(9 = 3 \times 3\)
- \(16 = 4 \times 4\)
- \(25 = 5 \times 5\)
- ...and so on.
-
Simplify Square Roots of Perfect Squares:When taking the square root of a perfect square, the result is simply the integer that was squared. For example:
- \(\sqrt{1} = 1\)
- \(\sqrt{4} = 2\)
- \(\sqrt{9} = 3\)
- \(\sqrt{16} = 4\)
- \(\sqrt{25} = 5\)
-
Simplify Non-Perfect Squares:For numbers that are not perfect squares, break them down into their prime factors and identify any perfect square factors within them. For instance:
- \(\sqrt{50} = \sqrt{25 \times 2} = \sqrt{25} \times \sqrt{2} = 5\sqrt{2}\)
- \(\sqrt{72} = \sqrt{36 \times 2} = \sqrt{36} \times \sqrt{2} = 6\sqrt{2}\)
- \(\sqrt{98} = \sqrt{49 \times 2} = \sqrt{49} \times \sqrt{2} = 7\sqrt{2}\)
Here is a table summarizing the simplification of various square roots involving perfect squares:
| Original Square Root | Simplified Form |
|---|---|
| \(\sqrt{36}\) | \(6\) |
| \(\sqrt{100}\) | \(10\) |
| \(\sqrt{144}\) | \(12\) |
| \(\sqrt{50}\) | \(5\sqrt{2}\) |
| \(\sqrt{72}\) | \(6\sqrt{2}\) |
| \(\sqrt{98}\) | \(7\sqrt{2}\) |
Understanding perfect squares and how to simplify them helps in simplifying more complex square roots and makes calculations easier and more intuitive.
Examples of Simplifying Square Roots
In this section, we will explore several examples of simplifying square roots step by step. These examples will help you understand the process and apply the techniques effectively.
Example 1: Simplifying √50
- Start by finding the prime factorization of 50:
- 50 = 2 × 5 × 5
- Group the prime factors into pairs:
- √(2 × 52)
- Take the square root of each pair of prime factors:
- √50 = √(2 × 52) = 5√2
Example 2: Simplifying √72
- Find the prime factorization of 72:
- 72 = 2 × 2 × 2 × 3 × 3
- Group the prime factors into pairs:
- √(23 × 32)
- Simplify by taking the square root of each pair:
- √72 = √(23 × 32) = √(2 × 22 × 32) = 6√2
Example 3: Simplifying √18
- Find the prime factorization of 18:
- 18 = 2 × 3 × 3
- Group the prime factors into pairs:
- √(2 × 32)
- Simplify by taking the square root of each pair:
- √18 = √(2 × 32) = 3√2
Example 4: Simplifying √200
- Find the prime factorization of 200:
- 200 = 2 × 2 × 2 × 5 × 5
- Group the prime factors into pairs:
- √(23 × 52)
- Simplify by taking the square root of each pair:
- √200 = √(2 × 22 × 52) = 10√2
Example 5: Simplifying √32
- Find the prime factorization of 32:
- 32 = 2 × 2 × 2 × 2 × 2
- Group the prime factors into pairs:
- √(25)
- Simplify by taking the square root of each pair:
- √32 = √(24 × 2) = 4√2
Special Cases in Square Root Simplification
Simplifying square roots can sometimes involve special cases that require particular methods or attention. Here, we'll explore some of these special cases and the steps to handle them.
1. Simplifying Square Roots of Perfect Squares
Perfect squares are numbers whose square roots are whole numbers. For example:
- \(\sqrt{16} = 4\)
- \(\sqrt{25} = 5\)
Since the square root of a perfect square is an integer, no further simplification is needed.
2. Square Roots of Products
When simplifying the square root of a product, use the property \(\sqrt{a \times b} = \sqrt{a} \times \sqrt{b}\). For example:
- \(\sqrt{12} = \sqrt{4 \times 3} = \sqrt{4} \times \sqrt{3} = 2\sqrt{3}\)
- \(\sqrt{45} = \sqrt{9 \times 5} = \sqrt{9} \times \sqrt{5} = 3\sqrt{5}\)
3. Square Roots of Fractions
To simplify the square root of a fraction, use the property \(\sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}}\). For example:
- \(\sqrt{\frac{25}{4}} = \frac{\sqrt{25}}{\sqrt{4}} = \frac{5}{2}\)
- \(\sqrt{\frac{18}{2}} = \sqrt{9} = 3\)
4. Square Roots Involving Variables
When variables are involved, remember to apply the same rules and consider the domain of the variable. For instance:
- \(\sqrt{x^2} = |x|\)
- \(\sqrt{a^2b} = a\sqrt{b}\)
5. Irrational Square Roots (Surds)
Some square roots cannot be simplified into a whole number or a simpler radical form. These are called surds. For example:
- \(\sqrt{2}\)
- \(\sqrt{3}\)
These values are left in their radical form as they cannot be simplified further.
6. Multiplying and Adding Radicals
When multiplying or adding square roots, combine like terms and simplify each radical before performing the operation. For example:
- \(\sqrt{6} \times \sqrt{15} = \sqrt{90} = 3\sqrt{10}\)
- \(2\sqrt{12} + 9\sqrt{3} = 4\sqrt{3} + 9\sqrt{3} = 13\sqrt{3}\)
7. Handling Complex Cases
Some expressions require multiple steps to simplify. For example:
- \(\sqrt{20} \times \sqrt{5\sqrt{2}} = \sqrt{20 \times 5\sqrt{2}} = \sqrt{100\sqrt{2}} = 10\sqrt[4]{2}\)
By following these methods, you can simplify square roots effectively, even in more complex scenarios.
Simplifying Non-Perfect Squares
Simplifying non-perfect square roots involves breaking down the number inside the square root into its prime factors and then simplifying the expression by factoring out any perfect squares. Here is a detailed step-by-step guide to help you simplify non-perfect square roots:
-
Identify the Prime Factors: Break down the number inside the square root into its prime factors.
Example: Simplify \( \sqrt{50} \)
- 50 can be factored into 2 and 25 (i.e., 50 = 2 × 25)
- 25 is a perfect square (i.e., 25 = 5 × 5)
-
Separate the Perfect Squares: Identify and separate the perfect squares from the prime factors.
Example: \( \sqrt{50} = \sqrt{2 \times 25} \)
-
Apply the Square Root Property: Use the property \( \sqrt{a \times b} = \sqrt{a} \times \sqrt{b} \) to simplify.
Example: \( \sqrt{50} = \sqrt{2 \times 25} = \sqrt{2} \times \sqrt{25} \)
-
Simplify the Perfect Squares: Simplify the square root of the perfect squares.
Example: \( \sqrt{25} = 5 \)
Therefore, \( \sqrt{50} = \sqrt{2} \times 5 = 5\sqrt{2} \)
Here are more examples to illustrate the process:
-
Example 1: Simplify \( \sqrt{18} \)
- Factor 18 into prime factors: 18 = 2 × 9
- Identify the perfect square: 9 (i.e., 9 = 3 × 3)
- Separate the perfect square: \( \sqrt{18} = \sqrt{2 \times 9} \)
- Apply the property: \( \sqrt{18} = \sqrt{2} \times \sqrt{9} \)
- Simplify the perfect square: \( \sqrt{9} = 3 \)
- Final result: \( \sqrt{18} = 3\sqrt{2} \)
-
Example 2: Simplify \( \sqrt{72} \)
- Factor 72 into prime factors: 72 = 2 × 36
- Identify the perfect square: 36 (i.e., 36 = 6 × 6)
- Separate the perfect square: \( \sqrt{72} = \sqrt{2 \times 36} \)
- Apply the property: \( \sqrt{72} = \sqrt{2} \times \sqrt{36} \)
- Simplify the perfect square: \( \sqrt{36} = 6 \)
- Final result: \( \sqrt{72} = 6\sqrt{2} \)
By following these steps, you can simplify any non-perfect square root to its simplest radical form.

Simplifying Fractions with Square Roots
Simplifying fractions that contain square roots can be done by following a few steps to ensure that the fraction is in its simplest form. Here’s a detailed guide:
-
Identify the Square Roots: Look at the fraction and identify the square roots in both the numerator and the denominator.
For example, consider the fraction: \( \frac{\sqrt{18}}{\sqrt{2}} \).
-
Combine the Square Roots: Use the property of square roots to combine them into a single square root.
\[
\frac{\sqrt{18}}{\sqrt{2}} = \sqrt{\frac{18}{2}}
\] -
Simplify the Fraction Inside the Square Root: Simplify the fraction inside the square root.
\[
\sqrt{\frac{18}{2}} = \sqrt{9}
\] -
Calculate the Square Root: Calculate the square root of the simplified fraction.
\[
\sqrt{9} = 3
\]
Thus, \( \frac{\sqrt{18}}{\sqrt{2}} \) simplifies to \( 3 \).
More Examples
Here are a few more examples to illustrate the process:
-
Example 1: Simplify \( \frac{\sqrt{50}}{\sqrt{2}} \).
\[
\frac{\sqrt{50}}{\sqrt{2}} = \sqrt{\frac{50}{2}} = \sqrt{25} = 5
\] -
Example 2: Simplify \( \frac{\sqrt{32}}{\sqrt{8}} \).
\[
\frac{\sqrt{32}}{\sqrt{8}} = \sqrt{\frac{32}{8}} = \sqrt{4} = 2
\]
Simplifying with Rationalization
Sometimes, you may need to rationalize the denominator to simplify the fraction. This involves multiplying the numerator and the denominator by a value that will eliminate the square root in the denominator.
-
Identify the Square Root in the Denominator: For example, simplify \( \frac{5}{\sqrt{3}} \).
-
Multiply by a Conjugate: Multiply the numerator and the denominator by \( \sqrt{3} \) to rationalize the denominator.
\[
\frac{5}{\sqrt{3}} \times \frac{\sqrt{3}}{\sqrt{3}} = \frac{5\sqrt{3}}{3}
\]
Thus, \( \frac{5}{\sqrt{3}} \) simplifies to \( \frac{5\sqrt{3}}{3} \).
These steps will help you simplify fractions with square roots effectively. Practice with different examples to get more comfortable with the process.
Common Mistakes to Avoid
When simplifying square roots, it's essential to avoid common mistakes to ensure accuracy and understanding. Here are some frequent pitfalls and how to avoid them:
- Forgetting to simplify completely:
Ensure that the radical expression is fully simplified by factoring the number under the radical sign into its prime factors and extracting all possible square roots.
- Ignoring prime factorization:
Skipping the step of breaking down the number into its prime factors can lead to incomplete simplification. Always factorize the number to identify which square roots can be extracted.
- Misunderstanding the square root of a product:
Remember that \(\sqrt{ab} = \sqrt{a} \times \sqrt{b}\). Misapplying this property can lead to incorrect results.
- Confusing addition and multiplication:
A common misconception is that \(\sqrt{a} + \sqrt{b} = \sqrt{a+b}\). This is incorrect. The square root of a sum is not the sum of the square roots.
- Overlooking pairs of factors:
Each pair of identical factors under the square root can be simplified outside the radical. Missing this step can complicate the expression unnecessarily.
- Applying incorrect operations on radicals:
Ensure that you're using the correct arithmetic operations. The rules for adding, subtracting, multiplying, and dividing radicals are specific and must be followed accurately.
By being mindful of these common mistakes and focusing on correct simplification procedures, you can improve your proficiency in working with square roots and ensure your mathematical expressions are simplified accurately.
Practice Problems
Below are a variety of practice problems to help you master the simplification of square roots. Work through each problem step by step and verify your answers using a calculator if needed.
Problem Set 1: Basic Simplification
- Simplify \( \sqrt{16} \).
- Solution: \( \sqrt{16} = 4 \)
- Simplify \( \sqrt{25} \).
- Solution: \( \sqrt{25} = 5 \)
- Simplify \( \sqrt{36} \).
- Solution: \( \sqrt{36} = 6 \)
Problem Set 2: Simplifying Non-Perfect Squares
- Simplify \( \sqrt{18} \).
- Solution: \( \sqrt{18} = \sqrt{9 \times 2} = \sqrt{9} \times \sqrt{2} = 3\sqrt{2} \)
- Simplify \( \sqrt{50} \).
- Solution: \( \sqrt{50} = \sqrt{25 \times 2} = \sqrt{25} \times \sqrt{2} = 5\sqrt{2} \)
- Simplify \( \sqrt{72} \).
- Solution: \( \sqrt{72} = \sqrt{36 \times 2} = \sqrt{36} \times \sqrt{2} = 6\sqrt{2} \)
Problem Set 3: Advanced Simplification
- Simplify \( \sqrt{200} \).
- Solution: \( \sqrt{200} = \sqrt{100 \times 2} = \sqrt{100} \times \sqrt{2} = 10\sqrt{2} \)
- Simplify \( \sqrt{45} \).
- Solution: \( \sqrt{45} = \sqrt{9 \times 5} = \sqrt{9} \times \sqrt{5} = 3\sqrt{5} \)
- Simplify \( \sqrt{98} \).
- Solution: \( \sqrt{98} = \sqrt{49 \times 2} = \sqrt{49} \times \sqrt{2} = 7\sqrt{2} \)
Problem Set 4: Simplifying Fractions
- Simplify \( \frac{\sqrt{50}}{\sqrt{2}} \).
- Solution: \( \frac{\sqrt{50}}{\sqrt{2}} = \sqrt{\frac{50}{2}} = \sqrt{25} = 5 \)
- Simplify \( \frac{\sqrt{18}}{\sqrt{2}} \).
- Solution: \( \frac{\sqrt{18}}{\sqrt{2}} = \sqrt{\frac{18}{2}} = \sqrt{9} = 3 \)
- Simplify \( \frac{\sqrt{72}}{\sqrt{8}} \).
- Solution: \( \frac{\sqrt{72}}{\sqrt{8}} = \sqrt{\frac{72}{8}} = \sqrt{9} = 3 \)
Problem Set 5: Mixed Problems
- Simplify \( 3\sqrt{12} + 2\sqrt{27} \).
- Solution: \( 3\sqrt{12} + 2\sqrt{27} = 3 \times 2\sqrt{3} + 2 \times 3\sqrt{3} = 6\sqrt{3} + 6\sqrt{3} = 12\sqrt{3} \)
- Simplify \( \sqrt{18} \times \sqrt{8} \).
- Solution: \( \sqrt{18} \times \sqrt{8} = \sqrt{18 \times 8} = \sqrt{144} = 12 \)
- Simplify \( \frac{\sqrt{50} \times \sqrt{8}}{\sqrt{2}} \).
- Solution: \( \frac{\sqrt{50} \times \sqrt{8}}{\sqrt{2}} = \frac{\sqrt{400}}{\sqrt{2}} = \frac{20}{\sqrt{2}} = 10\sqrt{2} \)
Practice these problems to enhance your understanding and proficiency in simplifying square roots. Remember, the key to mastering this skill is practice and consistency.
Applications in Real Life
Square roots play a crucial role in various real-life applications across multiple fields. Here are some of the key areas where square roots are utilized:
- Finance: Square roots are used to calculate stock market volatility, which is essential for assessing investment risks. The volatility is determined by taking the square root of the variance in stock returns. Additionally, the rate of return on investments over multiple periods can be computed using square roots.
- Architecture and Engineering: In engineering, square roots help determine the natural frequencies of structures like bridges and buildings, which is important for predicting how they will react to different loads. The Pythagorean theorem, which involves square roots, is frequently used to ensure structural integrity.
- Science: Square roots are used in various scientific calculations, including determining the velocity of moving objects, radiation absorption, and sound wave intensity. These calculations help scientists develop new technologies and deepen their understanding of natural phenomena.
- Statistics: In statistical analysis, the standard deviation is a key measure that involves taking the square root of the variance. This measure helps in understanding the spread and dispersion of data points in a dataset.
- Computer Science: Square roots are employed in algorithms for encryption, image processing, and game physics. For example, encryption algorithms use square roots in key generation processes to secure data transmission.
- Navigation: Pilots and navigators use square roots to calculate distances between points on maps or globes. This is crucial for plotting accurate courses and ensuring safe travel.
- Electrical Engineering: Calculations involving power, voltage, and current in electrical circuits often require the use of square roots. These computations are fundamental to designing and developing efficient electrical systems.
- Biology: Biologists use square roots to compare the surface area of animals, which is important for understanding their physiology and behavior. This helps in scientific research and ecological studies.
- Photography: The aperture of a camera lens is controlled using the f-number, which is related to the square root of the aperture area. Adjusting the f-number helps photographers manage the amount of light entering the camera for optimal exposure.

Additional Resources and References
To further your understanding and proficiency in simplifying square roots to their simplest form, here are some valuable resources and references:
-
Khan Academy: Offers a comprehensive set of video tutorials and practice exercises on square roots and their simplification.
-
Wikipedia: Provides a detailed explanation of square roots, including their properties, history, and methods for simplification.
-
Math is Fun: Offers easy-to-understand tutorials on simplifying square roots, along with interactive practice problems.
-
Paul's Online Math Notes: Contains detailed notes and examples on simplifying square roots using different methods, including prime factorization.
-
Purplemath: Provides step-by-step guides on simplifying square roots and includes helpful tips to avoid common mistakes.
-
Interactive Mathematics: Features articles and examples on various mathematical concepts, including square root simplification.
These resources will provide you with a broad range of learning materials, from basic introductions to more advanced topics. Utilizing these will help you master the simplification of square roots and enhance your overall mathematical skills.
Đơn Giản Hóa Căn Bậc Hai | Toán Học với Thầy J