Topic square root 89 simplified: The square root of 89 simplified may seem complex, but this guide will break it down for you. Learn why 89 is a prime number, how to express its square root in simplest form, and discover practical applications of this mathematical concept. Simplifying square roots has never been easier!
Table of Content
- Simplified Square Root of 89
- Introduction to Square Roots
- Understanding the Square Root of 89
- Prime Factorization of 89
- Why 89 is a Prime Number
- Methods to Simplify Square Roots
- Simplifying Square Roots of Prime Numbers
- Exact Value of Square Root of 89
- Approximate Decimal Value of Square Root of 89
- Comparison with Other Square Roots
- Applications of Square Root of 89
- Frequently Asked Questions
- Conclusion
- YOUTUBE: Video hướng dẫn cách đơn giản hóa các biểu thức căn bậc hai bằng máy tính TI-89, phù hợp cho những ai muốn hiểu rõ hơn về căn bậc hai và cách sử dụng máy tính TI-89.
Simplified Square Root of 89
To find the simplified form of the square root of 89, we need to determine if 89 can be broken down into any perfect square factors. Since 89 is a prime number, it does not have any factors other than 1 and 89 itself.
Therefore, the square root of 89 cannot be simplified further and remains in its radical form:
\[
\sqrt{89}
\]
For practical purposes, the approximate decimal value of \(\sqrt{89}\) is:
\[
\sqrt{89} \approx 9.434
\]
Thus, the simplest radical form of the square root of 89 is \(\sqrt{89}\).
READ MORE:
Introduction to Square Roots
Square roots are a fundamental concept in mathematics, representing a value that, when multiplied by itself, gives the original number. The square root is denoted by the radical symbol \(\sqrt{}\). For example, the square root of 9 is 3, because \(3 \times 3 = 9\).
Key points about square roots:
- The square root of a number \(x\) is written as \(\sqrt{x}\).
- Square roots can be positive or negative, but by convention, we refer to the principal (positive) square root.
- Perfect squares, such as 1, 4, 9, 16, and 25, have whole number square roots.
- Non-perfect squares, such as 2, 3, 5, and 89, result in irrational numbers when square rooted.
Calculating the square root of a number can be done using various methods:
- Prime Factorization: Breaking down the number into its prime factors can help simplify square roots, especially for perfect squares.
- Long Division Method: A manual method for finding the square root of a number, useful for non-perfect squares.
- Approximation: Using a calculator or numerical methods to find the approximate value of a square root.
In the case of prime numbers like 89, the square root does not simplify into a smaller radical form. Thus, the square root of 89 remains as \(\sqrt{89}\).
Understanding square roots is crucial in various fields, including algebra, geometry, and applied sciences, as it lays the foundation for solving equations, analyzing shapes, and interpreting scientific data.
Understanding the Square Root of 89
The square root of 89, denoted as \(\sqrt{89}\), is an irrational number. This means it cannot be expressed as a simple fraction and its decimal representation is non-terminating and non-repeating.
Here is a step-by-step approach to understanding and simplifying the square root of 89:
-
Prime Factorization:
Since 89 is a prime number, its only factors are 1 and 89. This means it cannot be broken down further into smaller factors that are perfect squares.
-
Simplification:
Because 89 is a prime number, \(\sqrt{89}\) cannot be simplified further. It remains in its simplest radical form:
\[
\sqrt{89}
\] -
Approximation:
To use \(\sqrt{89}\) in practical applications, it is often approximated as a decimal. The approximate value is:
\[
\sqrt{89} \approx 9.434
\]
To summarize, the square root of 89 cannot be simplified because it is a prime number. Its exact value remains as \(\sqrt{89}\), and its approximate decimal value is 9.434. This understanding is crucial for various mathematical applications, including solving equations and performing calculations that involve square roots.
Prime Factorization of 89
Prime factorization is the process of breaking down a number into its smallest prime factors. To understand the prime factorization of 89, we need to determine whether it can be divided by any prime numbers other than 1 and itself.
-
Identifying Primes:
First, we list the prime numbers less than 89: 2, 3, 5, 7, 11, 13, 17, 19, 23, 29, 31, 37, 41, 43, 47, 53, 59, 61, 67, 71, 73, 79, 83.
-
Testing Divisibility:
We test each prime number to see if it divides 89 without leaving a remainder:
- 89 is not divisible by 2 (since 89 is odd).
- 89 is not divisible by 3 (sum of digits, 8 + 9 = 17, which is not divisible by 3).
- 89 is not divisible by 5 (since it does not end in 0 or 5).
- 89 is not divisible by 7 (89 ÷ 7 ≈ 12.714).
- Continuing this process for all primes up to the square root of 89 (approximately 9.434) shows that 89 is not divisible by any of these primes.
-
Conclusion:
Since 89 is not divisible by any prime numbers other than itself, we conclude that 89 is a prime number. Its prime factorization is simply:
\[
89 = 89 \times 1
\]
In summary, 89 is a prime number and its prime factorization is trivial. This understanding helps us in simplifying expressions involving \(\sqrt{89}\), confirming that it cannot be simplified further due to the lack of smaller prime factors.
Why 89 is a Prime Number
A prime number is a natural number greater than 1 that has no positive divisors other than 1 and itself. To determine why 89 is a prime number, we need to check if it can be divided evenly by any numbers other than 1 and 89.
Here is a step-by-step explanation:
-
Definition of Prime Numbers:
A prime number is only divisible by 1 and itself. For example, 2, 3, 5, and 7 are prime numbers because they do not have divisors other than 1 and themselves.
-
Checking for Factors:
To determine if 89 is a prime number, we test its divisibility by all prime numbers less than or equal to the square root of 89 (approximately 9.434). These primes are 2, 3, 5, and 7.
- Divisibility by 2: 89 is odd, so it is not divisible by 2.
- Divisibility by 3: The sum of the digits of 89 is 8 + 9 = 17. Since 17 is not divisible by 3, neither is 89.
- Divisibility by 5: Numbers ending in 0 or 5 are divisible by 5. Since 89 ends in neither, it is not divisible by 5.
- Divisibility by 7: Dividing 89 by 7 gives approximately 12.714, which is not an integer. Therefore, 89 is not divisible by 7.
-
Conclusion:
Since 89 is not divisible by any prime numbers up to its square root, it has no divisors other than 1 and itself. Thus, 89 is confirmed to be a prime number.
\[
89 = 1 \times 89
\]
In summary, 89 is a prime number because it cannot be divided evenly by any other numbers except for 1 and 89. Understanding the primality of 89 is essential for simplifying expressions involving \(\sqrt{89}\) and for various mathematical applications.

Methods to Simplify Square Roots
Simplifying square roots involves expressing the root in its simplest radical form. Here are the detailed methods to simplify square roots:
-
Prime Factorization:
Break down the number under the square root into its prime factors and pair the prime factors.
- Example: Simplify \(\sqrt{72}\).
- Prime factorization of 72 is \(2 \times 2 \times 2 \times 3 \times 3\).
- Pair the prime factors: \( (2 \times 2) \) and \( (3 \times 3) \) and \(2\) remains unpaired.
- Thus, \(\sqrt{72} = \sqrt{(2 \times 2) \times (3 \times 3) \times 2} = 2 \times 3 \times \sqrt{2} = 6\sqrt{2}\).
-
Simplification Using Perfect Squares:
Identify and extract the largest perfect square factor of the number under the square root.
- Example: Simplify \(\sqrt{50}\).
- The largest perfect square factor of 50 is 25.
- Thus, \(\sqrt{50} = \sqrt{25 \times 2} = \sqrt{25} \times \sqrt{2} = 5\sqrt{2}\).
-
Rationalizing the Denominator:
When a square root appears in the denominator, multiply the numerator and the denominator by the same square root to rationalize.
- Example: Simplify \(\frac{1}{\sqrt{3}}\).
- Multiply by \(\sqrt{3}/\sqrt{3}\): \(\frac{1}{\sqrt{3}} \times \frac{\sqrt{3}}{\sqrt{3}} = \frac{\sqrt{3}}{3}\).
-
Using Algebraic Identities:
Utilize algebraic identities to simplify square roots involving sums or differences.
- Example: Simplify \(\sqrt{a^2 + 2ab + b^2}\).
- Recognize the identity: \((a + b)^2 = a^2 + 2ab + b^2\).
- Thus, \(\sqrt{a^2 + 2ab + b^2} = \sqrt{(a + b)^2} = |a + b|\).
In summary, simplifying square roots can be done through various methods including prime factorization, using perfect squares, rationalizing the denominator, and applying algebraic identities. Each method provides a systematic approach to expressing square roots in their simplest form.
Simplifying Square Roots of Prime Numbers
Simplifying the square roots of prime numbers follows a straightforward process since prime numbers have no divisors other than 1 and themselves. Here’s a step-by-step guide:
-
Understanding Prime Numbers:
A prime number is a number greater than 1 that has no positive divisors other than 1 and itself. Examples include 2, 3, 5, 7, 11, and 89.
-
Prime Factorization:
For prime numbers, the only prime factor is the number itself. For example, the prime factorization of 89 is:
\[
89 = 89 \times 1
\] -
Simplification Process:
Since prime numbers do not have any perfect square factors other than 1, the square root of a prime number cannot be simplified further. It remains in its radical form.
- Example: \(\sqrt{89}\) cannot be simplified because 89 is a prime number.
- Another example: \(\sqrt{7}\) remains \(\sqrt{7}\) because 7 is a prime number.
-
Approximation:
In practical applications, the square root of a prime number is often approximated to a decimal value for ease of use. For example:
\[
\sqrt{89} \approx 9.434
\]This approximation helps in calculations where an exact radical form may be inconvenient.
In summary, simplifying the square roots of prime numbers involves recognizing that these roots cannot be simplified further and understanding how to approximate them for practical purposes. The prime number's unique properties ensure that its square root remains in the simplest form, providing clarity and consistency in mathematical expressions.
Exact Value of Square Root of 89
The square root of 89 is represented as √89 in radical form. Since 89 is a prime number, it does not have any factors other than 1 and 89. Therefore, the square root of 89 cannot be simplified further in its radical form.
In terms of exact value:
- The exact value of the square root of 89 is √89.
- This is an irrational number, meaning it cannot be expressed as a simple fraction.
For a more detailed mathematical representation using MathJax:
\(\sqrt{89}\)
Since the square root of 89 is irrational, its exact value is often left in the radical form for precision:
- Radical Form: \(\sqrt{89}\)
- Decimal Form: Approximately 9.433981132056603
The decimal form is derived through numerical methods or using a calculator for practical applications.
Here is a table summarizing the forms of the square root of 89:
| Form | Representation |
|---|---|
| Radical Form | \(\sqrt{89}\) |
| Decimal Form | Approximately 9.433981132056603 |
In summary, the exact value of the square root of 89 is best expressed as \(\sqrt{89}\), maintaining the precision required for mathematical accuracy.
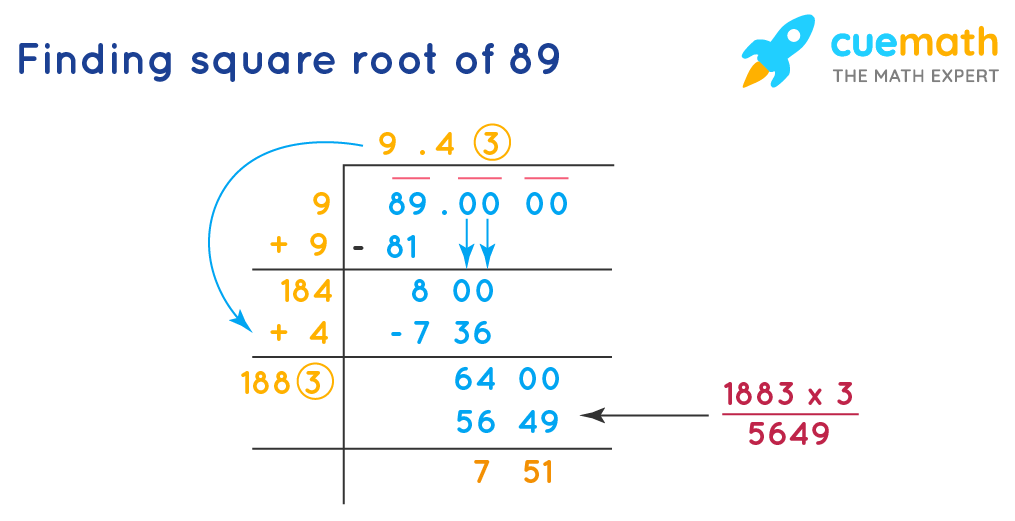
Approximate Decimal Value of Square Root of 89
The square root of 89 is an irrational number, meaning it cannot be expressed as a simple fraction and its decimal representation is non-repeating and non-terminating. To understand the approximate decimal value of the square root of 89, we use numerical methods or a calculator.
Using a calculator, we find that:
\(\sqrt{89} \approx 9.433981132056603\)
Here is a step-by-step method to find the approximate decimal value:
- Estimate: Find two perfect squares between which 89 lies. Since \(81 = 9^2\) and \(100 = 10^2\), we know that \(9 < \sqrt{89} < 10\).
- Refine: Use a more precise method such as the long division method or a calculator to get a more accurate decimal value.
For practical purposes, the square root of 89 is often rounded to a certain number of decimal places. Common approximations include:
- Rounded to 2 decimal places: \(\sqrt{89} \approx 9.43\)
- Rounded to 3 decimal places: \(\sqrt{89} \approx 9.434\)
- Rounded to 4 decimal places: \(\sqrt{89} \approx 9.4340\)
Here is a table summarizing the approximate decimal values of \(\sqrt{89}\) at different levels of precision:
| Decimal Places | Approximate Value |
|---|---|
| 2 | 9.43 |
| 3 | 9.434 |
| 4 | 9.4340 |
| Full Precision | 9.433981132056603 |
Therefore, the approximate decimal value of the square root of 89, when rounded to different decimal places, helps in practical calculations and applications.
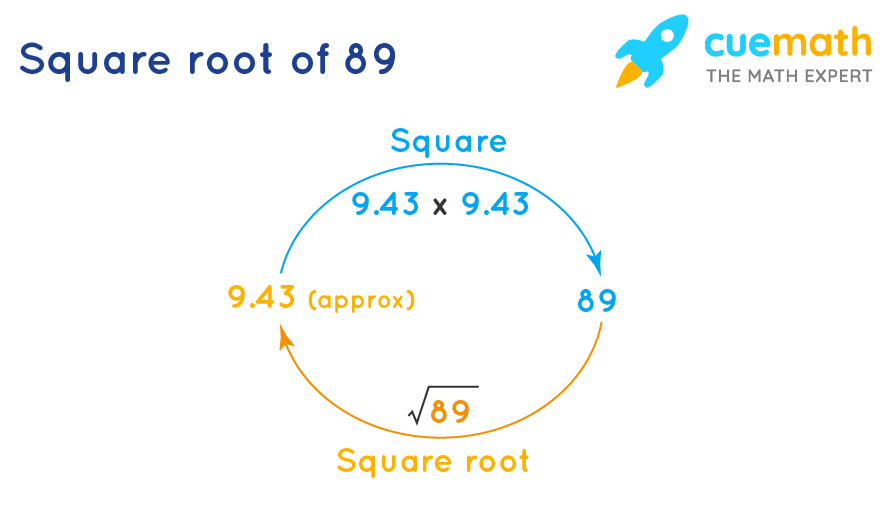
Comparison with Other Square Roots
To better understand the square root of 89, it is helpful to compare it with the square roots of other numbers. This comparison will highlight the relative magnitude and properties of \(\sqrt{89}\).
Let's compare \(\sqrt{89}\) with the square roots of both nearby perfect squares and other selected numbers:
- \(\sqrt{81} = 9\)
- \(\sqrt{100} = 10\)
- \(\sqrt{88} \approx 9.38083151964686\)
- \(\sqrt{90} \approx 9.48683298050514\)
By looking at these values, we can see that the square root of 89 falls between 9 and 10, and is closer to the square root of 88 than to the square root of 90.
For a more detailed comparison, consider the following table:
| Number | Square Root | Approximate Decimal Value |
|---|---|---|
| 81 | \(\sqrt{81}\) | 9 |
| 88 | \(\sqrt{88}\) | 9.3808 |
| 89 | \(\sqrt{89}\) | 9.433981132056603 |
| 90 | \(\sqrt{90}\) | 9.4868 |
| 100 | \(\sqrt{100}\) | 10 |
Additionally, comparing the square root of 89 with the square roots of prime numbers can provide further insight:
- \(\sqrt{2} \approx 1.414\)
- \(\sqrt{3} \approx 1.732\)
- \(\sqrt{5} \approx 2.236\)
- \(\sqrt{7} \approx 2.646\)
- \(\sqrt{11} \approx 3.317\)
- \(\sqrt{13} \approx 3.606\)
- \(\sqrt{17} \approx 4.123\)
- \(\sqrt{19} \approx 4.359\)
- \(\sqrt{23} \approx 4.796\)
- \(\sqrt{29} \approx 5.385\)
- \(\sqrt{31} \approx 5.568\)
- \(\sqrt{37} \approx 6.083\)
- \(\sqrt{41} \approx 6.403\)
- \(\sqrt{43} \approx 6.557\)
- \(\sqrt{47} \approx 6.856\)
- \(\sqrt{53} \approx 7.280\)
- \(\sqrt{59} \approx 7.681\)
- \(\sqrt{61} \approx 7.810\)
- \(\sqrt{67} \approx 8.191\)
- \(\sqrt{71} \approx 8.426\)
- \(\sqrt{73} \approx 8.544\)
- \(\sqrt{79} \approx 8.888\)
- \(\sqrt{83} \approx 9.110\)
- \(\sqrt{89} \approx 9.434
This comparison shows how the square root of 89 fits within the context of other prime numbers. The value of \(\sqrt{89}\) is relatively higher compared to smaller prime numbers but is consistent with the trend as numbers increase.
Overall, these comparisons help illustrate where \(\sqrt{89}\) stands among other square roots, both of perfect squares and prime numbers.
Applications of Square Root of 89
The square root of 89, represented as \(\sqrt{89}\), finds its applications in various fields of science, mathematics, engineering, and real-world problem-solving scenarios. Below are some key areas where the square root of 89 is applied:
- Mathematics and Geometry:
- In geometry, \(\sqrt{89}\) can be used to determine the length of the diagonal of a rectangle with side lengths that are related by specific ratios.
- It is also useful in solving equations involving quadratic terms where the square root of a number like 89 appears as part of the solution.
- Engineering and Physics:
- In engineering, the square root of 89 may appear in calculations involving distances, forces, and areas where precise measurements are required.
- In physics, it could be part of formulas calculating energy levels, wave functions, or other phenomena where irrational numbers provide accurate representations.
- Computer Science:
- Algorithms that involve optimization or numerical methods may use \(\sqrt{89}\) for precise calculations.
- It can also be relevant in computational geometry for calculating distances or other spatial properties.
- Architecture and Design:
- Architects may use \(\sqrt{89}\) in the design of structures to ensure accurate dimensions and proportions.
- It helps in creating aesthetically pleasing and structurally sound designs that require precise measurements.
- Everyday Problem Solving:
- In various practical scenarios, such as construction, crafting, or navigation, the square root of 89 can be used to calculate distances and dimensions accurately.
- It aids in creating objects or spaces with specific measurements that fit exact requirements.
Here is a table summarizing some of the applications of \(\sqrt{89}\):
| Field | Application |
|---|---|
| Mathematics | Solving quadratic equations, geometric calculations |
| Engineering | Distance and force calculations |
| Physics | Energy levels, wave functions |
| Computer Science | Optimization algorithms, computational geometry |
| Architecture | Design dimensions, structural calculations |
| Everyday Use | Construction, crafting, navigation |
In conclusion, the square root of 89, while an irrational number, has numerous practical applications that span across various disciplines, highlighting its importance in both theoretical and applied contexts.
Frequently Asked Questions
Below are some frequently asked questions about the square root of 89:
- What is the square root of 89?
The square root of 89 is an irrational number, represented as \(\sqrt{89}\). Its approximate decimal value is 9.433981132056603.
- Can the square root of 89 be simplified?
No, the square root of 89 cannot be simplified further because 89 is a prime number. It does not have any factors other than 1 and 89.
- Is the square root of 89 a rational or irrational number?
The square root of 89 is an irrational number because it cannot be expressed as a simple fraction and its decimal representation is non-repeating and non-terminating.
- How is the square root of 89 used in real-world applications?
The square root of 89 is used in various fields such as mathematics, engineering, physics, computer science, architecture, and everyday problem-solving. It helps in precise calculations involving distances, areas, energy levels, and design dimensions.
- How can I calculate the square root of 89?
You can calculate the square root of 89 using a calculator or numerical methods. For a quick approximation, you can use the fact that \(\sqrt{89}\) is between 9 and 10, closer to 9.434.
- What are some related concepts to the square root of 89?
Related concepts include prime numbers, irrational numbers, quadratic equations, and geometric calculations. Understanding these concepts can help in grasping the significance of \(\sqrt{89}\).
For further questions, feel free to reach out or explore additional resources to deepen your understanding of the square root of 89.
Conclusion
In conclusion, the square root of 89, denoted as \(\sqrt{89}\), is a unique and interesting number with several important properties and applications. Here are the key takeaways:
- Nature of \(\sqrt{89}\): The square root of 89 is an irrational number, meaning it cannot be expressed as a simple fraction and its decimal form is non-repeating and non-terminating. The exact value is \(\sqrt{89}\), and its approximate decimal value is 9.433981132056603.
- Simplification: Since 89 is a prime number, the square root of 89 cannot be simplified further in radical form.
- Comparative Analysis: By comparing \(\sqrt{89}\) with the square roots of other numbers, we can see that it falls between the square roots of 81 and 100, making it closer to 9 than to 10. It also aligns with the trend observed in the square roots of other prime numbers.
- Applications: The square root of 89 is utilized in various fields such as mathematics, engineering, physics, computer science, and architecture. It is essential for precise calculations in geometric measurements, quadratic equations, distance calculations, design dimensions, and more.
- Practical Use: Understanding and approximating \(\sqrt{89}\) helps in practical scenarios, including construction, navigation, and crafting, where accurate measurements are crucial.
The study of the square root of 89 offers a deeper insight into the properties of irrational numbers and their significance in both theoretical and applied contexts. By exploring its exact value, simplification methods, comparative analysis, and practical applications, we gain a comprehensive understanding of \(\sqrt{89}\) and its role in various disciplines.
We hope this guide has provided valuable information and clarity on the topic of the square root of 89. For further learning, continue to explore related mathematical concepts and their applications in real-world scenarios.

Video hướng dẫn cách đơn giản hóa các biểu thức căn bậc hai bằng máy tính TI-89, phù hợp cho những ai muốn hiểu rõ hơn về căn bậc hai và cách sử dụng máy tính TI-89.
Đơn giản hóa các biểu thức căn bậc hai với TI-89
READ MORE:
Video hướng dẫn cách đơn giản hóa căn bậc hai, giúp người xem nắm vững các bước đơn giản hóa căn bậc hai một cách dễ dàng và hiệu quả.
Cách Đơn Giản Hóa Căn Bậc Hai