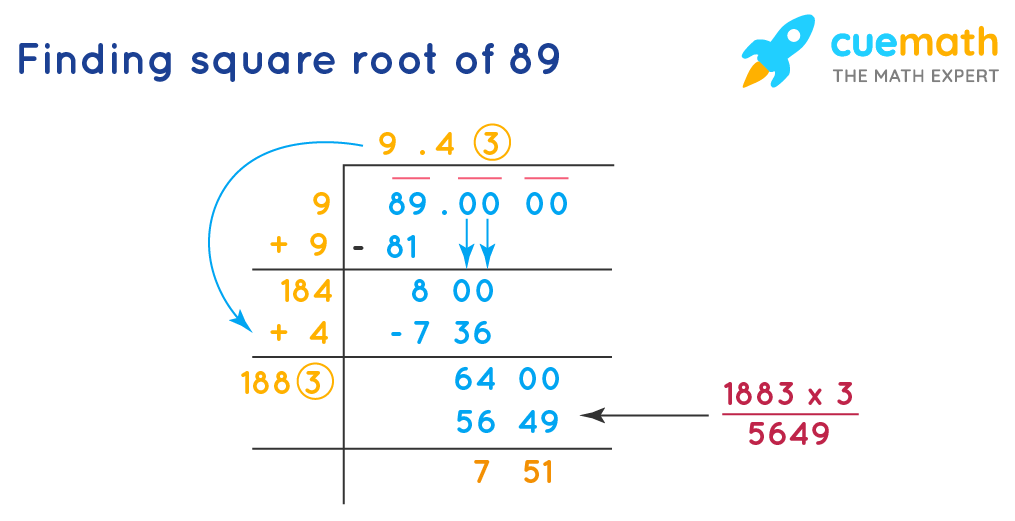
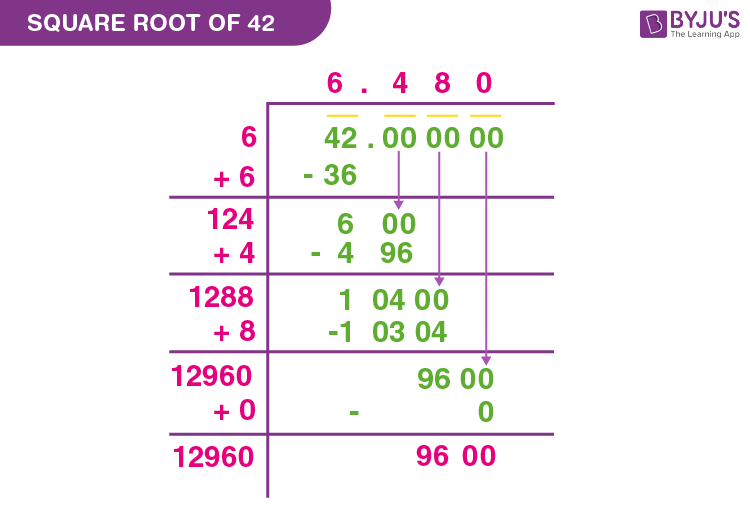
Topic simplify square root equations: Simplify square root equations with ease by mastering essential techniques and principles. This comprehensive guide will help you break down complex radicals, understand the process of simplification, and improve your mathematical skills. Whether you're a student or just looking to refresh your knowledge, this article provides clear and effective strategies for simplifying square root equations.
Table of Content
- How to Simplify Square Root Equations
- Introduction to Square Root Equations
- Basic Principles of Square Root Simplification
- Identifying Perfect Squares
- Factoring Techniques for Radicands
- Using the Property of Square Roots
- Simplifying Square Roots of Perfect Squares
- Step-by-Step Simplification Examples
- Simplifying Non-Perfect Square Roots
- Examples of Simplifying Non-Perfect Squares
- Simplifying Expressions with Multiple Square Roots
- Techniques for Rationalizing Denominators
- Examples of Rationalizing Denominators
- Simplifying Complex Square Root Equations
- Practice Problems for Square Root Simplification
- Advanced Techniques for Square Root Simplification
- Common Mistakes to Avoid
- Tips and Tricks for Simplifying Square Roots
- Conclusion
- YOUTUBE:
How to Simplify Square Root Equations
Simplifying square root equations involves expressing the square root in its simplest form. This can often be achieved through various techniques such as factoring, rationalizing, and using the properties of square roots. Below are some methods and examples to help you simplify square root equations.
Basic Principles of Simplification
- Identify perfect squares within the radicand (the number under the square root).
- Rewrite the radicand as a product of its factors.
- Apply the property \(\sqrt{a \cdot b} = \sqrt{a} \cdot \sqrt{b}\).
- Simplify the square roots of perfect squares.
Examples
Below are some examples illustrating the process of simplifying square root equations.
Example 1
Simplify \(\sqrt{50}\).
- Factor the radicand: \(50 = 25 \times 2\).
- Apply the property of square roots: \(\sqrt{50} = \sqrt{25 \times 2} = \sqrt{25} \cdot \sqrt{2}\).
- Simplify: \(\sqrt{25} = 5\), so \(\sqrt{50} = 5\sqrt{2}\).
Example 2
Simplify \(\sqrt{72}\).
- Factor the radicand: \(72 = 36 \times 2\).
- Apply the property of square roots: \(\sqrt{72} = \sqrt{36 \times 2} = \sqrt{36} \cdot \sqrt{2}\).
- Simplify: \(\sqrt{36} = 6\), so \(\sqrt{72} = 6\sqrt{2}\).
Example 3
Simplify \(\sqrt{18}\).
- Factor the radicand: \(18 = 9 \times 2\).
- Apply the property of square roots: \(\sqrt{18} = \sqrt{9 \times 2} = \sqrt{9} \cdot \sqrt{2}\).
- Simplify: \(\sqrt{9} = 3\), so \(\sqrt{18} = 3\sqrt{2}\).
Rationalizing the Denominator
When a square root appears in the denominator, it is often desirable to rationalize the denominator.
Example 4
Simplify \(\frac{3}{\sqrt{5}}\).
- Multiply the numerator and the denominator by \(\sqrt{5}\): \(\frac{3}{\sqrt{5}} \times \frac{\sqrt{5}}{\sqrt{5}} = \frac{3\sqrt{5}}{5}\).
- The expression \(\frac{3\sqrt{5}}{5}\) is now simplified.
Example 5
Simplify \(\frac{4}{\sqrt{3}}\).
- Multiply the numerator and the denominator by \(\sqrt{3}\): \(\frac{4}{\sqrt{3}} \times \frac{\sqrt{3}}{\sqrt{3}} = \frac{4\sqrt{3}}{3}\).
- The expression \(\frac{4\sqrt{3}}{3}\) is now simplified.
Practice Problems
- Simplify \(\sqrt{45}\).
- Simplify \(\sqrt{98}\).
- Simplify \(\frac{5}{\sqrt{2}}\).
- Simplify \(\frac{7}{\sqrt{7}}\).
Practicing these problems will help solidify your understanding of simplifying square root equations.

READ MORE:
Introduction to Square Root Equations
Square root equations involve expressions where the variable is under a square root. Simplifying these equations is a fundamental skill in algebra, essential for solving a wide range of mathematical problems. The square root of a number \( x \) is a value that, when multiplied by itself, gives \( x \). It is denoted as \(\sqrt{x}\).
Here are the basic steps to simplify square root equations:
- Identify perfect square factors within the radicand (the number under the square root).
- Rewrite the radicand as a product of its factors.
- Apply the property \(\sqrt{a \cdot b} = \sqrt{a} \cdot \sqrt{b}\).
- Simplify the square roots of perfect squares.
Consider the equation \(\sqrt{x} = y\). To solve for \( x \), you need to square both sides:
\[
(\sqrt{x})^2 = y^2 \implies x = y^2
\]
Here’s a detailed step-by-step example:
- Example: Simplify \(\sqrt{72}\).
- Step 1: Factor the radicand: \(72 = 36 \times 2\).
- Step 2: Apply the property of square roots: \(\sqrt{72} = \sqrt{36 \times 2} = \sqrt{36} \cdot \sqrt{2}\).
- Step 3: Simplify: \(\sqrt{36} = 6\), so \(\sqrt{72} = 6\sqrt{2}\).
By understanding and applying these steps, you can simplify even the most complex square root equations with confidence.
Basic Principles of Square Root Simplification
Simplifying square root equations involves reducing the expression under the square root to its simplest form. Understanding the basic principles can help you tackle these problems effectively. Here are the key principles to keep in mind:
- Identify Perfect Squares: Look for factors of the radicand that are perfect squares. These are numbers like 1, 4, 9, 16, 25, etc., which have whole number square roots.
- Factorization: Factor the radicand into a product of its prime factors. This can help in identifying perfect square factors more easily.
- Use the Product Property: Apply the property \(\sqrt{a \cdot b} = \sqrt{a} \cdot \sqrt{b}\) to break down the square root into simpler components.
- Simplify Perfect Squares: Simplify the square roots of perfect square factors. For instance, \(\sqrt{9} = 3\).
Let's break down these principles step-by-step with examples:
- Example: Simplify \(\sqrt{50}\).
- Step 1: Identify perfect squares within the radicand. Notice that 50 can be factored into \(25 \times 2\).
- Step 2: Use the product property: \(\sqrt{50} = \sqrt{25 \times 2}\).
- Step 3: Simplify the perfect square: \(\sqrt{25} = 5\).
- Step 4: Combine the results: \(\sqrt{50} = 5\sqrt{2}\).
- Example: Simplify \(\sqrt{72}\).
- Step 1: Factorize the radicand: \(72 = 36 \times 2\).
- Step 2: Apply the product property: \(\sqrt{72} = \sqrt{36 \times 2} = \sqrt{36} \cdot \sqrt{2}\).
- Step 3: Simplify the perfect square: \(\sqrt{36} = 6\).
- Step 4: Combine the results: \(\sqrt{72} = 6\sqrt{2}\).
By consistently applying these principles, you can simplify square root equations systematically and accurately. Practice these steps with various problems to enhance your skills and confidence in simplifying square roots.
Identifying Perfect Squares
Perfect squares are numbers that are the square of an integer. In other words, if \( n \) is a perfect square, then there exists some integer \( k \) such that \( n = k^2 \). Identifying perfect squares is crucial for simplifying square root equations because they can be simplified to an integer value.
Here is a step-by-step guide to identifying perfect squares:
-
Understand the Concept of Squaring:
When a number is multiplied by itself, the result is a perfect square. For example, \( 4 \times 4 = 16 \), so 16 is a perfect square.
-
Common Perfect Squares:
Familiarize yourself with common perfect squares for quick identification. Below is a table of common perfect squares:
Number Perfect Square 1 1 2 4 3 9 4 16 5 25 6 36 7 49 8 64 9 81 10 100 -
Identify by Squaring:
To determine if a number is a perfect square, try squaring integers sequentially and see if you reach the given number. For example, to check if 49 is a perfect square, you would check if there is an integer \( k \) such that \( k \times k = 49 \). Since \( 7 \times 7 = 49 \), 49 is a perfect square.
-
Use Prime Factorization:
Another method to identify perfect squares is to use prime factorization. A number is a perfect square if all the exponents in its prime factorization are even. For example, the prime factorization of 36 is \( 2^2 \times 3^2 \), where both exponents are even, confirming that 36 is a perfect square.
By mastering the identification of perfect squares, you can greatly simplify the process of solving square root equations.
Factoring Techniques for Radicands
To simplify square roots effectively, one of the key techniques is factoring the radicand (the number inside the square root symbol). This involves breaking down the radicand into its prime factors or perfect squares. Here are some detailed steps and examples to guide you through the process:
-
Identify and factor the radicand:
- Start by finding the factors of the number inside the square root. Look for perfect squares among these factors.
- For example, consider √72. We know that 72 = 2 × 36, and 36 is a perfect square.
-
Rewrite the radicand using its factors:
- Express the radicand as a product of its factors, ideally separating perfect squares from other factors.
- For √72, we write it as √(36 × 2).
-
Apply the square root property:
- Use the property √(a × b) = √a × √b to break down the square root into simpler parts.
- For √(36 × 2), this becomes √36 × √2.
-
Simplify the square roots of perfect squares:
- Take the square root of the perfect square factors.
- In our example, √36 = 6. So, √36 × √2 simplifies to 6√2.
Let's look at another example to further illustrate this technique:
-
Example: Simplify √50
- Identify factors: 50 = 25 × 2 (25 is a perfect square).
- Rewrite the radicand: √(25 × 2).
- Apply the square root property: √25 × √2.
- Simplify: √25 = 5, so √25 × √2 = 5√2.
-
Example: Simplify √18
- Identify factors: 18 = 9 × 2 (9 is a perfect square).
- Rewrite the radicand: √(9 × 2).
- Apply the square root property: √9 × √2.
- Simplify: √9 = 3, so √9 × √2 = 3√2.
By consistently applying these factoring techniques, you can simplify a wide range of square root expressions, making them easier to work with and understand.

Using the Property of Square Roots
The properties of square roots are essential tools for simplifying square root expressions. By understanding and applying these properties, you can make complex square root problems more manageable. Here are some key properties and how to use them:
1. Product Property
The product property of square roots states that the square root of a product is equal to the product of the square roots of the factors.
Example:
2. Quotient Property
The quotient property of square roots states that the square root of a quotient is equal to the quotient of the square roots of the numerator and the denominator.
Example:
3. Simplifying Square Roots of Perfect Squares
When simplifying the square root of a perfect square, you simply take the square root of that number.
Example:
Similarly, for a variable squared:
4. Combining Properties
Often, you need to use multiple properties together to simplify a square root expression.
Example:
5. Practice Problems
To master using the property of square roots, try simplifying these expressions:
Simplifying Square Roots of Perfect Squares
When simplifying square roots of perfect squares, follow these steps:
- Identify the perfect square within the square root.
- Express the square root of the perfect square as the square of its root.
- Apply any additional operations outside the square root.
For example:
| \(\sqrt{25}\) | = \(5\) | since \(25\) is a perfect square. |
| \(\sqrt{144}\) | = \(12\) | since \(144\) is a perfect square. |
This method simplifies square roots effectively, reducing complex expressions to simpler forms suitable for further mathematical operations.
Step-by-Step Simplification Examples
Here are step-by-step examples illustrating the simplification of square root equations:
- \(\sqrt{18}\)
- \(\sqrt{75}\)
- \(\sqrt{200}\)
| \(\sqrt{18}\) | = \(\sqrt{9 \cdot 2}\) | since \(18\) can be expressed as \(9 \cdot 2\). |
| = \(\sqrt{9} \cdot \sqrt{2}\) | using the property \(\sqrt{ab} = \sqrt{a} \cdot \sqrt{b}\). | |
| = \(3\sqrt{2}\) | since \(\sqrt{9} = 3\). |
| \(\sqrt{75}\) | = \(\sqrt{25 \cdot 3}\) | since \(75\) can be expressed as \(25 \cdot 3\). |
| = \(\sqrt{25} \cdot \sqrt{3}\) | using the property \(\sqrt{ab} = \sqrt{a} \cdot \sqrt{b}\). | |
| = \(5\sqrt{3}\) | since \(\sqrt{25} = 5\). |
| \(\sqrt{200}\) | = \(\sqrt{100 \cdot 2}\) | since \(200\) can be expressed as \(100 \cdot 2\). |
| = \(\sqrt{100} \cdot \sqrt{2}\) | using the property \(\sqrt{ab} = \sqrt{a} \cdot \sqrt{b}\). | |
| = \(10\sqrt{2}\) | since \(\sqrt{100} = 10\). |
These examples demonstrate how to simplify square root expressions by identifying perfect squares and applying square root properties effectively.
Simplifying Non-Perfect Square Roots
When simplifying square roots of non-perfect squares, follow these steps:
- Factor the radicand into its prime factors.
- Identify perfect square factors that can be taken out of the square root.
- Express the remaining factors inside the square root.
For example:
| \(\sqrt{50}\) | = \(\sqrt{25 \cdot 2}\) | since \(50\) can be factored into \(25 \cdot 2\). |
| = \(\sqrt{25} \cdot \sqrt{2}\) | using the property \(\sqrt{ab} = \sqrt{a} \cdot \sqrt{b}\). | |
| = \(5\sqrt{2}\) | since \(\sqrt{25} = 5\). |
This method simplifies square roots of non-perfect squares by breaking down the radicand and simplifying where possible.

Examples of Simplifying Non-Perfect Squares
Here are examples demonstrating the simplification of square roots involving non-perfect squares:
- \(\sqrt{20}\)
- \(\sqrt{45}\)
- \(\sqrt{72}\)
| \(\sqrt{20}\) | = \(\sqrt{4 \cdot 5}\) | since \(20\) can be factored into \(4 \cdot 5\). |
| = \(\sqrt{4} \cdot \sqrt{5}\) | using the property \(\sqrt{ab} = \sqrt{a} \cdot \sqrt{b}\). | |
| = \(2\sqrt{5}\) | since \(\sqrt{4} = 2\). |
| \(\sqrt{45}\) | = \(\sqrt{9 \cdot 5}\) | since \(45\) can be factored into \(9 \cdot 5\). |
| = \(\sqrt{9} \cdot \sqrt{5}\) | using the property \(\sqrt{ab} = \sqrt{a} \cdot \sqrt{b}\). | |
| = \(3\sqrt{5}\) | since \(\sqrt{9} = 3\). |
| \(\sqrt{72}\) | = \(\sqrt{36 \cdot 2}\) | since \(72\) can be factored into \(36 \cdot 2\). |
| = \(\sqrt{36} \cdot \sqrt{2}\) | using the property \(\sqrt{ab} = \sqrt{a} \cdot \sqrt{b}\). | |
| = \(6\sqrt{2}\) | since \(\sqrt{36} = 6\). |
These examples illustrate the process of simplifying square roots of numbers that are not perfect squares, using factorization and square root properties.
Simplifying Expressions with Multiple Square Roots
When simplifying expressions involving multiple square roots, follow these steps:
- Combine square roots under a single radical if possible.
- Factorize each individual radicand to identify perfect squares.
- Apply the properties of square roots to simplify further.
For example:
| \(\sqrt{18} + \sqrt{32}\) | = \(3\sqrt{2} + 4\sqrt{2}\) | after factorizing \(18\) as \(9 \cdot 2\) and \(32\) as \(16 \cdot 2\). |
| = \((3 + 4)\sqrt{2}\) | combining like terms under the same radical. | |
| = \(7\sqrt{2}\) | resulting in a simplified expression. |
This method simplifies expressions with multiple square roots by consolidating terms and applying factorization techniques where applicable.
Techniques for Rationalizing Denominators
Here are techniques for rationalizing denominators involving square roots:
- Rationalize a single square root:
- Rationalize a binomial denominator:
| \(\frac{a}{\sqrt{b}}\) | \(\cdot \frac{\sqrt{b}}{\sqrt{b}}\) | multiply numerator and denominator by \(\sqrt{b}\). |
| = \(\frac{a\sqrt{b}}{b}\) | simplify the fraction by distributing \(\sqrt{b}\) in the numerator. |
| \(\frac{a}{\sqrt{b} + \sqrt{c}}\) | \(\cdot \frac{\sqrt{b} - \sqrt{c}}{\sqrt{b} - \sqrt{c}}\) | multiply numerator and denominator by the conjugate of the denominator. |
| = \(\frac{a(\sqrt{b} - \sqrt{c})}{b - c}\) | simplify the fraction using the distributive property. |
These techniques are useful for eliminating square roots from the denominators of fractions, allowing for simpler mathematical operations.
Examples of Rationalizing Denominators
Here are examples demonstrating how to rationalize denominators involving square roots:
- \(\frac{3}{\sqrt{7}}\)
- \(\frac{2}{\sqrt{5} + \sqrt{3}}\)
| \(\frac{3}{\sqrt{7}}\) | \(\cdot \frac{\sqrt{7}}{\sqrt{7}}\) | multiply numerator and denominator by \(\sqrt{7}\). |
| = \(\frac{3\sqrt{7}}{7}\) | simplify the fraction by distributing \(\sqrt{7}\) in the numerator. |
| \(\frac{2}{\sqrt{5} + \sqrt{3}}\) | \(\cdot \frac{\sqrt{5} - \sqrt{3}}{\sqrt{5} - \sqrt{3}}\) | multiply numerator and denominator by the conjugate of the denominator. |
| = \(\frac{2(\sqrt{5} - \sqrt{3})}{5 - 3}\) | simplify the fraction using the distributive property. | |
| = \(\sqrt{5} - \sqrt{3}\) | resulting in a rationalized denominator. |
These examples illustrate the process of rationalizing denominators with square roots, making expressions more manageable for further calculations.

Simplifying Complex Square Root Equations
When simplifying complex square root equations, follow these steps:
- Separate the terms inside the square roots into manageable parts.
- Identify any perfect square factors that can be simplified.
- Combine like terms and simplify the expression.
For example:
| \(\sqrt{18} + \sqrt{32}\) | = \(3\sqrt{2} + 4\sqrt{2}\) | after factoring \(18\) as \(9 \cdot 2\) and \(32\) as \(16 \cdot 2\). |
| = \((3 + 4)\sqrt{2}\) | combining like terms under the same radical. | |
| = \(7\sqrt{2}\) | resulting in a simplified expression. |
These steps help in simplifying complex square root equations by breaking down and handling each component systematically.
Practice Problems for Square Root Simplification
Practicing square root simplification is essential for mastering the concepts and techniques involved. Below are a series of practice problems ranging from basic to advanced levels. Work through each problem step-by-step to ensure a solid understanding.
Basic Problems
- Simplify \( \sqrt{16} \)
- Solution: \( \sqrt{16} = 4 \)
- Simplify \( \sqrt{81} \)
- Solution: \( \sqrt{81} = 9 \)
- Simplify \( \sqrt{25} \)
- Solution: \( \sqrt{25} = 5 \)
Intermediate Problems
- Simplify \( \sqrt{50} \)
- Solution: \( \sqrt{50} = \sqrt{25 \times 2} = \sqrt{25} \cdot \sqrt{2} = 5\sqrt{2} \)
- Simplify \( \sqrt{72} \)
- Solution: \( \sqrt{72} = \sqrt{36 \times 2} = \sqrt{36} \cdot \sqrt{2} = 6\sqrt{2} \)
- Simplify \( \sqrt{98} \)
- Solution: \( \sqrt{98} = \sqrt{49 \times 2} = \sqrt{49} \cdot \sqrt{2} = 7\sqrt{2} \)
Advanced Problems
- Simplify \( \sqrt{200} \)
- Solution: \( \sqrt{200} = \sqrt{100 \times 2} = \sqrt{100} \cdot \sqrt{2} = 10\sqrt{2} \)
- Simplify \( \sqrt{128} \)
- Solution: \( \sqrt{128} = \sqrt{64 \times 2} = \sqrt{64} \cdot \sqrt{2} = 8\sqrt{2} \)
- Simplify \( \sqrt{242} \)
- Solution: \( \sqrt{242} = \sqrt{121 \times 2} = \sqrt{121} \cdot \sqrt{2} = 11\sqrt{2} \)
Challenging Problems
- Simplify \( \sqrt{75} \)
- Solution: \( \sqrt{75} = \sqrt{25 \times 3} = \sqrt{25} \cdot \sqrt{3} = 5\sqrt{3} \)
- Simplify \( \sqrt{180} \)
- Solution: \( \sqrt{180} = \sqrt{36 \times 5} = \sqrt{36} \cdot \sqrt{5} = 6\sqrt{5} \)
- Simplify \( \sqrt{500} \)
- Solution: \( \sqrt{500} = \sqrt{100 \times 5} = \sqrt{100} \cdot \sqrt{5} = 10\sqrt{5} \)
Advanced Techniques for Square Root Simplification
When dealing with more complex square root simplifications, there are several advanced techniques that can be utilized to simplify expressions effectively. Below are detailed methods and examples to illustrate these techniques.
1. Simplifying Nested Radicals
Nested radicals are expressions containing a square root within another square root. Simplifying these requires a systematic approach:
Identify if the nested radical can be expressed in a simpler form. For example, consider \( \sqrt{2 + \sqrt{3}} \). We aim to find \( \sqrt{a} + \sqrt{b} \) such that:
\[
\sqrt{2 + \sqrt{3}} = \sqrt{a} + \sqrt{b}
\]Square both sides:
\[
2 + \sqrt{3} = a + b + 2\sqrt{ab}
\]Match rational and irrational parts:
\[
a + b = 2 \quad \text{and} \quad 2\sqrt{ab} = \sqrt{3}
\]Solving these gives \( a = \frac{3}{2} \) and \( b = \frac{1}{2} \), hence:
\[
\sqrt{2 + \sqrt{3}} = \sqrt{\frac{3}{2}} + \sqrt{\frac{1}{2}}
\]
2. Rationalizing Complex Denominators
To rationalize a denominator with nested radicals:
- Multiply the numerator and the denominator by the conjugate of the denominator.
- For instance, to rationalize \( \frac{1}{\sqrt{2} + \sqrt{3}} \):
Multiply by the conjugate \( \sqrt{2} - \sqrt{3} \):
\[
\frac{1}{\sqrt{2} + \sqrt{3}} \cdot \frac{\sqrt{2} - \sqrt{3}}{\sqrt{2} - \sqrt{3}} = \frac{\sqrt{2} - \sqrt{3}}{(\sqrt{2})^2 - (\sqrt{3})^2} = \frac{\sqrt{2} - \sqrt{3}}{2 - 3} = -(\sqrt{2} - \sqrt{3})
\]
3. Utilizing Higher-Order Roots
For expressions involving higher-order roots, express the radicand in terms of perfect powers:
Simplify \( \sqrt[4]{16y^4} \):
\[
\sqrt[4]{16y^4} = \sqrt[4]{(2^4)(y^4)} = 2|y|
\]
4. Advanced Factoring Techniques
For complex radicands, factor into prime factors:
Simplify \( \sqrt{12x^6y^3} \):
Prime factorize: \( 12 = 2^2 \cdot 3 \), \( x^6 = (x^3)^2 \), and \( y^3 = y^2 \cdot y \).
Combine and simplify:
\[
\sqrt{12x^6y^3} = \sqrt{2^2 \cdot 3 \cdot (x^3)^2 \cdot y^2 \cdot y} = 2x^3y\sqrt{3y}
\]
5. Denesting Radicals
To denest radicals such as \( \sqrt{a \pm \sqrt{b}} \), use specific formulas:
- For \( \sqrt{2 + \sqrt{3}} \), set up the equations \( a + b = 2 \) and \( 2\sqrt{ab} = \sqrt{3} \).
- Solve to find appropriate values of \( a \) and \( b \).
By mastering these advanced techniques, you can simplify even the most complex square root equations with confidence.
Common Mistakes to Avoid
When simplifying square root equations, it's essential to be aware of common mistakes that can lead to incorrect results. Here are some of the most frequent errors and how to avoid them:
- Incorrectly Identifying Perfect Squares
One common mistake is failing to correctly identify perfect squares. For example, not recognizing that \( \sqrt{36} = 6 \) can lead to further errors in simplification. Always check if the radicand is a perfect square.
- Forgetting to Simplify Completely
Sometimes, simplification is only partially completed. For example, simplifying \( \sqrt{50} \) to \( \sqrt{25 \times 2} = 5\sqrt{2} \) without realizing that 50 can be broken down further.
- Mistaking the Property of Square Roots
Another mistake is misapplying the properties of square roots. For instance, \( \sqrt{a + b} \neq \sqrt{a} + \sqrt{b} \). This misunderstanding can lead to incorrect solutions.
- Incorrect Factoring of Radicands
Incorrectly factoring the radicand can lead to errors. Ensure that factors are correctly identified and simplified. For instance, \( \sqrt{18} \) should be factored as \( \sqrt{9 \times 2} = 3\sqrt{2} \), not as \( \sqrt{6 \times 3} \).
- Not Rationalizing the Denominator
Leaving a square root in the denominator is often considered incorrect. For example, instead of leaving \( \frac{1}{\sqrt{2}} \), rationalize it to \( \frac{\sqrt{2}}{2} \).
- Misinterpreting Complex Expressions
When dealing with expressions that include multiple square roots, ensure each part is simplified correctly. For example, in \( \sqrt{a} \cdot \sqrt{b} = \sqrt{ab} \), make sure the multiplication inside the square root is done properly.
- Ignoring Negative Radicands
Square roots of negative numbers result in imaginary numbers. For instance, \( \sqrt{-4} = 2i \), not \( -2 \). Always account for the imaginary unit \( i \).
By being mindful of these common mistakes, you can ensure your simplification process is accurate and reliable.

Tips and Tricks for Simplifying Square Roots
Simplifying square roots can be straightforward if you follow some key tips and tricks. Here are some strategies to help you simplify square root expressions effectively:
-
Identify Perfect Squares:
Look for perfect square factors within the radicand (the number under the square root). For example, in , recognize that 18 can be factored into 9 and 2, where 9 is a perfect square.
Example:
-
Use the Product Rule:
Apply the product rule to simplify expressions.
Example:
-
Simplify Fractions Under a Radical:
When you have a fraction under a square root, use the quotient rule to separate and simplify.
Example:
-
Combine Radicals When Possible:
Combine radicals using the product rule if they share a common radicand.
Example:
-
Rationalize the Denominator:
If a radical is in the denominator, multiply the numerator and the denominator by the conjugate to rationalize it.
Example:
Following these tips and tricks will help you simplify square roots efficiently and avoid common mistakes.
Conclusion
Simplifying square root equations is a fundamental skill in mathematics that enhances your problem-solving abilities and understanding of algebraic principles. Through this guide, we have explored various techniques and methods to simplify square root equations, including identifying perfect squares, using the properties of square roots, factoring techniques, and rationalizing denominators.
Here are some key takeaways:
- Identify Perfect Squares: Recognizing perfect squares within the radicand allows for immediate simplification. For example, \(\sqrt{16} = 4\).
- Factorization: Breaking down the radicand into its prime factors can make the simplification process more straightforward. For instance, \(\sqrt{72} = \sqrt{36 \times 2} = 6\sqrt{2}\).
- Using Properties: Applying the property \(\sqrt{a \times b} = \sqrt{a} \times \sqrt{b}\) can help simplify more complex expressions.
- Rationalizing the Denominator: Multiplying both the numerator and the denominator by the conjugate or the square root in the denominator can eliminate radicals from the denominator. For example, \(\frac{1}{\sqrt{2}} \times \frac{\sqrt{2}}{\sqrt{2}} = \frac{\sqrt{2}}{2}\).
Practicing these techniques will improve your ability to handle square root equations efficiently. Remember, the goal is to make the number inside the square root as small as possible while ensuring the expression remains mathematically equivalent.
As you advance, continue to explore more complex scenarios and applications of square root equations. Mastery of these concepts not only helps in academic settings but also in various real-world contexts where such mathematical operations are required.
Thank you for following along with this comprehensive guide. Keep practicing and exploring, and you will find that simplifying square root equations becomes second nature.
Làm Thế Nào Để Đơn Giản Hóa Căn Bậc Hai
READ MORE:
Đơn Giản Hóa Căn Bậc Hai | Số Mũ, Căn Bậc Hai và Ký Hiệu Khoa Học | Tiền Đại Số | Khan Academy