Topic square root of 25/16: The square root of 25/16 might seem complex at first glance, but it's quite straightforward when broken down. In this guide, we will walk you through the steps to simplify and calculate the square root of this fraction, making it easy to understand and apply in various mathematical contexts.
Table of Content
- Square Root of 25/16
- Introduction to Square Roots
- Basic Concept of Square Roots
- Understanding Fractions in Square Roots
- Step-by-Step Calculation of Square Root of 25/16
- Simplifying the Fraction
- Finding the Square Root of Numerator and Denominator
- Combining the Results
- Properties of Square Roots
- Rational vs. Irrational Numbers
- Practical Applications of Square Roots
- Square Roots in Real Life Scenarios
- Using Calculators for Square Roots
- Common Mistakes and How to Avoid Them
- Conclusion
- YOUTUBE: Video hướng dẫn cách đơn giản hóa căn bậc hai của 25/16. Học cách giải thích và tính toán căn bậc hai một cách dễ dàng và hiệu quả.
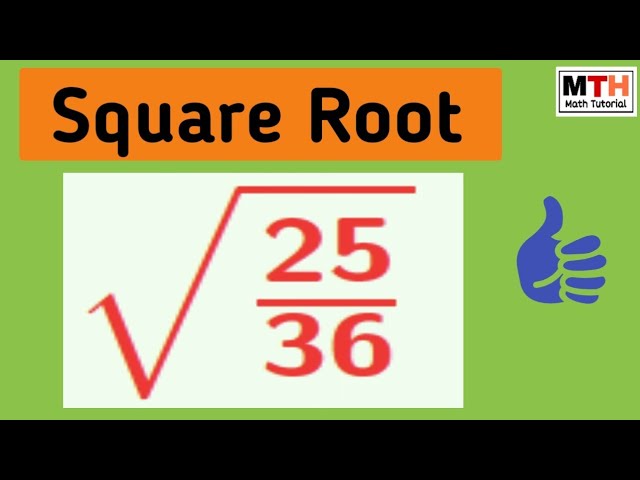
Square Root of 25/16
The square root of a fraction can be found by taking the square root of the numerator and the square root of the denominator separately.
Calculation
Given the fraction:
\[
\frac{25}{16}
\]
We can find its square root as follows:
- Find the square root of the numerator (25):
- \(\sqrt{25} = 5\)
- Find the square root of the denominator (16):
- \(\sqrt{16} = 4\)
Result
Therefore, the square root of \(\frac{25}{16}\) is:
\[
\sqrt{\frac{25}{16}} = \frac{5}{4}
\]
Decimal Representation
The decimal representation of \(\frac{5}{4}\) is:
\[
\frac{5}{4} = 1.25
\]
Thus, the square root of \(\frac{25}{16}\) can be expressed as \(\frac{5}{4}\) or 1.25 in decimal form.

READ MORE:
Introduction to Square Roots
Square roots are fundamental concepts in mathematics that help us understand the relationships between numbers. The square root of a number is a value that, when multiplied by itself, gives the original number. Mathematically, the square root of \( x \) is represented as \( \sqrt{x} \).
For example:
- \( \sqrt{16} = 4 \) because \( 4 \times 4 = 16 \)
- \( \sqrt{25} = 5 \) because \( 5 \times 5 = 25 \)
When dealing with fractions, the square root can be applied to both the numerator and the denominator separately. For instance, to find the square root of \( \frac{25}{16} \), we calculate:
- \( \sqrt{25} = 5 \)
- \( \sqrt{16} = 4 \)
Thus, the square root of \( \frac{25}{16} \) is:
\[
\sqrt{\frac{25}{16}} = \frac{\sqrt{25}}{\sqrt{16}} = \frac{5}{4}
\]
This principle allows us to simplify and understand more complex fractional square roots by breaking them down into simpler components. Square roots are widely used in various fields such as engineering, physics, and finance, making them an essential part of mathematical education.
Basic Concept of Square Roots
The square root of a number is a value that, when multiplied by itself, gives the original number. It is one of the most fundamental operations in mathematics, often represented by the radical symbol \( \sqrt{} \). For any positive number \( x \), the square root of \( x \) is written as \( \sqrt{x} \).
Here are some key points about square roots:
- Positive Numbers: Every positive number has two square roots: one positive and one negative. For example, \( \sqrt{9} = 3 \) and \( \sqrt{9} = -3 \) because \( 3 \times 3 = 9 \) and \( -3 \times -3 = 9 \).
- Zero: The square root of zero is zero: \( \sqrt{0} = 0 \).
- Negative Numbers: Negative numbers do not have real square roots. Their square roots are complex numbers. For example, \( \sqrt{-1} \) is represented as \( i \), where \( i \) is the imaginary unit.
When dealing with square roots of fractions, we can apply the square root to both the numerator and the denominator separately. For example, to find the square root of \( \frac{25}{16} \), we can follow these steps:
- Identify the numerator and denominator: \( 25 \) (numerator) and \( 16 \) (denominator).
- Calculate the square root of the numerator: \( \sqrt{25} = 5 \).
- Calculate the square root of the denominator: \( \sqrt{16} = 4 \).
- Combine the results: \( \sqrt{\frac{25}{16}} = \frac{\sqrt{25}}{\sqrt{16}} = \frac{5}{4} \).
This approach simplifies the process of finding square roots of fractions, making it easier to handle more complex calculations. Understanding the basic concept of square roots is essential for progressing in various areas of mathematics, including algebra, geometry, and calculus.
Understanding Fractions in Square Roots
When dealing with square roots, it's important to understand how to handle fractions. A fraction consists of a numerator and a denominator, and taking the square root of a fraction involves applying the square root to both parts separately. This process simplifies the expression and makes it easier to understand.
Let's break down the steps using the example of \( \frac{25}{16} \):
- Identify the numerator and denominator: In the fraction \( \frac{25}{16} \), 25 is the numerator and 16 is the denominator.
- Apply the square root to the numerator: Calculate \( \sqrt{25} \). Since \( 25 = 5 \times 5 \), we find that \( \sqrt{25} = 5 \).
- Apply the square root to the denominator: Calculate \( \sqrt{16} \). Since \( 16 = 4 \times 4 \), we find that \( \sqrt{16} = 4 \).
- Combine the results: After taking the square root of both the numerator and the denominator, we combine them to get the square root of the fraction:
\[
\sqrt{\frac{25}{16}} = \frac{\sqrt{25}}{\sqrt{16}} = \frac{5}{4}
\]
Understanding this method allows you to handle any fraction under a square root sign. Here are a few more examples to illustrate the process:
- \[ \sqrt{\frac{9}{4}} = \frac{\sqrt{9}}{\sqrt{4}} = \frac{3}{2} \]
- \[ \sqrt{\frac{1}{25}} = \frac{\sqrt{1}}{\sqrt{25}} = \frac{1}{5} \]
- \[ \sqrt{\frac{49}{36}} = \frac{\sqrt{49}}{\sqrt{36}} = \frac{7}{6} \]
This approach simplifies the calculation and provides a clear understanding of how to work with square roots of fractions. Mastering this technique is essential for solving more advanced mathematical problems involving square roots and fractions.
Step-by-Step Calculation of Square Root of 25/16
Calculating the square root of a fraction involves applying the square root to both the numerator and the denominator separately. Here, we will demonstrate this process step-by-step using the fraction \( \frac{25}{16} \).
- Identify the fraction: We start with the fraction \( \frac{25}{16} \).
- Separate the numerator and the denominator: The numerator is 25 and the denominator is 16.
- Calculate the square root of the numerator:
- Find \( \sqrt{25} \).
- Since \( 25 = 5 \times 5 \), we have \( \sqrt{25} = 5 \).
- Calculate the square root of the denominator:
- Find \( \sqrt{16} \).
- Since \( 16 = 4 \times 4 \), we have \( \sqrt{16} = 4 \).
- Combine the results:
- Combine the square roots of the numerator and the denominator to form the square root of the fraction.
- \[ \sqrt{\frac{25}{16}} = \frac{\sqrt{25}}{\sqrt{16}} = \frac{5}{4} \]
Thus, the square root of \( \frac{25}{16} \) is \( \frac{5}{4} \) or 1.25. This step-by-step approach helps simplify and accurately calculate the square roots of fractions, making it easier to handle similar problems in mathematics.

Simplifying the Fraction
Simplifying a fraction involves reducing it to its simplest form, where the numerator and denominator have no common factors other than 1. This process makes calculations easier and results more understandable. Here's how to simplify a fraction step-by-step, using \( \frac{25}{16} \) as an example:
- Identify the numerator and the denominator: In the fraction \( \frac{25}{16} \), 25 is the numerator and 16 is the denominator.
- Find the greatest common divisor (GCD):
- Determine the prime factors of the numerator (25): \( 25 = 5 \times 5 \).
- Determine the prime factors of the denominator (16): \( 16 = 2 \times 2 \times 2 \times 2 \).
- The numerator and the denominator have no common prime factors.
- Reduce the fraction (if possible):
- Since the GCD is 1, the fraction \( \frac{25}{16} \) is already in its simplest form.
- Confirm the fraction is simplified:
- Both 25 and 16 are perfect squares: \( 25 = 5^2 \) and \( 16 = 4^2 \).
- This confirms that the fraction is in its simplest form.
By ensuring that a fraction is simplified before performing further calculations, we make the math more manageable and the results clearer. In the case of \( \frac{25}{16} \), since it is already simplified, we can proceed directly to finding its square root.
Finding the Square Root of Numerator and Denominator
To find the square root of a fraction, we need to separately calculate the square roots of the numerator and the denominator. Let's go through this process step-by-step using the fraction \( \frac{25}{16} \):
- Identify the fraction:
We start with the fraction \( \frac{25}{16} \).
- Separate the numerator and the denominator:
The numerator is 25 and the denominator is 16.
- Calculate the square root of the numerator:
- Identify the square root of 25.
- Since \( 25 = 5 \times 5 \), we find that \( \sqrt{25} = 5 \).
- Calculate the square root of the denominator:
- Identify the square root of 16.
- Since \( 16 = 4 \times 4 \), we find that \( \sqrt{16} = 4 \).
- Combine the results:
- Now, we combine the square roots of the numerator and the denominator to find the square root of the fraction:
- \[ \sqrt{\frac{25}{16}} = \frac{\sqrt{25}}{\sqrt{16}} = \frac{5}{4} \]
By calculating the square roots of the numerator and the denominator separately, we simplify the process and ensure accurate results. Thus, the square root of \( \frac{25}{16} \) is \( \frac{5}{4} \) or 1.25.
Combining the Results
After finding the square roots of the numerator and the denominator separately, the next step is to combine these results to determine the square root of the entire fraction. Let's review this process using the fraction \( \frac{25}{16} \).
- Identify the results:
- The square root of the numerator (25) is \( \sqrt{25} = 5 \).
- The square root of the denominator (16) is \( \sqrt{16} = 4 \).
- Combine the square roots:
To find the square root of the fraction \( \frac{25}{16} \), we combine the individual square roots of the numerator and the denominator:
\[
\sqrt{\frac{25}{16}} = \frac{\sqrt{25}}{\sqrt{16}} = \frac{5}{4}
\] - Interpret the result:
The combined result, \( \frac{5}{4} \), is the square root of the fraction \( \frac{25}{16} \). This can also be expressed as a decimal:
\[
\frac{5}{4} = 1.25
\] - Verify the result:
- To ensure accuracy, we can square the result to see if we get back the original fraction.
- \[ \left( \frac{5}{4} \right)^2 = \frac{5^2}{4^2} = \frac{25}{16} \]
Since squaring \( \frac{5}{4} \) returns \( \frac{25}{16} \), we confirm that our calculation is correct.
By combining the results of the square roots of the numerator and the denominator, we successfully find that the square root of \( \frac{25}{16} \) is \( \frac{5}{4} \) or 1.25. This method can be applied to any fraction to simplify the process of finding its square root.
Properties of Square Roots
The square root function has several important properties that are useful in various mathematical contexts. Understanding these properties helps in simplifying and solving square root-related problems.
- Non-Negativity: The square root of a non-negative number is always non-negative. This means for any real number \( x \geq 0 \), \( \sqrt{x} \geq 0 \).
- Product Property: The square root of a product is equal to the product of the square roots of each factor. Mathematically, this is expressed as:
\(\sqrt{a \cdot b} = \sqrt{a} \cdot \sqrt{b}\)
This property is particularly useful when dealing with large numbers or variables. - Quotient Property: The square root of a quotient is equal to the quotient of the square roots of the numerator and denominator. This can be written as:
\(\sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}}\)
This property is directly applicable to our example of \(\sqrt{\frac{25}{16}} = \frac{\sqrt{25}}{\sqrt{16}} = \frac{5}{4}\). - Power Property: The square root of a number can be expressed as that number raised to the power of one-half:
\(\sqrt{x} = x^{\frac{1}{2}}\)
- Sum and Difference: The square root function does not distribute over addition or subtraction. That is:
\(\sqrt{a + b} \neq \sqrt{a} + \sqrt{b}\)
\(\sqrt{a - b} \neq \sqrt{a} - \sqrt{b}\)
- Even and Odd Properties: For even integers \( n \), \( \sqrt{x^n} = x^{n/2} \) holds for all \( x \geq 0 \). For odd integers \( n \), \( \sqrt{x^n} = |x|^{n/2} \) applies.
- Square Roots of Negative Numbers: In the realm of real numbers, the square root of a negative number is not defined. However, in the complex number system, \( \sqrt{-x} = i\sqrt{x} \), where \( i \) is the imaginary unit.
These properties are fundamental to understanding and working with square roots in both pure and applied mathematics.

Rational vs. Irrational Numbers
Understanding the distinction between rational and irrational numbers is crucial when dealing with square roots. Let's break down the concepts:
- Rational Numbers: A rational number is any number that can be expressed as the quotient or fraction
p/qof two integers, wherepandqare integers andqis not zero. Rational numbers include integers, fractions, and terminating or repeating decimals. For example,25/16is a rational number because it can be expressed as a fraction of two integers. - Irrational Numbers: An irrational number cannot be expressed as a simple fraction. It is a number that has a decimal expansion that neither terminates nor repeats. Common examples of irrational numbers include
π(pi) and√2(the square root of 2).
When we find the square root of a fraction like 25/16, we need to determine whether the result is rational or irrational.
Let's calculate the square root of 25/16 step by step:
- Separate the Numerator and Denominator: The square root of
25/16can be expressed as the square root of the numerator divided by the square root of the denominator:√(25/16) = √25 / √16
- Calculate the Square Roots:
- The square root of 25 is 5:
√25 = 5 - The square root of 16 is 4:
√16 = 4
- The square root of 25 is 5:
- Combine the Results:
√25 / √16 = 5 / 4
Since both 5 and 4 are integers, the result 5/4 is a rational number. Thus, the square root of 25/16 is rational.
This example illustrates that the square root of a fraction is rational if both the numerator and the denominator are perfect squares. If either the numerator or the denominator is not a perfect square, the result would be an irrational number.
Practical Applications of Square Roots
Square roots are a fundamental concept in mathematics with numerous practical applications in various fields. Understanding and utilizing square roots can significantly impact our daily lives and numerous industries. Here are some key applications:
- Architecture and Engineering: Square roots are crucial in calculating distances and designing structures. For example, the Pythagorean theorem, which involves square roots, is used to determine the lengths of sides in right-angled triangles, ensuring the stability and aesthetic appeal of buildings and bridges.
- Finance: In finance, square roots help in determining the standard deviation of investment returns. The standard deviation is a measure of market risk, and its calculation involves the square root of the variance, which helps investors understand the volatility of their investments.
- Physics: Square roots are used in various physics formulas, particularly those involving speed, acceleration, and force. For example, the formula for the period of a pendulum \( T = 2\pi \sqrt{\frac{L}{g}} \) includes a square root, illustrating how the length of the pendulum and gravity affect its period.
- Computer Science: Algorithms often utilize square roots for optimization purposes, graphics rendering, and machine learning models. For instance, the Euclidean distance formula, which involves square roots, is used in clustering algorithms to measure the distance between points in space.
- Geography: Square roots are used in calculating distances between points on maps, aiding in navigation and travel planning. The haversine formula, which determines the shortest distance between two points on a sphere, includes square roots in its calculations.
These examples highlight the diverse and significant role of square roots beyond just theoretical mathematics, demonstrating their importance in practical, real-world scenarios.
Square Roots in Real Life Scenarios
Square roots are not just abstract mathematical concepts; they have practical applications in various real-life scenarios. Here are some ways square roots are used in everyday life:
-
Engineering and Construction:
Engineers and architects frequently use square roots when designing and constructing buildings, bridges, and other structures. For example, they might use the Pythagorean theorem, which involves square roots, to calculate the length of a side of a right triangle. This is crucial for ensuring the stability and safety of structures.
-
Physics:
In physics, square roots are used to calculate quantities such as velocity, acceleration, and force. For instance, the formula for the period of a pendulum involves the square root of its length. Understanding these concepts allows scientists to predict and analyze the behavior of physical systems.
-
Finance:
Square roots are used in finance to calculate the standard deviation of investment returns, which measures the risk or volatility of an investment. This helps investors make informed decisions about their portfolios.
-
Medicine:
Medical professionals use square roots in various calculations, such as determining dosages for medications. For example, the surface area of a human body can be estimated using the square root of height and weight, which is important for calculating the correct dosage of certain drugs.
-
Computer Graphics:
In computer graphics, square roots are used in algorithms for rendering images and animations. Calculations involving distances, lighting, and shading often require the use of square roots to create realistic graphics in video games and simulations.
Understanding the square root of can be particularly useful in these scenarios. For instance, knowing that or 1.25 can simplify calculations involving fractional distances or measurements, which are common in engineering and computer graphics.
Using Calculators for Square Roots
Calculators are invaluable tools for finding square roots, especially for complex numbers and fractions. Here’s a detailed guide on how to use a calculator to find the square root of a fraction, using the example of \(\sqrt{\frac{25}{16}}\).
-
Understanding the Calculation: The square root of a fraction can be determined by separately finding the square roots of the numerator and the denominator. For \(\sqrt{\frac{25}{16}}\), this means calculating \(\sqrt{25}\) and \(\sqrt{16}\) separately.
-
Using a Calculator:
- Turn on your calculator and select the square root function, often labeled as \(\sqrt{}\) or \(x^{\frac{1}{2}}\).
- First, enter the numerator (25). Press the square root function key to get the result: \(\sqrt{25} = 5\).
- Next, enter the denominator (16). Press the square root function key to get the result: \(\sqrt{16} = 4\).
-
Combining Results: Now, divide the square root of the numerator by the square root of the denominator: \(\frac{5}{4} = 1.25\).
-
Using Online Calculators: Online calculators simplify the process further. Simply input the fraction directly (e.g., 25/16) into the online square root calculator and it will provide the result, along with step-by-step explanations.
-
Verification: It’s a good practice to verify the result by squaring the answer. For \(\frac{5}{4}\), squaring gives \( (\frac{5}{4})^2 = \frac{25}{16} \), confirming the correctness of the calculation.
Calculators are user-friendly and provide quick results, ensuring accuracy in mathematical operations like finding square roots. Whether using a physical calculator or an online tool, the process is straightforward and efficient.

Common Mistakes and How to Avoid Them
When calculating the square root of a fraction like , several common mistakes can occur. Below, we outline these errors and provide steps to avoid them:
-
Incorrectly Simplifying the Fraction:
Many students try to simplify the fraction before taking the square root. However, it is already in its simplest form. Attempting to simplify it further can lead to incorrect results.
-
Forgetting to Apply the Square Root Separately:
Another common mistake is failing to apply the square root to both the numerator and the denominator separately. The correct approach is:
-
Incorrectly Identifying Perfect Squares:
Ensure that you correctly identify perfect squares. In the fraction , both 25 and 16 are perfect squares. Thus, their square roots are integers (5 and 4, respectively).
-
Not Simplifying Radicals Properly:
For non-perfect square fractions, ensure proper simplification. For example, should be simplified as:
Steps to Avoid Common Mistakes
-
Verify the Simplification:
Always double-check if the fraction is already in its simplest form before proceeding.
-
Apply the Square Root Separately:
Use the property to simplify the process.
-
Identify Perfect Squares:
Recognize and correctly identify perfect squares for easier calculation.
-
Simplify Radicals Properly:
Ensure accurate simplification of radicals and rationalize the denominator when necessary.
Conclusion
Understanding the square root of a fraction such as \( \frac{25}{16} \) can seem challenging at first, but with a clear, step-by-step approach, it becomes manageable and even straightforward. Let's summarize the key points we've covered:
- Introduction to Square Roots: We started by understanding the basic concept of square roots, which are values that, when multiplied by themselves, give the original number.
- Understanding Fractions in Square Roots: We learned that taking the square root of a fraction involves taking the square root of the numerator and the denominator separately.
- Simplifying the Fraction: The fraction \( \frac{25}{16} \) was simplified by identifying that both 25 and 16 are perfect squares.
- Finding the Square Root of Numerator and Denominator: The square root of 25 is 5, and the square root of 16 is 4.
- Combining the Results: By combining the results of the square roots, we found that the square root of \( \frac{25}{16} \) is \( \frac{5}{4} \).
- Properties of Square Roots: We reviewed some important properties of square roots, including how they interact with fractions and the concept of rational versus irrational numbers.
- Practical Applications: Finally, we explored how square roots are used in real-life scenarios and why understanding these concepts is valuable.
In conclusion, the process of finding the square root of \( \frac{25}{16} \) is a clear demonstration of how mathematical principles can be applied methodically to simplify and solve problems. By breaking down the problem into smaller, manageable steps, we can approach even complex mathematical tasks with confidence and clarity. Whether dealing with pure mathematics or practical applications, the ability to understand and manipulate square roots is a fundamental skill that enhances our problem-solving capabilities.
Video hướng dẫn cách đơn giản hóa căn bậc hai của 25/16. Học cách giải thích và tính toán căn bậc hai một cách dễ dàng và hiệu quả.
1 25 16 G ĐƠN GIẢN HÓA CĂN BẬC HAI #1
READ MORE:
Video này sẽ giúp bạn hiểu rõ cách tính căn bậc hai của phân số 25/16, cách tìm căn bậc hai của phân số hoặc phép chia một cách dễ hiểu và chi tiết.
Căn Bậc Hai của 25/16, Căn Bậc Hai của Phân Số. Căn của một Phân Số hoặc Phép Chia